following functions, where x represents the number of radios produced and sold. C(x)=650,000+45x,R(x)=70x Find and interpret (R-C)(13,000),(R-C)(26,000), and (R-C)(39,000).
Answers
The expression (R-C)(13,000), (R-C)(26,000), and (R-C)(39,000) represent the difference between the revenue and the cost of producing and selling a certain number of radios. The values need to be calculated based on the given functions.
1. Calculate the cost function C(x) using the given equation: C(x) = 650,000 + 45x.
- For (R-C)(13,000): C(13,000) = 650,000 + 45(13,000) = 650,000 + 585,000 = 1,235,000.
- For (R-C)(26,000): C(26,000) = 650,000 + 45(26,000) = 650,000 + 1,170,000 = 1,820,000.
- For (R-C)(39,000): C(39,000) = 650,000 + 45(39,000) = 650,000 + 1,755,000 = 2,405,000.
2. Calculate the revenue function R(x) using the given equation: R(x) = 70x.
- For (R-C)(13,000): R(13,000) = 70(13,000) = 910,000.
- For (R-C)(26,000): R(26,000) = 70(26,000) = 1,820,000.
- For (R-C)(39,000): R(39,000) = 70(39,000) = 2,730,000.
3. Calculate the difference (R-C) for each scenario.
- For (R-C)(13,000): (R-C)(13,000) = R(13,000) - C(13,000) = 910,000 - 1,235,000 = -325,000.
- For (R-C)(26,000): (R-C)(26,000) = R(26,000) - C(26,000) = 1,820,000 - 1,820,000 = 0.
- For (R-C)(39,000): (R-C)(39,000) = R(39,000) - C(39,000) = 2,730,000 - 2,405,000 = 325,000.
Interpretation:
- (R-C)(13,000): The difference between revenue and cost at 13,000 radios sold is -$325,000. This implies that the company is experiencing a loss of $325,000 when selling 13,000 radios.
- (R-C)(26,000): The difference between revenue and cost at 26,000 radios sold is $0. This suggests that the revenue generated from selling 26,000 radios is exactly equal to the cost incurred.
- (R-C)(39,000): The difference between revenue and cost at 39,000 radios sold is $325,000. This indicates that the company has a profit of $325,000 when selling 39,000 radios.
Learn more about function : brainly.com/question/28278690
#SPJ11
Related Questions
Order the numbers from least to greatest.
1/8, 5/7, 0.35,0.39, 9/10
Answers
Answer:
1/8, 0.35, 0.39, 5/7, 9/10
Step-by-step explanation:
1/8 = 0.125
5/7 = 0.714
0.350
0.390
9/10 = 0.900
now let's order them from least to greatest:
0.125 , 0.350 , 0.390 , 0.714 , 0.900
= 1/8, 0.35, 0.39, 5/7, 9/10
if you have trouble with these types of problems change them all into decimals which will make things easier!
:D
I need help showing my work

Answers
Hope this was helpful

what is 6.729 x 8.3 (show ur work thanks)
Answers
Answer:
55.8507
Step-by-step explanat
55.8507 is the answer
hope i helped ;D
If x equals 5 and y equals negative 2 evaluate following expressions 7(2x-3y)
Answers
Answer:
given
x=5
y= -2
expression :-> 7(2(5)-3(-2))
7(10+6)
7 * 16
112
therefore 112 is ur ans
If x equals 5 and y equals negative 2 evaluate following expressions 7(2x-3y)
Answer:-112
Explanation:-Please look at the attached picture :)

If Dan was 1 year old 17 years ago, how old will he be 9 years from now?
Answers
Answer:
27
Step-by-step explanation:
1+17=18 and then 18+9=27
Answer: 27 years old
Step-by-step explanation: 1 + 17 = 18. He is 18 years old now.
18 + 9 = 27. He will be 27 years old then.
simultaneous eqations 3x + y = 7 2x + y = 6
Answers
Answer:
Hey there!
3x+y=7
2x+y=6
3x+y=7
-2x-y=-6
Add these two equations vertically so the y terms cancel out (this is known as the elimination method)
x=1
3x+y=7
3(1)+y=7
3+y=7
y=4
(x=1, y=4)
Hope this helps :)
4. Allison drew a triangle with 2 congruent sides and 1 obtuse angle. Which terms accurately describe the triangle? Select all that apply. A. Acute B. Isosceles C. Obtuse D. Scalene
Answers
Answer: I have been asked the same question I believe that it is obtuse and isosceles.
Step-by-step explanation: Well a triangle with 1 obtuse angle means it is an obtuse triangle but a triangle with 2 congruent means it is an isosceles triangle.
This angle is isosceles as well as obtuse.
What is an isosceles triangle?
Isosceles triangle is a triangle that has two equal sides.
What is an obtuse-angled triangle?
An obtuse-angled triangle is a triangle that consists of an interior angle of more than a right-angle (90)°.
As this triangle has two congruent side, it is an isosceles triangle.
Similarly, it has one obtuse angle. Therefore, this triangle is an obtuse-angled triangle.
Hence, for this triangle, B. Isosceles C. Obtuse both apply.
Learn more about isosceles and obtuse-angled triangle here: https://brainly.com/question/9872652
#Tag #SPJ2
Please help me I need to finish this :)

Answers
Carl surveyed his classmates about their favorite colors. The graph shows the survey results. A circle graph titled Favorite Colors. 50 percent is blue, 25 percent is orange, 25 percent is green. Which statements about the circle graph are correct? Check all that apply.
25% of the students said blue was their favorite color.
33% of the students said green was their favorite color.
25% of the students said orange was their favorite color.
50% of the students said either green or orange was their favorite color.
66% of the students said either orange or blue was their favorite color.
Answers
Answer: 25% of the students said orange was their favorite color.
50% of the students said either green or orange was their favorite color.
Step-by-step explanation:
The statements about the circle graph are correct;
C. 25% of the students said orange was their favorite color.
D. 50% of the students said either green or orange was their favorite color.
What is a circle?A circle is a shape consisting of all points in a plane that are given the same distance from a given point called the center.
Given that circle graph titled Favorite Colors. 50 percent is blue, 25 percent is orange, 25 percent is green.
In total, there are 100 students.
50 %is blue,
25 %is orange,
25 %is green.
Therefore, 25% of the students said orange was their favorite color and 50% of the students said either green or orange was their favorite color.
More can be learned about circle graph at;
https://brainly.com/question/16483602
#SPJ2
How many square feet are there in a rectangular-shaped building that is 34 feet wide and 72 feet long?
Answers
The area of the rectangular-shaped building is 2448 square feet.
What is the area of the rectangle?The space occupied by any two-dimensional figure in a plane is called the area. The space occupied by the rectangle in a two-dimensional plane is called the area of the rectangle.
Given that a rectangular-shaped building that is 34 feet wide and 72 feet long. The area of the rectangle will be calculated as below:-
Area of rectangle = Length x width
Area of rectangle = 34 x 72
Area of rectangle = 2448 square feet
Therefore, the area of the rectangular-shaped building is 2448 square feet.
To know more about the area follow
https://brainly.com/question/25292087
#SPJ4
Which is the graph of y =(2/x)+2

Answers
Answer: The correct answer is B.
Question 8
Find the value of "X". Round to the nearest tenth.

Answers
Answer:
8
Step-by-step explanation:
The intersecting chord theorem is expressed as;
7 * x = 4 * 14
7x = 56
Divide both sides by 7
7x/7 = 56/7
x = 8
Hence the value of x is 8
e. Miss Arnold opened a checking account
with her first paycheck of $1,287.95.
Her bank charges a service fee of $5 per
month plus 25¢ per check if the account
balance is less than $1,500. The bank
charged her $17.50 for 200 checks.
During the first month, she wrote checks
for $140, $123.74, $143.78, and $12.97.
If no deposits were made, what was
her balance at the end of the month?
please help
Answers
Miss Arnold's balance at the end of the month is $812.46.
To calculate Miss Arnold's balance at the end of the month, we need to consider her initial deposit, the service fee, check charges, and the amounts she wrote checks for.
Initial Deposit: Miss Arnold opened her account with her first paycheck of $1,287.95.
Service Fee: The bank charges a service fee of $5 per month. Therefore, her balance is reduced by $5.
Check Charges: The bank charges 25¢ per check if the account balance is less than $1,500. Miss Arnold was charged $17.50 for 200 checks, so each check cost $0.25. Thus, the total check charges can be calculated as follows:
Total check charges = Number of checks × Cost per check
Total check charges = 200 × $0.25 = $50
Check Amounts: Miss Arnold wrote checks for $140, $123.74, $143.78, and $12.97. We need to subtract the sum of these check amounts from her balance.
Check amounts = $140 + $123.74 + $143.78 + $12.97 = $420.49
Now, let's calculate Miss Arnold's balance at the end of the month:
Initial balance = $1,287.95
Service fee = -$5.00
Check charges = -$50.00
Check amounts = -$420.49
Balance at the end of the month = Initial balance + Service fee + Check charges + Check amounts
Balance at the end of the month = $1,287.95 - $5.00 - $50.00 - $420.49
Balance at the end of the month = $812.46
Therefore, Miss Arnold's balance at the end of the month is $812.46.
For more such questions on balance , Visit:
https://brainly.com/question/23447356
#SPJ11
explain the difference between the reciprocal of a function and the inverse of a function. why must the domains of the sine, cosine, and tangent functions be restricted in order to define their inverse functions? be specific. provide examples and graphs to support your answers.3
Answers
The main difference between the reciprocal and inverse of a function is their definition and properties. The reciprocal of a function f(x) is defined as 1/f(x), while the inverse of a function f(x) is a function f^(-1)(x) such that \(f(f^(-1)(x))=x and f^(-1)(f(x))=x\) for all x in their respective domains.
The domains of sine, cosine, and tangent functions need to be restricted to define their inverse functions because they are not one-to-one functions. In order to have an inverse, a function must be both one-to-one (each output has only one input) and onto (each output value is mapped by at least one input value).
For example:
- sine: restricted domain to [-π/2, π/2] to define its inverse, arcsin (sin^(-1))
- cosine: restricted domain to [0, π] to define its inverse, arccos (cos^(-1))
- tangent: restricted domain to (-π/2, π/2) to define its inverse, arctan (tan^(-1))
These restrictions ensure that the functions become one-to-one and onto, making it possible to define their inverses uniquely.
for such more questions on function
https://brainly.com/question/11624077
#SPJ11
um someone pls help me

Answers
Answer:
s : m : l
2u : 3u : 7u
small bag=15kg
medium bag=22.5kg
large bag=52.5kg
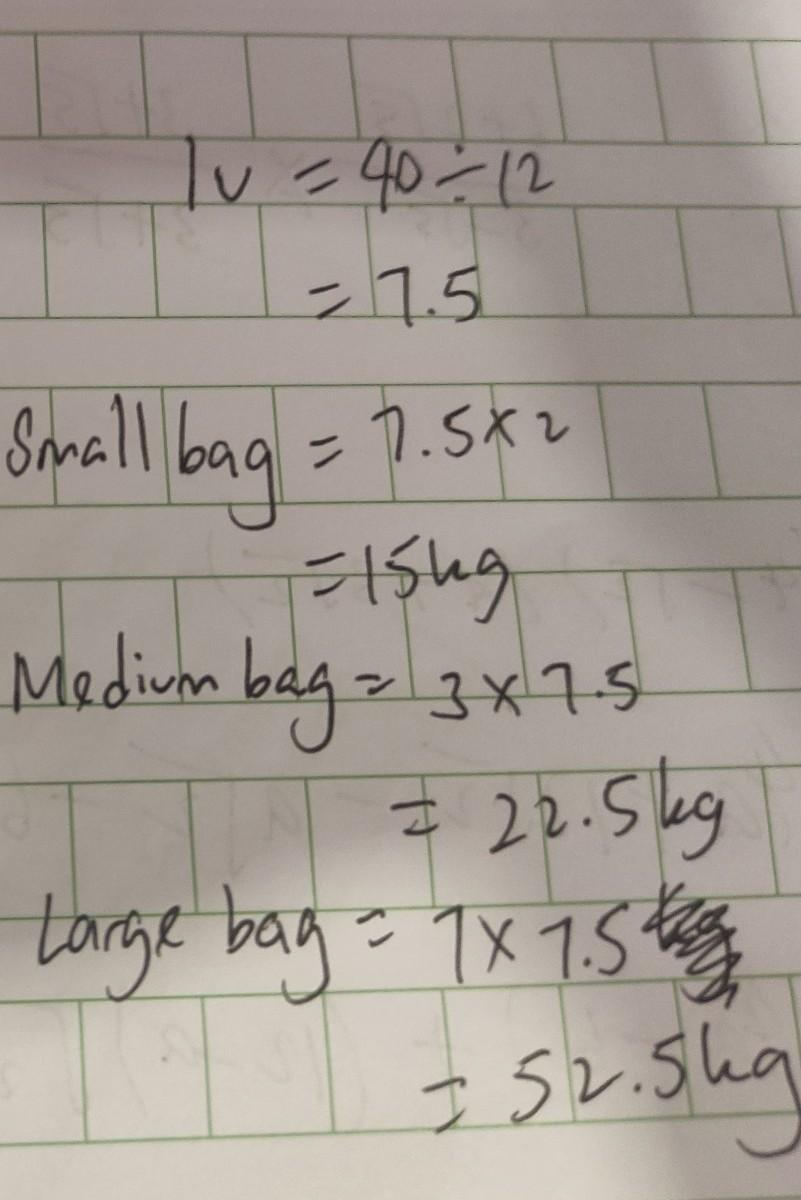
can anyone help me plzz :)

Answers
Answer:
your answer is 3
Step-by-step explanation:
36 divide by 3 = 12 and 12 divide by 2 = 6 and 6 divide by 2 = 3
PLEASE ANSWER WILL GIVE BRAINLIEST IF CORRECT!!!
The triangle below is equilateral. Find the length of side x to the nearest tenth.

Answers
Answer:
5.7
Step-by-step explanation:
Equilateral triangle means all the sides are the same length. So each side is basically \(\sqrt{11}\) times 2
After we know that, we then look to the Pythagorean theorum:
a^2 + b^2 = c^2
Before that, we have to find what the length of the hypotenuse is. 90 degrees equals \(\sqrt{11}\) times 2. So plug this info into the equation:
\(\sqrt{11}\)^2 + b^2 = \(2\sqrt{11}\)^2
11 + b^2 = 4(11)
11 + b^2 = 44
b^2 = 33
b = \(\sqrt{33}\)
b = 5.744 = 5.7
Plssss help
I need AC

Answers
Answer:
AC = 18
Step-by-step explanation:
Using the cosine ratio in the right triangle and the exact value
cos30° = \(\frac{\sqrt{3} }{2}\) , then
cos30° = \(\frac{adjacent}{hypotenuse}\) = \(\frac{AC}{AB}\) = \(\frac{AC}{12\sqrt{3} }\) = \(\frac{\sqrt{3} }{2}\) ( cross- multiply )
2 AC = 12\(\sqrt{3}\) × \(\sqrt{3}\) = 12 × 3 = 36 ( divide both sides by 2 )
AC = 18
∠ACB=90° ⇒ ΔABC is right triangle
AB is hypotenuse, ∠BAC=30° ⇒
\(CB=\dfrac{AB}{2}=\dfrac{12\sqrt{3} }{2} =6\sqrt{3}\)
Let's use Pythagorean theorem
\(AB^2=BC^2+AC^2\\AC^2=AB^2-BC^2\\AC^2=(12\sqrt{3})^2-(6\sqrt{3} )^2\\AC=\sqrt{(12\sqrt{3}-6\sqrt{3})(12\sqrt{3}+6\sqrt{3}))} \\AC=\sqrt{6\sqrt{3}\times18\sqrt{3} } \\AC=\sqrt{3\times6\times18} \\AC=18\)
A real estate agent claims that the average price of a condominium in Naples, Florida, is at most $50,000. The standard deviation is s = $8,500. A sample of 81 condominiums has an average selling price of $51,500. Use a = 0.10 level of significance to test the claim.
H0:
H1:
Test Statistic:
P-value:
Decision:
Answers
In this hypothesis test, we want to determine whether the average price of a condominium in Naples, Florida is at most $50,000.
H0: The average price of a condominium in Naples, Florida is $50,000 or less.
H1: The average price of a condominium in Naples, Florida is greater than $50,000.
To calculate the test statistic, we can use the formula:
test statistic = (sample mean - hypothesized mean) / (standard deviation / sqrt(sample size))
Given that the sample mean is $51,500, the hypothesized mean is $50,000, the standard deviation is $8,500, and the sample size is 81, we can substitute these values into the formula to calculate the test statistic.
The p-value represents the probability of obtaining a sample mean as extreme as the observed one, assuming the null hypothesis is true. To determine the p-value, we will use the test statistic and the appropriate distribution (in this case, the t-distribution).
Based on the p-value and the significance level of 0.10, we will make a decision. If the p-value is less than 0.10, we will reject the null hypothesis in favor of the alternative hypothesis. If the p-value is greater than or equal to 0.10, we will fail to reject the null hypothesis.
In the decision, we will conclude whether there is enough evidence to support the claim that the average price of a condominium in Naples, Florida is at most $50,000, based on the calculated p-value and the chosen significance level.
Learn more about statistic here:
https://brainly.com/question/32237714
#SPJ11
1 0points pls solve this small math

Answers
Answer:
your answer should be A
have a good day:)
Step-by-step explanation:
Answer: A.
Step-by-step explanation: I did all of the work in my notebook this is too easy have a good rest of your day.
Just a question about the problem, dont need the answer if you dont want to solve it, but would 15 be negative? Thats all, Thanks!
\(f(x)=x^{2} +15x+3\)

Answers
Answer:
\(x = -\frac{15}{2}\)
Step-by-step explanation:
Hello!
The formula for the AOS is given to you, and you simply have to plug in the information given by the equation.
Equation of a Parabola (Standard): \(y = ax^2 + bx + c\)
Comparing it to our equation:
a = 1b = 15c = 3Plug it in to the AOS formula:
\(x = \frac{-b}{2a}\)\(x = \frac{-15}{2(1)}\)\(x = -\frac{15}{2}\)You only have to input 15 in to the correct box since the negative sign is already placed for you.
Triniti had $500 in a savings account at the
beginning of the summer. She wants to
have at least $200 in the account by the
end of the summer. She withdraws $25
each week for food, clothes, and movie
tickets. How many weeks can Triniti
withdraw money? Write an inequality you
could use to represent this problem.
please hurry
Answers
Answer: 12
Step-by-step explanation: by the end of 12 weeks shell have 200 dollars left
Find the limit. Use l'Hospital's Rule where appropriate. If there is a more elementary method, consider using it. lim [In(x9 - 1) - In(x5- 1)]
Answers
The limit of the given expression as x approaches 1 from the right is 1.8.
To evaluate the limit of the given expression:
\(lim_{x - > 1} + [ln(x ^ 9 - 1) - ln(x ^ 5 - 1)]\)
We can start by directly substituting x = 1 into the expression:
[ln(1⁹ - 1) - ln(1⁵ - 1)]
= [ln(0) - ln(0)]
However, ln(0) is undefined, so this approach doesn't provide a meaningful answer.
To apply L'Hôpital's Rule, we need to rewrite the expression as a fraction and differentiate the numerator and denominator separately. Let's proceed with this approach:
\(lim_{x - > 1}\)+ [ln(x⁹ - 1) - ln(x⁵ - 1)]
= \(lim_{x - > 1}\)+ [ln((x⁹ - 1)/(x⁵ - 1))]
Now, we can differentiate the numerator and denominator with respect to x:
Numerator:
d/dx[(x⁹ - 1)] = 9x⁸
Denominator:
d/dx[(x⁵ - 1)] = 5x⁴
Taking the limit again:
\(lim_{x - > 1}\)+ [9x⁸ / 5x⁴]
= \(lim_{x - > 1}\)+ (9/5) * (x⁸ / x⁴)
= (9/5) * \(lim_{x - > 1}\)+ (x⁸ / x⁴)
Now, we can substitute x = 1 into the expression:
(9/5) * \(lim_{x - > 1}\)+ (1⁸ / 1⁴)
= (9/5) * \(lim_{x - > 1}\)+ 1
= (9/5) * 1
= 9/5
= 1.8
The complete question is:
Find the limit. Use l'Hospital's Rule where appropriate. If there is a more elementary method, consider using it. \(lim_{x - > 1} + [ln(x ^ 9 - 1) - ln(x ^ 5 - 1)]\)
To know more about limit:
https://brainly.com/question/12211820
#SPJ4
Is 80, 150, 170 a pythagorean triple?
Answers
Answer:
Yes
Step-by-step explanation:
80² + 150² = 170²
6400 + 22500 = 28900
28900 = 28900
I have 12 black socks and 12 white socks in a drawer. In complete darkness how many socks would I have to grab out to make sure I got a matching pair?
Answers
Answer:
3 socks
Step-by-step explanation:
The number of socks in the box does not matter. There are only 2 choices of socks in the box. So, if you take out 3 random socks from the box, you're guaranteed a pair.
solve the problem. a basketball player makes approximately 69% of free throws. if she plays in a game in which she shoots 7 free throws, what is the probability the she will make all 7?
Answers
If she plays in a game in which she shoots 7 free throws, the probability that she will make all 7 = 0.0188
For given question,
a basketball player makes approximately 69% of free throws.
So, the probability of success (p) = 0.69
the probability of failure (q) = 0.31
She plays in a game in which she shoots 7 free throws.
So, n = 7
We need to find the probability that she will make all 7.
Using Binomial probability Distribution,
For x = 7,
\(P(x = 7) =~ ^7C_7\times (0.67)^7\times (0.31)^{7-7}\\\\P(x=7)= 1\times 0.0606\times 0.31\\\\P(x=7)=0.0188\)
Therefore, if she plays in a game in which she shoots 7 free throws, the probability that she will make all 7 = 0.0188
Learn more about the Binomial probability Distribution here:
https://brainly.com/question/15902935
#SPJ4
find the area of the region which is bounded by the polar curves θ=π θ=π and r=10θ, 0≤θ≤1.5π r=10θ, 0≤θ≤1.5π
Answers
The area of the region bounded by the polar curves θ = π and r = 10θ, 0≤θ≤1.5π is 2603.54 square units.
The polar curve θ = π is a vertical line passing through the point (-π, y) in Cartesian coordinates, where y can take any value. The polar curve r = 10θ can be rewritten as θ = r/10, so it represents a spiral that starts at the origin and winds around the pole as θ increases.
To find the region bounded by these curves, we need to find the points where they intersect. When θ = π, we have r = 10π. When r = 10θ, we have θ = π/10. These two polar coordinates correspond to the point (-10π, 0) and (π/10, 10π/10), respectively.
We can now calculate the area of the region using the formula:
A = (1/2) ∫[a,b] (f(θ)*f(θ)) dθ
where a and b are the angles of the region, and f(θ) is the distance from the pole to the curve at angle θ.
In this case, the angles are a = π/10 and b = π, and the distance from the pole to the curve r = 10θ is simply r = 10θ. Therefore, the area is:
A = (1/2) ∫[π/10,π] (10θ)*(10θ) dθ
= (1/2) ∫[π/10,π] 100θ*θ dθ
= (1/2) [ (100/3) \(\pi ^{3}\) - (100/3) (π/10)^3 ]
= 4975π/6
So the area of the region bounded by the polar curves θ = π and r = 10θ, 0≤θ≤1.5π is approximately 2603.54 square units.
To learn more about area here:
https://brainly.com/question/32070352
#SPJ4
You choose a marble at random from a bag containing:
3 blue marbles
7 red marbles
4 green marbles
What is the probability that you pick a red marble, replace it, and then pick a
green marble?
Express your answer in percentage form, round to the nearest tenth.
Answers
Answer: 14.3%
Step-by-step explanation:
3 blue
7 red
4 green
14 total
pick a red: 7/14
replace it
pick a green: 4/14
7/14*4/14 = 1/7 or 14.285714%
rounded that is 14.3%
The given planes intersect in a line. Find parametric equations for the line of intersection. [Hint: The line of intersection consists of all points (x, y, z) that satisfy both equations. Solve the system and designate the unconstrained variable as t .]
x + 2y + z = 1, 2x+5y + 32 = 4
Answers
The parametric equations for the line of intersection are:
x = 61 - 5t
y = 2t - 30
z = t
To find the parametric equations for the line of intersection of the given planes, we first need to solve the system of equations:
1. x + 2y + z = 1
2. 2x + 5y + 32 = 4
Step 1: Solve for x from equation 1:
x = 1 - 2y - z
Step 2: Substitute x in equation 2 with the expression found in step 1:
2(1 - 2y - z) + 5y + 32 = 4
Now we can use elimination to solve for one variable. Let's eliminate y by multiplying the first equation by 5 and subtracting it from the second equation:
Step 3: Simplify and solve for y:
2 - 4y - 2z + 5y + 32 = 4
y - 2z = -30
Step 4: Designate z as the parameter t:
z = t
Step 5: Substitute z with t in the expression for y:
y = 2t - 30
Step 6: Substitute z with t in the expression for x:
x = 1 - 2(2t - 30) - t
x = 1 - 4t + 60 - t
x = 61 - 5t
Now we have the parametric equations for the line of intersection:
x = 61 - 5t
y = 2t - 30
z = t
Note that we can choose any value of z for the parameter t, since z is unconstrained.
Learn more about Parametric:
brainly.com/question/15585522
#SPJ11
Korie randomly selects a number from the numbers shown below 54, 60, 11, 0, 5, 7, 20
what is the probability that the number she selected is an even number
Answers
Answer:
I think its 3 out of 7