Find the missing factor.
80 X
640.000
Answers
640 / 80 =. 8
80 x 8 =. 640
Related Questions
Solve the system of equations:
x+4y-z=6
2x+11y+4z=9
x+5y+z=5
Answers
2.) x= -11/2y - 2z + 9/2
3.) x= -5y - z + 5
A parallelogram has sides measuring 10 and 18, and an angle measuring 100 degrees. What is its area?
Answers
Answer:
180
Step-by-step explanation:
The area of a parallelogram is given by the formula A = b × h, where b is the base and h is the height. In this case, the base is 10 and the height is 18. Therefore, the area is:
A=10×18
A=180
The area of the parallelogram is 180 square units
Like nobody is helpping me i been postting the same questions alot of time what are you doing yall want to to answer what yall want to answer i post the easy question that yall can and it ju0st like. who ever want to help there are questions on my page they are easy im telling you! 50 pts for who ever do and brainliest
Answers
Answer:
Sure if they are not too hard
Step-by-step explanation:
how much money deposited now will provide payment of Rs. 15000 at the end of each half year for 10 years, if interest is 16% compounded six-monthly
Answers
The interest is 16% compounded semi-annually, is Rs. 121,179.10.
To determine how much money needs to be deposited now to provide a payment of Rs. 15,000 at the end of each half year for 10 years, we will use the formula for the present value of an annuity.
Present value of an annuity = (Payment amount x (1 - (1 + r)^-n))/rWhere:r = interest rate per compounding periodn = number of compounding periodsPayment amount = Rs. 15,000n = 10 x 2 = 20 (since there are 2 half years in a year and the payments are made for 10 years)
So, we have:r = 16%/2 = 8% (since the interest is compounded semi-annually)Payment amount = Rs. 15,000Using the above formula, we can calculate the present value of the annuity as follows:
Present value of annuity = (15000 x (1 - (1 + 0.08)^-20))/0.08 = Rs. 121,179.10Therefore, the amount that needs to be deposited now to provide payment of Rs. 15,000 at the end of each half year for 10 years, if the interest is 16% compounded semi-annually, is Rs. 121,179.10.
For more such questions on semi-annually
https://brainly.com/question/30573341
#SPJ8
Answer all questions please

Answers
Answer:
a) f(1) = 3
b) f(-1) = -0.25
c) x = 0, 3
d) x = -0.75
e) domain [-2, 4]
range [-1, 3]
Step-by-step explanation:
In this graph, f(x) is represented by the y-axis.
a) We can see that f(1) (the y-value when x = 1) is 3.
b) Looking at the graph, we can estimate f(-1) as about -0.25.
c) We need to find the x-values when the y-axis is at 1. We can see that there are two points at x = 0, 3.
d) Looking at the graph, we can estimate that f(x) = 0 around when x = -0.75.
e) The domain of a function is its set of x-values. We can see that f(x) spans from x = -2 to x = 4. That range in interval notation is: [-2, 4]
The range of a function is its set of y-values. We can see that f(x) spans from y = -1 to y = 3. That range in interval notation is: [-1, 3].
Plot the intercepts to graph the equation: 4x-2y=4
Answers
Answer:
•x-intercept = 1
•y-intercept = -2
Explanation:
Given the linear equation
\(4x-2y=4\)x-intercept
The x-intercept is the value where the line crosses the x-axis.
We calculate this by setting y=0
\(\begin{gathered} \text{When y=0} \\ 4x-2y=4 \\ 4x-2(0)=4 \\ 4x-0=4 \\ 4x=4 \\ \text{Divide both sides by 4} \\ \frac{4x}{4}=\frac{4}{4} \\ x=1 \end{gathered}\)The x-intercept is 1.
We have the point (1, 0)
y-intercept
The y-intercept is the value where the line crosses the y-axis.
We calculate this by setting x=0
\(\begin{gathered} \text{When x=0} \\ 4x-2y=4 \\ 4(0)-2y=4 \\ -2y=4 \\ \text{Divide both sides by -2} \\ -\frac{2y}{-2}=\frac{4}{-2} \\ y=-2 \end{gathered}\)The y-intercept is -2.
We have the point (0, -2)
We then plot the points on the graph below:

Help I Dont Understand?

Answers
How many 9/4 hours are there in 3/4 hour?
Answers
Notice that:
\(\frac{3}{4}=\frac{1}{3}\times\frac{9}{4}.\)Therefore, there are 1/3 of 9/4 hours in 3/4 hours.
Answer:
\(\frac{1}{3}.\)For questions 3-4, use the graph of the polynomial function to find the factorization of the polynomial. Assume there is no constant term. 3

Answers
3. The factored polynomial is p(x) = (x - 1)(x - 5)
4. The factored polynomial is p(x) = (x + 3)²
What is a polynomial?A polynomial is a mathematical expression in which the power of the unknown is greater than or equal to 2.
3. To factorize the polynomial using the graph, we see that the polynomial cuts the x - axis at x = 1 and x = 5.
This implies that its factors are (x - 1) and (x - 5)
So, the factored polynomial is p(x) = (x - 1)(x - 5)
4. To factorize the polynomial using the graph, we see that the polynomial touches the x - axis at only one point x = -3. So,it has repeated roots
This implies that its factors are (x - (-3)) = (x + 3) twice
So, the factored polynomial is p(x) = (x + 3)²
Learn more about factorization of polynomial here:
brainly.com/question/30806486
#SPJ1
Shannon wants to buy 4 coffee mugs for the lowest amount.a set of 4 mugs costs £12
Answers
i can help, but you need to write the whole question..
Need help with this question. PLS helpppppp

Answers
Answer:
x = 0.39 or
x = -1.72
Step-by-step explanation:
The quadrateic formula is:
\(x = \frac{-b\pm\sqrt{b^2 - 4ac} }{2a}\)
eq: 3x² + 4x - 2
which is of the form ax² + bx + c = 0
where a = 3, b = 4 and c = -2
sub in quadratic formuls,
\(x = \frac{-4\pm\sqrt{4^2 - 4(3)(-2)} }{2(3)}\\\\=\frac{-4\pm\sqrt{16 + 24} }{6}\\\\=\frac{-4\pm\sqrt{40} }{6}\\\\=\frac{-4\pm2\sqrt{10} }{6}\\\\=\frac{-2\pm\sqrt{10} }{3}\\\\=\frac{-2+\sqrt{10} }{3} \;or\;=\frac{-2-\sqrt{10} }{3}\\\\=0.39 \;or\; -1.72\)
Assumptions: Tax depreciation is straight-line over three years. Pre-tax salvage value is 25 in Year 3 and 50 if the asset is scrapped in Year 2. Tax on salvage value is 40% of the difference between salvage value and book value of the investment. The cost of capital is 20%.
Answers
Based on the given assumptions and calculations, the net present value (NPV) of the investment in the new piece of equipment is -$27,045.76, indicating that the investment is not favorable.
To calculate the after-tax cash flows for each year and evaluate the investment decision, let's use the following information:
Assumptions:
Tax depreciation is straight-line over five years.
Pre-tax salvage value is $10,000 in Year 5 and $15,000 if the asset is scrapped in Year 4.
Tax on salvage value is 30% of the difference between salvage value and book value of the investment.
The cost of capital is 12%.
Given:
Initial investment cost = $50,000
Useful life of the equipment = 5 years
To calculate the depreciation expense each year, we divide the initial investment by the useful life:
Depreciation expense per year = Initial investment / Useful life
Depreciation expense per year = $50,000 / 5 = $10,000
Now, let's calculate the book value at the end of each year:
Year 1:
Book value = Initial investment - Depreciation expense per year
Book value \(= $50,000 - $10,000 = $40,000\)
Year 2:
Book value = Initial investment - (2 \(\times\) Depreciation expense per year)
Book value \(= $50,000 - (2 \times$10,000) = $30,000\)
Year 3:
Book value = Initial investment - (3 \(\times\) Depreciation expense per year)
Book value = $50,000 - (3 \(\times\) $10,000) = $20,000
Year 4:
Book value = Initial investment - (4 \(\times\) Depreciation expense per year)
Book value \(= $50,000 - (4 \times $10,000) = $10,000\)
Year 5:
Book value = Initial investment - (5 \(\times\) Depreciation expense per year)
Book value \(= $50,000 - (5 \times $10,000) = $0\)
Based on the assumptions, the salvage value is $10,000 in Year 5.
If the asset is scrapped in Year 4, the salvage value is $15,000.
To calculate the tax on salvage value, we need to find the difference between the salvage value and the book value and then multiply it by the tax rate:
Tax on salvage value = Tax rate \(\times\) (Salvage value - Book value)
For Year 5:
Tax on salvage value\(= 0.30 \times ($10,000 - $0) = $3,000\)
For Year 4 (if scrapped):
Tax on salvage value\(= 0.30 \times ($15,000 - $10,000) = $1,500\)
Now, let's calculate the after-tax cash flows for each year:
Year 1:
After-tax cash flow = Depreciation expense per year - Tax on salvage value
After-tax cash flow = $10,000 - $0 = $10,000
Year 2:
After-tax cash flow = Salvage value - Tax on salvage value
After-tax cash flow = $0 - $0 = $0
Year 3:
After-tax cash flow = Salvage value - Tax on salvage value
After-tax cash flow = $0 - $0 = $0
Year 4 (if scrapped):
After-tax cash flow = Salvage value - Tax on salvage value
After-tax cash flow = $15,000 - $1,500 = $13,500
Year 5:
After-tax cash flow = Salvage value - Tax on salvage value
After-tax cash flow = $10,000 - $3,000 = $7,000
Now, let's calculate the net present value (NPV) using the cost of capital of 12%.
We will discount each year's after-tax cash flow to its present value using the formula:
\(PV = CF / (1 + r)^t\)
Where:
PV = Present value
CF = Cash flow
r = Discount rate (cost of capital)
t = Time period (year)
NPV = PV Year 1 + PV Year 2 + PV Year 3 + PV Year 4 + PV Year 5 - Initial investment
Let's calculate the NPV:
PV Year 1 \(= $10,000 / (1 + 0.12)^1 = $8,928.57\)
PV Year 2 \(= $0 / (1 + 0.12)^2 = $0\)
PV Year 3 \(= $0 / (1 + 0.12)^3 = $0\)
PV Year 4 \(= $13,500 / (1 + 0.12)^4 = $9,551.28\)
PV Year 5 \(= $7,000 / (1 + 0.12)^5 = $4,474.39\)
NPV = $8,928.57 + $0 + $0 + $9,551.28 + $4,474.39 - $50,000
NPV = $22,954.24 - $50,000
NPV = -$27,045.76
The NPV is negative, which means that based on the given assumptions and cost of capital, the investment in the new piece of equipment would result in a net loss.
Therefore, the investment may not be favorable.
Please note that the calculations above are based on the given assumptions, and additional factors or considerations specific to the business should also be taken into account when making investment decisions.
For similar questions on assumptions.
https://brainly.com/question/15424356
#SPJ8
The complete question may be like :
Assumptions: Tax depreciation is straight-line over five years. Pre-tax salvage value is $10,000 in Year 5 and $15,000 if the asset is scrapped in Year 4. Tax on salvage value is 30% of the difference between salvage value and book value of the investment. The cost of capital is 12%.
You are evaluating an investment in a new piece of equipment for your business. The initial investment cost is $50,000. The equipment is expected to have a useful life of five years.
Using the given assumptions, calculate the after-tax cash flows for each year and evaluate the investment decision by calculating the net present value (NPV) using the cost of capital of 12%.
what is 3/5 Is it divison=÷
Answers
Answer:
0.6
Step-by-step explanation:
uhh, just divide?
Select the correct answer from each drop-down menu. Astronomers can use geometry to measure the objects in space and describe their
Please Help.

Answers
Astronomers can use geometry to measure the distance of objects in space and describe ttheir relationship.
Who are astronomers?It should be noted that astronomers study stars, planets, and other celestial bodies. It should be noted that they use ground-based equipment, like optical telescopes, and space-based equipment. Some astronomers study distant galaxies and phenomena such as black holes and neutron stars.
In this case, astronomers can use geometry to measure the distance of objects in space and describe ttheir relationship.
Learn more about distance on:
https://brainly.com/question/15445792
#SPJ1
Answer:
distance between, motion
Step-by-step explanation:
plato, I got it right
sin(x)-cos(x)/sin²(x)-cos²(x) = 1

Answers
The prove of the given trigonometric function is given below.
The given trigonometric function is,
(sin⁴(x) - cos⁴(x))/(sin²(x) - cos²(x))
Now proceed left hand side of the given expression:
We can write the expression as,
⇒[(sin²(x))² - (cos²(x))²]/(sin²(x) - cos²(x))
Since we know that ,
Algebraic identity:
a² - b² = (a-b)(a+b)
Therefore the above expression be
⇒(sin²(x) - cos²(x))(sin²(x) + cos²(x))/(sin²(x) - cos²(x))
⇒(sin²(x) + cos²(x))
Since we know that,
Trigonometric Identities come in handy when trigonometric functions are used in an expression or equation. Trigonometric identities hold for all values of variables on both sides of an equation. Geometrically, these identities include one or more trigonometric functions (such as sine, cosine, and tangent).
Then,
sin²(x) + cos²(x)² = 1 is an trigonometric identity
Hence,
(sin⁴(x) - cos⁴(x))/(sin²(x) - cos²(x)) = 1
Hence proved.
Learn more about the triangle visit;
brainly.com/question/1058720
#SPJ1
One final question, what would be the proper way to write this? I thought it was the last one answer but I’m not sure

Answers
Answer:
OMG NO- KEEP IT THAT WAY
You're already right! :)
A computer store sells an average of 3 laptops per week.
1. What is the probability that no laptops will be sold next week?
2. What is the probability that a laptop will be sold next week?
3. Assuming that the store only works from Saturday to Wednesday, then there are two days in which no computers are sold: what is the probability that tomorrow (Tuesday) only one laptop will be sold?
Answers
Answer:7%
Step-by-step explanation:the store is rapidly selling laptops
1. The probability that no laptops will be sold next week is very low, as the store has an average of 3 laptops sold per week. This probability can be calculated using the Poisson distribution, which is a probability distribution used to model the number of times an event occurs within a given time period. The probability of no events occurring within a given time period is given by the formula:P(x=0) = e^(-lambda)
Where lambda is the average number of events per time period. In this case, the probability that no laptops will be sold next week is:
P(x=0) = e^(-3) = 0.0498
2. The probability that a laptop will be sold next week can also be calculated using the Poisson distribution. The probability of at least one event occurring within a given time period is given by the formula:
P(x>=1) = 1 - P(x=0) = 1 - e^(-lambda)
In this case, the probability that a laptop will be sold next week is:
P(x>=1) = 1 - e^(-3) = 0.9502
3. The probability that only one laptop will be sold tomorrow (Tuesday) can be calculated using the Poisson distribution. The probability of x events occurring within a given time period is given by the formula:
P(x) = (lambda^x * e^(-lambda)) / x!
Where lambda is the average number of events per time period and x is the number of events being considered. In this case, the probability that only one laptop will be sold tomorrow is:
P(x=1) = (3^1 * e^(-3)) / 1! = 3 * e^(-3) = 0.224
Note that this probability assumes that the store sells laptops at a constant rate throughout the week, and does not take into account any variations in demand or other factors that may affect the number of laptops sold on a given day.
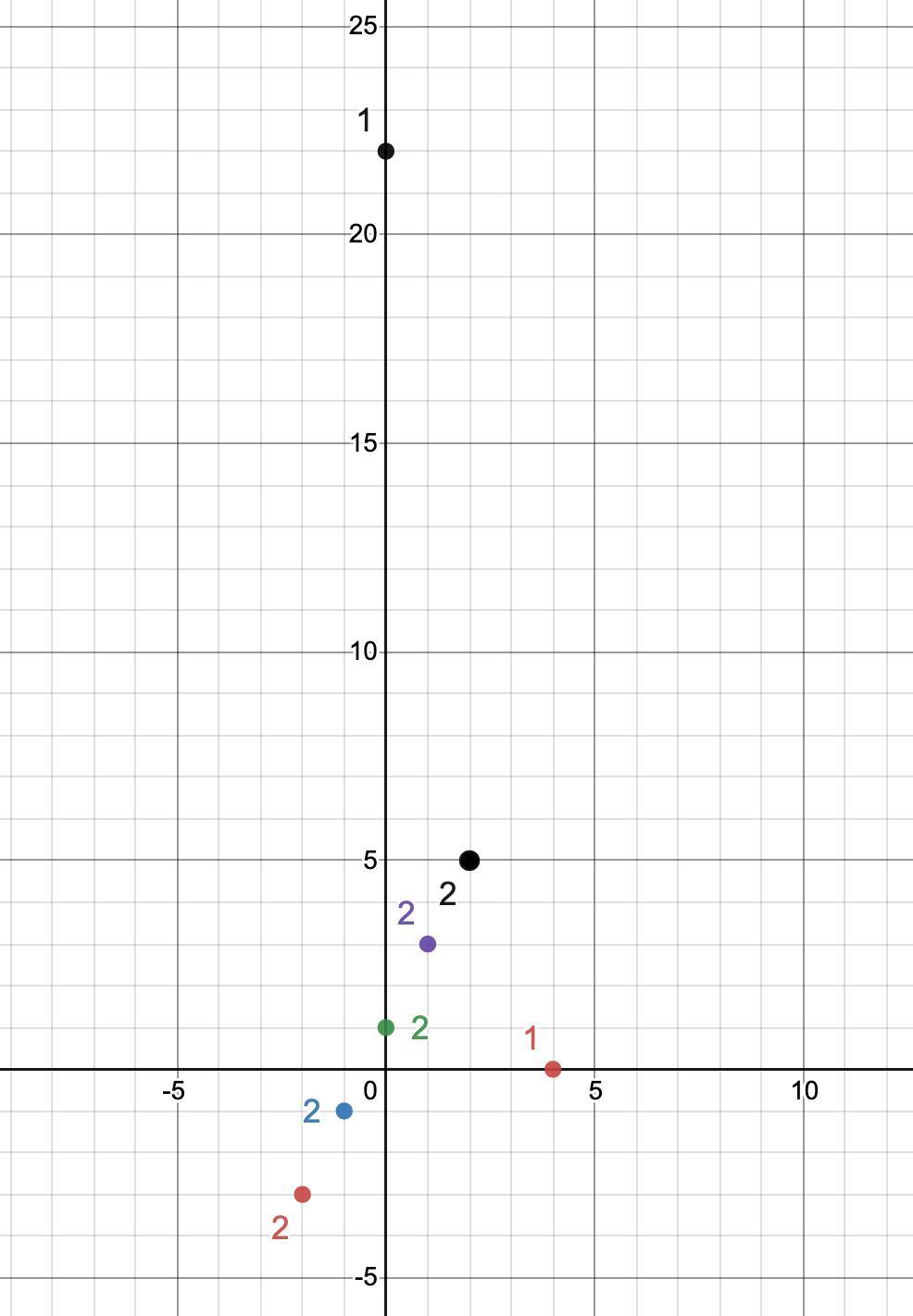
Triangle DEF has vertices D(1,1), E(2,0), and F(0,4). It is transformed by a rotation 180 degrees about the origin followed by a dilation with a scale factor of 3. Find the coordinates of the vertices of triangle D”E”F”.
Answers
Check the picture below.

What is 90.125 written in word form?
Answers
Answer: ninety point one hundred and twenty five?
Step-by-step explanation:
Answer:
ninty point one two five
Step-by-step explanation:
PLEASE HELP ASAP
Use the discriminant to determine how many real number solutions exist for the quadratic equation −4x2 + 3x –28 = 0.
Question 2 options:
A)
0
B)
3
C)
2
D)
1
Answers
What is 2 1/2 in ft?
Answers
Answer:
0.20
Step-by-step explanation:
Answer:
30 inches
Step-by-step explanation:
jenns age is 3 years less than marks age the sum of their ages is 30 let m be marks age find Jenna age
Answers
Answer:
you could do 27+x=30
Step-by-step explanation:
Which polynomial is in standard form?
N
O 26x5 + 12x7-8x3 + 6x
O 3x5 +x-6x2 + 5
11x5-6x2-9x+12
5x2 + 18x3 - 12x4 +4x7
Answers
Answer:
i am pretty sure it is D
Step-by-step explanation:
Answer:
Its C.) 11x^5 - 6x^2 - 9x + 12
Step-by-step explanation:
ejenuwitee
=D
=P
minimum point of the quadratic x^2+6x-2
Answers
Answer:
(-3,-11)
Step-by-step explanation:
Compare the given quadratic equation with the general quadratic equation.
a=1, b=6 and c=-2
\(x=-\dfrac{(6)}{2(1)}\\=-3\)
Subsitute \(-3\) for \(x\) in given quadratic equation.
\(y=(-3)^2+6(-3)-2\\=9-18-2\\=-11\)
The minimum point is (-3,-11).
brainliest and 20 points goes to whoever shows the most work

Answers
The original figure and Picture B are similar, with a scale factor of 1.5.
What are similar figures?Two figures are classified as similar if the side lengths of each figure is proportional.
Then the constant of proportionality representing the ratio between the side lengths is the scale factor.
The ratios of the Picture A and the original figure are given as follows:
12/8 = 1.5.14/10 = 1.4.As the ratios are different, picture A and the original figure are not similar.
The ratios of the Picture B and the original figure are given as follows:
12/8 = 1.5.15/10 = 1.5.Hence they are similar with a scale factor of 1.5.
More can be learned about similar figures at brainly.com/question/14285697
#SPJ1
Write the equation in slope intercept form (y=mx+b)
y = 3 + 4(x − 1)
Answers
Answer:
y=4x-1
Step-by-step explanation:
y=3+4(x-1) <------ distribute property
y=3+4x-4 <---- combine 3 and -4
y=4x-1
Answer: y=4x-1
Step-by-step explanation:
y=3+4(x-1) Given
y=3+4x-4 Distributive Property
y=4x-1 Combine Like Terms
I WILL GIVE BRAINILY PLSSSSS HELPLPPP
Which linear function is increasing at a greater
rate? Explain your reasoning.
Linear Function 1 has an x-intercept of (4, 0) and
a y-intercept at (0, 22)
Linear function 2 includes the points in the table
below

Answers
Answer:
Linear function 2
Step-by-step explanation:
If Linear function 1 has an x-intercept of 4,0 and a y-intercept of 0,22
It's a negative equation, Its not increasing Its decreasing.
So it must be Linear function 2
the second function
step-by-step explanation:
“increasing at a greater rate” is the slope
Function 1 has points at (4, 0) (0, 22)
to find the slope it would be
m = y2 - y1 / x2 - x1
m = 22 - 0 / 0 - 4
m = 22 / -4
m = -5.5
Function 2 we can take any two points from the table
I’m going to take (1, 0) and (3, 1)
m = 3 - 1 / 0 - 1
m = -2 / -1
m = 2 / 1
m = 2
because function 1 is negative function 2 would be increasing at a greater rate.
what is the difference of twice a number and 3 is 11
Answers
Answer:
2x - 3 = 11
x = -21
Step-by-step explanation:
Given the following data set: 3 5 6 7 7 8 8 8 9 9 9 10 102 Researcher detected the technical error in the last observation and replaced 102 by 10.2. What happens to Interquartile Range (IQR) and Standard Deviation (SD)
Answers
Answer:
After correcting the error, IQR remains unchanged while standard deviation reduced drastically from 26.3 to 2.04
Step-by-step explanation:
Given the data:
3 5 6 7 7 8 8 8 9 9 9 10 102
Ordered data: 3, 5, 6, 7, 7, 8, 8, 8, 9, 9, 9, 10, 102
The interquartile range (IQR) for the data:
IQR = Q3 - Q1
Q3 = 3/4(n + 1) th term.
n = sample size = 13
Q3 = 3/4(14) = 10.5th term
Q3 = (10 + 11)th term / 2 = (9+9)/2 = 9
Q1 = 1/4(14) = 3.5th term
Q3 = (3 + 4)th term / 2 = (6+7)/2 = 6.5
IQR = Q3 - Q1 = 9 - 6.5 = 2.5
THE STANDARD DEVIATION :
Sqrt[Σ(X - m)²/n-1]
Using calculator :
Standard deviation = 26.3
Correcting error in the data:
3 5 6 7 7 8 8 8 9 9 9 10 10.2
Q3 = 3/4(n + 1) th term.
n = sample size = 13
Q3 = 3/4(14) = 10.5th term
Q3 = (10 + 11)th term / 2 = (9+9)/2 = 9
Q1 = 1/4(14) = 3.5th term
Q3 = (3 + 4)th term / 2 = (6+7)/2 = 6.5
IQR = Q3 - Q1 = 9 - 6.5 = 2.5
THE STANDARD DEVIATION :
Sqrt[Σ(X - m)²/n-1]
Using calculator :
Standard deviation = 2.04
After correcting the error, IQR remains unchanged while standard deviation reduced drastically from 26.3 to 2.04
Solve the following quadratic inequality x^2+x-6>0
Answers
Answer:
x < -3 or x > 2
Step-by-step explanation:
x² + x - 6 > 0
Convert the inequality to an equation.
x² + x - 6 = 0
Factor using the AC method and get:
(x - 2) (x + 3) = 0
If any individual factor on the left side of the equation is equal to 0, the entire expression will be equal to 0.
x - 2 = 0
x = 2
x + 3 = 0
x = -3
So, the solution is x < -3 or x > 2