Cans of soda are packaged in boxes containing the same number of cans.
There are 36 cans in 4 boxes.
a) How many cans are there in 7 boxes?
b) How many boxes are needed to package 99 cans of soda?
Answers
a)
63 cans in 7 boxes.
b)
11 boxes are needed for 99 cans.
What is a unit rate?It is a rate of one of another quantity.
In 2 hours, a man can walk for 6 miles
In 1 hour, a man will walk for 3 miles.
We have,
36 cans in 4 boxes.
This means,
1 box = 36/4 cans
1 box = 9 cans
Multiply 7 on both sides.
7 box = 63 cans ______(1)
1 box = 9 cans
Multiply 11 on both sides.
11 box = 99 cans ______(2)
Now,
From (1) and (2)
a)
63 cans in 7 boxes.
b)
11 boxes are needed for 99 cans.
Thus,
63 cans in 7 boxes.
11 boxes are needed for 99 cans.
Learn more about unit rates here:
https://brainly.com/question/11258929
#SPJ9
Related Questions
Find the margin of error (at 95%) for a poll of 263 people, 78 of which were found to be in favor of increasing funding for educational technology budgets in schools. Give your answer to the nearest hundredth.
Answers
The margin of error (at 95% confidence level) for the poll of 263 people, with 78 in favor, is approximately 0.0550 or 0.06 rounded to the nearest hundredth.
To find the margin of error for a poll, we can use the formula:
Margin of Error = Critical Value * Standard Error
For a 95% confidence level, the critical value (z-score) is approximately 1.96. The standard error can be calculated using the formula:
Standard Error = \(\sqrt{(p * (1 - p)) / n)}\)
where p is the proportion of people in favor (78/263) and n is the sample size (263).
Let's calculate the margin of error:
p = 78/263 ≈ 0.2966
n = 263
\(Standard Error = \sqrt{(0.2966 * (1 - 0.2966)) / 263)} \\= \sqrt{(0.2075 / 263)} \\= \sqrt{(0.0007889)} \\= 0.0281\\Margin of Error = 1.96 * 0.0281\\= 0.0550\)
Therefore, the margin of error (at 95% confidence level) for the poll of 263 people, with 78 in favor, is approximately 0.0550 or 0.06 rounded to the nearest hundredth.
Read more about margin of error on:
brainly.com/question/29328438
#SPJ4
PLEASE HELP WITH DETAILED ANSWER ASAP FOR 50 POINTS!! You notice these two old suitcases stacked in the closet. The smaller suitcase is 25 in x 8 in x 9 in, and the larger suitcase is 75 in x 20 in x 18 in.
1. The big suitcase is how many times larger than the smaller suitcase?
2. You decide to use the larger suitcase to transport rectangular prism watermelons back home. Though their dimensions vary, the average rectangular watermelon has a volume of roughly 720 cubic inches. If one of these watermelons is about 10 inches long and 9 inches wide, about how tall would it be?
3. If the average rectangular watermelon has a volume of 720 cubic inches, what’s the maximum number of watermelons you’ll be able to bring home in your larger suitcase, assuming all you have in the suitcase are watermelons?
Answers
Step-by-step explanation:
1. To find out how many times larger the big suitcase is than the small suitcase, we need to compare their volumes. The volume of the small suitcase is:
25 in x 8 in x 9 in = 1800 cubic inches
The volume of the large suitcase is:
75 in x 20 in x 18 in = 27,000 cubic inches
To find out how many times larger the big suitcase is, we can divide its volume by the volume of the small suitcase:
27,000 cubic inches ÷ 1800 cubic inches = 15
Therefore, the big suitcase is 15 times larger than the small suitcase.
2. To find the height of the watermelon, we need to use the formula for volume of a rectangular prism:
V = l x w x h
We know that the volume is 720 cubic inches, the length is 10 inches, and the width is 9 inches. Rearranging the formula to solve for the height, we get:
h = V ÷ (l x w)
h = 720 cubic inches ÷ (10 inches x 9 inches)
h ≈ 8 inches
Therefore, the watermelon would be about 8 inches tall.
3. To find out how many watermelons you can fit in the large suitcase, we need to divide its volume by the volume of one watermelon:
27,000 cubic inches ÷ 720 cubic inches = 37.5
However, we can't fit a decimal number of watermelons in the suitcase, so we need to round down. Therefore, the maximum number of watermelons you can bring home in the larger suitcase is 37.
What equation is represented by the equation on the graph?
X
OA. y = x - 2
OB. y=-X-2
OC. y=-3x+2
OD. y=-X+2
Reset Meet

Answers
Answer:
y= -x +2
Step-by-step explanation:
This is a negative slope of one because the line is going down and has a ride over run of 1/1. Then the y intercept is +2 because this line intercepts the y axis at positive 2.
hope it helps!
Answer:
y = -x +2
Step-by-step explanation:
just trust me on this
Solve the following system of equations graphically on the set of axes y= x -5 y=-/x -8
Answers
Answer:
(-3/2, -13/2)
Step-by-step explanation:
To solve the system of equations graphically, we need to plot the two equations on the same set of axes and find the point of intersection.
To plot the first equation y = x - 5, we can start by finding the y-intercept, which is -5. Then, we can use the slope of 1 (since the coefficient of x is 1) to find other points on the line. For example, if we move one unit to the right (in the positive x direction), we will move one unit up (in the positive y direction) and get the point (1, -4). Similarly, if we move two units to the left (in the negative x direction), we will move two units down (in the negative y direction) and get the point (-2, -7). We can plot these points and connect them with a straight line to get the graph of the first equation.
To plot the second equation y = -x - 8, we can follow a similar process. The y-intercept is -8, and the slope is -1 (since the coefficient of x is -1). If we move one unit to the right, we will move one unit down and get the point (1, -9). If we move two units to the left, we will move two units up and get the point (-2, -6). We can plot these points and connect them with a straight line to get the graph of the second equation.
The point of intersection of these two lines is the solution to the system of equations. We can estimate the coordinates of this point by looking at the graph, or we can use algebraic methods to find the exact solution. One way to do this is to set the two equations equal to each other and solve for x:
x - 5 = -x - 8 2x = -3 x = -3/2
Then, we can plug this value of x into either equation to find the corresponding value of y:
y = (-3/2) - 5 y = -13/2
So the solution to the system of equations is (-3/2, -13/2).
Need help I’ll give brainliest

Answers
Answer: there are better people out there
Step-by-step explanation:
2. Group each number as rational and irrational, then graph and label each number on the
number line below. You may label the number with the letter.
A 0.75
B√√3
C√√√9
1
D -2-
2
15
10
E
F 2.6
G-√√2
HTT
Rational
Irrational …the answer is what???
Answers
Therefore , the solution of the given problem of rational numbers comes out to be A) 0.75 can be written as 75/100.
What is rational number?Rational numbers are those that may be written as a ratios (or fraction) of 2n. A fraction with a nonzero denominator is said to be rational. A few instances of rational numbers are 1/2, 1/5, and 3/4. As a real function, "0" can also be stated in a number of different ways, such as 0/1, 0/2, etc 0/3. But 1/0, 2/0, 3/0, and so forth. The number seven makes sense. A rational number is produced when three integers are split. To get the rational number 7, divide whole 7 ps by the total number 1. Since 7 can be obtained by dividing two integers, it is referred to as a real function.
Here,
Given :
=> Any number that can be expressed as a straightforward fraction is considered rational. This is the shape of a fraction: => a/b
where the denominator is "b" and the numerator is "a". Since both are integers, b 0.
=>Simple fractions cannot be used to represent irrational values.
So,
A) 75/100 is equivalent to 0.75 rational.
B) √√3 is irrational.
C) √√√9 is irrational.
To know more about rational numbers visit:
https://brainly.com/question/24540810
#SPJ1
there is a mound of g pounds of gravel in a quarry. throughout the day, 300 pounds of gravel are added to the mound. two orders of 900 pounds are sold and the gravel is removed from the mound. at the end of the day, the mound has 1,300 pounds of gravel. write the equation that describes the situation. then solve for g.
Answers
The required equation is g+300-900-900=1300.
The value of g is 2800 pounds.
What is the solution to the equation?The definition of an equation in algebra is a mathematical statement that demonstrates the equality of two mathematical expressions.
Initially, there is a mound of g pounds of gravel, then 300 pounds are added, and then two orders of 900 pounds are sold. At the end of the day, the mound has 1,300 pounds of gravel.
The equation for the given information is formed as follows:
g+300-900-900=1300
Solve the equation to find the value of g,
g+300-1800=1300
g-1500=1300
g=1300+1500
g=2800
Learn more about equations here:
https://brainly.com/question/2972832
#SPJ4
The American Hospital Association stated in its annual report that the mean cost to community hospitals per patient per day in U.S. hospitals was $1231 in 2007. In that same year, a random sample of 25 daily costs in the state of Utah hospitals yielded a mean of $1103. Assuming a population standard deviation of $252 for all Utah hospitals, do the data provide sufficient evidence to conclude that in 2007 the mean cost in Utah hospitals is below the national mean of $1231? Perform the required hypothesis test at the 5% significance level.
Answers
We can conclude that the null hypothesis is rejected. There is sufficient evidence to support the claim that the mean cost in Utah hospitals is below the national mean of $1231.
How is this so?H₀: μ ≥ 1231 (The mean cost in Utah hospitals is greater than or equal to the national mean)
Hₐ: μ < 1231 (The mean cost in Utah hospitals is below the national mean)
Given
Sample mean (x) = $1103Sample size (n) = 25Population standard deviation (σ) = $252Significance level (α) = 0.05The test statistic for a one-sample t-test is given by
t = (x - μ) / (σ / √n)
Substituting we have
t = (1103 - 1231) / (252 / √25)
≈ -6.103
To determine the critical value, we need to find the critical t-value at the 5% significance level with degrees of freedom
(df) equal to (n - 1)
= (25 - 1)
= 24.
Using a t-distribution table or calculator, the critical value is approximately -1.711.
Since the calculated test statistic (-6.103) is smaller than the critical value (-1.711) and falls into the critical region, we reject the null hypothesis.
Learn more about standard deviation at:
https://brainly.com/question/475676
#SPJ4
the diagram Shows a sector of a circle radius 10cm and angle 135 degrees find out perimeter
Answers
Answer: 22.4 cm
Step-by-step explanation:
Perimeter of sector is: P = 2r + 2πr(θ/360) P = 2*10 + 2(3.14) (135/360) = 22.4 cm (rounded)

Mr. Greene is trying to decide which charter bus
company to use for the 8th grade Outer Banks trip.
Holiday Tours charges a down payment of $300 and $3
per mile. Diamond Tours charges a $500 down payment
and $1 per mile. Write and solve an equation to find the
number of miles when the cost of both companies will be
the same.
Answers
Answer: 100 miles
Step-by-step explanation:
300+3x=500+1x
subtract 1 from both sides
300+2x=500
subtract 300 from both sides
2x=200
divide 2
x=100
It is what I’m looking for thank you for helping me out
Answers
nice
Step-by-step explanation:
challenging: what is [po43–] at equilibrium when 75 ml of 0.80 m pb(no3)2(aq) is mixed 10. ml of 0.10 m na3po4(aq) at 25 °c given that the ksp of pb3(po4)2 is 1.0×10–54? 1.
Answers
The calculated concentration of [PO43-] at equilibrium is approximately 7.28 x 10^20 M.
To determine the concentration of [PO43-] at equilibrium, we need to consider the reaction between Pb(NO3)2 and Na3PO4. The balanced equation for the reaction is:
3Pb(NO3)2 + 2Na3PO4 -> Pb3(PO4)2 + 6NaNO3
Given the initial volumes and concentrations of the solutions, we can calculate the moles of Pb(NO3)2 and Na3PO4 used in the reaction. From there, we can determine the moles of Pb3(PO4)2 formed and the resulting concentration of [PO43-] at equilibrium.
Using the volumes and concentrations provided (75 ml of 0.80 M Pb(NO3)2 and 10 ml of 0.10 M Na3PO4), we find that the moles of Pb(NO3)2 used is 0.06 mol and the moles of Na3PO4 used is 0.001 mol. Since the stoichiometric ratio between Pb3(PO4)2 and PO43- is 2:1, we can calculate that 0.03 mol of Pb3(PO4)2 is formed.
To determine the concentration of [PO43-] at equilibrium, we divide the moles of Pb3(PO4)2 formed by the total volume of the solution (85 ml), resulting in a concentration of approximately 7.28 x 10^20 M.
Therefore, at equilibrium, the concentration of [PO43-] is approximately 7.28 x 10^20 M. This value is obtained by considering the stoichiometry of the reaction and the initial concentrations and volumes of the solutions.
Learn more about equilibrium here:
https://brainly.com/question/14281439
#SPJ11
please help me answer this question about rectangles

Answers
Answer:
5.14
Step-by-step explanation:
The similarity ratio is 4/7 we can write an equation to find x
4/7 = 9/x cross multiply
7x = 36 divide both sides by 7
x = 5.14 approximately
Answer:
The answer is B
Step-by-step explanation:
5.14 hope this helps. :)
The half-life of a radioactive kind of praseodymium is 17 minutes. If you start with 56 grams of it, how much will be left after 34 minutes? grams Submit
Answers
Answer:
14
Step-by-step explanation:
First, find out how many half-lives have passed. Divide the number of minutes by the length of a half-life. 34÷17=22 half-lives have passed. Now figure out how much praseodymium will be left after 2 half-lives have passed. Multiply the starting amount of praseodymium by 12 a total of 2 times. 56 x 1/2 x 1/2 = 14 That calculation could also be written with exponents:56x (1/2)^2=14 After 2 half-lives, the amount of praseodymium left will be 14 grams.
Assuming the half-life of a radioactive kind of praseodymium is 17 minutes.The amount that will be left after 34 minutes is 14g.
Final amountSince 34 minutes is exactly 2 half-lives of 17 minutes.
Hence, remaining amount will then be:
Remaining amount= (1/2)×(1/2) = (1/2)² of the initial amount
Final amount:
Final amount = (1/2)² (56g)
Final amount=0.25 (56g)
Final amount = 14 g
Inconclusion the half-life of a radioactive kind of praseodymium is 17 minutes.The amount that will be left after 34 minutes is 14g.
Learn more about final amount here:https://brainly.com/question/25109150
Can someone help me please?

Answers
Answer:
56
Step-by-step explanation:
The angle sum theorem *I think* is that for an n-sided shape, the total sum of its angles is 180(n-2).
The sum of a triangles' angles is 180 degrees.
Therefore 4v-44 = 180.
v - 11 = 45
v = 56 degrees
Note: always check if the answer makes sense
Answer:
56 degrees
Step-by-step explanation:
Select ALL the quadratic functions that open UP
f(x) = -x² + 2x + 9
f(x) = 7x² - 8x - 53
g(x) = -2(x+3)² – 1
h(x) = 4(x-2)(x + 9)
f(x) = x² + 4x − 1
Answers
Answer:
f(x) and g(x) are the quadratic functions that open UP.
The art club spent $45 on supplies for valentines to sell during lunches. They made 60 valentines. What selling price for a valentine would result in a profit of zero if all the valentines are sold?
Answers
The value of R2 always ...
lies below 0
lies above 1
lies between 0 and 1
lies between -1 and +1
Answers
The value of R2 always lies between 0 and 1.The value of R2 represents the proportion of the variation in the dependent variable that can be explained by the independent variables, ranging from 0 to 1.
The value of R2, also known as the coefficient of determination, measures the goodness of fit of a regression model. It represents the proportion of the total variation in the dependent variable that is explained by the independent variables in the model.
R2 ranges between 0 and 1, where 0 indicates that the independent variables have no explanatory power and cannot predict the dependent variable's variation. On the other hand, an R2 value of 1 indicates that the independent variables perfectly explain all the variation in the dependent variable.
An R2 value greater than 1 or less than 0 is not possible because it would imply that the model explains more than 100% or less than 0% of the dependent variable's variation, which is not meaningful. Therefore, the value of R2 always lies between 0 and 1, providing a measure of the model's explanatory power.
To learn more about coefficient, click here:
/brainly.com/question/1594145
#SPJ1
Please answer it now

Answers
Answer:
1306.90≅ 1307 rounded to the nearest tens
Step-by-step explanation:
r= radius = 13 cm
l is the slant height =19 cm
surface area of the cone= area of the circle + area of the curved of the cone
SA=πrl +πr²
SA=π(13)(19) +169π
SA= 247π +169π= 416π=1306.90≅ 1307 rounded to the nearest tens
Let Z
26
={0,1,…,25} be the set of remainders modulo 26 . Define the function f:Z
26
→Z
26
as f(x)=3x
5
−9x
3
−5x+1(mod26) Consider the following encryption scheme which can encrypt messages consisting of lowercase letters (a to z ) only. To encrypt an ℓ-character message m=m
1
m
2
⋯m
ℓ
: - Interpret every letter m
i
as an element of Z
26
. That is, a↔0,b↔1, and so on. - Compute the values f(m
1
),f(m
2
),…,f(m
ℓ
). - Re-interpret these values as letters and output the concatenation as the ciphertext. For example, the encryption of "helloworld" is "ghaadvduae". Your task is to decrypt the following ciphertexts. a) Decrypt the ciphertext "gbshbjuhbwxhzhxwhu" b) Decrypt the ciphertext "ahwxhbwefmmbwdebn" c) Explain your methods. Note: it is recommended to use a computer program in this problem.
Answers
The decryption of ciphertext "gbshbjuhbwxhzhxwhu"
The function f: Z26→Z26 is defined as f(x) = 3x5−9x3−5x+1(mod 26).The decryption is calculated as f−1(y)=(7y+15)(−3)(mod26)Step-by-step solution: Using the formula for decryption:
f-1(y) = (7y+15)(-3)(mod 26)
Calculating the decryption:
=> f-1(g) = (7 * 6 + 15) * (-3) = -159= -159 + 4 * 26 = 49
=> f-1(b) = (7 * 1 + 15) * (-3) = -72= -72 + 3 * 26 = 6=> f-1(s) = (7 * 18 + 15) * (-3) = -333= -333 + 13 * 26 = 55
=> f-1(h) = (7 * 7 + 15) * (-3) = -138= -138 + 6 * 26 = 24
=> f-1(j) = (7 * 9 + 15) * (-3) = -198= -198 + 8 * 26 = 10
=> f-1(u) = (7 * 20 + 15) * (-3) = -483= -483 + 18 * 26 = 15
=> f-1(h) = (7 * 7 + 15) * (-3) = -138= -138 + 6 * 26 = 24
=> f-1(b) = (7 * 1 + 15) * (-3) = -72= -72 + 3 * 26 = 6
=> f-1(w) = (7 * 22 + 15) * (-3) = -534= -534 + 20 * 26 = 46
=> f-1(x) = (7 * 23 + 15) * (-3) = -561= -561 + 22 * 26 = 39
=> f-1(h) = (7 * 7 + 15) * (-3) = -138= -138 + 6 * 26 = 24
=> f-1(x) = (7 * 23 + 15) * (-3) = -561= -561 + 22 * 26 = 39
=> f-1(w) = (7 * 22 + 15) * (-3) = -534= -534 + 20 * 26 = 46
=> f-1(h) = (7 * 7 + 15) * (-3) = -138= -138 + 6 * 26 = 24
=> f-1(u) = (7 * 20 + 15) * (-3) = -483= -483 + 18 * 26 = 15
Decrypted text: gbshbjuhbwxhzhxwhu → gszwjeitjpkotixibq
Learn more about ciphertext from the given text:
https://brainly.com/question/9380417
#SPJ11
HELLPP HURRRY FIND THE AREA

Answers
Answer:
254.47
Step-by-step explanation:
A=πr2=π·92≈254.469
Answer:
THE ANSWER IS D :)
Step-by-step explanation:
1+2-5*67-8
Need it now please
Answers
Answer:
-340
Step-by-step explanation:
Hey there!
1 + 2 - 5 * 67 - 8
= 3 - 5 * 67 - 8
= 3 - 335 - 8
= -332 - 8
= -340
Therefore, your answer is: -340
Good luck on your assignment & enjoy your day!
~Amphitrite1040:)
6) The solution interval to 2sinx <- 3cosx; [- ,] is [- 3. 142,-0.983) U (2.159, 6. 283]. If true, justify. If false, justify. Show your step by step process of how you got the answer.
Answers
True. The solution interval to 2sinx < -3cosx on the interval [-π, π] is [-3.142, -0.983) ∪ (2.159, 6.283].
What is the solution of the interval?
To solve the inequality 2sinx < -3cosx, we can divide both sides by cosx (since cosx ≠ 0 for x ∈ [-π, π]), which gives:
2tanx < -3
Dividing both sides by 2 and taking the arctangent of both sides, we have:
x < arctan(-3/2)
Using a calculator, we can find that arctan(-3/2) ≈ -0.983 radians and arctan(-3/2) + π ≈ 2.159 radians.
Therefore, the solution interval to 2sinx < -3cosx on the interval [-π, π] is given by:
[-3.142, -0.983) ∪ (2.159, 6.283].
This is true, as it corresponds to the values of x for which 2sinx < -3cosx is satisfied on the given interval.
Learn more about solution interval here: https://brainly.com/question/29190944
#SPJ1
The complete question is below:
The solution interval to 2sinx <- 3cosx ; [- π, π] is
[- 3.142, - 0.983) ∪ (2.159, 6.283].
If true, justify. If false, justify.
Use the Change of Base Formula to evaluate each expression.
log₃33
Answers
Using the change of base formula the evaluated value of \(log_{3} 33\) is 3.184
Change of base formula is used to change the base of logarithm. It is used to write a logarithm of a number with a given base as the ratio of two logarithms each with the same base that is different from the base of the original logarithm.
According to the change of base formula :
\(log_{a} b = \frac{log_{x} b}{log_{x} a}\)
where base x is base 10
According to the question, we have to find the value of \(log_{3} 33\)
Thus applying change of base formula we get
\(log_{3} 33 = \frac{log_{10} 33}{log_{10} 3}\)
calculating the value of log from the calculator we get,
\(log_{3} 33 = \frac{1.519}{0.477}\\log_{3} 33 = 3.184\)
Thus using the change of base formula the evaluated value of \(log_{3} 33\) is 3.184
Learn more about logarithm here : https://brainly.com/question/10208274
#SPJ4
find a function from the set {1, 2, …, 30} to {1, 2, …, 10} that is a 3-to-1 correspondence. (you may find that the division, ceiling or floor operations are useful.)
Answers
One possible function that is a 3-to-1 correspondence from the set {1, 2, ..., 30} to {1, 2, ..., 10} is f(x) = ⌈x/3⌉.where ⌈x/3⌉ denotes the ceiling function of x/3, which rounds up x/3 to the nearest integer.
Intuitively, the function groups every three consecutive integers in the domain {1, 2, ..., 30} into the same integer in the range {1, 2, ..., 10}.
Specifically, the first three integers 1, 2, 3 in the domain map to 1 in the range, the next three integers 4, 5, 6 map to 2, and so on, until the last three integers 28, 29, 30 map to 10.
To see that this function is indeed a 3-to-1 correspondence, we can note that for any integer k in the range {1, 2, ..., 10}, there are three integers in the domain that map to it.
Specifically, if k = 1, then the integers 1, 2, 3 in the domain map to it; if k = 2, then the integers 4, 5, 6 map to it; and so on, until if k = 10, then the integers 28, 29, 30 map to it.
Conversely, for any integer x in the domain {1, 2, ..., 30}, the function f(x) maps it to an integer in the range {1, 2, ..., 10}, and this integer is unique. Therefore, the function f is a 3-to-1 correspondence from the domain to the range.
Know more about function click here;
https://brainly.com/question/30721594
#SPJ11
Jazmin and Justine went shopping for back to chool clothes. Jazmin purchasrd three shirts and one pair of shorts ans spent $38. 0. Justine bought four shirts and three pairs of shorts and spent $71. 50.
Part A: Assumibg all the shirts cost the same amount and all the shorts cost the same amount, write a system os equations to represent each girl's shoppins spree
Part B: Use the elimination method to solve for the price of one pair of shorts
Answers
The equations to represent each girl's shoppins spree is 3x + y = 38.0 and 4x + 3y = 71.50. Price of one shirt costs $8.50 and price of one pair of shorts costs $12.50.
Let x be the price of one shirt, and y be the price of one pair of shorts. Then, we can write the following system of equations
For Jazmin
3x + y = 38.0
For Justine
4x + 3y = 71.50
To solve for the price of one pair of shorts using elimination method, we need to eliminate one of the variables. We can do this by multiplying the first equation by 3 and the second equation by -1, and then adding them together
9x + 3y = 114.0
-4x - 3y = -71.50
5x = 42.50
x = 8.50
So, one shirt costs $8.50.
To find the price of one pair of shorts, we can substitute the value of x into either of the original equations and solve for y. Using the first equation
3(8.50) + y = 38.0
25.50 + y = 38.0
y = 12.50
Therefore, one pair of shorts costs $12.50.
To know more about price:
https://brainly.com/question/18909234
#SPJ4
Hi Can you help me with this question. Thank you x
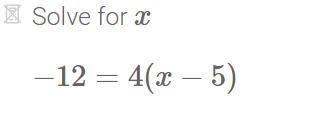
Answers
Answer:
x=3
Step-by-step explanation:
please mark me as brainliest
Answer:
x = 2
Step-by-step explanation:
first we solve inside brackets (x-5) = 20 so it be -12 = 4x -20
then we add 20 to both sides so it be -12 + 20 = 4x -20 +20
then we simplify so it be 8 = 4x
then we divide both sides with 4 so it be 8/4 = 4x/4
then we simplify so it be x=2
SO THE ANSWER IS x=2 OR 2
hope that helps!! pls brainliey if u dont mind! :))
Nichole gets paid $13 and hour washing cars.
A) create an equation that models nicholes pay where x is the number of hours and y is the pay.
B) What is the slope? what is the intercept of the equation?
Answers
Answer:
A) y = 13x
B) Slope is 13, intercept is 0
Step-by-step explanation:
Answer:
y = 13x
The slope is 13
The y intercept is 0
Step-by-step explanation:
Since he gets $13 per hour, his pay can be represented by 13x, as x is the number of hours.
To create the equation, set this equal to y, as it represents his total pay.
So, the equation is y = 13x.
Using the equation y = mx + b, we can find the slope (m) and the y intercept (b)
Since this equation has 13 as the coefficient, the slope is 13. There is also no b value since nothing is being added to 13x, so the y intercept is 0.
Let X be a binomial random variable with the following parameters: and PE 1 /4 : r = 0,1,..., Find the probability distribution of the random variable Y = X^2 + 1 n.
Answers
To find the probability distribution of the random variable Y = X^2 + 1, where X is a binomial random variable with parameters n and p = 1/4, we need to determine the probability mass function (PMF) of Y.
The PMF gives the probability of each possible value of the random variable. In this case, Y can take on values of 1, 2, 5, 10, and so on, depending on the values of X. Let's calculate the PMF for Y: P(Y = y) = P(X^2 + 1 = y) = P(X^2 = y - 1). Since X is a binomial random variable, its possible values are 0, 1, 2, ..., n. Therefore, we need to find the values of X that satisfy the equation X^2 = y - 1.
For each value of y, we can find the corresponding values of X and calculate the probability of X taking on those values using the binomial probability formula: P(X = r) = C(n, r) * p^r * (1 - p)^(n - r) where C(n, r) is the binomial coefficient given by C(n, r) = n! / (r! * (n - r)!). Let's calculate the PMF for each possible value of Y: For y = 1: P(Y = 1) = P(X^2 = 1 - 1) = P(X^2 = 0). The only value of X that satisfies X^2 = 0 is X = 0. P(X = 0) = C(n, 0) * p^0 * (1 - p)^(n - 0) = (1 - p)^n. For y = 2: P(Y = 2) = P(X^2 = 2 - 1) = P(X^2 = 1). The values of X that satisfy X^2 = 1 are X = -1 and X = 1. P(X = -1) = C(n, -1) * p^(-1) * (1 - p)^(n - (-1)) = 0 (since n cannot be negative), P(X = 1) = C(n, 1) * p^1 * (1 - p)^(n - 1) = n * p * (1 - p)^(n - 1). For y = 5: P(Y = 5) = P(X^2 = 5 - 1) = P(X^2 = 4).
The values of X that satisfy X^2 = 4 are X = -2 and X = 2. P(X = -2) = C(n, -2) * p^(-2) * (1 - p)^(n - (-2)) = 0 (since n cannot be negative), P(X = 2) = C(n, 2) * p^2 * (1 - p)^(n - 2). Similarly, you can continue this process for other values of y. Please provide the value of n (the number of trials) to calculate the specific probabilities for each value of Y.
To learn more about probability, click here: brainly.com/question/16988487
#SPJ11
Please help me with this math problem!! Will give brainliest!! :)

Answers
Answer:
180 ft cubed.
Step-by-step explanation:
The formula for volume is V = lwh
6 is the height, 10 is the length, and 3 is the width.
So then you would multiply 6, 10 and 3, which equals 180.