Answers
Equivalence means to be same, whether it be value, temperature, size, etc.
Let's take a look at shape 1.
Shape 1 has a base of 3, and a height of 4, but there is a gap with a width of 1 and a height of 2. So, we can use this equation to figure out the area:
(3 × 4) - (1 × 2) = 10So, shape 1 has an area of 10 units.
Let's look at shape 2.
Shape 2 has a base of 2, and a height of 4, but there is also a gap, with a width of 1 and a height of 3. So, we can use this equation to figure out the area:
(2 × 4) - (1 × 3) = 5So, shape 2 has an area of 5 units.
Let's look at shape 3.
Shape 3 consists of 4 right triangles, put together to form an arrowhead. The shape has a base of 4, and a height of 2. So, we can use this equation to figure out the area:
(4 × 2) ÷ 2 = 4So, shape 3 has an area of 4 units.
Let's look at shape 4.
Shape 4 consists of 4 right triangles, put together to form a diamond. The shape has a base of 4, and a height of 2. So, we can use this equation to figure out the area:
(4 × 2) ÷ 2 = 4So, shape 4 has an area of 4 units.
Looking to the final shape (5), we can see it consists of a long rectangle and 2 right triangles. Instead of calculating the base and height of the entire shape, we can break it into pieces, making a rectangle that has a base of 3, and a height of 1. Then we are left a shape that has a base of 2, and a height of 3. So, we can use this equation to figure out the area:
(3 × 1) + ([2 × 3] ÷ 2) = 6So, shape 5 has an area of 6 units.
Let's compare the area of all of the shapes.
Has an area of 10 square units. Has an area of 5 square units. Has an area of 4 square units. Has an area of 4 square units. Has an area of 6 square units.Therefore, the two figures that have equal areas are shapes 3 and 4.
Related Questions
A cone-shaped container is completely filled with liquid. The container has a radius of 60 cm and an height of 210 cm. The liquid is drained from the container at a rate of 1099 cm³ per hour. How many hours will it take to drain all of the liquid? Use 3. 14 to approximate pi. Enter your answer in the box. Hours.
Answers
Find the volume of the cylinder to know how much liquid is in it:
volume of a cylinder = Pi x r^2 x height.
Volume = 3.14 x 60^2 x 210
Volume = 2,373,840 cubic cm
now to find the length of time divide total cubic cm by the drainage rate:
2,373,840 / 1099 = 2,160 hours
Answer: 2,160 hours
These are radical and complex number questions, i need to simplify both WITH WORK, please help

Answers
The values of the radical expressions are \(\frac{5x^4z^3}{7y}\) and \(2x^2y\sqrt{5z}\)
What are expressions?Expressions are mathematical statements that are represented by variables, coefficients and operators
How to evaluate the radical expression?Expression 1
Here, we have
\(\sqrt{\frac{25x^8z^6}{49y^2}}\)
Take the square roots of 25 and 49
So, we have
\(\frac{5}{7}\sqrt{\frac{x^8z^6}{y^2}}\)
Next, we apply the law of indices in simplifying the radical expressions
This gives
\(\frac{5}{7}\frac{x^{(8/2)}z^{(6/2)}}{y^{(2/2)}}\)
Evaluate
\(\frac{5}{7}\frac{x^4z^3}{y}\)
Rewrite as
\(\frac{5x^4z^3}{7y}\)
Expression 2
Here, we have
\(\frac{\sqrt{100x^4y^2z}}{\sqrt 5}}\)
Take the square roots of 100
So, we have
\(10\frac{\sqrt{x^4y^2z}}{\sqrt 5}}\)
Rationalize
\(10\frac{\sqrt{5x^4y^2z}}{5}\)
Next, we apply the law of indices in simplifying the radical expressions
This gives
\(10\frac{\sqrt{5z}x^{4/2}y^{2/2}}{5}\)
Evaluate
\(2\sqrt{5z}x^2y\)
Rewrite as
\(2x^2y\sqrt{5z}\)
Hence, the solution is \(2x^2y\sqrt{5z}\)
Read more about expressions at
https://brainly.com/question/723406
#SPJ1
Solve the equation
- 3 = x - 8
Answers
Answer:
x=5
Step-by-step explanation:
-3=x-8
8-3=x
x=5
Please can someone hell me with this!!!!!
Plzz any 1!!

Answers
Step-by-step explanation:
I hope this makes sense...and is visible..
:)

8x + 2+ 4y; x = 9, y = 2
Answers
Answer: 82
Step-by-step explanation:
We simply need to substitute the values for x and y into the equation
Both variables are already given to us, x=9, y=2
8(9)+2+4(2)
72+2+8
82
Write an exponential equation for the following graph. Will give brainiest if you answer
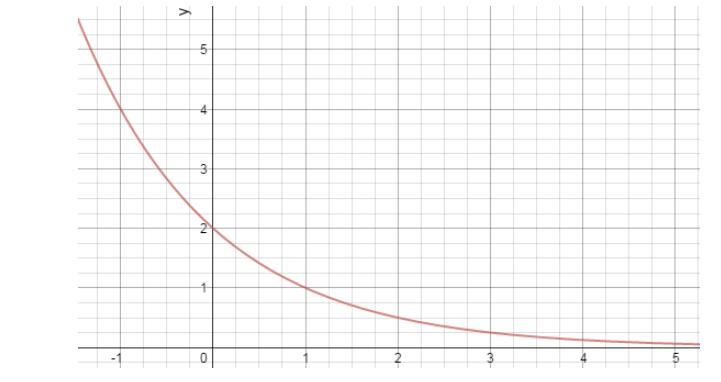
Answers
Answer:
f(x) = \(2(\frac{1}{2})^x\)
Step-by-step explanation:
Let the exponential function is,
f(x) = a(b)ˣ
Since, the given graph passes through two points (0, 2) and (1, 1)
For point (0, 2),
2 = a(b)⁰
2 = a
For point (1, 1),
1 = a(b)¹
ab = 1
2(b) = 1
b = \(\frac{1}{2}\)
Therefore, equation of the exponential function will be,
f(x) = \(2(\frac{1}{2})^x\)
Jason frequently travels for work. This year he plans to make 15 trips to Chicago. What is the total cost for the airfare? Write an equation that represents the problem. Then solve the equation.
Which would cost more; 15 trips to Boston or 11 trips to New York? Explain.
Boston: $178 New York: 225 Chicago: $489 Los Angeles: $1.240
Answers
1) The total cost for 15 trips to Chicago is $7,335.
2) The 15 trips to Boston cost more.
What is the total cost for the airfare?We know that each travel to chicago costs $489, then the cost of x travels to chicago is:
C(x) = $489*x
Here we know that Jason frequently travels for work, and he makes 15 travels to Chicago, so we can replace x by 15, so we will get:
C(15) = $489*15 = $7,335
So the total cost for the airfare is $7,335.
Second question, a tripo to Boston costs $178, then 15 trips to Boston costs:
15*$178 = $2,670
While a trip to New York costs $225, then the cost of 11 trips is:
11*$225 = $2,475
So we can see that 15 trips to Boston costs more.
Learn more about cost equations:
https://brainly.com/question/25109150
#SPJ1
help me as fast as you can

Answers
jane is going on a backpacking trip with her family. they need to hike to their favorite camping spot and set up the camp before it gets dark. sunset is at 5:52 p.m. it will take 1 hour and 36 minutes to hike to the camping spot and 57 minutes to set up the camp. what is the latest time jane and her family can start hiking?
Answers
The latest time Jane and her family can start hiking is 3:19 p.m.
The latest time Jane and her family can start hiking is as follows:
1. First, find the total time needed for hiking and setting up camp: 1 hour and 36 minutes for hiking, plus 57 minutes for setting up camp.
2. Convert the hours and minutes to minutes only: 1 hour = 60 minutes, so 1 hour and 36 minutes = 60 + 36 = 96 minutes.
3. Add the hiking time (96 minutes) to the camp setup time (57 minutes): 96 + 57 = 153 minutes.
4. Convert 153 minutes back to hours and minutes: 153 minutes = 2 hours and 33 minutes.
5. Subtract the total time needed (2 hours and 33 minutes) from the sunset time (5:52 p.m.): 5:52 p.m. - 2 hours and 33 minutes.
6. The latest time Jane and her family can start hiking is 3:19 p.m.
Your answer: The latest time Jane and her family can start hiking is 3:19 p.m.
Learn more about time here,
https://brainly.com/question/11245663
#SPJ11
Bella is learning to type. She typed a letter to her teacher but misspelled 8 words. If she misspelled 25% of the words she typed, how many words did she type in her letter.
Answers
Bella is learning to type. She typed a letter to her teacher but misspelled 8 words. If she misspelled 25% of the words she typed, how many words did she type in her letter?
_________________________________________
She misspelled 8 words = 25% of the words she typed
25%= (8 / total words)* 100
0.25 = 8 / total words
total words = 8/0.25
total words = 32
__________________________-
The total words she typed in her letter were 32
A study measured the weights of a sample of 30 rats under experiment controls. Suppose that 12 rats were underweight.
1. Calculate a 95% confidence interval on the true proportion of underweight rats from this experiment._____...______
2. Using the point estimate of p obtained from the preliminary sample, what is the minimum sample size needed to be 95% confident that the error in estimating the true value of p is less than 0.02?__________
3. How large must the sample be if you wish to be 95% confident that the error in estimating p is less than 0.02, regardless of the true value of
p?__________
Answers
The 95% confidence interval on the true proportion of underweight rats from this experiment is (0.189, 0.611), the minimum sample size required to be 95% confident that the error in estimating the true value of p is less than 0.02 is 576 and, the sample size required to be 95% confident that the error in estimating p is less than 0.02, regardless of the true value of p, is 9604.
1. Calculation of a 95% confidence interval on the true proportion of underweight rats:
Here, n = 30, and p = 12/30 = 0.4 (12 rats out of 30 were underweight).
We will use the following formula to calculate the 95% confidence interval on the true proportion of underweight rats: (p - E, p + E),
where E = zα/2 * √[p (1 - p) / n]We know that α = 0.05 (since the confidence level is 95%).
Therefore, zα/2 = z0.025 = 1.96 (from the standard normal table).
E = 1.96 * √[(0.4)(0.6) / 30] = 0.211(p - E, p + E) = (0.4 - 0.211, 0.4 + 0.211) = (0.189, 0.611)
Therefore, a 95% confidence interval on the true proportion of underweight rats from this experiment is (0.189, 0.611).
2. Calculation of the minimum sample size required to be 95% confident that the error in estimating the true value of p is less than 0.02:
Here, we will use the following formula to calculate the minimum sample size required:n = [zα/2 / E]² * p * (1 - p)
We know that α = 0.05 (since the confidence level is 95%). T
herefore, zα/2 = z0.025 = 1.96 (from the standard normal table).
E = 0.02 (since we want the error to be less than 0.02).p = 0.4 (using the point estimate of p obtained from the preliminary sample).n = [1.96 / 0.02]² * 0.4 * 0.6 = 576
Therefore, the minimum sample size required to be 95% confident that the error in estimating the true value of p is less than 0.02 is 576.
3. Calculation of the sample size required to be 95% confidence that the error in estimating p is less than 0.02, regardless of the true value of p:
We will use the following formula to calculate the sample size required:
n = [zα/2 / E]²We know that α = 0.05 (since the confidence level is 95%).
Therefore, zα/2 = z0.025 = 1.96 (from the standard normal table).
E = 0.02 (since we want the error to be less than 0.02).n = [1.96 / 0.02]² = 9604
Therefore, the sample size required to be 95% confident that the error in estimating p is less than 0.02, regardless of the true value of p, is 9604.
To know more about 95% confidence interval visit:
https://brainly.in/question/35949101
#SPJ11
Question 7
GEOMETRY The perimeter of a square is four times the length of one side. If the side length of a square is 1 centimeter, then the perimeter of the
square is 4 centimeters. Write an equation in point-slope form to find the perimeter y of a square with side length x.
Answers
Answer:
y= 4x + 0
Step-by-step explanation:
The perimeter y of a square with side length x can be described by the equation y = 4x + 0.
The perimeter of a square is equal to four times the length of one side. If the side length of a square is 1 centimeter, then the perimeter of the square is 4 centimeters. We can use this information to write an equation in point-slope form to describe the relationship between the side length of a square and its perimeter.
Point-slope form is a way of writing the equation of a line when we know the slope of the line and the coordinates of a point on the line. In this case, the slope of the line is 4, since the perimeter of a square is four times the length of one side. The coordinates of a point on the line are (1, 4), since the side length of a square is 1 centimeter and the perimeter of the square is 4 centimeters.
We can use this information to write the equation of the line in point-slope form. The equation of a line in point-slope form is given by the following equation:
y - y1 = m(x - x1)
where y is the y-coordinate of a point on the line, y1 is the y-coordinate of a known point on the line, m is the slope of the line, and x is the x-coordinate of a point on the line.
In this case, we can substitute the values we know into the equation above to get:
y - 4 = 4(x - 1)
This simplifies to:
y = 4x + 0
Therefore, the equation in point-slope form that describes the relationship between the side length x of a square and its perimeter y is y = 4x + 0.
This equation tells us that for any square, the perimeter is four times the side length plus zero. For example, if the side length of a square is 5 centimeters, then the perimeter is 4 x 5 + 0 = 20 centimeters. If the side length of a square is 10 centimeters, then the perimeter is 4 x 10 + 0 = 40 centimeters.
In summary, the perimeter y of a square with side length x can be described by the equation y = 4x + 0.
A King in ancient times agreed to reward the inventor of chess with one grain of wheat on the first of the 64 squares of a chess board. On the second square the King would place two grains of wheat, on the third square, four grains of wheat, and on the fourth square eight grains of wheat. If the amount of wheat is doubled in this way on each of the remaining 30 squares, how many grains of wheat should be placed on square ? Also find the total number of grains of wheat on the board at this time and their total weight in pounds. (Assume that each grain of wheat weighs 1/7000 pound.)
Answers
The number of grains on square 13 will be 4096 grains.
How to calculate the number of grain?No. of grains on the first square = 1 = 2^0
No. of grains on the second square = 2 = 1*2 = 2¹
No. of grains on the third square = 4 = 2*2 = 2²
No. of grains on the fourth square = 8 = 2*4 = 2³
From the pattern it could be seen that amount of wheat is doubled for every next square
So, the number of grains in the nth square = 2^n-1
Therefore, No. of grains on 13th square
= 2^13 -1
= 4096
Learn more about sequence on:
https://brainly.com/question/6561461
#SPJ1
sat math scores follow a normal distribution with a mean of 511 and a standard deviation of 110. suppose we choose a student at random. what is the probability that the student scores between 450 and 600?
Answers
The probability that a student scores between 450 and 600 on the SAT math section is approximately 0.4147 or 41.47%.
To find the probability that a student scores between 450 and 600 on the SAT math section, we need to use the properties of the normal distribution. We know that the mean is 511 and the standard deviation is 110.
First, we need to standardize the values of 450 and 600 using the formula:
z = (x - μ) / σ
where x is the value we want to standardize, μ is the mean, and σ is the standard deviation.
For 450:
z = (450 - 511) / 110 = -0.55
For 600:
z = (600 - 511) / 110 = 0.81
Next, we need to find the area under the normal curve between these two standardized values. We can use a table or a calculator to find that the area between z = -0.55 and z = 0.81 is approximately 0.4147.
Know more about probability here:
https://brainly.com/question/30034780
#SPJ11
Help me pookie dookie

Answers
Hope this helps :p
Answer:
1/4
Step-by-step explanation:
poooooooooooooooooooooooooooooooooooookie doooooooooooooooooooooooooooooooookie
Use the distubitive property to write an expression that is equivilent to 3(7+x)
Answers
The solution to the expression 3(7 + x) is 21 + 3x
How to apply the Distributive Property?From the question, we have the following parameters that can be used in our computation:
3(7+x)
Rewrite the expression properly
So, we have the following representation
3(7 + x)
Open the bracket using the Distributive Property
So, we have the following representation
3(7 + x) = 3 * 7 + 3 * x
Evaluate the products on both sides of the equation
3(7 + x) = 21 + 3x
Hence, the solution is 21 + 3x
Read more about the Distributive Property at
brainly.com/question/4770445
#SPJ1
a ___ equation is an equation that contains a variable within a radical expression.
Answers
A radical equation is an equation that contains a variable within a radical expression.
To know more about radical equation refer here:
https://brainly.com/question/9370639
#SPJ11
Translate the sentences into an equation the product of Rhondas savings and 4 is 52us the variable r to represent Rhondas savings
Answers
multiplicationAnswer:
4r = 52
Explanation:
If we represent Rhondas saving by the letter r, the product of r and 4 is written as
\(4\times r\)we are told that this must equal 52, and therefore,
\(4\times r=52\)We can assume that the mulipication sign is there and rewrite the above as
\(4r=52\)cuanto da 1 sobre 3 convertidos en un decimal?
Answers
converting 1/3 to decimal will give 0.3333
What are decimals?The decimal numeral system is the standard system for denoting integer and non-integer numbers. It is the extension to non-integer numbers of the Hindu–Arabic numeral system. The way of denoting numbers in the decimal system is often referred to as decimal notation.
Decimals occur in fractions when both the numerator and the denominator are not a factor of each other. For example 3/5. 3/5 can be expressed in decimal by bringing out the number of 5 in tens of 3. This will be represented with decimal point behind i.e 3/5 = 0.6
Similarly 1/3 = 0.333.....
The value of 1/3 to decimal is continuous, we stop at a convenient point.
Therefore 1/3 = 0.333
learn more about decimal from
https://brainly.com/question/1827193
#SPJ1
Write an explicit formula for a n , the n ^ (th) term of the sequence =6,-30,150,....
Answers
Answer:
\(t(n) = -5^{n-1}*6\)
Step-by-step explanation:
\(t(n) = -5^{n-1}*6\)
PLEASE HELP ASAP ILL GIVE 11 points

Answers
Answer:
11
Step-by-step explanation:
6^2 = 6 x 6 = 36
5^2 = 5 x 5 = 25
36 - 25 = 11
Step-by-step explanation:
everything can be found in the picture above

Ashley makes house calls. For each she is payed a base amount and makes additional money for each hour she works. The graph below shows her pay(in dollars) versus the number of hours worked.
How much does her pay increase for each hour?

Answers
Answer:
her pay increases by 30$ each hour she work
Step-by-step explanation:
hope it helps
find f-1 (x)for the function f(x)=x/3
PLEASE HELP QUICK

Answers
Answer:
f(x)^-1=3x
Step-by-step explanation:
f(x)^-1=3x if we multiple it by x/3 you get result 1
Mr. Zuro finds the mean height of all 16 students in his statistics class to be 67.0 inches. Just as Mr. Zuro finishes explaining how to get the mean, Danielle walks in late. Danielle is 63.6 inches tall. What is the mean height of the 17 students in the class?
Answers
Answer:
66.8 inches
Step-by-step explanation:
The mean height of 16 students is 67.0"
Total height of 16 students = 67 x 16 = 1,072"
When Danielle walks into the class an additional height of 63.6" is added to the total and the new total becomes 1072 + 63.6 = 1135.6"
Since there are now 17 students, mean = 1135.6/17 = 66.8"
Omar has four times as many apples as bananas. He has 30 pieces of fruit in all. If a represents the number of apples and b represents the number of bananas, how many of each fruit does Omar have? Use the table to answer the question
Answers
Answer:
6 bananas, 24 bapples.
Step-by-step explanation:
If Omar has 4 times as many apples as bananas: then if a is the number of apples and b is the number of bananas, then:
Let's say there are b bananas
Therefore there must be 4b apples
In total that gives 5b fruit.
Therefore since there are 30 fruit in all:
5b = 30
Therefore, b = 6
And since there are 30 fruit overall, 30 - 6 = 24
So there 24 apples overall and 6 bananas.
Answer: 6 bananas, 24 bapples.
Step-by-step explanation: I’m so tired HELP MEEEEE!
The price of gasoline drops from $1.00 per
gallon to 95° per gallon. What is the percent of
decrease?
Answers
lmk if it’s the wrong answer
what is the factored form 8g^2+6g-9
Answers
Answer:
A.
Step-by-step explanation:
You purchase 3 action figures per week. You have 27 action figures, which is 60% of the total number of action figures you need to complete your collection. How many weeks until your collection is complete?
Answers
45-27=18
18/3 per week=6 weeks
how many numbers can you get by multiplying two or more distinct members of the set $\{1,2,3,5,11\}$ together?\
Answers
You can get 11 different numbers by multiplying two or more distinct members of the set (1, 2, 3, 5, and 11) together.
To find the number of numbers you can get by multiplying two or more distinct members of the set {1, 2, 3, 5, 11} together, we can use the concept of combinations. There are 5 elements in the set, and we want to choose 2 or more elements to multiply.
To choose 2 elements, there are 5C2 = 10 combinations.
To choose 3 elements, there are 5C3 = 10 combinations.
To choose 4 elements, there are 5C4 = 5 combinations.
To choose all 5 elements, there is 1 combination.
However, we need to subtract the cases where we multiply by 1, as 1 does not change the result.
For choosing 2 elements including 1, there are 4C1 = 4 combinations.
For choosing 3 elements including 1, there are 4C2 = 6 combinations.
For choosing 4 elements including 1, there are 4C3 = 4 combinations.
For choosing all 5 elements including 1, there is 1 combination.
Subtracting the cases with 1, we get:
(10 - 4) + (10 - 6) + (5 - 4) + (1 - 1) = 6 + 4 + 1 + 0 = 11 numbers.
So, you can get 11 different numbers by multiplying two or more distinct members of the set {1, 2, 3, 5, 11} together.
Visit here to learn more about combinations:
brainly.com/question/28042664
#SPJ11
If they work together, Mark and Yvonne can sort 50 library books in 1.5 hours. Working alone, it would take
Yvonne 5 hours to do the same job. How long would it take Mark if he worked alone? Express your answer in
hours and minutes.
veres
e out of
Answers
Answer:
B
Step-by-step explanation:
