Using the intermediate value theorem, determine, if possible, whether the function f has at least one real zero between a and b.f(x) = x^4 − 2x^2 − 8a = 2b = 5Select the correct choice below and, if necessary, fill in the answer boxes to complete your choice.a) By the intermediate value theorem, the function has at least one real zero between a and b because f(a) = _____ and f(b) = _____b) By the intermediate value theorem, the function does not have at least one real zero between a and b because f(a) = _____ and f(b) = _____c) It is impossible to use the intermediate value theorem in this case.
Answers
a) By the intermediate value theorem, the function has at least one real zero between a and b because f(a) = (a^4 - 2a^2 - 8) < 0 and f(b) = (b^4 - 2b^2 - 8) > 0. b) By the intermediate value theorem, the function does not have at least one real zero between a and b because f(a) = (a^4 - 2a^2 - 8) < 0 and f(b) = (b^4 - 2b^2 - 8) > 0. (c)The given statement is true "It is impossible to use the intermediate value theorem in this case." Because there are opposite signs of f(a) and f(b)
a) By the intermediate value theorem, the function has at least one real zero between a and b because f(a) = (a^4 - 2a^2 - 8) < 0 and f(b) = (b^4 - 2b^2 - 8) > 0.
Therefore, f(a) and f(b) have opposite signs and since f is a polynomial function and therefore continuous, by the intermediate value theorem, there exists at least one real zero of f between a and b.
So the correct choice is: a) By the intermediate value theorem, the function has at least one real zero between a and b because f(a) = (a^4 - 2a^2 - 8) < 0 and f(b) = (b^4 - 2b^2 - 8) > 0.
We cannot select b) because f(a) and f(b) have opposite signs and hence, f must have at least one zero between a and b. It is also not impossible to use the intermediate value theorem in this case.
To know more about intermediate value theorem:
https://brainly.com/question/30403106
#SPJ4
Related Questions
In a random sample of 200 school district residents, 94 stated they are in favor of starting the school day 15 minutes later each day. Calculate a 90% confidence interval for the true proportion of district residents who are in favor of starting the day later
Answers
The 90% confidence interval for the proportion of district residents in favor of starting the school day 15 minutes later is (0.392, 0.548). The true proportion is estimated to lie within this interval with 90% confidence.
To calculate the 90% confidence interval for the true proportion of district residents who are in favor of starting the school day 15 minutes later, we can use the following formula:
CI = p ± z*(√(p*(1-p)/n))
where:
CI: confidence interval
p: proportion of residents in favor of starting the day later
z: z- score based on the confidence level (90% in this case)
n: sample size
First, we need to calculate the sample proportion:
p = 94/200 = 0.47
Next, we need to find the z- score corresponding to the 90% confidence level. Since we want a two-tailed test, we need to find the z- score that cuts off 5% of the area in each tail of the standard normal distribution. Using a z-table, we find that the z- score is 1.645.
Substituting the values into the formula, we get:
CI = 0.47 ± 1.645*(√(0.47*(1-0.47)/200))
Simplifying this expression gives:
CI = 0.47 ± 0.078
Therefore, the 90% confidence interval for the true proportion of district residents who are in favor of starting the school day 15 minutes later is (0.392, 0.548). We can be 90% confident that the true proportion lies within this interval.
Learn more about the confidence interval here: brainly.com/question/24131141
#SPJ4
What is the sum of the pink and green area?

Answers
Answer:
I think the answer is 11x
Answer:
11x
Step-by-step explanation:
Pink area = 6x
Green area = 5x
Total (sum) = 6x+5x =11x
Susie and friends rent 3 life jackets and a boat. The life jacket rents for $5.00 each. The boat rents for $25.00 per hour. The total cost is $115.
What is an equation that can be written to represent this situation? Explain your steps.
Answers
3(5) + 25x = 115
15 + 25x = 115
15 + 25(4) = 115
115 = 115
Find the value of X:

Answers
Answer:
Step-by-step explanation:
If AS is an angle bisector, then angle XAS is congruent to angle PAS; mathematically, that looks like this:
3x + 15 = 2x + 30 and x = 15.
3(15) + 15 = angle XAP, which is 120.
Because angle XAS is congruent to angle PAS, then the sides across from those congruent angles also should be congruent. Let's check it, just to make sure:
XS = PS --> 2(15) + 9 = 39 and 4(15) - 21 = 39. So that checks out. Because AS is congruent to itself by the reflexive property, then what this means is that triangle XAS is congruent to triangle PAS, making angle x and P the same measure. Looking at the whole big triangle, triangle XAP, if angle XAP measures 120, then angle X = angle P = (180 - 120) / 2, so angle X = 30.
The times taken by Amal to run three races were 3 minutes 10 seconds, 2 minutes 58.2 seconds and 3 minutes 9.8 seconds. Find the average time taken, giving your answer in minutes.
Answers
Approximately it is:
3 minutes 19 seconds
PLEASE HELP I WILL MARK BRAINLIEST!!!
Select the correct answer
from each drop-down menu.
Brian makes an investment in his company's stock. What does the stock chart say about the stock price today?
The down arrow indicates the stock price has decreased from yesterday's price of $___
at the _____ of the market day.
Option price 1- 66.20 OR 66.26 OR 65.75
Option blank 2- Open OR Close OR High

Answers
Answer: $66.20; close
Step-by-step explanation:
The down arrow indicates the stock price has decreased from yesterday’s price of $ 66.20 at the close of the market day.
7/1 in simplest form
Answers
Answer:
7
Step-by-step explanation:
hope it helps!
Answer:
7
Step-by-step explanation:
7 over one is a whole number
Calculate the rate of change for the quadratic function over the given interval: f\left( x \right) = {x^2} + 4x + 5;\; - 1 \le x \le 2f(x)=x 2 +4x+5;−1≤x≤2 1 5 2 -4
Answers
Answer: The rate of change is 5.
Step-by-step explanation:
Our equation is:
f(x) = x^2 + 4x + 5
And we want to find the rate of change in the range −1≤ x ≤2
when we want to find the rate between x1 ≤ x ≤ x2
we have:
Rate = (f(x2) - f(x1))/(x2 - x1)
So we have:
Rate = ( f(2) - f(-1))/(2 - 1)
Rate = (2^2 + 4*2 + 5 - (-1)^2 - 4*(-1) - 5)/3 = 15/3 = 5
The rate of change is 5.
In a science lab, a number of rock samples are weighed. If the scientist finds one of the rocks to weigh 3 pounds and this is 49. 3% of the total weight of all of the rocks, what is the weight of all of the rocks? If necessary, round your answer to the nearest tenth
Answers
The weight of all the rocks in the science lab is approximately 6.1 pounds.
We are given that one of the rocks weighs 3 pounds, and this weight represents 49.3% of the total weight of all the rocks. Let's denote the total weight of all the rocks as "W".
To find the weight of all the rocks, we set up the equation:
3 = 0.493W
Here, we have multiplied the percentage (49.3%) by the decimal form (0.493).
To solve for W, we divide both sides of the equation by 0.493:
W = 3 / 0.493 ≈ 6.08
The weight of all the rocks, represented by W, is approximately 6.08 pounds.
Since the question asks us to round our answer to the nearest tenth, we round 6.08 to one decimal place, giving us 6.1 pounds as the weight of all the rocks.
To learn more about decimal Click Here: brainly.com/question/30958821
#SPJ11
If an object travels for 10 seconds at a velocity of 50 feet per second, how far did it travel?
Answers
Answer:
500
Step-by-step explanation:
50x10 = 500
50 feet per second so we multiply 50 (the speed per second) by 10 (the time given). You can also use a formula like this:
S x T
S= Speed
T= Time
Let f(x) = 3 -2+x, if x <3 4 - x, if x 2 3 Evaluate the following expressions. lim f(x) = x-+3- lim f(x) = x-+
3+ f (3 ) = Is the function f continuous at 3? ?
Answers
To evaluate the limit of f(x) as x approaches 3 from the left, we substitute the value of x that is slightly less than 3 into the first part of the piecewise function. Therefore\(, lim f(x)\)as x approaches 3- is equal to lim (3 - 2 + x) as x approaches 3-. Simplifying further, we get lim f(x) as x approaches 3- = 3 - 2 + 3 = 4.
To evaluate the limit of f(x) as x approaches 3 from the right, we substitute the value of x that is slightly greater than 3 into the second part of the piecewise function. Therefore, lim f(x) as x approaches 3+ is equal to lim (4 - x) as x approaches 3+. Simplifying further, we get lim f(x) as x approaches 3+ = 4 - 3 = 1. Now, to determine whether the function f is continuous at x = 3, we compare the left-hand limit\((lim f(x)\)as x approaches 3-) and the right-hand limit \((lim f(x)\) as x approaches 3+).
If the two limits are equal and the function value at x = 3 (f(3)) is equal to the limits, then the function is continuous at x = 3. In this case, the left-hand limit is 4, the right-hand limit is 1, and the function value at x = 3 is not given. Since the left-hand and right-hand limits are not equal, the function f is not continuous at x = 3.
Learn more about the left-hand limit here: brainly.com/question/33064616
#SPJ11
when using a graduated pipet, at which point do you measure the volume?
Answers
The volume is measured at the bottom of the meniscus when the liquid level is between the two etched marks on the pipet.
1. Place the graduated pipet on a flat surface and make sure it is level
2. Draw the liquid up until the meniscus is level with the etched line that corresponds to the desired volume
3. Make sure the liquid is not touching the etched line above it
4. Observe the bottom of the meniscus and take the reading at the point where the liquid level is between the two etched lines
The volume is measured at the bottom of the meniscus when the liquid level is between the two etched marks on the pipet.
Learn more about volume here
https://brainly.com/question/16134180
#SPJ4
let u d 2 4 5 6 7 3 5 , and let w be the set of all x in r 3 such that u ? x d 0. what theorem in chapter 4 can be used to show that w is a subspace of r 3 ? describe w in geometric language.
Answers
Geometrically, S represents a plane in ℝ^3 that is orthogonal (perpendicular) to the vector m.
How to solve
To establish that S is a subspace of ℝ^3, we must confirm that S is closed under vector summation and scalar multiplication.
A pertinent theorem from linear algebra that can be applied here is the "Subspace Criterion" theorem. This principle states that a non-empty subset S of a vector space V is a subspace if and only if it fulfills the following conditions:
For any vectors a, b ∈ S, their addition a + b ∈ S.
For any vector a ∈ S and any scalar k, the product ka ∈ S.
Now, let's define a vector m = v - e.
Observe that for any y in S, we have:
m • y = (v - e) • y = (v • y) - (e • y) = 0.
This implies that y is orthogonal to the vector m.
Geometrically, S represents a plane in ℝ^3 that is orthogonal (perpendicular) to the vector m.
As the plane is closed under vector summation and scalar multiplication, S is indeed a subspace of ℝ^3.
Read more about vector here:
https://brainly.com/question/28047791
#SPJ1
Suppose v = (2, 4, 5) and e = (6, 7, 3) are a pair of vectors in ℝ^3. Let S be the collection of all y in ℝ^3 such that the inner product of v and y equals the inner product of e and y (i.e., v • y = e • y).
What principle can be utilized to demonstrate that S is a subspace of ℝ^3? Describe S in geometric terms.
A principal wishes to implement a decision that has to be a number between 0 and 1; that is, a decision d needs to be implemented where 0 sdS1. The difficulty for the principal is that she does not know what decision is appropriate given the current state of the economy, but she would like to implement a decision that exactly equals what is required given the state of the economy. In other words, if the economy is in state s (where 0 sS 1) the principal would like to implement a decision d s as the principal's utility Up (or loss from the maximum possible profit) is given by Up--s-d With such a utility function, maximising utility really means making the loss as small as possible. For simplicity, the two possible levels of s are 0.4 and 0.7, and each occurs with probability 0.5 There are two division managers A and B who each have their own biases. Manager A always wants a decision of 0.4 to be implemented, and incurs a disutility Ua that is increasing the further from 0.4 the decision d that is actually implement, specifically U-0.4-d.Similarly, Manager B always wants a decision of 0.7 to be implement, and incurs a disutility UB that is (linearly) increasing in the distance between 0.7 and the actually decision that is implemented - that is Ug--10.7 Each manager is completely informed, so that each of them knows exactly what the state of the economy s is (a) The principal can opt to centralise the decision but before making her decision given she does not know what the state of the economy is - she asks for recomm endation s from her two division mana gers. Centralisation means that the principal commits to implement a decision that is the average of the two recommendations she received from her managers. The recommendations are sent simultaneously and cannot be less than 0 or greater than 1 Assume that the state of the economy s = 0.7. What is the report (or recommendation) that Manager A will send if Manager B always truthfully reports s? (b) Again the principal is going to centralise the decision and will ask for a recommendation from both managers, as in the previous question. Now, however assume that both managers strategically make their recommendations. What are the recommendations rA and rB made by the Managers A and B, respectively, in a Nash equilibriunm
Answers
A. Manager A wants the decision to be 0.4, so they would recommend a decision of 0.4 to the principal.
B. The recommendations in the Nash equilibrium would be rA = 0.4 and rB = 0.7.
(a) If Manager B always truthfully reports the state of the economy (s = 0.7), Manager A would send a recommendation that minimizes their disutility Ua. In this case, Manager A wants the decision to be 0.4, so they would recommend a decision of 0.4 to the principal.
(b) In a Nash equilibrium, both managers strategically make their recommendations based on their own utility. Manager A wants to minimize their disutility Ua, which increases as the decision deviates from 0.4. Manager B wants to minimize their disutility UB, which increases as the decision deviates from 0.7.
To find the Nash equilibrium, we need to consider the recommendations made by both managers simultaneously. Let's denote the recommendations as rA (from Manager A) and rB (from Manager B). The principal's decision, d, would be the average of the recommendations, so d = (rA + rB) / 2.
Given that both managers strategically choose their recommendations, they will aim to minimize their disutility. In this case, Manager A would recommend a decision of 0.4 (as it minimizes Ua), and Manager B would recommend a decision of 0.7 (as it minimizes UB). Therefore, the recommendations in the Nash equilibrium would be rA = 0.4 and rB = 0.7.
Learn more about Nash equilibrium from
https://brainly.com/question/34120544
#SPJ11
how many virtual pages can a virtual address space in this system have? how large is each page? express your answer in kb, mb, or gb, whichever is most appropriate.
Answers
The virtual address space in this system can have 2^20 virtual pages, with each page having a size of 256 MB. The total virtual address space is 256 GB.
The virtual address space of 248 bytes can be represented as:
2^48 bytes
Each virtual page number is 20 bits long, which gives a total of 2^20 virtual pages.
The size of each page can be calculated by finding the number of bits used to represent the offset. Since the right 28 bits of the virtual address are used for the offset, the page size is 2^28 bytes.
Converting to a more appropriate unit, we have:
Page size = 2^28 bytes = 256 MB
Therefore, the virtual address space has:
Number of virtual pages = 2^20
Page size = 256 MB
To calculate the total size of the virtual address space, we can multiply the number of virtual pages by the page size:
Total virtual address space = Number of virtual pages x Page size
= 2^20 x 256 MB
= 262,144 MB
= 256 GB
Learn more about virtual address space here
brainly.com/question/29573538
#SPJ4
The given question is incomplete, the complete question is:
A certain computer provides its users with a virtual address space of 2^48 bytes. The computer has 2^34 bytes of physical memory. The virtual memory is implemented by paging with virtual addresses formatted as follows: the left 20 bits are used for the Virtual Page Number and the right 28 bits are used for the OFFSET. How many virtual pages can a virtual address space in this system have? How large is each page? Express your answer in KB, MB, or GB, whichever is most appropriate.
Write each of the following three statements in symbolic form and determine which pairs are logically equivalent. Include truth tables and a few words of explanation.
If it walks like a duck and it talks like a duck, then it is a duck.
Either it does not walk like a duck or it does not talk like a duck, or it is a duck.
If it does not walk like a duck and it does not talk like a duck, then it is not a duck.
Answers
Answer:
If it walks like a duck and it talks like a duck, then it is a duck.
and
Either it does not walk like a duck or it does not talk like a duck, or it is a duck.
are logically equivalent to each other.
but neither of the two is logically equivalent to
If it does not walk like a duck and it does not talk like a duck, then it is not a duck.
Step-by-step explanation:
Given statements:
Statemen 1:
If it walks like a duck and it talks like a duck, then it is a duck.
Statement 2:
Either it does not walk like a duck or it does not talk like a duck, or it is a duck.
Statement 3:
If it does not walk like a duck and it does not talk like a duck, then it is not a duck.
Let
p be the statement: it walks like a duck q be the statement: it talks like a duck r be the statement: it is a duckUsing p = it walks like a duck , q =it talks like a duck, r = it is a duck the given statements can be written in symbolic form as:
Statement 1:
p ∧ q → r
The ∧ symbol shows that if both p and q are true then, they imply r. This means both p and q together imply r
Statement 2:
~p ∨ ~q ∨ r
Here the statement p and q are negated and joined using or. So either negation p or negation of q or r (alternative)
Statement 3:
~p ∧ ~q → ~r
The ∧ symbol shows that if both negation of p and negation of q are true then, they imply r. This means both negated p and negated q together imply negated r
Statement 1:
p ∧ q → r ≡ ~(p∨q) ∨ r Using conditional equivalence p→q ≡ ~p ∨ q
≡ (~p ∧ ~q ) ∨ r
You can see it is equivalent to Statement 2 i.e. ~p ∧ ~q → ~r
Hence Statement 1 and Statement 2 are logically equivalent.
Now Statement 3:
~p ∧ ~q → ~r ≡ ~(~p ∧ ~q ) ∨ ~r Using conditional equivalence p→q ≡ ~p ∨ q
≡ ~(~p ) ∨ ~(~q ) ∨ ~r Using De Morgan's Law ~(p∧q) ≡ ~p ∨~q
≡ p ∨ q ∨ ~r Using Double Negation Law ~(~p)≡p
This shows that Statement 3 is neither logically equivalent to Statement 1 nor logically equivalent to Statement 2.
Proof by truth table is attached. The table shows that the columns for Statement 1 and Statement 2 have same truth values.
Hence
"If it walks like a duck, and it talks like a duck, then it is a duck,"
and
"If it does not walk like a duck, and does not talk like a duck, then it is not a duck,"
are logically equivalent.
The table also shows that column for Statement 3 does not match with either of the columns for Statement 1 and Statement 2. So
If it does not walk like a duck and it does not talk like a duck, then it is not a duck.
is not logically equivalent to Statement 1 and Statement 2.

Answer:
duck walk
Step-by-step explanation:
Find the axis of symmetry and vertex for the parabola y=−x2+2x+8.

Answers
Step 1: Concept
The axis of symmetry always passes through the vertex of the parabola. The x -coordinate of the vertex is the equation of the axis of symmetry of the parabola.
Step 2: Find the axis of symmetry using the formula
\(\begin{gathered} The\text{ general expression for a quadratic equation is} \\ y=ax^2\text{ + bx + c } \end{gathered}\)The axis of symmetry is a vertical line.
\(\begin{gathered} x\text{ = }\frac{-b}{2a} \\ y=-x^2\text{ + 2x + 8} \\ a\text{ = -1 , b = 2 and c = 8} \\ x\text{ = }\frac{-2}{-2} \\ x\text{ = 1} \end{gathered}\)The x -coordinate of the vertex is the equation of the axis of symmetry of the parabola.
Therefore, the axis of symmetry is x= 1
Step 3: Find the vertex
To find the vertex, you substitute x = 1 in the equation of a parabola.
\(\begin{gathered} \text{Therefore, we have} \\ y=-x^2\text{ + 2x + 8} \\ y=-1^2\text{ + 2(1) + 8} \\ \text{y = -1 + 2 + 8} \\ y\text{ = 9} \end{gathered}\)The vertex = (1,9)
What is the recursive formula for this geometric sequence?
-4, -24, -144, -864, ...
= -4
O A.
an =
2n-1 • 30
OB.
la = -4
ar = 2n-16
fa
= -6
C.
= 2n-1 • 30
= -6
O D.
lan
ar = 2n-1.4
Answers
Answer:
a1 = -4
an = an-1 * 6
Step-by-step explanation:
-4, -24, -144, -864, ...
First find the common ratio
Take the second term and divide by the first term
-24/-4 = 6
The common ratio is 6
The recursive formula is
a1 = -4
an = an-1 * 6
Solve for x provide proofs pleaseee

Answers
Answer:
x = ~4.40 or \(\sqrt{19.37}\)
Step-by-step explanation:
This is a right triangle, so we will use the Pythagorean Theorem.
The theorem goes as follows: \(a^2 + b^2 = c^2\)
Let's set x as the a value in the theorem. Plug in 6.8 for b, and 8.1 for c.
\(x^2 + (6.8)^2 = (8.1)^2\)
Then, simplify!
\(x^2 + 46.24 = 65.61\)
Subtract 46.24 on both sides.
\(x^2 = 19.37\)
Take the square root of both sides
\(x =\sqrt{19.37}\)
Voila!
if Sudi and Tosha are eating pancakes. If they each can eat one pancake in 3 minutes, how long will it take the two of them to eat 10 pancakes?
Answers
Answer:
30 mins i think its jus simple maths unless theres a twist
This figure consists of a rectangle and a quarter circle.
What is the perimeter of this figure?
Use 3. 14 for π.
Enter your answer as a decimal in the box.
Answers
The perimeter of the figure is the sum of the lengths of all its sides. To calculate it, we need to find the perimeter of the rectangle and the circumference of the quarter circle and then add them together.
To find the perimeter of the rectangle, we need to sum up the lengths of all its sides. Let's assume the length of the rectangle is 'l' and the width is 'w'. Therefore, the perimeter of the rectangle is 2(l + w).
For the quarter circle, we need to find the circumference. The formula for the circumference of a circle is 2πr, where π is approximately 3.14 and 'r' is the radius. In this case, the radius is equal to half of the width of the rectangle. So the circumference of the quarter circle is 0.5πw.
To find the total perimeter, we add the perimeter of the rectangle and the circumference of the quarter circle:
Perimeter = 2(l + w) + 0.5πw
Now, you can substitute the given values for 'l' and 'w' to find the perimeter. Remember to use the approximation 3.14 for π.
learn more about perimeter here:
https://brainly.com/question/7486523
#SPJ11
Lmk if you understand thanks

Answers
Answer:
y = 100,000 (1 + 0.04) ²⁰
Step-by-step explanation:
Here:
100,000 = original amount.
0.04 = rate (a percent)
and
20 = number of times you need to run the simulation.
What is the value of x? enter your answer in the box. x = cm
Answers
The value of x in the given equation will be 2/5
From the data,
We have to determine the value of x.
The given equation is: 18x-16=-12x-4
For determining the value of x, we will first shift the like terms on one side of the equation.
So, for solving the value of x we will shift the terms containing x and the constant on both sides of the equation.
So, shifting -12x from the right-hand side of the equation to the left-hand side of the equation,
We will get it as:
18x+12x = -4+16
30x=12
Now for solving the value of x we will shift x from the left side of the equation to the right side of the equation.
So, the value of x will be = 12/30 = 2/5
For more questions on Equation
https://brainly.com/question/25678139
#SPJ4
The correct question may be:
What is the value of x
18x-16=-12x-4
Enter your answer in the box.
A dairyman wishes to mix milk containing 5% butterfat and cream containing 75% butterfat to produce a total mixture of 56 liters. This final mixture should contain 53% butterfat. How much of the milk mixture and how much of the cream mixture should he use
Answers
18% of the milk mixture and how much of the cream mixture should he use.
How much of the milk mixture and how much of the cream mixture should he use?A dairyman wants to combine milk with 5 percent butterfat and cream with 75 percent butterfat to create a 56-liter combination. Butterfat should make up 53% of the final combination.
Let x represent the volume of MILK required for the combination, in liters.
Consequently, x/56 is the PROPORTION of milk in the mixture. [because the final mixture has 56 liters in total]
We require 56 - x liters of CREAM in the mixture because we have 56 liters overall in the mixture.
The PROPORTION of cream in the combination is therefore equal to (56 - x)/56.
Our goal is for the final mixture to have 75% butterfat.
Fill in the equation with each of these values to obtain:
50 = (x/60)(5) + ((60 - x)/60) (75)
Add 56 to both sides to get: 3000 = (5)(x) + (56 - x)(75)
The formula is:
Multiply both sides by 56 to get: 3000 = (5)(x) + (56 - x)(75)
Expand: 3000 = 5x + 4200 - 75x
Simplify: 3000 = 4200 - 70x
Subtract 4500 from both sides: -1300 = -70x
Solve: x = (-1300)/(-70) = (1300)/(70) = 130/7
If you don't want to divide 130 by 7, you can evaluate this quickly by first realizing that 30/7 = 18.
Consequently, 130/7 must be a little larger than 18.
Learn more about milk mixture here:
https://brainly.com/question/11026475
#SPJ4
PLEASE HELP MEEEEEEEEE
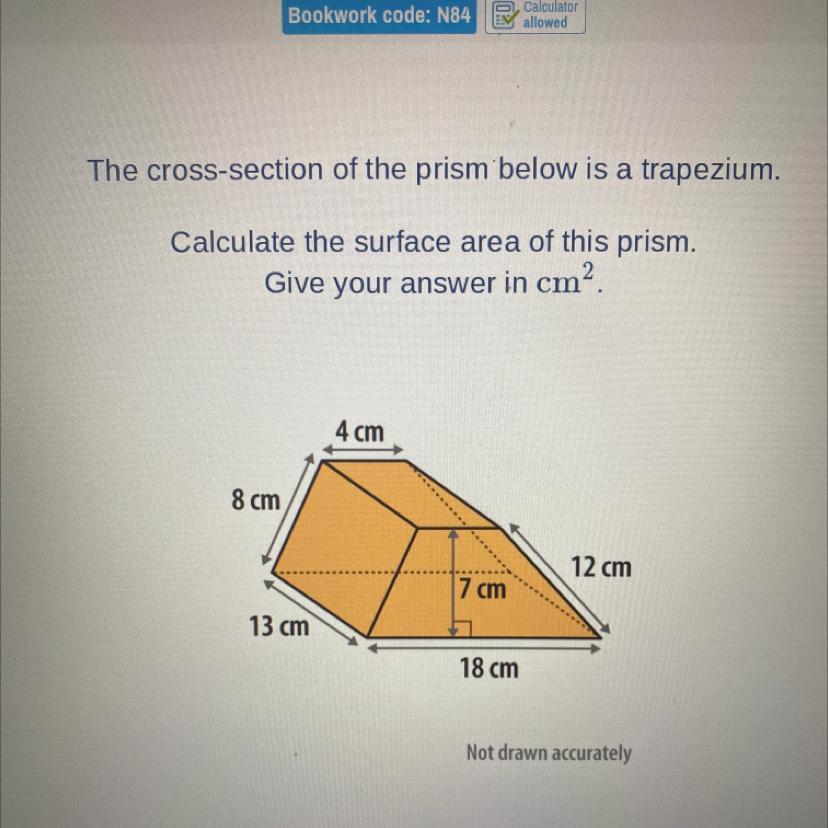
Answers
The top and bottom faces of the prism are rectangles with dimensions 12 cm by 17 cm, so each face has an area of:
12 cm x 17 cm = 204 cm²
The front and back faces of the prism are trapezoids with bases 8 cm and 13 cm, height 4 cm, and slant height √(4² + (13-8)²) = √(16 + 25) = √41 cm. Therefore, each face has an area of:
1/2 x (8 cm + 13 cm) x 4 cm x √41 cm ≈ 100.07 cm²
The left and right faces of the prism are trapezoids with bases 8 cm and 13 cm, height 17 cm, and slant height √(17² + (13-8)²) = √(289 + 25) = √314 cm. Therefore, each face has an area of:
1/2 x (8 cm + 13 cm) x 17 cm x √314 cm ≈ 571.69 cm²
To find the total surface area, we add up the areas of all six faces:
Total surface area = 2(204 cm²) + 2(100.07 cm²) + 2(571.69 cm²) = 1743.52 cm²
Therefore, the surface area of the prism is 1743.52 cm².
A large green jar of candy contains red, green, and yellow candy. The probability of pulling a red candy out of the jar is 1/4. The probability of pulling a green candy out of the jar is 5/8. What is the probobility of randomly pulling a yellow candy out of the jar
Answers
Answer:
1/8
Step-by-step explanation:
total probability = 1
total probability = probability of red + probability of green + probability of yellow
1 = 1/4 + 5/8 + yellow
we want to find a common denominator
in this case, we know 4 * 2 = 8, so we can multiply 1/4 by 2/2 = 1 to get
1/4 * 2/2 = 2/8
1 = 2/8 + 5/8 + yellow
1 = 7/8 + yellow
subtract 7/8 from both sides to isolate yellow
1 = 8/8
1 - 7/8 = 8/8 - 7/8 = 1/8 = yellow
Robert works at a car dealership. Each month, he receives a base salary of $1,854.00, plus a commission of $478.00 for each vehicle he sells. Which of the following equations could be used to determine Robert's total income each month? (Let x represent the number of cars sold by Robert and y represent his total monthly income.)
Answers
Answer:
y = 478x + 1,854
Step-by-step explanation:
what is the solution to the inequality below? 2(x-15)> x/2
Answers
Answer:
x>20
Step-by-step explanation:
2(x-15)>x/2
2x-30>x/2
Multiply both sides by 2
4x-60>x
Subtract 4x from both sides
-60>3x
-20<x
Answer: x>20
Answer:
The correct answer is x ≥ 20.
Determine the x- and y-coordinates of the centroid of the shaded area. assume c = 5, a = 1.30, b = 2.25.
Answers
The x-coordinate and y-coordinate of the centroid is approximately 0.323 and 1.244 respectively.
To determine the x- and y-coordinates of the centroid of the shaded area,
Calculate the centroid coordinates for each individual shape
and then find the weighted average of those coordinates based on the area of each shape.
Let's break down the shaded area into two shapes: a rectangle and a semicircle.
Rectangle,
The width of the rectangle is 'a' and the height is 'c'
The centroid of a rectangle is located at the midpoint of its height and width.
x-coordinate of rectangle centroid
= a/2
= 1.30/2
= 0.65
y-coordinate of rectangle centroid
= c/2
= 5/2
= 2.50
Semicircle,
The radius of the semicircle is 'b'
The centroid of a semicircle is located at a distance of 4/3πr from the base of the semicircle.
x-coordinate of semicircle centroid = 0
y-coordinate of semicircle centroid
= (4/3πb)/2
= (4/3π × 2.25)/2
≈ 3.77
Now, find the weighted average of the centroids based on the area of each shape.
Total area of the shaded region = area of rectangle + area of semicircle
Total area = a × c + (1/2 × π × b²)/2
Total area = 1.30 × 5 + (1/2 × π ×2.25²)/2
Total area ≈ 9.09 + 3.98
Total area ≈ 13.07
Now ,calculate the weighted centroid coordinates,
x-coordinate of centroid = (area of rectangle × x-coordinate of rectangle centroid + area of semicircle × x-coordinate of semicircle centroid) / total area
x-coordinate of centroid = (1.30 × 5 × 0.65 + (1/2 × π × 2.25²)/2 × 0) / 13.07
x-coordinate of centroid ≈ 4.225 / 13.07
x-coordinate of centroid ≈ 0.323
y-coordinate of centroid
= (area of rectangle × y-coordinate of rectangle centroid + area of semicircle × y-coordinate of semicircle centroid) / total area
= (1.30 × 5 × 2.50 + (1/2 × π × 2.25²)/2× 3.77) / 13.07
≈ 16.25 / 13.07
≈ 1.244
Therefore, the x-coordinate of the centroid is approximately 0.323 and the y-coordinate of the centroid is approximately 1.244.
learn more about centroid here
brainly.com/question/29730343
#SPJ4
The information provided is insufficient to determine the coordinates of the centroid of the shaded area. The specific shape of the shaded area and its orientation in the coordinate plane are necessary information to solve this problem.
Explanation:The original question involves determining the x- and y-coordinates of the centroid of the shaded area for given values of c = 5, a = 1.30, and b = 2.25. However, the question is missing information about the specific shape of the shaded area, which is crucial to calculate its centroid coordinates. Typically, for basic geometric shapes, the formula used to calculate the centroid is different for each type of shape.
For instance, if the shaded area is a triangle, its centroid coordinates (x, y) can be determined as follows:
x-coordinate = (a + b + c) / 3y-coordinate = (a + b + c) / 3Where a, b, and c represent the x-coordinates of the vertices of the triangle. However, without information about the shape, an accurate calculation of the centroid cannot be made.
It's also important to note that the provided references do not apply directly to this problem, as they concern different mathematical contexts and principles.
Learn more about Centroid Calculation here:https://brainly.com/question/35459746
#SPJ6
Solving one step equations:
Multiply or divide by the same amount on both sides so that the variable is by itself.
9i = -63
Answers
Answer;
i=-7
Step-by-step explanation;
TBH we have all we need its given to us in the question, divide both sides by the same amountt
9i=-63
divide by 9 to leave i alone
i=-7the one step equation is solved
Answer:
\( \sf \: i = - 7\)
Step-by-step explanation:
Now we have to,
→ find the required value of i.
The equation is,
→ 9i = -63
Then the value of i will be,
→ 9i = -63
→ (9i) ÷ 9 = (-63) ÷ 9
→ (i) = (-7)
→ [ i = -7 ]
Hence, the value of i is -7.