Two curves are orthogonal if their tangent lines are perpendicular at each point of intersection. Are the given families of curves orthogonal trajectories of each other? That is, is every curve in one family orthogonal to every curve in the other family?
x^2 + y^2 = ax
x^2 + y^2 = by
a. Yes, every curve in the first family of curves is orthogonal to every curve in the second family of curves. b. No, there are curves in the first family of curves that are not orthogonal to every curve in the second family of curves.
Answers
The correct answer is a. Yes, every curve in the first family of curves is orthogonal to every curve in the second family of curves.
To see why this is true, let’s consider the two families of curves x^2 + y^2 = ax and x^2 + y^2 = by. We can rewrite these equations in terms of y to get y = sqrt(ax - x^2) and y = sqrt(by - x^2). Taking the derivative of both equations with respect to x, we get:
dy/dx = (a/2)(1/sqrt(ax - x^2)) - x/sqrt(ax - x^2) for the first family of curves and
dy/dx = (b/2)(1/sqrt(by - x^2)) - x/sqrt(by - x^2) for the second family of curves.
Now let’s consider a point (x0,y0) that lies on both a curve from the first family and a curve from the second family. This means that x0^2 + y0^2 = ax0 and x0^2 + y0^2 = by0. Solving these equations for a and b, we find that a = (x0^2 + y0^2)/x0 and b = (x0^2 + y0^2)/y0.
Substituting these values into our expressions for the derivatives above, we find that at the point (x0,y0), the slope of the tangent line to a curve from the first family is (1/2)(1/y0) - x0/y0 and the slope of the tangent line to a curve from the second family is (1/2)(1/x0) - y0/x0.
Two lines are perpendicular if and only if their slopes have a product of -1. In this case, we can see that at any point (x0,y0) that lies on both a curve from the first family and a curve from the second family, the product of their slopes is indeed -1.
Therefore, every curve in one family is orthogonal to every curve in the other family.
To learn more about orthogonal curves visit: https://brainly.com/question/20308962
#SPJ11
Related Questions
What is the value of Measure of angle a + measure of angle b
3 lines intersect to form 5 angles. Clockwise, from top left, the angles are b, 90 degrees, c, 124 degrees, a.
A. 34°
B. 56°
C. 90°
D. 180°
Answers
Answer:
D)
Step-by-step explanation:
Assuming that the x-axis tick marks will be separated by 5 (5, 10, 15, and so on), what is the largest value that should appear on the x-axis
Answers
The largest value that should appear on the x-axis assuming that the tick marks will be separated by 5 is 95.
Since the tick marks are separated by 5, we can start with the smallest tick mark at 0 and add 5 for each subsequent tick mark.
To determine the largest value that should appear on the x-axis, we need to find the largest multiple of 5 that is less than or equal to the largest value in the data set. In this case, we don't have any information about the data set, so we can't determine the largest value.
However, we can assume that the x-axis should be long enough to accommodate the largest value that we expect to see. If we assume that the largest value is 100, then the largest multiple of 5 that is less than or equal to 100 is 95.
Therefore, the largest value is 95.
To know more about multiple, refer here:
https://brainly.com/question/21920862#
#SPJ11
If integer constraints are added to a linear programming model, then the optimal objective value will improve.
true or false?
Answers
Adding integer constraints to a linear programming model may or may not improve the optimal objective value.
When solving a linear programming problem, the standard approach is to relax the integer constraints and find an optimal solution in the continuous domain. This is known as linear programming (LP) relaxation. However, the optimal solution obtained from the LP relaxation may not satisfy the integer constraints. In such cases, if the integer constraints are added back to the problem, it becomes an integer programming (IP) problem.
The addition of integer constraints introduces discrete decisions into the problem, allowing for more precise control over the variables. In some cases, adding integer constraints can lead to a better optimal objective value because it forces the solution to select values that align with the discrete nature of the problem. This is especially true when the problem exhibits combinatorial or logical structures where discrete choices are crucial.
However, there are instances where adding integer constraints may not improve the optimal objective value. This can happen when the LP relaxation already provides an optimal solution that satisfies the problem's requirements. In such cases, the introduction of integer constraints may restrict the feasible solution space, making it harder to find a better solution.
In summary, while adding integer constraints to a linear programming model has the potential to improve the optimal objective value by incorporating discrete decisions, it is not guaranteed to do so. The impact of integer constraints depends on the problem structure and whether the LP relaxation already provides an optimal solution that meets the problem's criteria.
For more questions like Integer constraints click the link below:
https://brainly.com/question/32597572
#SPJ11
see screenshot below

Answers
Answer:
4x^2 +2x +1
Step-by-step explanation:
(4x^3 -14x^2 -7x-4) / (x-4)
Using synthetic division
Bringing down the 4 and multiplying and adding
4 4 -14 -7 -4
↓ 16 8 4
-------------------------------
4 2 1 0
The quotient is 4x^2 +2x +1 and the remainder is 0
Answer:
\( {4x}^{2} + 2x + 1\)
Step-by-step explanation:
the most handy way to solve the question is Polynomial long division which is done below:
\( \begin{array}{ccc} \qquad {4x}^{2} + 2x + 1 \\ \hline x - 4 \Bigg)4 {x}^{3} - {14x}^{2} - 7x - 4 \\ - {4x}^{3} + 16 {x}^{2} \\ \hline \qquad \qquad \qquad {2x}^{2} - 7x - 4 \\ \qquad \qquad- {2x}^{2} + 8x \\ \hline \qquad \qquad \qquad \qquad \qquad x - 4 \\ \qquad \qquad \qquad \qquad \qquad - x + 4 \\ \hline\qquad \qquad \qquad \qquad \qquad0 \end{array}\)
since our remainder is 0 we'll put the answer in P(x) form only
note: please see the answer in the brainly app for a better view
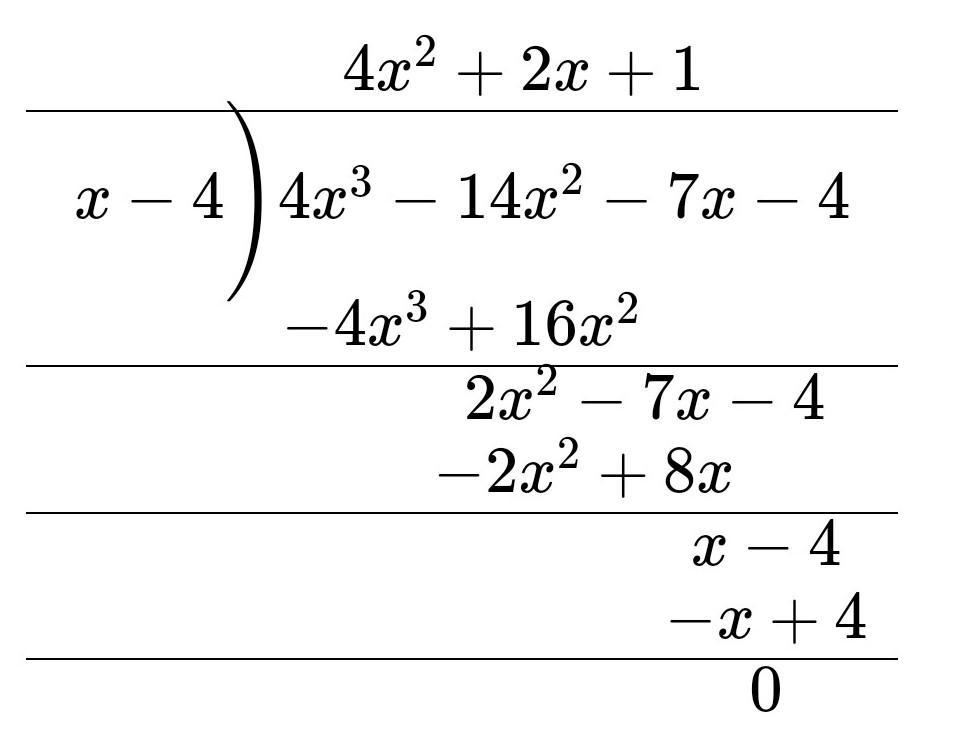
a new crew of painters can paint a small apartment in hours. an experienced crew can paint the small apartment in hours. how many hours does it take to paint the apartment when the two crews work together? it takes blank hours to paint the apartment when the two crews work together. the solution is
Answers
Let's say the new crew of painters can paint the small apartment in x hours, and the experienced crew can paint the small apartment in y hours. Let the time taken by the new crew of painters to paint the small apartment be N hours, and the time taken by the experienced crew be E hours.
We are supposed to find the time it takes for both crews to paint the apartment together.
Step 1: Find the work rate of each crew.
The new crew's work rate is 1/N (apartments painted per hour).
The experienced crew's work rate is 1/E (apartments painted per hour).
Step 2: Calculate the combined work rate of both crews.
Combined work rate = New crew's work rate + Experienced crew's work rate
Combined work rate = (1/N) + (1/E)
Step 3: Find the time it takes for both crews to paint the apartment together.
Let T be the time it takes for both crews to paint the apartment together. Their combined work rate can be expressed as the reciprocal of T.
1/T = (1/N) + (1/E)
Step 4: Solve for T.
T = 1 / [(1/N) + (1/E)]
So, it takes T hours to paint the apartment when the two crews work together. Once you know the specific values of N and E, you can plug them into the equation and solve for T to find the solution.
To learn more about reciprocal : brainly.com/question/18634761
#SPJ11
Solve the system below for x and y in terms of a and b.
\(ax+y=7\\3x-y=b\)
Answers
![Solve the system below for x and y in terms of a and b.[tex]ax+y=7\\3x-y=b[/tex]](https://i5t5.c14.e2-1.dev/h-images-qa/answers/attachments/E0MzG1BcdxhYiYAQ54lED87Rwi3fUdYG.png)
x + y + 8x + 1; use x=6 and y=8
Answers
Answer:
63
Step-by-step explanation:
Step 1:
6 + 8 + 48 + 1
Step 2:
14 + 49
Answer:
63
Hope This Helps :)
Find the general solution of the differential equation dy/dt = 3t2/8y. Choose the correct answer below.
a. y = ±√t^3/4 + C
b. y = 4t^3 + C
c. y = ±√4t^3+C
d. y = t^3/4+C
Answers
Thus, the general solution of the given differential equation dy/dt = 3t^2/8y is y = ±√(4t^3+C).
To find the general solution of the given differential equation dy/dt = 3t^2/8y, we can use separation of variables.
First, rewrite the equation as: (dy/y) = (3t^2/8)dt.
Now, integrate both sides of the equation:
∫(1/y) dy = ∫(3t^2/8) dt.
After integration, we get:
ln|y| = (t^3/8) + C1,
where C1 is the constant of integration.
Now, exponentiate both sides to remove the natural logarithm:
y = e^((t^3/8) + C1).
We can rewrite the constant as follows:
y = e^(t^3/8) * e^C1.
Let C = e^C1, which is also a constant. So,
y = Ce^(t^3/8).
Comparing with the given options, none of them exactly matches our solution. However, option c is the closest to the correct form.
To match the given options, we can rewrite our solution as:
y = ±√(C*4t^3).
This is similar to option c, which is:
y = ±√(4t^3+C).
Note that the given options may not perfectly represent the actual general solution. In this case, the closest answer is option c.
Know more about the differential equation
https://brainly.com/question/1164377
#SPJ11
What is the difference between homogeneous and nonhomogeneous differential equation?
Answers
After answering the provided question, we can conclude that there are two possible outcomes for a homogeneous system of linear equations: either there are several solutions or there is only one, trivial solution.
What is equation?A mathematical equation is a formula that joins two statements and indicates equality with the equal symbol (=). In algebra, an equation is a mathematical statement that establishes the equality of two mathematical expressions. In the equation 3x + 5 = 14, for example, the equal sign separates the variables 3x + 5 and 14. A mathematical formula describes the relationship between the two sentences on either side of a letter. There is frequently only one variable, which also serves as the symbol. For example, 2x - 4 = 2.
For a homogeneous system of linear equations, there are two possible outcomes: either there are several solutions or there is just one, trivial solution. There are three possible outcomes for a non-homogeneous system: either there is only one (unique) solution, several solutions, or no solutions at all.
homogeneous system of linear equations example = \(2x-3y=\\\) and \(-4x+6y=0\)
non-homogeneous system of linear equations example = \(4x-2y+6z=8\) and \(x+y-3z =\)\(-1\)
To know more about equations visit:
brainly.com/question/649785
#SPJ4
a ball thrown by a(n) __________ travels an average speed of 29 feet per second.
Answers
A ball thrown by a(n) person travels an average speed of 29 feet per second.
The average speed is the total distance traveled by the object in a particular time interval. The average speed is a scalar quantity. It is represented by the magnitude and does not have direction.
The word "person" fills the blank in the sentence. This suggests that the sentence is referring to a human individual who throws the ball. By specifying that a person throws the ball, it provides context and clarifies who is responsible for the ball's movement. The sentence implies that the average speed of the ball is 29 feet per second when thrown by a person.
Know more about average speed here:
https://brainly.com/question/10449029
#SPJ11
What is 2x^2-13x+15?
Answers
Answer:
Step-by-step explanation:

Answer:
\( (2x - 3)(x - 5) \\ \)
Step-by-step explanation:
\(2 {x}^{2} - 13x + 15 \\ 2 {x}^{2} - 10x - 3x + 15 \\ 2x(x - 5) - 3(x - 5) \\ (2x - 3)(x - 5)\)
hope this helps you.
brainliest appreciated
good luck! have a nice day!
A monkey is swinging from a tree. On the first swing, she passes through an arc of 20m. With each swing, she passes through an arc 4/5 of the previous swing. What is the total distance the monkey has traveled when she completes her 10th swing?
Answers
The total distance that the monkey has traveled when she completes her 10th swing is of:
89.26 m.
How to obtain the total distance?With each swing, she passes through an arc 4/5 of the previous swing, hence the distance is modeled by a geometric sequence with common ratio of q = 4/5 = 0.8.
On the first swing, she passes through an arc of 20m, hence the first term of the geometric sequence is of 20.
The sum of the first n terms of a geometric sequence is given by the equation presented as follows:
\(S_n = a_1\frac{(r^n - 1)}{r - 1}\)
The parameters for this problem are given as follows:
\(n = 10, a_1 = 20, r = 0.8\)
Hence the total distance the monkey has traveled when she completes her 10th swing is calculated as follows:
Distance = 20 x (0.8^10 - 1)/(0.8 - 1) = 89.26 m.
More can be learned about geometric sequences at https://brainly.com/question/24643676
#SPJ1
Answer:
89
Step-by-step explanation:
khan
What is the place value of 432?.
Answers
Place value of 432 is 400 +30+02 .
What is place value?
In math, each digit in a very variety features a place value. Place value is outlined because the price pictured by a digit in a very variety on the premise of its position within the variety.
Main body:
The place value of a digit will increase by 10 times as we move left on the place worth chart and reduces by ten times as we tend to move right.
The place of digit 4 will be hundreds.
The place of digit 3 will be tens.
The place of digit 2 will be ones.
Hence place value of 432 is 400 +30+02 .
To know more about place value click on the link below
https://brainly.com/question/569339
#SPJ4
Ciara bought four burgers.
She paid with a £20 note and got £6 change.
a
How much did each burger cost?
Answers
£3.50 for each burger
3.50×4= 14 left over is 6 from the 20 pound note
Please I need some answers

Answers
Step-by-step explanation:
1)4+9 = 13
2)9+16 = 25
3)4+16 = 18
4)9+25 = 34
Answer:
i) 13 ii) 25 iii) 20 iv) 34
Step-by-step explanation:
they are all exponents of 2 so you multiply each number by itself once.
i) 2x2 + 3x3
ii) 3x3 + 4x4
iii) 2x2 + 4x4
iv) 3x3 + 5x5
(next time you can use a calculator lol)
tina randomly selects two distinct numbers from the set$$\{1,2,3,4,5\},$$and sergio randomly selects a number from the set$$\{1,2,\ldots,10\}.$$what is the probability that sergio's number is larger than the sum of the two numbers chosen by tina?
Answers
The probability that Sergio's number is larger than the sum of the two numbers chosen by Tina is 0.4
We can calculate the probability by finding the number of favorable outcomes, i.e., the number of ways that Sergio can choose a number that is larger than the sum of the two numbers chosen by Tina, and dividing it by the total number of possible outcomes, which is
5C2 * 10C1 = (5!/(2! * 3!)) * 10
= 100
Tina's sum Sergio's favorable outcomes
3 7
4 6
5 5
6 4
7 3
8 2
9 1
For example the sum 5 can be achieved by two ways, that is 1+4 and 2+3. So
Tina's sum Number of ways to achieve that sum
3 1
4 1
5 2
6 2
7 2
8 1
9 1
The number of favourable outcomes is
= 1*7 + 1*6 + 2*5 + 2*4 + 2*3 + 1*2 +1*1
= 40
So probability is
= 40/ 100
= 0.4
To know more on probability
https://brainly.com/question/5706420
#SPJ4
There are 5 white balls,8 red balls ,7 yellow balls and 4 green balls in a container a ball is choosen at random.what is the probabilty of chooseing neither white or green? .
Answers
15/19 + 14/19 = 29/19
Step-by-step explanation:
Add the number of balls in the basket together.
Subtract the number of white balls from the sample space ( the total amount of balls) your answer is written over the sample space and the same process is done for the green ball
Can somebody plz help me with surveys in math?
Answers
Help plsssssssssssss

Answers
Answer:
a) The sensor will read 75 liters at 5 minutes
Step-by-step explanation:
a) set up as a proportion and cross multiply to solve.
15 liters/1 minute = x/5 minutes
x = 75 liters
I just need the second part please! It is an exponential function

Answers
Solution:
The volume of the cases in 2001 is given below as
\(=17.8billion\)The percentage increase from 2000 is given below as
\(=4\%\)The exponential function is given below as
\(\begin{gathered} y=ab^t \\ where, \\ b=1+r \\ r=4\% \end{gathered}\)By substituting the values, we will have
\(\begin{gathered} y=ab^{t} \\ 17.8=a(1+\frac{4}{100})^1 \\ 1.04a=17.8 \\ \frac{1.04a}{1.04}=\frac{17.8}{1.04} \\ a=17.12billion \end{gathered}\)Hence,
The final answer is YES, THE EXPONENTIAL MODEL FUNCTION IS APPROPRIATE
Therefore,
The exponential model after y years will be
\(C(y)=17.12(1.04)^y\text{ }billion\text{ }cases\)
Abby is using the Distributive Property to find 27 x 52
Drag the correct number into each box to show her work.
Numbers may be used once, more than once, or not at all.
27 x 52 = (20 +
) x (50 +
= (20+7)x
+ (20+7)x
= (20 x 50) + (7 x 50) + (20 X 2) + (7 2)
11
Answers
Answer:
27 x 52 = ( 20 + 7 ) x ( 50 + 2 ) = 1404
Step-by-step explanation:
27 x 52
( 20 + 7 ) x ( 50 + 2 )
(20 x 50) + (20 x 2) + (7 x 50) + (7 x 2)
= 1000 + 40 + 350 + 14
= 1404
(Comparing Data MC)
The data given represents the number of gallons of coffee sold per hour at two different coffee shops.
Coffee Ground
1.5 20 3.5
12 2 4
11 7 2.5
9.5 3 5
Perks A Lot
10 3.5 3
5 2.5 7
8 5.5 9.5
6 9 4.5
Compare the data and use the correct measure of center to determine which shop typically sells the most amount of coffee per hour. Explain.
Perks A Lot, with a mean value of 6.125 gallons
Perks A Lot, with a median value of 6.125 gallons
Coffee Ground, with a mean value of 6.75 gallons
Coffee Ground, with a median value of 6.75 gallons
Answers
The data shows that Coffee Ground typically sells the most amount of coffee per hour.
How to explain the informationThe mean value of the number of gallons of coffee sold per hour at Coffee Ground is 6.75 gallons, while the mean value at Perks A Lot is 6.125 gallons. The median value of the number of gallons of coffee sold per hour at Coffee Ground is also 6.75 gallons, while the median value at Perks A Lot is 6.125 gallons.
The mean is calculated by adding up all of the values in a set of data and dividing by the number of values. The median is calculated by finding the middle value in a set of data after the values have been arranged in order from least to greatest.
In this case, the mean and median values are both higher for Coffee Ground than for Perks A Lot
Learn more about mean on
https://brainly.com/question/1136789
#SPJ1
when lydia performed an operation on the matrix below, she forgot to include one element. what is the value of the unknown element, b? –7 –5 –3 –2
Answers
In the given transformation of the matrix, the value of b is -7. So, the correct answer is option (a) -7.
A matrix is a rectangular array of numbers, often called elements of that matrix. If there are m rows and n columns in a matrix, the order of that matrix is said to be m × n. The element in the ith row and jth column is denoted as \(a_{ij}\).
Let A be the given matrix, then
\(A = \left[\begin{array}{cccc}1&2&3&5\\5&3&-1&-11\\3&2&-2&-13\end{array}\right]\)
Multiply the first row with 5 and subtract the second row from the resultants to transform the second row, i.e., \(R_2 \to R_2-5R_1\)
\(A = \left[\begin{array}{cccc}1&2&3&5\\5-5&3-10&-1-15&-11-25\\3&2&-2&-13\end{array}\right]\)
\(= \left[\begin{array}{cccc}1&2&3&5\\0&-7&-16&-36\\3&2&-2&-13\end{array}\right]\)
Thus, it is obtained that b = -7. Option (a) is correct.
Learn more about Matrix here:
https://brainly.com/question/29132693
#SPJ12
The complete question is as follows:
When L performed an operation on the matrix below, she forgot to include one element.
\(\left[\begin{array}{cccc}1&2&3&5\\5&3&-1&-11\\3&2&-2&-13\end{array}\right] \to \left[\begin{array}{cccc}1&2&3&5\\0&b&-16&-36\\3&2&-2&-13\end{array}\right]\)
What is the value of the unknown element, b?
(a) –7
(b) –5
(c) –3
(d) –2
What additional information could you use to show that δstu ≅ δvtu using sas? check all that apply. uv = 14 ft and m∠tuv = 45° tu = 26 ft m∠stu = 37° and m∠vtu = 37° st = 20 ft, uv = 14 ft, and m∠ust = 98° m∠ust = 98° and m ∠tuv = 45°
Answers
Options A and D are both correct. UV = 14 feet and mTUV = 45 degrees D. ST = 20 feet, UV = 14 feet, and mUST = 98 degrees by using congruence of triangles.
What is congruence triangle?Two triangles are congruent if their corresponding sides are the same length and their corresponding angles are the same size.
What exactly is SAS congruence?According to the SAS criterion for triangle congruence, two triangles are congruent if they have two pairs of congruent sides and the included angle (the one between the congruent sides) in one triangle is congruent to the included angle in the other triangle.
Given
See the attachment for the triangle.
What evidence do you have?
SAS is used by STU and VTU.
To demonstrate their resemblance, we must examine the corresponding sides and angles of both triangles.
To begin, UST must equal UVT.
As a result, UST=UVT=98⁰.
Then, UV must equal US.
So:
UV = US = 14⁰
Furthermore, ST must equal VT.
So:
Furthermore, ST must equal VT.
As a result, ST = VT = 200.
Finally, TUV must be equal to TUS.
So: TUV = TUS = 450
Options A and D are both correct. UV = 14 feet and mTUV = 45 degrees D. ST = 20 feet, UV = 14 feet, and mUST = 98 degrees.
To know more about the congruence of triangles visit :
https://brainly.com/question/20521780
#SPJ4
Plz help me and plz no links first person to answer it correctly will get brainliest

Answers
Answer:
B
Step-by-step explanation:
The standard form of a circle equation is:
(x - h)^2 + (y - k)^2 = r^2
where (h, k) is the center and r is the radius.
We can plug in the given values to get:
(x - 1)^2 + (y - 2)^2 = 3^2
This is the sam as option B
Find the value for that makes the statement true: sin = cos( + 40)
Answers
Answer:
\(\boxed{\theta=25}\)
Step-by-step explanation:
I guess the correct equation is something like this:
\(\sin( \theta)= \cos(\theta + 40)\)
I will use the following trigonometric identity:
\(\cos(x+y)=\cos(x) \cos(y)-\sin(x) \sin(y)\)
rewriting the equation
\(\sin(\theta)=\cos(\theta) \cos(40)-\sin(\theta) \sin(40)\\\sin(\theta)+\sin(\theta) \sin(40)=\cos(\theta) \cos(40)\)
common factor:
\(\sin(\theta)(1+\sin(40))=\cos(\theta) \cos(40) \\\\\frac{\sin(\theta)}{\cos(\theta)}= \frac{\cos(40)}{1+\sin(40))}\)
And using also the following identity:
\(\frac{\sin(\theta)}{\cos(\theta)} =tan(\theta)\)
rewriting the equation
\(\tan{\theta}= \frac{\cos(40)}{1+\sin(40))}\\\theta= \tan^{-1}(\frac{\cos(40)}{1+\sin(40))})\\\theta=25\)
By this, we have solved the exercise.
\(\text{-B$\mathfrak{randon}$VN}\)
24. [0/1 Points] DETAILS PREVIOUS ANSWERS WANEFMAC7 13.2.074. MY NOTES ASK YOUR TEACHER PRACTICE ANOTHER Find f(x) if f(2) = 0 and the tangent line at (x, f(x)) has slope x/(x^² + 3).
f(x) = 1/2en|x^2 + 3|-1/2en|7|
Answers
The function f(x) is: f(x) = \((1/2) ln|x^2 + 3| - (1/2) ln|7|\) given that f(2) = 0 and the tangent line at (x, f(x)) has a slope x/(x² + 3).
Let's find f(x) step-by-step:
1. We know that the slope of the tangent line is the derivative of the function, f'(x). So, we have f'(x) = x/(x² + 3).
2. To find f(x), we need to integrate f'(x) with respect to x.
3. Integrate x/(x² + 3) dx. Using substitution, let u = x² + 3, so du = 2x dx. Therefore, the integral becomes \((1/2)\int\ {(1/u)} \, du\).
4. Now, integrate \((1/2)\int\ {(1/u)} \, du\) = (1/2) ln|u| + C = (1/2) ln|x² + 3| + C.
5. We have f(x) = (1/2) ln|x² + 3| + C. To find the constant C, we'll use the given condition f(2) = 0.
6. Plug in x = 2: 0 = (1/2) ln|2² + 3| + C. Simplify and solve for C: C = - (1/2) ln|7|.
7. Now we have the function f(x) = \((1/2) ln|x^2 + 3| - (1/2) ln|7|\).
So, f(x) = \((1/2) ln|x^2 + 3| - (1/2) ln|7|\).
To know more about slope, refer here
https://brainly.com/question/2491620#
#SPJ11
use the digits 3,5 and 6 only to complete these calculations. you can use each digit more than once.??.?+??.?=100
Answers
Answer:
35 and 65
Step-by-step explanation:
35 + 65 = 100
Answer:
36.5 +63.5 = 100 Hope it helps......Distribute and combine like terms
8(-6+10)-14x
Answers
Distributing and combining like terms 8(-6+10)-14x we get 32 - 14x .
The equation is
8 ( - 6 + 10 ) - 14 x
Distributing the number in Distributive property multiplying the sum of two or more addends by a number produces the same result as when each addend is multiplied individually by the number and the products are added together.
Applying distributive property on the equation we get
8 × ( - 6 ) + 8 × ( 10 ) - 14x
On multiplying we get,
-48 + 80 - 14x
Combining the like terms
32 - 14x
To know more about distributing click here :
https://brainly.com/question/12192455
#SPJ4
What is 750 Milliliters in Cups? Convert 750 ml to cup
Answers
The answer is that 750 milliliters is equal to approximately 3.17 cups.
Milliliters (ml) and cups are units used to measure volume or capacity. Milliliters are part of the metric system, which is used worldwide, while cups are part of the imperial system, which is still used in some countries, including the United States.
To convert milliliters to cups, you can use the conversion factor of 1 cup being equal to approximately 236.588 milliliters. To convert 750 milliliters to cups, you would divide 750 by 236.588:
750 ml / 236.588 ml/cup = 3.17 cups
It's important to note that conversions between different units of measurement can sometimes result in values that are not an exact number, but instead a close approximation. This is because different units of measurement were developed in different parts of the world, and sometimes have different levels of precision.
To learn more about Milliliters in Cups, visit
https://brainly.com/question/10494767
#SPJ11