The width of a school playground is 2\times 10^(3) meters and the length is 6\times 10^(4) meters. If the playground is rectangular in shape, the area of the playground using scientific notation is____ square meters. a.12\times 10^(10) b.12\times 10^(9) c.1.2\times 10^(8) d./1.2\times 10^(10)
Answers
The width of a school playground is 2×103 meters and the length is 6×104 meters. Therefore, the answer is (a) 12 × 10¹⁰ square meters.
We know that the area of a rectangle can be calculated by multiplying the length and the width of the rectangle.
Here, the width is given to be 2 × 10³ meters, and the length is given to be 6 × 10⁴ meters.
Therefore, Area of the rectangle = Length × Width
Area of the rectangle = (2 × 10³) × (6 × 10⁴)
Area of the rectangle = (2 × 6) × (10³ × 10⁴)
Area of the rectangle = 12 × 10⁷
Now, the answer needs to be written in scientific notation.
Therefore, we need to write the number 12 as 1.2 × 10¹ (as 12 = 1.2 × 10¹)Therefore, Area of the rectangle = 1.2 × 10¹ × 10⁷
Area of the rectangle = 1.2 × 10¹⁰
Therefore, the answer is (a) 12 × 10¹⁰ square meters.
Learn more about area of a rectangle here:
https://brainly.com/question/8663941
#SPJ11
Related Questions
The hanger diagram models the equation 2b = 4. Use the diagram to find the
value of b. Show your reasoning.
Answers
The value of b is 2.
Since the hanger diagram models the equation 2b = 4, we can see that the bar representing 2b has a length of 4 units.
To find the value of b, we need to divide both sides of the equation by 2. This gives us:
2b/2 = 4/2
b = 2
Therefore, the value of b is 2.
Learn more about Equation here:
https://brainly.com/question/29657983
#SPJ1
Question 9 of 10
In the diagram below, AB and BC are tangent to O. What is the measure of
AC?
B
68
'0
10
248
A. 68°
OB. 90°
O C. 112°
OD. 136°
Answers
The measure of AC is 112° (option c).
1. We are given a diagram with a circle O and two tangents, AB and BC, intersecting at point B.
2. According to the properties of tangents, when a tangent line intersects a radius, it forms a right angle.
3. Therefore, angle AOB is a right angle because AB is tangent to circle O.
4. Similarly, angle BOC is also a right angle because BC is tangent to circle O.
5. Since the sum of angles in a triangle is 180°, we can find angle ABC by subtracting the measures of angles AOB and BOC from 180°.
- Angle ABC = 180° - (90° + 90°) = 180° - 180° = 0°
6. However, an angle of 0° is not possible in a triangle, so we need to consider the exterior angle at point B, angle ACD.
7. The measure of the exterior angle is equal to the sum of the measures of the two interior angles of the triangle that it is outside.
- Angle ACD = angle ABC + angle BAC = 0° + 68° = 68°
8. Finally, the measure of AC is the supplement of angle ACD, as it is the adjacent interior angle.
- Measure of AC = 180° - 68° = 112°.
Therefore, the measure of AC is 112°.
Thus, the correct option is c.
For more such questions on measure, click on:
https://brainly.com/question/25716982
#SPJ8
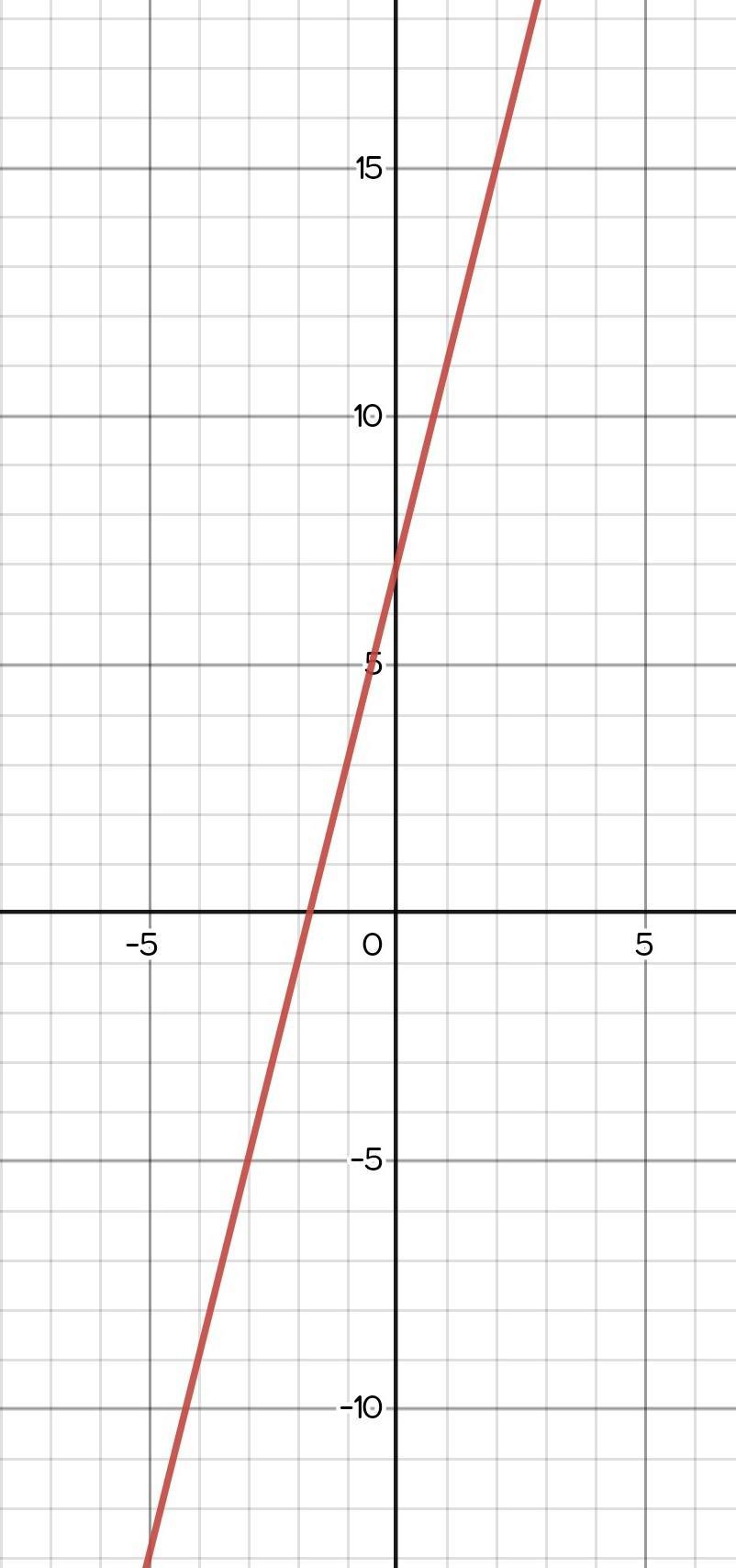
Graph the function f(x) = 7 + 4x. Then find the value of f(x) when x = 1/2
Answers
Answer:
See belowStep-by-step explanation:
Given function:
f(x) = 7 + 4xThe graph is attached
The value of the function when x = 1/2 is:
f(1/2) = 7 + 4*(1/2) = 7 + 2 = 9This is reflected on the graph as well

f(x)=8+4x
f(1/2)
7+4(1/2)7+29Graph attached
Which of the following ordered pairs is a solution to the inequality y>(1/3x)+3?
O (9,5)
O (5,4)
O (6,6)
O (3,4)
Answers
Janet just mulched her yard and had 2 1/4 bags of mulch left. She divided it evenly and gave 3/8 of a bag to each of the people on her block. How many people live on Janet's block?
Answers
Answer:
6 people
Step-by-step explanation:
2 1/4 = 9/4 = 18/8
She gave 3/8 of a bag to each of the people => she gave 18/8 : 3/8 = 6 people
So, there are 6 people that live on Janet's block (maybe 7 if count Janet herself)
Answer:
6
Step-by-step explanation:
2.25 divided by 3/8 (0.375)
To double check multiply 3/8 by 6 and you'll get 2.25 (2 1/4).
The relationship between income (I), in dollars, and the time (h), in hours, for 2 different jobs is represented. Compare the hourly rates of Job A and Job B.
Which job offers a higher rate per hour and by how much? Job A pays $5. 25 more per hour than Job B. Job A pays $4. 75 more per hour than Job B. Job B pays $5. 25 more per hour than Job A. Job B pays $4. 75 more per hour than Job A
Answers
The correct answer is: Job A pays $5.25 more per hour than Job B. To compare the hourly rates of Job A and Job B, need to determine which job pays more per hour and by how much based on given information.
Given Information: Job A pays $5.25 more per hour than Job B.
Let's assume the hourly rate of Job B is represented by x dollars per hour.
According to the given information, the hourly rate of Job A would be x + $5.25.
To determine which job offers a higher rate per hour, we need to compare the hourly rates of Job A and Job B.
Comparing the rates, we find that Job A pays $5.25 more per hour than Job B.
To learn more about rate click here:
brainly.com/question/25565101
#SPJ11
a rectangular storage container without a lid is to is to have a volume of 10 cubic meters. the length of its base is thrice the width. material for the base costs $20 per square meter. material for the sides cost $10 per square meter. find the cost of material for the least expensive box
Answers
The least expensive rectangular storage container without a lid, with a volume of 10 cubic meters, has a length three times its width. The total cost of the least expensive box is $750.
Let's assume the width of the rectangular container is x meters. According to the given information, the length of the base is three times the width, so the length is 3x meters. The height of the box can be determined by dividing the volume by the area of the base, giving us a height of 10/(3x^2) meters.
The cost of the base can be calculated by multiplying the area of the base (3x * x = 3x^2) by the cost per square meter ($20). Therefore, the cost of the base is 3x^2 * $20 = $60x^2.
The cost of the sides can be calculated by finding the area of the four sides (2 * 3x * 10/(3x^2) + 2 * x * 10/(3x^2)), which simplifies to 20/x. Multiplying this by the cost per square meter ($10) gives us a cost of $200/x.
To find the total cost, we sum the cost of the base and the cost of the sides: $60x^2 + $200/x. To minimize the cost, we can take the derivative with respect to x, set it equal to zero, and solve for x. The result is x = √(100/3). Substituting this value back into the cost equation, we find the minimum cost is approximately $750.
Learn more about derivative here:
https://brainly.com/question/29144258
#SPJ11
17) When filled to maximum capacity, a silo can hold about 4,000 cubic meters of corn. The radius of the silo is 8 meters. To the nearest meter, what is the approximate height (h) of the silo?

Answers
Answer: ion know i'm guessing 4
Step-by-step explanation: because it shows 8 at the top, so since it's full and it's 4,000 then that'll basically make 8 the 4000, so since 4 is half of 8 then we prolly have our answer right there
Answer: It would be 20
Step-by-step explanation: The formula for height would be h= v/pi r squared, just plug in the numbers
What are the values of each Halloween icon?
Answers
wdym “What are the values of each Halloween icon”?
Which is the most reasonable estimate of 132% of 48?
5.2
6.5
52
65
Answers
Answer:
65
Step-by-step explanation
Just did it on egde
A 4-pack of boncy balls costs $1.33. What is the unit price, rounded to the nearest cent?
Answers
Answer:
$0.33 per ball
Step-by-step explanation:
haha we meet again.
Divide 1.33 by 4
1.33/4=0.3325
Round to the nearest tenth
0.33
help please, with explanation

Answers
Answer:
3rd option
Step-by-step explanation:
A porportional relationship can be described by
\(y = kx\)
where x is the number of days, y is the amounts of food and k is the constant rate. (for this instance)
Looking at Classrom B, the amounts of food and cinstant rate isn't porpotional. Soxthat eliminates B and D.
For the 3rd option, the food amount is porportional as x increases. The unit rate here is 1,9 so the correct option is the 3rd option.
How do I find this?

Answers
Answer:
16pi cm^2, 112pi cm^3, 351.68 cm^3
Step-by-step explanation:
Given: Height=7cm, radius=4cm
1. The base is a circle and Area of circle = pi * r^2
Plug in the radius to get --> A = pi * (4cm)^2 = 16*pi cm^2
2. This is asking for the volume of the cylinder.
Volume of cylinder is V=h*pi*r^2 --> 7cm * pi * (4cm)^2 = 112 pi cm^3
3. Just plug 3.14 into pi --> 112 * pi cm^3 = 351.68 cm^3
PLEASE HELP I WILL GIVE BRAILIEST PLSSSSHELP
Simplify (6⁵ / 7³)²
a- 6⁷ / 7³
b- 6¹⁰ / 7⁶
c- 6³ / 7
d- 12⁵ / 14³
Answers
b. 6¹⁰ / 7⁶
Step-by-step explanation:(6⁵/7³)²
6 × 6 × 6 × 6 × 6 = 7776
7 × 7 × 7 = 343
(7776/343)²
7776²/343²
7776 × 7776 = 60.466.176
343 × 343 = 117.649
6¹⁰/7⁶
6 × 6 × 6 × 6 × 6 × 6 × 6 × 6 × 6 × 6 = 60.466.176
7 × 7 × 7 × 7 × 7 × 7 = 117.649
6¹⁰ = 7776²
7⁶ = 343²
= b. 6¹⁰ / 7⁶
I need help with Question 9 ?

Answers
Answer:
x/6 = 3/2
Step-by-step explanation:
Solve for v 4. 4 ( v - 16. 8 ) - -2. 3 = 3. 62
Answers
Simplifying the equation of v 4. 4 ( v - 16. 8 ) - -2. 3 = 3. 62, we find that v = 21.45.
To solve for v, we will use the following steps:
Simplify the left-hand side of the equation by distributing the 4.4:
4.4v - 73.92 + 2.3 = 3.62
Simplify further by combining like terms:
4.4v - 71.62 = 0
Add 71.62 to both sides of the equation:
4.4v = 71.62
Solve for v by dividing both sides by 4.4:
v = 71.62 / 4.4
v = 16.27727...
Round the answer to two decimal places:
v = 21.45
Therefore, the solution to the equation 4.4(v - 16.8) - (-2.3) = 3.62 is v = 21.45.
Learn more about Simplification:
https://brainly.com/question/28723467
#SPJ4
AWARDING EXTRA POINTS question in pick below

Answers
Answer:
x=35
Step-by-step explanation:
So the line XYZ is 180. The angles together should equal 180 in total.
The equation would be (2x-5) + (3x+10) = 180.
2x-5+3x+10=180
You want to combine like terms.
2x+3x and -5+10
5x+5=180
subtract 5 from both sides. Need variable alone.
5x+5(-5)=180(-5)
5-5 is 0 and 180-5 is 175
5x=175
Divide by 5 on both sides.
5x/5=175/5
x=35
Answer:
x=35
Step-by-step explanation:
Angles on a straight line=180
therefore,(2x-5)+(3x+10)=180
2x-5+3x+10=180
collecting like terms we have,
2x+3x-5+10=180
5x+5=180
subtract 5 from both sides
5x+5-5=180-5
5x=175
divide both sides by coefficient of x
5x/5=175/5
x=35
Two cars start at a given point and travel in the same direction at average speeds of 45 miles per hour and 52 miles per hour (see figure). How far apart will they be in 4 hours?
Answers
Answer:
half apart
Step-by-step explanation:
hope it help you
Which of the following are the most likely factors of the function graphed above?
A. (x-3)(x+4)
B. (x+3)(x-4)
C. (x+2)(x-4)
D. (x)(x+12)

Answers
Answer:
Option (B)
Step-by-step explanation:
From the graph attached,
x-intercepts of the graphed function are,
x = -3 and x = 4
Therefore, zero factors of the function will be,
(x + 3) and (x - 4)
So the quadratic function given in the graph will be in the form of,
f(x) = (x + 3)(x - 4)
Option (B) will be the correct option.
A vertical number line is given.
A vertical number line starting at negative 5 with tick marks every one fourth unit up to positive 5. The point negative 1.25 is labeled.
What is the absolute value of the number plotted on the number line?
−1.5
−1.25
1.25
1.5
Answers
The absolute value of the number plotted on the number line is 1.25
pls any body.......

Answers
оооо
Yes, because it has a constant rate of change.
O Yes, because it does not have a constant rate of change.
No, because it has a constant rate of change.
O No, because it does not have a constant rate of change.

Answers
Answer: A) No, because it has a constant rate of change
=============================================================
Explanation:
Each time x goes up by 1, y drops by 2.
This means,
slope = rise/run
slope = (change in y)/(change in x)
slope = (y drops by 2)/(x goes up by 1)
slope = -2/1
slope = -2
-----------
We can pick two points from the table to plug into the slope formula. I'll pick the first two rows
m = slope
m = (y2-y1)/(x2-x1)
m = (2-4)/(7-6)
m = -2/1
m = -2
If you tried every pair of rows possible for this table, you'll get the same slope each time. This is why the rate of change is constant.
This means the data is linear instead of nonlinear. Plotting the points leads to a single straight line going through all four points. See the diagram below. The graph was made with GeoGebra.
The equation of that red line is y = -2x+16 which is equivalent to 2x+y = 16.
In the graph, going from A to B has us go down 2 and to the right 1, which is one visual way of seeing the slope is -2/1 = -2.

a tree t with 50 end-vertices has an equal number of vertices of degree 2, 3, 4 and 5 and no vertices of degree greater than 5. what is the order of t?
Answers
The order of T is 82.
Let n and e be the order and number of edges of T respectively.
Also, let a, b, c and d be the number of vertices of degree 2, 3, 4 and 5 respectively.
Then, 50+a+b+c+d = n
And by the Handshake Lemma, the sum of the degrees of all the vertices of T is twice the number of edges, so we have
50+2a+3b+4c+5d = 2e
Since a=b=c=d then we have the following two equations,
50+4a = n
50+14a = 2e
But, T is a tree so e=n=1, then the two equations become,
50+4a = n ...............(1)
50+14a = 2(n-1) .............(2)
Solving for a in (1) we get,
a = \(\frac{n-50}{4}\)
Plugging a into (2) we get,
50+14(\(\frac{n-50}{4}\)) = 2(n-1)
50+3.5n-175 = 2n-2
3.5n-2n = 175-50-2
1.5n = 123
n = \(\frac{123}{1.5}\) = 82
Therefore, the order of T is 82.
Learn more about Vertices at,
https://brainly.com/question/1217219
#SPJ4
Which values represent the independent variable? (–2, 4), (3, –2), (1, 0), (5, 5) A. {–2, 3, 1, 5} B. {4, –2, 0, 5} C. {–2, 4, 3, –2} D. {–2, –1, 0, 5} Please select the best answer from the choices provided A B C D
Answers
Answer:
The independent variable is the variable that is manipulated or changed during an experiment. In this case, the independent variable is represented by the x-values of the given points.
So, the answer would be option A: {-2, 3, 1, 5}
Step-by-step explanation:
brainliest Plsssss
given the fuction g(x)=-4x-7 find g(3)
Answers
Diego, this is the solution:
We have the following function:
g (x) = 4x - 7
In consequence,
g (3) = 4 * 3 - 7
g (3) = 12 - 7
g (3) = 5
Which of the following is not included in the cost of merchandise inventory? O Purchase discounts. O Purchase returns and allowances. O Purchase price of the inventory. O Freight costs paid by the seller. O Freight costs paid by the buyer. 4 pts 0 Question 2 Sunshine Cleaning purchased $3,500 worth of merchandise. The seller offered a 2% cash discount. Transportation costs for the buyer were an additional $310. The company returned $240 worth of merchandise and then paid the invoice within the discount period. The total cost of this merchandise is: O $3.570.00. O $3,500.00 O $3,332.00 O $3,430.00. $3,504.80 Question 5 A company has not sales of $759.300 and cost of goods sold of $548.300. Its not income is $10.280. The company's gross margin and operating expenses, respectively, are: O $211.000 and $230,750 $739.550 and $191,720 O $529,020 and $230.750 O $211.000 and $191,720 $230,750 and $529,020 4 pts D D Question 5 A company has not sales of $750,300 and cost of goods sold of $548,300. Its not income is $10.280 The company's groas margin and operating expenses, respectively, arm O $211,000 and $230,750 O $739,550 and $191,720 $529,020 and $230,750 O $211.000 and $191,720 O $230.750 and $529,020 Question 6 Sales less sales discounts, less sales returns and allowances equals: Cost of Goods Sold Net Income O Net Sales O Gross Profit 4 pts 4 pts Goods in transit are included in a purchaser's inventory: O At any time during transit. O After the half-way point between the buyer and seller, When the supplier is responsible for freight charges. When the goods are shipped FOB shipping point. OIf the goods are shipped FOB destination. Question 11 The inventory costing method that smooths out erratic changes in costs is: O LCM. O FIFO. OLIFO. O Specific Identification. O Weighted average. 4 t ne 0 Question 12 Krusty Krab has the following products in its ending inventory Compute lower of cost or market for inventory. applied separately to each product Inventory by Product Product Quantity Cost per Unit 500 $ 500 $ 30 600 Scuba Masks Scuba Sults O $265,000 O $290,000. O $250,000 $268,000 O $275,000. Question 13 Market per Unit $ 550 $ 25 If equity is $368,000 and liabilities are $186,000, then assets equal: O $554,000. $922,000. $368,000. $186,000. O $182,000. 2 pts
Answers
Question 1: The item not included in the cost of merchandise inventory is "Purchase discounts."
2: The total cost of the merchandise is $3,332.00.
5: The company's gross margin and operating expenses, are $211,000 and $191,720.
What is the cost of merchandise inventory?Merchandise inventory expenses normally consist of the price paid for the inventory, deductions from the purchase price resulting from purchase returns and allowances, and freight expenses paid by the purchaser.
Although purchase discounts reduce the cost of merchandise inventory, they are not considered part of it. Instead, they are treated as a distinct discount in the accounting records.
Learn more about merchandise inventory from
https://brainly.com/question/27046371
#SPJ1
g(x,y)={x2+y22xy,0,(x,y)∈R\(0,0)(x,y)=(0,0)., for R=[0,1]×[0,1] q) Present the numerical value of the double integral ∬RG(x,y)dydx. i) Calculate the double integral presented in Part 1q ). This volume must be calculated using rectangular coordinates for the double integration. No marks will awarded for any other method of calculating the volume.
Answers
To calculate the double integral ∬Rg(x,y)dydx, we first need to evaluate the integral in the given region R=[0,1]×[0,1]. Using rectangular coordinates, we can express the double integral as \(∬Rg(x,y)dydx = ∫[0,1] ∫[0,1] g(x,y) dy dx\).
Now, let's plug in the expression for g(x,y) into the integral:
\(∫[0,1] ∫[0,1] (x^2 + y^2)/(2xy) dy dx.\) To evaluate this integral, we can integrate with respect to y first, then with respect to x.
\(∫[0,1] [(1/2)x^2y + (1/6)y^3] [y=0 to 1] dx.\) Simplifying this expression, we have: ∫[0,1] [(1/2)x^2 + (1/6)] dx.Now we integrate with respect to x:
\([(1/6)x^3 + (1/2)x] [x=0 to 1].\)
Evaluating this expression, we get:
\([(1/6)(1)^3 + (1/2)(1)] - [(1/6)(0)^3 + (1/2)(0)].\)
Simplifying further, we obtain:
(1/6) + (1/2) - 0.
Combining the terms, we find that the numerical value of the double integral ∬Rg(x,y)dydx is 4/6 or 2/3.
To know more about calculate visit:
https://brainly.com/question/32553819
#SPJ11
The numerical value of the double integral ∬RG(x,y)dydx is 1/4. The double integral of g(x,y) over the region R=[0,1]×[0,1] does not exist.
To calculate the double integral, we need to integrate the given function g(x,y) over the region R=[0,1]×[0,1]. Let's break down the integral into two parts: the inner integral and the outer integral.The inner integral represents the integration with respect to y, and the outer integral represents the integration with respect to x.For the inner integral, we integrate g(x,y) with respect to y while keeping x constant. Since g(x,y) is a function of x and y, we need to evaluate the integral using the limits of y. The limits of y are determined by the region R=[0,1]×[0,1]. In this case, the limits for y are from 0 to 1.
Now, let's evaluate the inner integral:
∫[0,1] g(x,y)dy = ∫[0,1] (x^2+y^2)/(2xy) dy.
To integrate this, we can split the fraction into two separate fractions:
∫[0,1] x^2/(2xy) dy + ∫[0,1] y^2/(2xy) dy.
Simplifying these integrals, we get:
(1/2)∫[0,1] (x/y) dy + (1/2)∫[0,1] (y/x) dy.
Now, let's integrate each term separately:
(1/2)∫[0,1] (x/y) dy = (1/2) [xln|y|] from 0 to 1 = (1/2)(xln|1| - xln|0|) = 0.
(1/2)∫[0,1] (y/x) dy = (1/2) [yln|x|] from 0 to 1 = (1/2)(ln|x| - ln|0|) = ∞ (diverges).
As we can see, the second term diverges. This means that the inner integral does not exist. Since the inner integral does not exist, the double integral does not exist either.
To know more about integration, visit:
https://brainly.com/question/31744185
#SPJ11
The population of weights of a particular fruit is normally distributed, with a mean of 535 grams and a standard deviation of 13 grams. If 8 fruits are picked at random, then 18% of the time, their mean weight will be greater than how many grams? Round your answer to the nearest gram.
Answers
Pn(Z>zp)=1−p%
Given a variable X following a normal distribution with mean μ and standard deviation σ, the area below the curve that corresponds to all values that are less than a that is, X<a
is the probability P(X<a). This probability is usually calculated by converting the variable X to a standard normal variable Z (forming z scores) defined by:
\(z=\frac{x-\mu}{\sigma}\)
The variable Z follows the standard normal distribution with a mean of 0 and a standard deviation of 1.
The standard normal probabilities are typically found by using the standard normal tables or other computational means such as calculators or software.
Pn(Z>zp)=1−p%
Here Pn is the probability for the standard normal variable.
Learn more about mean here https://brainly.com/question/18060320
#SPJ4
What is the value of the algebraic expression if x=1/2, y = -1 and z=2?Algebraic Expression: 6x (y to the second power z)A. -12B. -6C. 1D. 6
Answers
The given expression is
\(6xy^2z\)Replacing the given values, we have
\(6\cdot\frac{1}{2}\cdot(-1)^2\cdot(2)=3\cdot1\cdot2=6\)Hence, the answer is D. 6.How many significant digits are there in exact numbers?
A) Exact numbers have only eight significant figures.
B) Exact numbers have only three significant figures.
C) All the figures written in the exact number are significant.
D) Exact numbers have an unlimited number of significant figures.
Answers
Exact numbers have an unlimited number of significant figures and is therefore denoted as option D.
What are Exact numbers?These are the numbers which are used in counting and defining other quantities which are assumed to be exact and usually have no remainders and they have an infinite number of significant figures.
An example is number “3” is exact as well and could be expressed as 3.0000000000000. This means that the number of significant figures can be unlimited because any amount of figures can be placed after the number which is therefore the reason why it was chosen as the correct choice.
Read more about Exact numbers here https://brainly.com/question/28294859
#SPJ1