The sides of a triangle are in the ratio of 3:4:5. A man jogged around it 5 times and cover a distance of 3000m What are the sides of the triangle?
Answers
The sides of the triangle are 150m, 200m and 250m.
What is distance?
Distance is a measurement of how far apart two things or points are, either numerically or occasionally qualitatively. Distance can refer to a physical length in physics or to an estimate based on other factors in common usage.
Given: The sides of a triangle are in the ratio of 3:4:5. A man jogged around it 5 times and cover a distance of 3000m.
As man jogged around triangle 5 times and cover a distance of 3000m.
So, the distance for one round is 3000/5 = 600m.
The covered distance is nothing but the perimeter of the triangle.
So, the perimeter of the triangle is 600m.
Let, 3x, 4x, 5x are the sides of the triangle.
⇒ 3x + 4x + 5x = 600
12 x = 600
x = 50
Therefore,
3x = 3(50) = 150
4x = 4(50) = 200
5x = 5(50) = 250
Hence, the sides of the triangle are, 150m, 200m and 250m.
To know more about the distance, click on the link
https://brainly.com/question/17146782
#SPJ9
Related Questions
Yui makes a list of the balances in her savings account at the end of each month. She notices that each month’s total is 5% greater than the previous month’s total. She writes a recursive formula to describe the account balances.
Which value should she use as the common ratio?
0.05
0.5
1.05
5.0
Answers
The answer is:
Answer:
C. 1.05
if u mark as brainly thatll be cool
The common ratio she used is 1.05
What is ratio?A ratio is a comparison between two amounts that is calculated by dividing one amount by the other. The quotient a/b is referred to as the ratio between a and b if a and b are two values of the same kind and with the same units, such that b is not equal to 0. Ratios are represented by the colon symbol (:). As a result, the ratio a/b has no units and is represented by the notation a: b.
Given:
Yui notices that each month’s total is 5% greater than the previous month’s total.
Now, let the previous month saving be x.
Saving after End of second Month
= Previous Month Saving + (5% of Previous Month Saving)
= x + (5 % of x )
= x + (5*x/100)
= x + 0.05x
= 1.05x.
Hence, Yui Salary is 1.05 times of Previous Month salary.
Learn more about ratio here:
https://brainly.com/question/13419413
#SPJ1
The expression ( x 22 ) ( x 7 ) 3 is equivalent to x p. What is the value of p?
Answers
The given expression is equivalent to x^43, and the value of p is 43.
When we have an expression with exponents, such as (x^22)(x^7)^3, we can simplify it by applying the rules of exponents. In this case, we can use the rule that states that when we have a power raised to another power, we can multiply the exponents. That is, (a^m)^n = a^(m*n).
Using this rule, we can simplify the given expression as follows:
(x^22)(x^7)^3 = x^(22 + 7*3)
= x^(22 + 21)
= x^43
Therefore, the given expression is equivalent to x^43, and the value of p is 43.
It's important to understand the rules of exponents when dealing with expressions that involve powers. These rules allow us to simplify complex expressions and make calculations more manageable. In general, we should always try to simplify expressions as much as possible, as this can help us to identify patterns and relationships that might not be apparent in more complex forms.
learn more about expression here
https://brainly.com/question/28170201
#SPJ11
a professional basketball player makes 80% of the free throws he tries. assuming this percentage holds true for future attempts, use the binomial formula to find the probability that in the next eight tries, the number of free throws he will make is: a. exactly 8 b. exactly 5 c. 3 or 4
Answers
a) The probability that in the next eight tries, the number of free throws he will make exactly 8 is equals to the 0.27249.
b) The probability that in the next eight tries, the number of free throws he will make exactly 5 is equals to the 0.08386.
We have a professional basketball player makes 80% of the free throws he tries.
let X be the number of free throws he will make in the next 8 tries. He makes 80% of the free thwors he tries. So, for binomial distribution, X~Bin(8,0.80)
so the probability mass function of X is P[X =x] = ⁸Cₓ 0.85ˣ (1-0.85)⁽⁸⁻ˣ⁾, x = 0,1, 2,..8
P[ X = x] = 0 otherwise.
a) So, the probability that he makes exactly 8 throws isP,(X=8)
= ⁸C₈(0.85)⁸ (1- 0.85)⁰
=0.27249
b) the probability that he makes exactly 5 throws is, P[X=5]
=⁸C₅0.855(1-0.85)⁽⁸⁻³⁾=56×0.855×0.153
=0.08386
Hence required probability is 0.083.
For more information about probability, refer:
https://brainly.com/question/25870256
#SPJ4
Is there a trick for 8 times tables?
Answers
One common trick for memorizing the 8 times table is to pair it with the 4 times table.
For example, 8 x 4 = 32, so 8 x 5 = 40 because 5 is one more than 4. Similarly, 8 x 6 = 48 because 6 is two more than 4, and so on. Another trick is to think of it as 8 x 10 = 80 and then subtract 8. For example, 8 x 7 = 80 - 8 = 72.
The eight times table trick states that when you multiply eight by a number between one and five, the result always begins with a digit one lower than the number from one to five. The answer begins with a digit that is two less than the number from 6 to 10 when multiplying 8 by a number between 6 and 10.
Learn more about 8 times tables here:https://brainly.com/question/13060199
#SPJ4
ABCD i a trapezoid. = 1 cm and = 5 cm, and the area = 7. 5 cm2. What i the altitude of the trapezoid?
Answers
The required altitude of the trapezoid ABCD is 2.5 cm.
What is trapezoid?The Greek roots of the term trapezoid are trapeza, which means "table," and -oeides, which means "formed." A trapezoid is shaped like a table. It has two parallel sides, which are often referred to as the figure's bases. Take the average of the bases and multiply it by the height to determine the area of a trapezoid.
According to question:Here, we must utilise the area of a trapezoid formula, which is
where h is the height or altitude and b1 and b2 are the two bases.
\(Area =\frac{ (b_{1}+b_{2})h}{2}\)
Given are the values of Area = 7.5, b1 = 1, b2 = 5, and h is unknown.
Then,
7.5 = (1+5)h/2
7.5 = 3h
h = 2.5cm
To know more about trapezoid visit:
brainly.com/question/8643562
#SPJ4
3. The decimal expansion of 13/625 will terminate
after how many places of decimal?
(a) 1
(b) 2
(c) 3
(d) 4
Answers
The decimal expansion of the given fraction is 0.0208. Therefore, the correct answer is option D.
The given fraction is 13/625.
Decimals are one of the types of numbers, which has a whole number and the fractional part separated by a decimal point.
Here, the decimal expansion is 13/625 = 0.0208
So, the number of places of decimal are 4.
Therefore, the correct answer is option D.
To learn more about the decimal numbers visit:
https://brainly.com/question/1578006.
#SPJ1
Graph y=−2x+5 and y=−12x−3 . Are the lines parallel, perpendicular, or neither?
Answers
the slopes are not the same so they aren’t parallel
the slopes are not opposite reciprocals so they aren’t perpendicular
the phase change line demonstrates a change in conditions on a graph and is represented by
Answers
The phase change line, also known as the phase boundary or phase transition line, is a line on a graph that represents a change in conditions for a substance. This line shows the temperature and pressure conditions at which a substance will change from one phase to another, such as from a solid to a liquid or from a liquid to a gas.
The phase change line is represented on a graph by a diagonal line that separates two regions where the substance is in different phases. This line usually slopes upwards from left to right, indicating that higher temperatures and pressures are needed to change the substance from one phase to another.
The phase change line is an important concept in thermodynamics and is used to understand the behavior of substances under different conditions. For example, the phase change line for water shows that at normal atmospheric pressure, water will freeze at 0°C and boil at 100°C. However, at higher pressures, the freezing and boiling points will change, and water may exist in different phases, such as a supercritical fluid.
To know more about transition line visit:-
https://brainly.com/question/13461671
#SPJ11
2. Find all the absolute maximums and minimum values on each interval. (12 marks) b. f(x)=x-2x², -2≤x≤5 a. f(x)=x²-3x², -1≤x≤4 c. f(x)=(x + 2)², -3≤x≤3 d. f(x)=2x³ + 3x2 -36x +17, -3�
Answers
a. For the function f(x) = x² - 3x² on the interval -1 ≤ x ≤ 4:
Absolute maximum value: 0 at x = 0
Absolute minimum value: -32 at x = 4
b. For the function f(x) = x - 2x² on the interval -2 ≤ x ≤ 5:
Absolute maximum value: 1/4 at x = 1/4
Absolute minimum value: -45 at x = 5
c. For the function f(x) = (x + 2)² on the interval -3 ≤ x ≤ 3:
Absolute maximum value: 25 at x = 3
Absolute minimum value: 1 at x = -3
d. For the function f(x) = 2x³ + 3x² - 36x + 17 on the interval -3≤x≤6:
Absolute maximum value: 92 at x = -3
Absolute minimum value: -11 at x = 2
To find the absolute maximum and minimum values of the given functions on each interval, we will analyze the critical points and endpoints of the intervals.
a. For the function f(x) = x² - 3x² on the interval -1 ≤ x ≤ 4:
First, let's find the critical points by taking the derivative of f(x):
f'(x) = 2x - 6x = -4x.
Setting f'(x) equal to zero, we get:
-4x = 0,
x = 0.
Next, we evaluate the function at the critical point and endpoints:
f(-1) = (-1)² - 3(-1)² = -2,
f(0) = (0)² - 3(0)² = 0,
f(4) = (4)² - 3(4)² = -32.
Therefore, the absolute maximum value is 0 at x = 0, and the absolute minimum value is -32 at x = 4.
b. For the function f(x) = x - 2x² on the interval -2 ≤ x ≤ 5:
Taking the derivative of f(x):
f'(x) = 1 - 4x.
Setting f'(x) equal to zero, we have:
1 - 4x = 0,
4x = 1,
x = 1/4.
Next, we evaluate the function at the critical point and endpoints:
f(-2) = (-2) - 2(-2)² = -6,
f(1/4) = (1/4) - 2(1/4)² = 1/4,
f(5) = (5) - 2(5)² = -45.
Therefore, the absolute maximum value is 1/4 at x = 1/4, and the absolute minimum value is -45 at x = 5.
c. For the function f(x) = (x + 2)² on the interval -3 ≤ x ≤ 3:
Since this is a quadratic function, it is always increasing. Thus, the absolute maximum value will occur at x = 3, and the absolute minimum value will occur at x = -3.
Evaluating the function at the critical points and endpoints:
f(-3) = (-3 + 2)² = 1,
f(3) = (3 + 2)² = 25.
Therefore, the absolute maximum value is 25 at x = 3, and the absolute minimum value is 1 at x = -3.
d. For the function f(x) = 2x³ + 3x² - 36x + 17 on the interval -3 ≤ x ≤ 6:
Taking the derivative of f(x):
f'(x) = 6x² + 6x - 36 = 6(x² + x - 6).
Setting f'(x) equal to zero, we get:
x² + x - 6 = 0.
Factoring the quadratic equation, we have:
(x + 3)(x - 2) = 0.
This gives us two critical points: x = -3 and x = 2.
Next, we evaluate the function at the critical points and endpoints:
f(-3) = 2(-3)³ + 3(-3)² - 36(-3) + 17 = 92,
f(2) = 2(2)³ + 3(2)² - 36(2) + 17 = -11,
f(-3) = 2(-3)³ + 3(-3)² - 36(-3) + 17 = 92.
Therefore, the absolute maximum value is 92 at x = -3, and the absolute minimum value is -11 at x = 2.
To know more about critical points, refer here:
https://brainly.com/question/31402315
#SPJ11
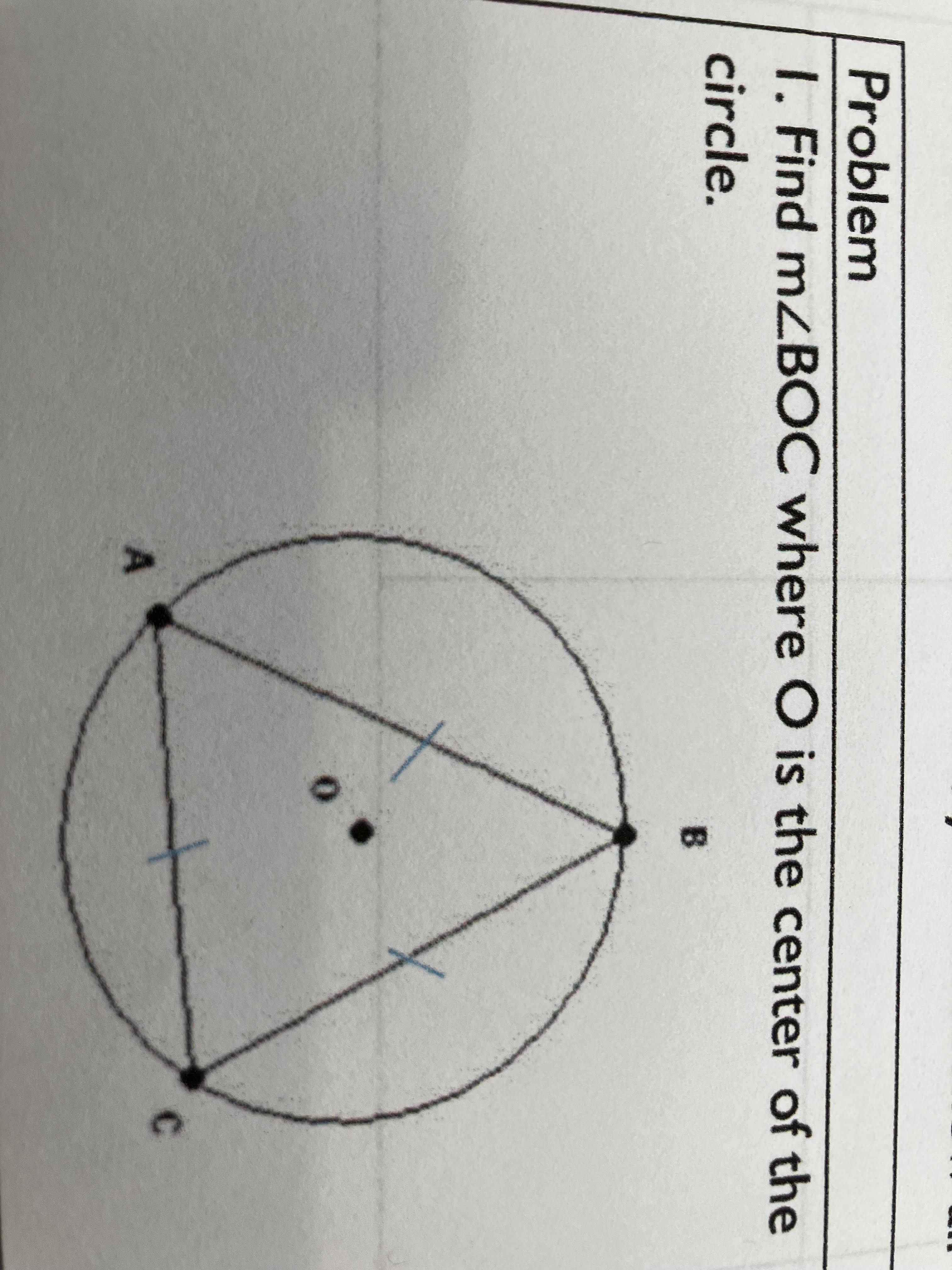
Can't Seem to be able to solve this help
Answers
An angle measurement, m∠BOC = 60° degrees where O is the center of the corcle.
What is an angle?
Since the ΔABC has three similar sides, we know that it is an equilateral triangle, meaning that all three angles are equal and each angle measures 60°.
Since A, B, and C are points on a circle with O as the center, we know that each of the distances OA, OB, and OC is equal to the radius of the circle.
Let's call this radius r.
Since ΔABC is equilateral, we know that each side has length r. We can also draw radii from the center O to points A, B, and C to form three congruent triangles AOB, BOC, and COA, each with two sides of length r and one angle of 60°.
Since the sum of the angles in a triangle is 180° degrees, each of the other two angles in each of these congruent triangles must measure 60° as well.
Thus, we see that ∠BOC is one of the angles in the isosceles ΔBOC, which has two angles of 60° and a third angle x, which we want to find.
We can use the fact that the sum of the angles in a triangle is 180° to find x:
x + 60 + 60 = 180
Simplifying, we get:
x = 60
Therefore, angle BOC measures 60°
To know more about angle, visit:
https://brainly.com/question/28451077
#SPJ1
Complete question is: m∠BOC = 60° degrees where O is the center of the corcle.
Use the iterative formula xn+1 = 4 - √x with x0 = 2 to find the solution to f(x) correct to 2 decimal places.

Answers

if you received a random sample size of 345 drawn from a population with a mean of 150 and a standard deviation of 180. what is the standard deviation of the sample mean? finally, what does the standard deviation mean in this question? explain. what are your assumptions with the confidence interval at 95%? explain. when observing hours discrepancy in the workplace, we analyze 32 workers. we noticed the sample mean was found to be 42.1 hours a week, with a standard deviation of 10.4. test the claim that the standard deviation was at least 13 hours.
Answers
the standard deviation is not less than 13 hours based on the given sample data.
To calculate the standard deviation of the sample mean, we can use the formula:
Standard deviation of the sample mean = Standard deviation of the population / √(sample size)
Given that the population standard deviation is 180 and the sample size is 345, we can plug in these values:
Standard deviation of the sample mean = 180 / √345 ≈ 9.693
The standard deviation of the sample mean represents the average variability or spread of sample means that could be obtained from repeated sampling. In this case, it indicates how much the sample means would typically vary from the true population mean. A smaller standard deviation of the sample mean suggests that the sample means are closer to the population mean, leading to greater precision and accuracy in estimating the population mean.
Regarding the assumptions for the confidence interval at 95%, they typically include:
1. Random Sample: The sample should be selected randomly from the population to ensure its representativeness.
2. Independence: The observations within the sample should be independent of each other. Each data point should not be influenced by others.
3. Normality or Large Sample Size: The population distribution should be approximately normal, or the sample size should be large enough to satisfy the Central Limit Theorem. The CLT states that for a large sample size, the distribution of the sample mean becomes approximately normal, regardless of the population distribution.
4. Unbiasedness: The sample should be unbiased and free from selection or measurement bias.
As for testing the claim that the standard deviation is at least 13 hours, we can use a one-sample t-test with the null and alternative hypotheses:
Null hypothesis (H0): The standard deviation is less than 13 hours.
Alternative hypothesis (Ha): The standard deviation is at least 13 hours.
Using the sample data, we can calculate the test statistic:
t = (sample standard deviation - claimed standard deviation) / (standard deviation of the sample mean / √sample size)
t = (10.4 - 13) / (10.4 / √32) ≈ -2.035
Next, we determine the critical value at a significance level (α) of 0.05 for a one-tailed test with degrees of freedom (df) = sample size - 1 = 32 - 1 = 31. Checking a t-distribution table or using a statistical calculator, we find the critical value to be approximately -1.697.
Since the calculated t-value (-2.035) is less than the critical value (-1.697), we have evidence to reject the null hypothesis. Therefore, we can conclude that the standard deviation is not less than 13 hours based on the given sample data.
to know more about interval visit:
brainly.com/question/29152839
#SPJ11
1. Which one does not belong *
O y=(x+4)(x-6)
O y=2x²-88-24
O y=x2+5x-25
O y=x®+3x?-10x-24
Answers
Answer:
I believe the answer is 2x² - 88 - 24, since it is the only one with a number in front of the x²
Step-by-step explanation:
y = x² - 2x - 24
y = 2x² - 88 - 24
y = x² + 5x - 25
y = x² + 3x - 10x - 24
Find the nth term of the arithmetic sequence (an) whose initial term a and common difference d are given below. What is the forty-fifth term?
a₁ = 2; d = 1/4
Answers
Answer:
a₄₅ = 13
Step-by-step explanation:
The n-th term of an arithmetic sequence with first term a₁ and common difference d is given by the formula ...
aₙ = a₁ +d(n -1)
SetupYou want the 45th term where a₁ = 2 and d = 1/4. Putting these values into the formula gives ...
a₄₅ = a₁ +d(n -1) = 2 +(1/4)(45 -1)
SolutionEvaluating this expression, we have ...
a₄₅ = 2 +44/4 = 2 +11
a₄₅ = 13
The 45th term of the sequence is 13.
The forty-fifth term of the sequence whose initial term a = \(2\) and common difference d = \(\frac{1}{4}\) is \(13\)
How to find the nth term of the Arithmetic series?
The nth term of the arithmetic series is find by \(Tn = a+(n-1)d\) where Tn is the nth term of the series a is called the initial number and is the common difference between two number. n is the number of term of that arithmetic series.
In the given series initial term a = \(2\) and common difference d = \(\frac{1}{4}\)
We have the find the forty-fifth term of the given series.
= \(Tn=a+(n-1)d\)
= \(Tn = 2+(45-1)\frac{1}{4}\)
= \(Tn=2+44\cdot\frac{1}{4}\)
= \(Tn = 2+11\)
= \(Tn = 13\)
So, the forty-fifth term of the sequence whose initial term a = \(2\) and common difference d = \(\frac{1}{4}\) is \(13\)
To learn more about the arithmetic series. You can visit.
https://brainly.com/question/24295771
#SPJ2
darryl can paint a room in 9 hours. valerie can paint the same room in 15 hours. how long does it take for both darryl and valerie to paint the room it they are working together?
Answers
It will take 5.625 hours for both Darryl and Valerie to paint the room if they work together .
In the question ,
it is given that ,
Darryl can paint the room in 9 hours .
So , in 1 hour Darryl can paint 1/9 of the room .
Valerie can paint the room in 15 hours .
So , in 1 hour Valerie can paint 1/15 of the room .
So , the time taken to paint the room together can be calculated using ,
1/t = 1/9 + 1/15
1/t = 5/45 + 3/45
1/t = 8/45
Simplifying further ,
we get ,
t = 45/8
t = 5.625
Therefore , the time taken will be 5.625 hours .
Learn more about Algebra here
https://brainly.com/question/26957806
#SPJ4
a deck of cards is dealt out. what is the probability that the 14th card dealt is an ace? what is the probability that the first ace occurs on the 14th card?
Answers
The probability of picking an ace on any draw is 4/52 or 1/13.
The probability that the 14th card dealt is an ace is 0.002641
We can first calculate the probability of not getting an ace on the first 13 draws, and then find the probability of getting an ace on the 14th draw:
P(No ace on 1st draw) = 48/52
P(No ace on 2nd draw) = 47/51
P(No ace on the 3rd draw) = 46/50
And so on...
P(No ace on the 13th draw) = 36/40.
P(Ace on 14th draw) = 4/39
To find the probability of these independent events, we multiply their probabilities:
P(Ace on 14th draw) = P(No ace in first 13 draws) x P(Ace on 14th draw)
P(Ace on 14th draw) = (48/52) x (47/51) x ... x (4/40) x (4/39)
P(Ace on 14th draw) ≈ 0.002641
To know more about the "probability": https://brainly.com/question/251701
#SPJ11
NEEDDD HELPPPP ASAPPPPPPPPPP !!

Answers
Answer:
42 = 8x + 13x
42 = 21x
x = 2
8 = 8c -4(c + 8)
8 = 8c - 4c - 32
8 = 4c - 32
40 = 4c
c = 10
Answer:
42 = 8x + 13x42 = 21x
42/21 = x
x = 2
check:
42 = 8*2 + 13*2
42 = 16 + 26
8 = 8c - 4(c+8)8 = 8c -4*c -4*8
8 = 8c - 4c - 32
8 + 32 = 4c
40 = 4c
40/4 = c
c = 10
Check:
8 = 8*10 - 4(10+8)
8 = 80 - 4*18
8 = 80 - 72
What is sum of the geometric series?

Answers
Answer:
Sn=a1(1−rn)1−r,r≠1 C.
what percentage of cases in a normal distribution are between 0.5 standard deviations above and below the mean.
Answers
Approximately 68% of the cases in a normal distribution are between 0.5 standard deviations above and below the mean.
To find the percentage of cases that are between 0.5 standard deviations above and below the mean, we can add these two probabilities:
34% + 34% = 68%
The normal distribution, also known as the Gaussian distribution or the bell curve, is a probability distribution that is widely used in statistics, science, and engineering. A bell-shaped curve that is symmetrical around the mean value best describes the distribution. The distribution's mean, median, and mode are all equal, and the standard deviation defines the curve's width.
Height, weight, IQ, and measurement mistakes are only a few examples of the numerous random variables and natural phenomena that follow the normal distribution. In addition, the central limit theorem predicts that regardless of the underlying distribution of the variables themselves, the total or average of several independent random variables with similar distributions will converge to a normal distribution. Because of this characteristic, the normal distribution is a key idea in inferential statistics and hypothesis testing.
To learn more about Normal distribution visit here:
brainly.com/question/29509087
#SPJ4
Complete Question:-
What percentage of cases in a normal distribution are between 0.5 standard deviations above and below the mean? Give one percentage.
Volume of a rectangular prism calculator is ?
Answers
The volume of a rectangular prism is the product of length, width, and height. The length, width, and height are equal for a cube prism.
What is a rectangular prism?
Having six faces, a rectangular prism is a three-dimensional shape (two at the top and bottom, and four are lateral faces). The prism's faces are all rectangular in shape. There are three sets of identical faces as a result. A rectangular prism is also referred to as a cuboid because of its shape.
The number of face of rectangular prism is 6.
The product of all dimensions of a prism is the volume of the rectangular prism.
Volume= length × width × height
To learn more about the Volume of a prism, click on the below link:
https://brainly.com/question/15808751
#SPJ4
Which graph shows h(x) = f(x)g(x)
Answers
The attached graph represents the function h(x), where h(x) = f(x)g(x)
Complete questionConsider the functions f(x) = x - 6 and g(x) = x + 6. Which graph shows h(x) = f(x)g(x)?
How to determine the graph of h(x)?We have
f(x) = x - 6
g(x) = x + 6
The equation h(x) = f(x)g(x) means
h(x) = f(x) * g(x)
So, we have:
h(x) = (x - 6) * (x + 6)
Expand
h(x) = x² - 36
See attachment for the graph of function h(x)
Read more about functions at:
https://brainly.com/question/3791183
#SPJ1

Answer:
A on edg. 2020
Step-by-step explanation:
Which graph shows h(x) = f(x)/g(x)
Daniel is playing on the swings at the playground. At the top of his swing, he will have _____.
neither potential nor kinetic energy
some potential energy and some kinetic energy
no potential energy and all kinetic energy
all potential energy and no kinetic energy
big points
Answers
Answer: all potential energy and no kinetic energy
Step-by-step explanation:
Evaluate the expression for f = –19.8.
Write your answer as a decimal or whole number.
17.8 + f =

Answers
Answer:
-2
Step-by-step explanation:
17.8 + f =
17.8 + (-19.8)
= -2
Answer:
r = radius
h = height
s = slant height
V = volume
L = lateral surface area
B = base surface area
A = total surface area
π = pi = 3.1415926535898
√ = square root
Calculator Use
This online calculator will calculate the various properties of a right circular cone given any 2 known variables. The term "circular" clarifies this shape as a pyramid with a circular cross section. The term "right" means that the vertex of the cone is centered above the base. Using the term "cone" by itself often commonly means a right circular cone.
Units: Note that units are shown for convenience but do not affect the calculations. The units are in place to give an indication of the order of the results such as ft, ft2 or ft3. For example, if you are starting with mm and you know r and h in mm, your calculations will result with s in mm, V in mm3, L in mm2, B in mm2 and A in mm2.
Below are the standard formulas for a cone. Calculations are based on algebraic manipulation of these standard formulas.
Circular Cone Formulas in terms of radius r and height h:
Volume of a cone:
V = (1/3)πr2h
Slant height of a cone:
s = √(r2 + h2)
Lateral surface area of a cone:
L = πrs = πr√(r2 + h2)
Base surface area of a cone (a circle):
B = πr2
Total surface area of a cone:
A = L + B = πrs + πr2 = πr(s + r) = πr(r + √(r2 + h2))
Circular Cone Calculations:
Use the following additional formulas along with the formulas above.
Given radius and height calculate the slant height, volume, lateral surface area and total surface area.
Given r, h find s, V, L, A
use the formulas above
Given radius and slant height calculate the height, volume, lateral surface area and total surface area.
Given r, s find h, V, L, A
h = √(s2 - r2)
Given radius and volume calculate the height, slant height, lateral surface area and total surface area.
Given r, V find h, s, L, A
h = (3 * v) / (πr2)
Given radius and lateral surface area calculate the height, slant height, volume and total surface area.
Given r, L find h, s, V, A
s = L / (πr)
h = √(s2 - r2)
Given radius and total surface area calculate the height, slant height, volume and lateral surface area.
Given r, A find h, s, V, L
s = [A - (πr2)] / (πr)
h = √(s2 - r2)
Given height and slant height calculate the radius, volume, lateral surface area and total surface area.
Given h, s find r, V, L, A
r = √(s2 - h2)
Given height and volume calculate the radius, slant height, lateral surface area and total surface area.
Given h, V find r, s, L, A
r = √[ (3 * v) / (π * h) ]
Given slant height and lateral surface area calculate the radius, height, volume, and total surface area.
Given s, L find r, h, V, A
r = L / (π * s)
h = √(s2 - r2)
Step-by-step explanation:
this is my answer on cone question
true or false? in deductive thinking, you start with a given set of rules and conditions and determine what must be true as a conseqence
Answers
Answer: true hope it helps
Which one of the following is not an assumption about residuals in a regression model?
A. variance of zero
B. normality
C. independence
D. constant variance
Answers
The option that is not an assumption about residuals in a regression model is (A) variance of zero
The assumption of normality means that the residuals follow a normal distribution. This is important because many statistical tests and techniques assume normality, and violating this assumption can lead to biased or inefficient estimates.
The assumption of independence means that the residuals are not correlated with each other. This is important because if the residuals are correlated, it indicates that there are systematic patterns in the unexplained variability that are not accounted for by the model. This can also lead to biased estimates and predictions.
The assumption of constant variance means that the variability of the residuals is the same across all values of the independent variables. This is important because if the variability of the residuals is not constant, it can indicate that the model is not capturing all of the relevant predictors or that there are other sources of unexplained variability that are not accounted for.
The assumption of variance of zero is not an assumption about residuals in a regression model because it is impossible for the residuals to have a variance of zero. If the residuals had zero variance, it would mean that the predicted values perfectly match the observed values, which would render the regression analysis unnecessary.
Therefore, the correct option is (A) variance of zero
Learn more about regression model here
brainly.com/question/14983410
#SPJ4
On which surface is the student unable to apply enough force to overcome the force of friction
Answers
Rough surfaces
Ex=carpet
The student unable to apply enough force to overcome the force of friction on rough surfaces.
What is Force?
A force is an influence that can change the motion of an object.
Friction is the force resisting the relative motion of solid surfaces, fluid layers, and material elements sliding against each other.
We need to find the type of surface the student unable to apply enough force to overcome the force of friction.
A rough surface will have more friction because on the rough surface there will be more irregularities.
So rough surface is the student unable to apply enough force to overcome the force of friction.
Hence, the student unable to apply enough force to overcome the force of friction on rough surfaces.
To learn more on Friction click:
https://brainly.com/question/13000653
#SPJ2
Use the product notation to rewrite the following expression. (t − 6) · (t2 − 6) · (t3 − 6) · (t4 − 6) · (t5 − 6) · (t6 − 6) · (t7 − 6) = π7k = 1
Answers
The expression ((t − 9) · (t² − 9) · (t³ − 9) · (t⁴ − 9) · (t⁵ − 9) · (t⁶ − 9) · (t⁷ − 9) can be written in terms of product notation as Π⁷k=1 \((t^k - 9)\).
As per the question, we can write the expression as:
(t − 9) · (t² − 9) · (t³ − 9) · (t⁴ − 9) · (t⁵ − 9) · (t⁶ − 9) · (t⁷ − 9)
Using product notation, we can write this as:
Π⁷k =1 \((t^k - 9)\)
where Π represents the product of terms, k is the index of the product, and the subscript 7 indicates that the product runs from k = 1 to k = 7.
Therefore, the expression ((t − 9) · (t² − 9) · (t³ − 9) · (t⁴ − 9) · (t⁵ − 9) · (t⁶ − 9) · (t⁷ − 9) can be written in terms of product notation as Π⁷k=1 \((t^k - 9)\).
Learn more about Expressions here:
brainly.com/question/21751419
#SPJ4
Choose the system of equations which matches the following graph.
A. 3x-6y=12
9x-18y=36
B. 3x+6y=12
9x+18y=36
Answers
The system of equations that matches the given graph is:
A. 3x - 6y = 12
9x - 18y = 36
To determine which system of equations matches a given graph, we need to analyze the slope and intercepts of the lines in the graph.
Looking at the options provided:
A. 3x - 6y = 12
9x - 18y = 36
B. 3x + 6y = 12
9x + 18y = 36
Let's analyze the equations in each option:
For option A:
The first equation, 3x - 6y = 12, can be rearranged to slope-intercept form: y = (1/2)x - 2.
The second equation, 9x - 18y = 36, can be simplified to 3x - 6y = 12, which is the same as the first equation.
In option A, both equations represent the same line, as they are equivalent. Therefore, option A does not match the given graph.
For option B:
The first equation, 3x + 6y = 12, can be rearranged to slope-intercept form: y = (-1/2)x + 2.
The second equation, 9x + 18y = 36, can be simplified to 3x + 6y = 12, which is the same as the first equation.
In option B, both equations also represent the same line, as they are equivalent. Therefore, option B does not match the given graph.
for such more question on system of equations
https://brainly.com/question/4262258
#SPJ8
5 and 6 What are the first five terms of the recursive sequence?

Answers
Answer:
4th option and 3rd option
Step-by-step explanation:
Using the recursive rule and a₁ = 7 , then
a₂ = 3a₁ - 6 = (3 × 7) - 6 = 21 - 6 = 15
a₃ = 3a₂ - 6 = (3 × 15) - 6 = 45 - 6 = 39
a₄ = 3a₃ - 6 = (3 × 39) - 6 = 117 - 6 = 111
a₅ = 3a₄ - 6 = (3 × 111) - 6 = 333 - 6 = 327
The first 5 terms are 7, 15, 39, 111, 327
---------------------------------------------------------------------
Similarly using the recursive rule and a₁ = 9
a₂ = 3a₁ + 3 = (3 × 9) + 3 = 27 + 3 = 30
a₃ = 3a₂ + 3 = (3 × 30) + 3 = 90 + 3 = 93
a₄ = 3a₃ + 3 = (3 × 93) + 3 = 279 + 3 = 282
a₅ = 3a₄ + 3 = (3 × 282) + 3 = 846 + 3 = 849
The first 5 terms are 9, 30, 93, 282, 849
which measure of center best represents this set of data: red, blue, red, yellow, red, green, black, red, white, red, red. a) Mean. b) Mode. c) Median.
Answers
The mode best represents the set of data: red, blue, red, yellow, red, green, black, red, white, red. The mode is a statistical measure that represents the most frequently occurring value or values in a dataset.
The mode is the measure of center that represents the most frequently occurring value in a dataset. In this case, the color "red" appears most frequently, occurring 6 times out of the 11 data points. Therefore, the mode of the dataset is "red."
The mean, on the other hand, calculates the average value by summing all the data points and dividing by the total number of data points. The mean can be influenced by extreme values or outliers, which may not accurately represent the overall data.
The median is the middle value when the data is arranged in ascending or descending order. In this case, since there are 11 data points, the median would be the sixth value. However, there is no clear middle value as there are multiple occurrences of "red" in the dataset.
Hence, the mode, representing the most frequently occurring value, is the measure of center that best represents this set of data.
Learn more about mode here:
brainly.com/question/1157284
#SPJ11