The Price of Pollo
In El Salvador, "Country Chicken" is the most popular fried chicken franchise
in the country. Like most fast-food establishments, they provide a carry-out
service on their menu. You can buy their chicken in several different
quantities: 2, 6, 9, 15, or 21 pieces per box.
Over the years, prices have steadily risen, as things have a way of doing in
many areas of modern life. For example, on July 1, 1993, a box of two
pieces cost 8.35 colones, and on December 31, 1995, that same purchase
would cost you 11.25 colones.
(Note: prices are given in their original Salvadorean currency, colones; $1 U.S
colones.)
Using these two data 'points', you can form a linear equation of the slope-inter
mx + b. The independent variable x is time; the dependent variable y represe
Your task for this problem is to:
1. Find this equation.
2. Use your equation to predict what the price should have been for a 2
July 1, 1999.
Answers
Answer:
Hope I helped!~
Step-by-step explanation:
To find the equation of the line, we can use the slope-intercept form of the equation:
y = mx + b
where m is the slope of the line and b is the y-intercept.
Using the two data points given, we can calculate the slope:
m = (11.25 - 8.35) / (1995 - 1993) = 1.95
To find the y-intercept, we can use one of the data points:
8.35 = 1.95(1993) + b
b = -3884.65
So the equation of the line is:
y = 1.95x - 3884.65
To predict the price of a box of two pieces on July 1, 1999, we can substitute x = 6 (since 1999 is 6 years after 1993) into the equation:
y = 1.95(6) - 3884.65
y = 11.7 - 3884.65
y = -3872.95
This gives us a negative price, which obviously does not make sense. It is likely that the price of a box of two pieces was not linearly increasing during this time period, or that there were other factors influencing the price. Therefore, we cannot use this equation to accurately predict the price of a box of two pieces on July 1, 1999.
Related Questions
Billy started $68.00 in his checking account. he went out to dinner. he now has $47.50. how much money did he spend on dinner?
A. $20.00
B. $12.50
C. $10.00
D. $20.50
Answers
Step-by-Step Explanation:
Starting cash = $ 68.00
Balance cash = $ 47.50
We can obtain the amount of money he spent on dinner by subtracting the Balance cash from the Starting cash:
= 68 - 47.50
= 20.50
Therefore, Billy spent $20.50 on Dinner.
Simplify: −6ru2−ur2−22u2r2
Answers
The simplified form of\(-6ru^2 -ur^2 - 22u^2r^2\)is \(-u^2(7r + 22r^2)\).
To simplify the expression\(-6ru^2 -ur^2 - 22u^2r^2\), we can combine like terms and factor out common factors.
First, let's look at the variables r and u separately:
For r:
We have terms\(-6ru^2\) and \(-ur^2.\) We can factor out r from these terms:
\(r(-6u^2 - u^2)\\r(-7u^2)\)
For u:
We have term\(-22u^2r^2\). We can factor out\(u^2\)from this term:
\(u^2(-22r^2)\)
Combining the simplified terms for r and u, we get:
\(r(-7u^2) + u^2(-22r^2)\)
Now, we can factor out the common factor of\(-u^2\):
\(u^2(7r + 22r^2)\)
Therefore, the simplified expression is\(-u^2(7r + 22r^2)\) .
For more such questions on simplified visit:
https://brainly.com/question/723406
#SPJ8
Use properties of exponents and logarithms to evaluate the expression as a rational number.

Answers
y= 1/16 is the solution of the equation where y is \(e^{-2ln4}\)
The expression is given to be \(e^{-2ln4}\)
The formula is given to be \(e^{lnx} =x\\\)
also it is given that ln\(e^{x}\)=x
Now to find and evaluate \(e^{-2ln4}\)
let y=\(e^{-2ln4}\)
taking ln both sides,
ln y = ln\(e^{-2ln4}\)
ln y= -2 ln4
ln y= ln 4^-2
Removing ln from both sides,
y = 4^-2
y=1/16
Thus , y= 1/16 is the solution of the equation
#SPJ9
please help meeeeeeeeeeeeeeeee

Answers
Answer:
hi
Step-by-step explanation:
i think so...
\(\pi \times {r}^{2} \times \frac{h}{3} \\ 3.14 \times 3.5 \times \frac{9}{3} \\ = 32.97\)
hope it helps
bye
A population of values has a normal distribution with �=189.7 and �=96.7. You intend to draw a random sample of size �=62.
Find the probability that a single randomly selected value is between 189.7 and 213.
P(189.7 < X < 213) =
Find the probability that a sample of size �=62 is randomly selected with a mean between 189.7 and 213.
P(189.7 < M < 213) =
Enter your answers as numbers accurate to 4 decimal places. Answers obtained using exact z-scores or z-scores rounded to 3 decimal places are accepted.
Answers
The probability that a sample of size n = 62 is randomly selected with a mean between 189.7 and 213 is approximately 0.9702.
To find the probability that a single randomly selected value is between 189.7 and 213, we can use the standard normal distribution.
Step 1: Calculate the z-scores for the given values using the formula:
z = (x - μ) / σ
For 189.7:
z1 = (189.7 - 189.7) / 96.7 = 0
For 213:
z2 = (213 - 189.7) / 96.7 ≈ 0.2417
Step 2: Utilize a standard typical conveyance table or number cruncher to find the probabilities comparing to the z-scores.
P(189.7 < X < 213) = P(0 < Z < 0.2417) ≈ 0.0939
Therefore, the probability that a single randomly selected value is between 189.7 and 213 is approximately 0.0939.
To find the probability that a sample of size n = 62 is randomly selected with a mean between 189.7 and 213, we use the central limit theorem. Under specific circumstances, the testing dispersion of the example mean methodologies a typical conveyance
Step 1: Calculate the standard error of the mean (σ_m) using the formula:
σ_m = σ / sqrt(n)
σ_m = 96.7 / sqrt(62) ≈ 12.2878
Step 2: Convert the given qualities to z-scores utilizing the equation:
z = (x - μ) / σ_m
For 189.7:
z1 = (189.7 - 189.7) / 12.2878 = 0
For 213:
z2 = (213 - 189.7) / 12.2878 ≈ 1.8967
Step 3: Utilize a standard typical conveyance table or mini-computer to find the probabilities relating to the z-scores.
P(189.7 < M < 213) = P(0 < Z < 1.8967) ≈ 0.9702
Therefore, the probability that a sample of size n = 62 is randomly selected with a mean between 189.7 and 213 is approximately 0.9702.
For more such questions on probability, click on:
https://brainly.com/question/7965468
#SPJ8
Consider the function \(y=\sqrt{5x-5}+1\)
Which inequality is used to find the domain?
Answers
The inequality is used to find the domain of the given function is
5x-5 ≥ 0
What is a function?A function is a relation from a set of inputs to a set of possible outputs, where each input is related to exactly one output.
Given is function y = √(5x-5)+1, we have to find which inequality can be used to find the domain.
Since, the function is a square root function.
We know that,
The square root function is defined only for the positive values, including 0. i.e. the expression inside the square root must be greater than or equal to 0.
The expression inside the square root is (5x-5) so that must be greater than or equal to 0 which can be written as :
5x-5 ≥ 0
Hence, the inequality is used to find the domain of the given function is 5x-5 ≥ 0
Learn more about functions, click;
https://brainly.com/question/21145944
#SPJ1
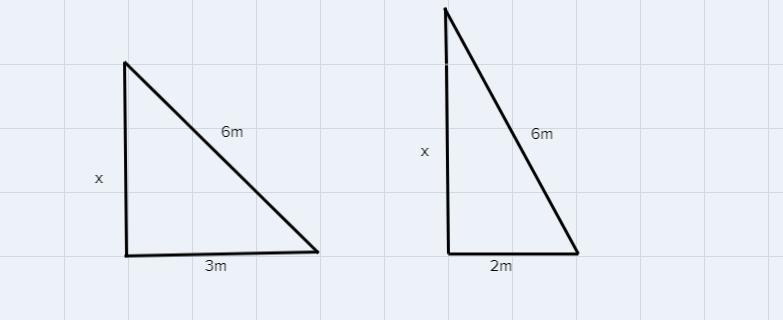
A ladder is 6 m long. How much farther up a wall does the ladder reach when the base of the ladder is 2 m from the wall than when it is 3 m from the wall? Round to the nearest tenth of a meter.
Answers
ANSWER
0.5m UP
EXPLANATION
Given:
Determine the value of x at 3m using Pythagorean Theorem
\(\begin{gathered} Hyp^2\text{ = Opp + Adj}^2 \\ 6^2\text{ = x}^2\text{ + 3}^2 \\ x^2\text{ = 36 - 9} \\ x\text{ = }\sqrt{27} \\ x\text{ = 5.2} \end{gathered}\)Determine the value of x at 2m using Pythagorean Theorem
\(\begin{gathered} Hyp^2\text{ = Opp}^2\text{ + Adj}^2 \\ 6^2\text{ = x}^2\text{ + 2}^2 \\ x^2\text{ = 36 -4} \\ x\text{ = }\sqrt{32} \\ x\text{ = 5.7} \end{gathered}\)The Difference
5.7 - 5.2 = 0.5 m
Hence, the ladder reach 0.5m farther up when the base of the ladder is 2 m from the wall than when it is 3 m from the wall
if you take away 25 from a number you will be left with two and halftimes 30. what is the number?
Answers
If you take away 25 from (100) you get 75.
70 also equals 2.5*30
Solve for y.
−2y+5=−11
Responses
y = 8
y, = 8
y = 3
y, = 3
y=−3
y equals negative 3
y=−8
Answers
Answer:
8
Step-by-step explanation:
bResponses
y = 8
y, = 8
y = 3
y, = 3
y=−3
If the relationship is proportional, what is the missing value from the table?

Answers
How many sides does an octagon have?
sides
Answers
Answer:
8 do u have a pic of it but I think it’s 8
Step-by-step explanation:
Answer:
eight
Step-by-step explanation:
3 - Triangle
4 - Quadrilateral
5 - Pentagon
6 - Hexagon
7 - Heptagon or Septagon
8 - Octagon
9 - Nonagon
For a certain horse race ,the odds in favor of a certain horse finishing in second place are given as 49 to 51.
Express the indicated degree of likelihood as a probability value between 0 and 1 inclusive.
The probability is = ?
Answers
The probability value between 0 and 1 inclusive represents the given degree of likelihood as 19/100.
What is the probability?Simply put, the probability is the likelihood that something will occur. When we don't know how an event will turn out, we can discuss the likelihood or likelihood of several outcomes.
Statistics is the study of events that follow a probability distribution.
In terms of percentage notation, it is expressed as a number between 0 and 1, or between 0% and 100%.
The higher the likelihood, the more likely it is that the event will take place.
So, in the given situation, the probability would be:
first 19+81=100
then P=m/n
so P=19/100
Therefore, the probability value between 0 and 1 inclusive represents the given degree of likelihood as 19/100.
Know more about probability here:
https://brainly.com/question/24756209
#SPJ1
Correct question:
For a certain horse race, the odds in favor of a certain horse finishing in second place horse race, odds in favor of a certain horse finishing in second place are given as 19to 81. Express the indicated degree of likelihood as a probability value between 0 and 1 inclusive.
BRAINLIEST STARS
Simplify:
4+ (15 – 14) X 6
O A. 65
B. 30
O c. 24
D. 10
Answers
The perimeter of the triangle is
equal to the area of the rectangle.
What is the value of x?

Answers
Answer:
x=-21
Step-by-step explanation:
make the equation
2x+9x-1+40=6(x-11)
simplify
11x+39=6x-66
solve
5x=-105
x=-21
Solve the inequality and graph the solution on the line provided. 6x-6<-30
Answers
The solution to the inequality 6x - 6 < -30 is x < -4, and it is graphically represented as a closed circle at -4 and shading to the left of -4 on the number line.
To solve the inequality 6x - 6 < -30, we can follow these steps:
Step 1: Add 6 to both sides of the inequality to isolate the variable:
6x - 6 + 6 < -30 + 6
6x < -24
Step 2: Divide both sides of the inequality by 6 to solve for x:
(6x)/6 < (-24)/6
x < -4
The solution to the inequality is x < -4. This means that any value of x less than -4 will satisfy the inequality.
To graph the solution on the number line, we represent -4 as a closed circle (since it is not included in the solution) and shade the region to the left of -4 to indicate all values less than -4.
On the number line, mark a point at -4 with a closed circle:
<--------●-----------------
Then, shade the region to the left of -4:
<--------●================
The shaded region represents the solution to the inequality x < -4.
For more such question on inequality visit:
https://brainly.com/question/30238989
#SPJ8
Find the simplified product:
sqrt 2x^2 * sqrt18^5
Answers
Answer:
1944x^2.
Step-by-step explanation:
sqrt 2x^2 * sqrt18^5
= sqrt2x^2 * (sqrt(9^5*2^5))
= sqrt 2 x^2 * (sqrt9^5 * sqrt 2^5)
= sqrt2 x^2 * (243* sqrt2^5)
= 2^0.5 (243 * 2^2.5) x^2
= 2^3 * 243 x^2
= 1944x^2.
The simplified product of √(2x³) × √(18⁵) is 1944 \(x^{(3/2)\).
To simplify the product √(2x³) × √(18⁵), we can use the properties of square roots.
First, let's simplify each square root separately:
√(2x³) can be written as √(2) × √(x³).
√(18⁵) can be written as √(18) × √(18⁴).
Now, let's simplify each term:
√(2) is the square root of 2.
√(x³) is the square root of x³, which can be written as \(x^{(3/2)\).
√(18) is the square root of 18.
√(18⁴) is the square root of 18⁴, which can be written as 18².
Combining these simplified terms, we get:
√(2x³) × √(18⁵) = (√(2) × √(x³)) × (√(18) × √(18⁴))
\(= (\sqrt2 \times x^{(3/2)}) \times (\sqrt18 \times 18^2)\)
= 3 × √2 × √2 \(x^{(3/2)\) × 18²
= 3 × 2 × \(x^{(3/2)\) × 18²
= 6 \(x^{(3/2)\) × 18²
= 6 \(x^{(3/2)\) × 324
= 1944 \(x^{(3/2)\).
Therefore, the simplified product of √(2x³) × √(18⁵) is 1944 \(x^{(3/2)\).
Learn more about properties of square roots click;
https://brainly.com/question/11956803
#SPJ6
Write down the equation in slope intercept form if slope m=4 and y intercept is (0,-5). Do not leave spaces.

Answers
Answer:
y=4x-5
Step-by-step explanation:
y=mx+c
m=4
c = y-intercept = -5
y=4x-5
if the company decides to produce 2,000 containers of regular crunchy peanut butter, how many containers of new extra crunchy peanut butter would it produce?
Answers
The number of extra crunchy peanut butter that the company would produce (current production units) is 1,700 containers.
How is the number determined?Using the mathematical operation of subtraction, the number of units to be produced can be determined.
First, we have that the ending inventory for the last period was 300 containers.
This ending inventory is subtracted from the required production level, to determine the number of containers to produce for this period.
Thus, to meet the production requirements, the company would produce additional 1,700 containers of crunchy peanut butter.
Learn more about mathematical operations at brainly.com/question/20628271
#SPJ1
Question Completion:Ending inventory of regular crunchy peanut butter from last period = 300 containers.
Which are solutions of the equation x2 - 16 = 0? Check all that apply.
x= -8
x=-4
x=-2
x= 2
x= 4
x=8
Answers
Answer:
B) -4 and E) 4
Step-by-step explanation:
Compare√150and 114/9 using <,>, or }=
√150>114/9
√150=114/9
114/9<√150
114/9>√150

Answers
114/9 > √150 that is, 114/9 is greater than √150
Given,
We have to compare √150 and 114/9 using <, >, or =
Comparison between two numbers;
Mathematical number comparison is the process or method of comparing two numbers to determine whether one is less, bigger, or equal to the other. The comparison symbols for numbers are "=", which stands for "equal to," "=", which stands for "greater than," and " ", which stands for "less than."
Here,
First we have to find the value of √150 and 114/9
That is,
The value of √150 = 12.25
The value of 114/9 = 12.66
Therefore,
We came to know that 114/9 is greater than √150 that is, 114/9 > √150
Learn more about comparison between numbers here;
https://brainly.com/question/28899705
#SPJ4
In a sample of 528 customers, 237 say they are happy with the service. If you select three customers without replacement for a commercial, what is the probability they will all say they are happy with the service
Answers
Answer: 0.0898
Step-by-step explanation:
Probability = \(\dfrac{favorable\ outcomes}{Total\ outcomes}\)
Given : Sample size : 528
Number pf customers say they are happy = 237
Probability of getting one happy customer= \(\dfrac{237}{528}\)
Probability of getting second happy customer= \(\dfrac{236}{527}\) [subtract 1 from both numbers]
Probability of getting third happy customer= \(\dfrac{235}{526}\)
If 3 customers are elected without replacement
The probability they will all say they are happy with the service = \(\dfrac{237}{528}\times\dfrac{236}{527}\times\dfrac{235}{526}\)
\(\approx0.0898\)
Hence, the required probability = 0.0898
What is 56 divided by 89?
Answers
Answer:
0 or 0.62921348314 (if done by the calculator)
Step-by-step explanation:
Step 1:
Start by setting it up with the divisor 89 on the left side and the dividend 56 on the right side like this:
8 9 ⟌ 5 6
Step 2:
The divisor (89) goes into the first digit of the dividend (5), 0 time(s). Therefore, put 0 on top:
0
8 9 ⟌ 5 6
Step 3:
Multiply the divisor by the result in the previous step (89 x 0 = 0) and write that answer below the dividend.
0
8 9 ⟌ 5 6
0
Step 4:
Subtract the result in the previous step from the first digit of the dividend (5 - 0 = 5) and write the answer below.
0
8 9 ⟌ 5 6
- 0
5
Step 5:
Move down the 2nd digit of the dividend (6) like this:
0
8 9 ⟌ 5 6
- 0
5 6
Step 6:
The divisor (89) goes into the bottom number (56), 0 time(s). Therefore, put 0 on top:
0 0
8 9 ⟌ 5 6
- 0
5 6
Step 7:
Multiply the divisor by the result in the previous step (89 x 0 = 0) and write that answer at the bottom:
0 0
8 9 ⟌ 5 6
- 0
5 6
0
Step 8:
Subtract the result in the previous step from the number written above it. (56 - 0 = 56) and write the answer at the bottom.
0 0
8 9 ⟌ 5 6
- 0
5 6
- 0
5 6
f(x)=5x-12what is f(-4)
Answers
multiply 5(-4) and get -20
-20 and add -12
answer is -32
The figure shows three tennis balls in a can with each tennis ball having a diameter of 2.5 inches. What is the total volume of the air space around the three tennis balls?
Answers
The total volume of the air space of spherical ball is A = 12.265625 inches³
Given data ,
Since each tennis ball has a diameter of 2.5 inches, the radius of each ball is 1.25 inches.
The air space around the balls can be thought of as a cylinder with a height equal to the diameter of one ball and a radius equal to the radius of one ball.
The height of the cylinder is 2.5 inches, and the radius is 1.25 inches.
The formula for the volume of a cylinder is:
V = πr²h
V = ( 3.14 ) ( 1.25 )² ( 7.5 )
V = 36.796875 inches³
where V is the volume, r is the radius, and h is the height.
So, the volume of the one ball is:
V₁ = ( 4/3 )π(1.25)³
V₁ = 8.177083 inches³
The total volume of three balls is = volume of 3 spherical balls
V₂ = 3V₁ = 3(8.177083) ≈ 24.53125 cubic inches
Therefore, the total volume of the air space around the three tennis balls is approximately A = 36.796875 inches³ - 24.53125 inches³
A = 12.265625 inches³
Hence , the volume of air space is A = 12.265625 inches³
To learn more about sphere click :
https://brainly.com/question/27933834
#SPJ1
If 20% of X =y, then y% of 20 is the same as:
Answers
Answer:
4% of x
Step-by-step explanation:
20% of x
20% of x = 0.2x20% of x = y ⇒ 0.2x = yy% of 20
y% of 20 = 20*y/100 = 0.2y0.2y = 0.2*0.2x = 0.04xy% of 20 = 4% of xHope it is what you are looking for
Find the midpoint of the segment with the following endpoints.
(-4,-9) and (4, -1)
Answers
Answer:
\((0,-5)\)
General Formulas and Concepts:
Pre-Alg
Order of Operations: BPEMDASAlg I
Midpoint Formula: \((\frac{x_1+x_2}{2},\frac{y_1+y_2}{2})\)Step-by-step explanation:
Step 1: Define
Point (-4, -9)
Point (4, -1)
Step 2: Find midpoint
Substitute: \((\frac{-4+4}{2},\frac{-9-1}{2})\)Add/Subtract: \((\frac{0}{2},\frac{-10}{2})\)Divide: \((0,-5)\)URGENT PLZ HELP
Solve for x. Assume that lines which appear to be diameters are actual diameters.

Answers
Answer:
Step-by-step explanation:
This is a central angel so the degree of the angle is equal to the degree of the arc which is 111.
X+114=111
add negative -114 to each side to get X alone.
-114+X+114= 111+-114
X=-3 . Answer negative 3
A function of random variables used to estimate a parameter of a distribution is a/an _____.
Answers
Answer:
an unbiased estimator
Step-by-step explanation:
The Venn diagram shows the number of customers who have purchased different types of pets from a pet store, where C represents customers who have purchased cats, D represents customers who have purchased dogs, and F represents customers who have purchased fish.
Circles C, D, and F overlap. Circle C contains 15, circle D contains 21, and circle F contains 12. The overlap of C and F contains 2, the overlap of F and D contains 0, and the overlap of D and C contains 3. The overlap of all 3 circles contains 1. Number 14 is outside of the circles.
How many people are in the set C ∩ D?
4
6
36
38
Answers
The number of people in the set C ∩ D (customers who purchased both cats and dogs) is obtained by adding the overlap of D and C (3) with the overlap of all 3 circles (1), resulting in a total of 4 individuals.
The correct answer is 4.
To determine the number of people in the set C ∩ D (customers who have purchased both cats and dogs), we need to analyze the overlapping regions in the Venn diagram.
Given information:
- Circle C (cats): 15
- Circle D (dogs): 21
- Circle F (fish): 12
- Overlap of C and F: 2
- Overlap of F and D: 0
- Overlap of D and C: 3
- Overlap of all 3 circles: 1
- Number outside of circles: 14
To determine the number of people in the set C ∩ D (customers who have purchased both cats and dogs), we need to consider the overlapping region between circles C and D.
From the information given, we know that the overlap of D and C is 3. Additionally, we have the overlap of all 3 circles, which is 1. The overlap of all 3 circles includes the region where customers have purchased cats, dogs, and fish.
To calculate the number of people in the set C ∩ D, we add the overlap of D and C (3) to the overlap of all 3 circles (1). This gives us 3 + 1 = 4.
Therefore, from the options given correct one is 4.
For more such information on: set
https://brainly.com/question/24713052
#SPJ8
Answer: 4
Step-by-step explanation:
trust me bro
A 6 inch personal pizza has 620 calories, with 240 of those from fat. A 12 inch pizza is cut into 8 slices. Estimate the number of calories in one slice of a 12 inch pizza.
Answers
9514 1404 393
Answer:
310 calories (120 from fat)
Step-by-step explanation:
A 12-inch pizza has double the diameter of the 6-inch pizza, so will have 2² = 4 times the area. 1/8 of that pizza will have 4/8 = 1/2 the area of the personal pizza. If calories are proportional to the area, then the slice will have ...
(1/2)(620 calories) = 310 calories . . . in 1 slice of 12-in pizza