Test the claim that the mean GPA of night students is smaller than 2.4 at the .05 significance level. The null and alternative hypothesis would be:
a. H0 : μ = 2.4 Ha : μ > 2.4 b. H0 : p = 0.6 Ha : p < 0.6 c. H0 : p = 0.6 Ha : p ≠ 0.6 d. Η0 : μ = 2.4 Ha :μ ≠ 2.4 e. H0 : p = 0.6 Ha : p > 0.6 f. H0 : μ = 2.4 Ha : μ < 2.4 The test is: a. right-tailed b. left-tailed
c. two-tailed
Answers
The null and alternative hypotheses for testing the claim that the mean GPA of night students is smaller than 2.4 at the 0.05 significance level would be: H0: μ = 2.4 and Ha: μ < 2.4. The test is left-tailed.
In hypothesis testing, the null hypothesis (H0) represents the claim we are testing, while the alternative hypothesis (Ha) represents the alternative claim or what we suspect to be true. In this case, we are testing whether the mean GPA of night students is smaller than 2.4.
Since we are interested in determining if the mean GPA is smaller, the alternative hypothesis (Ha) would be that the mean GPA (μ) is less than 2.4. Therefore, the correct pair of hypotheses is H0: μ = 2.4 (null hypothesis) and Ha: μ < 2.4 (alternative hypothesis).
To determine the type of test (right-tailed, left-tailed, or two-tailed), we consider the alternative hypothesis. In a left-tailed test, the alternative hypothesis suggests a decrease or a shift to the left of the null hypothesis. In this case, since we are testing if the mean GPA is smaller than 2.4, it implies a left-tailed test.
Therefore, the correct answer is b. left-tailed, corresponding to the null and alternative hypotheses H0: μ = 2.4 and Ha: μ < 2.4.
Learn more about significance level here:
brainly.com/question/31519103
#SPJ11
Related Questions
Kenneth earns $9 per hour mowing lawns. The total amount earned t equals the amount earned per
hour times the number of hours h. Which equation gives the total amount earned tif Kenneth worksh
hours?
Answers
hope this helped :)
T=9h
I probably anwserd Late also I was stumped on this to
A hot air balloon descends from an altitude of 2,000 feet at a constant rate of 90 feet per minute. The graph shows the altitude of the balloon over time. Write a linear function in the form y = mx + b to represent the situation.

Answers
90 is the rate of change because the hot air balloon descends 90 feet per minute.
And 2,000 is the starting point because the hot air balloon started the descent at 2,000 feet.
Answer:
y=90x+2,000
y=mx+b
The combination of a lock uses numbers 1-10
Each number is only used once and it is a 3 number code.
What is the probability that you will get the combination correct on the first try?
a
1/6
b
3/80
c
1/720
d
1/1000
Answers
Answer:If the digit of 0 is allowed, then:
1/(10*9*8)= 1/720
If it is not permitted, then:
1/(9*8*7)= 1/504
I hope this helps you!
Step-by-step explanation:
Enter the solution (x, y) to the system of equations shown.
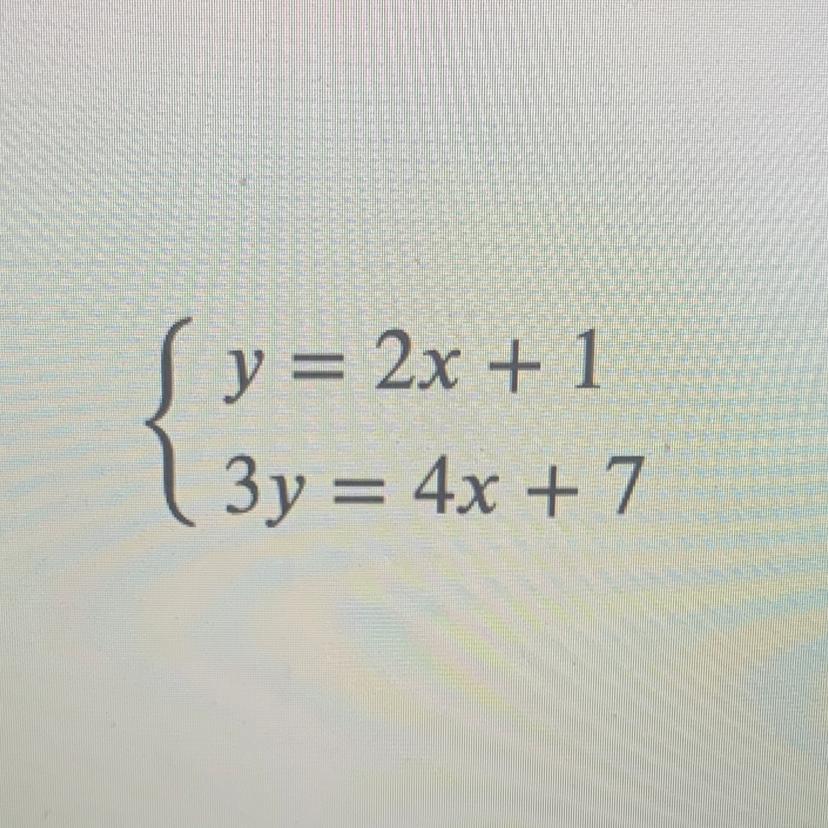
Answers
Answer:
(2, 5 )
Step-by-step explanation:
y = 2x + 1 → (1)
3y = 4x + 7 → (2)
substitute y = 2x + 1 into (2)
3(2x + 1) = 4x + 7 ← distribute parenthesis on left side
6x + 3 = 4x + 7 ( subtract 4x from both sides )
2x + 3 = 7 ( subtract 3 from both sides )
2x = 4 ( divide both sides by 2 )
x = 2
substitute x = 2 into (1) for corresponding value of y
y = 2(2) + 1 = 4 + 1 = 5
solution is (2, 5 )
Sara will use 7 cups of apples to make 4 batches of applesauce. Choose all of the expressions that show the number of cups of apples in one batch.

Answers
To make 4 batches of applesauce, 7 cups of apples are needed.
So the number of cups, of apples in one batch is calculated as by taking the ratio as 4:7 to 1:x . Here xis the number of cups for 1 batch.
\(\begin{gathered} \frac{4}{7}=\frac{1}{x} \\ 4x=7 \\ x=\frac{7}{4} \end{gathered}\)So, from the expressions, we can see that,
(A) is correct option,
(B) is correct option,
(E) is correct option
(F) is correct option
As these all corresponds to "7/4"
Factor the polynomial 2^2+ 16x + 24:
Answers
Answer:
4(4x+7)
Step-by-step explanation:
all I did was evaluate the exponent then added all the numbers to then rearrange the terms and the use your common factors
Can someone help me wit da question listed in da picture?

Answers
Answer:
Add 8 to both sides
Step-by-step explanation:
Normally after adding 8 to both sides you'd solve for x by then dividing by -5, but they just want u to explain what to do lol.
F(x)= (x-2)(x+4)(x+5)What is the sign of f on the interval -4
Answers
By evaluating the polynomial, we will see that the sign on the interval [-4, 2] is negative.
What is the sign of f on the interval [-4, 2]?Here we have the polynomial:
f(x) = (x - 2)*(x + 4)*(x + 5).
You can see that the roots of the polynomial are at:
x = 2
x = -4
x = -5.
Then, on the interval [-4, 2], the sign of the polynomial don't change. So to get the sign of f(x) on that interval we can just evaluate it in any value of x that belongs to the interval, for example, x = 0.
f(0) = (0 - 2)*(0 + 4)*(0 + 5) = -2*4*5 = -40
With this, we can conclude that the sign of f(x) on the given interval is negative.
if you want to learn more about polynomials:
https://brainly.com/question/4142886
#SPJ1
Answer:
always negative
Step-by-step explanation:
If Y has a binomial distribution with parameters n and p, then p(hat)1 = Y/n is an unbiased estimator of p. Another estimator of p is p(hat)2 = (Y+1)/(n+2).
a. Derive the biase of p(hat)2.
b. Derive MSE(Pphat)1) and MSE(p(hat)2).
c. For what values of p is MSE(p(hat)1) < MSE(p(hat)2)?
Answers
a. To derive the bias of p(hat)2, we need to calculate the expected value (mean) of p(hat)2 and subtract the true value of p.
Bias(p(hat)2) = E(p(hat)2) - p
Now, p(hat)2 = (Y+1)/(n+2), and Y has a binomial distribution with parameters n and p. Therefore, the expected value of Y is E(Y) = np.
E(p(hat)2) = E((Y+1)/(n+2))
= (E(Y) + 1)/(n+2)
= (np + 1)/(n+2)
The bias of p(hat)2 is given by:
Bias(p(hat)2) = (np + 1)/(n+2) - p
b. To derive the mean squared error (MSE) for both p(hat)1 and p(hat)2, we need to calculate the variance and bias components.
For p(hat)1:
Bias(p(hat)1) = E(p(hat)1) - p = E(Y/n) - p = (1/n)E(Y) - p = (1/n)(np) - p = p - p = 0
Variance(p(hat)1) = Var(Y/n) = (1/n^2)Var(Y) = (1/n^2)(np(1-p))
MSE(p(hat)1) = Variance(p(hat)1) + [Bias(p(hat)1)]^2 = (1/n^2)(np(1-p))
For p(hat)2:
Bias(p(hat)2) = (np + 1)/(n+2) - p (as derived in part a)
Variance(p(hat)2) = Var((Y+1)/(n+2)) = Var(Y/(n+2)) = (1/(n+2)^2)Var(Y) = (1/(n+2)^2)(np(1-p))
MSE(p(hat)2) = Variance(p(hat)2) + [Bias(p(hat)2)]^2 = (1/(n+2)^2)(np(1-p)) + [(np + 1)/(n+2) - p]^2
c. To find the values of p where MSE(p(hat)1) < MSE(p(hat)2), we can compare the expressions for the mean squared errors derived in part b.
(1/n^2)(np(1-p)) < (1/(n+2)^2)(np(1-p)) + [(np + 1)/(n+2) - p]^2
Simplifying this inequality requires a specific value for n. Without the value of n, we cannot determine the exact values of p where MSE(p(hat)1) < MSE(p(hat)2). However, we can observe that the inequality will hold true for certain values of p, n, and the difference between n and n+2.
To learn more about binomial distribution: -brainly.com/question/29137961
#SPJ11
In the given scenario, we have two estimators for the parameter p of a binomial distribution: p(hat)1 = Y/n and p(hat)2 = (Y+1)/(n+2). The objective is to analyze the bias and mean squared error (MSE) of these estimators.
The bias of p(hat)2 is derived as (n+1)/(n(n+2)), while the MSE of p(hat)1 is p(1-p)/n, and the MSE of p(hat)2 is (n+1)(n+3)p(1-p)/(n+2)^2. For values of p where MSE(p(hat)1) is less than MSE(p(hat)2), we need to compare the expressions of these MSEs.
(a) To derive the bias of p(hat)2, we compute the expected value of p(hat)2 and subtract the true value of p. Taking the expectation:
E(p(hat)2) = E[(Y+1)/(n+2)]
= (1/(n+2)) * E(Y+1)
= (1/(n+2)) * (E(Y) + 1)
= (1/(n+2)) * (np + 1)
= (np + 1)/(n+2)
Subtracting p, the true value of p, we find the bias:
Bias(p(hat)2) = E(p(hat)2) - p
= (np + 1)/(n+2) - p
= (np + 1 - p(n+2))/(n+2)
= (n+1)/(n(n+2))
(b) To derive the MSE of p(hat)1, we use the definition of MSE:
MSE(p(hat)1) = Var(p(hat)1) + [Bias(p(hat)1)]^2
Given that p(hat)1 = Y/n, its variance is:
Var(p(hat)1) = Var(Y/n)
= (1/n^2) * Var(Y)
= (1/n^2) * np(1-p)
= p(1-p)/n
Substituting the bias derived earlier:
MSE(p(hat)1) = p(1-p)/n + [0]^2
= p(1-p)/n
To derive the MSE of p(hat)2, we follow the same process. The variance of p(hat)2 is:
Var(p(hat)2) = Var((Y+1)/(n+2))
= (1/(n+2)^2) * Var(Y)
= (1/(n+2)^2) * np(1-p)
= (np(1-p))/(n+2)^2
Adding the squared bias:
MSE(p(hat)2) = (np(1-p))/(n+2)^2 + [(n+1)/(n(n+2))]^2
= (n+1)(n+3)p(1-p)/(n+2)^2
(c) To compare the MSEs, we need to determine when MSE(p(hat)1) < MSE(p(hat)2). Comparing the expressions:
p(1-p)/n < (n+1)(n+3)p(1-p)/(n+2)^2
Simplifying:
(n+2)^2 < n(n+1)(n+3)
Expanding:
n^2 + 4n + 4 < n^3 + 4n^2 + 3n^2
To learn more about binomial distribution: -brainly.com/question/29137961
#SPJ11
Write the equation in standard form that has a slope of -5/2 and a y-intercept of -2
Answers
Answer:
y = -5/2 x - 2
Step-by-step explanation:
The standard form of equation of a line is expressed as;
y = mx+c
m is the slope
c is the yintercept
Given
m = -5/2
c = -2
Substotute into the exoression above
y = -5/2 x + (-2)
y = -5/2 x - 2
Hence the equation in standard form is y = -5/2 x - 2
You report a confidence interval to your boss but she says that she wants a narrower range. SELECT ALL of the ways you can reduce the width of the confidence interval. o Increase the sample size o Decrease the sample size o Increase the confidence level o Decrease the confidence level I
o ncrease the mean o Decrease the mean
Answers
We can reduce the width of the confidence interval by increasing the sample size, reducing the confidence level and decreasing the mean (A, D, and F)
What is a confidence interval?A confidence interval is an estimate of the interval that has a specified probability of including an unknown population parameter.
The purpose of a confidence interval is to estimate the true value of the population parameter being measured, such as a mean, a standard deviation, or a proportion.
In general, a wider confidence interval indicates more uncertainty about the estimate, while a narrower confidence interval suggests more precision in the estimate.
We want narrower confidence intervals to demonstrate more precision, as this allows us to draw more reliable conclusions about population parameters.
We cannot reduce the width of the confidence interval by increasing the sample size, increasing the confidence level or increasing the mean.
Thus, the correct options are a, d, and f.
Learn more about confidence interval here:
https://brainly.com/question/15712887
#SPJ11
please help on this

Answers
Answer:
The first on is -80,
next one is -60
third one 3
last one is -54
Step-by-step explanation:
How to graph y=x^2+6x+7 on a quadratic graph

Answers
The graph of the function y = x²+ 6x + 7 is given in the attachment.
To graph the quadratic function y = x²+ 6x + 7:
Find the vertex using the formula -b/(2a), where a is the coefficient of x²and b is the coefficient of x.
The vertex will be at (-3, -2).
Plot the vertex (-3, -2) on the graph.
Choose additional x-values and calculate the corresponding y-values by substituting them into the equation.
Plot these points on the graph.
Connect the plotted points smoothly to form a U-shaped curve, which represents the parabola.
To learn more on Graph click:
https://brainly.com/question/17267403
#SPJ1

a busy student must complete 3 problem sets before doing laundry. each problem set requires 1 day with probability 2/3 and 2 days with probability 1/3. let b be the number of days a busy student delays laundry. what is ex(b)
Answers
The value of expected time ex(b)=1 day approximately if b is the number of days a busy student delays Laundry.
What is meant by expected time?The basic anticipated value formula is (P(x) * n): the probability of an occurrence multiplied by the number of times the event occurs.
The expected value (also known as the expectation, expectancy, mathematical expectation, mean, average, or first moment) is a generalization of the weighted average in probability theory. The anticipated value is the arithmetic mean of a large number of independently chosen random variable outcomes.
Let b be the number of days a busy student puts off doing laundry.
The given information is tabulated as below:
Days(b) 1 2
Probability, p(b) 2/3 1/3
The expected time is given as,
E(b)=∑i=1ᵇp(b)
=(1×(2/3))+(2×(1/3))
=4/3
=1.3333
Therefore, the value of ex(b):
1.3333 day ≈ 1 day
To know more about expected time, visit:
https://brainly.com/question/15362430
#SPJ4
Adult tickets to the fall play cost $8 and student tickets cost $4. The drama class sold 30 more adult tickets than student tickets to the fall play. If the class collected 840 from ticket sales, how many adult tickets were sold?
Answers
The number of adult tickets that were sold would be = 435 tickets.
What is a ticket?A ticket is an official document that gives an individual access to an event.
The cost of adult tickets = $8
The cost for student tickets = $4
The number of students tickets sold = X
The number of adults tickets sold = X +30
The told number of tickets sold = 840
To find X;
X + X + 30 = 840
2x + 30 = 840
2x = 840-30
2x = 810
X = 810/2
X = 405
Therefore, the number of tickets sold for adults = 405 +30 = 435 tickets.
Learn more about tickets here:
https://brainly.com/question/25333829
#SPJ1
Decorative pots for flowers in the shape of a regular four-sided prism with external dimensions a = 18 cm. h = 16 cm are made of concrete. The thickness of the walls is 3 cm. How many m³ of concrete is needed to make 80 of such pots, if 5% more concrete should be counted due to waste?
Answers
The amount of concrete required to make 80 pots is 0.24192 cubic meters after adding 5% of the required concrete.
Given that:External dimensions of the prism, a = 18 cmHeight of the prism, h = 16 cm Thickness of the walls, t = 3 cmNumber of pots, n = 80Wastage is 5% of the required concreteLet the side of the inner square be 'a1'. Then,a = a1 + 2t⇒ a1 = a - 2t = 18 - 2×3 = 12 cmVolume of the pot = volume of the outer prism - volume of the inner prismVolume of the outer prismVolume of the outer prism = a²hVolume of the outer prism = 18²×16 = 5184 cm³Volume of the inner prismVolume of the inner prism = a1²hVolume of the inner prism = 12²×16 = 2304 cm³Volume of the potVolume of the pot = 5184 - 2304 = 2880 cm³In m³, Volume of the pot = 2880/1000000 m³Volume of 80 such potsVolume of 80 pots = 80 × 2880/1000000 m³= 0.2304 m³Concrete required for the potsConcrete required = volume of 80 pots × (100 + 5)/100Concrete required = 0.2304 × 105/100 m³Concrete required = 0.24192 m³
for more such question on cubic meters
https://brainly.com/question/30106291
#SPJ8
find the sum of 23 and -23
Answers
The sum of a number and its opposite, or additive inverse, is always zero. Therefore, 23 added to its additive inverse, -23, equals zero.
When two numbers are added together, the result is their sum. In this case, we are adding 23 and -23.
The number 23 is a positive integer, and its opposite or additive inverse is the negative of 23, which is -23. When added to the original number, results in a sum of zero, because of the additive inverse of a number property.
So, when we add 23 to -23, we get:
23 + (-23) = 0
This is because adding a number and its additive inverse always results in a sum of zero.
In general, when we add any number to its additive inverse, we get a sum of zero. This is a fundamental property of addition in mathematics and is known as the additive inverse property.
For example, if we add 10 to its additive inverse, -10, we get:
10 + (-10) = 0
Likewise, if we add -7 to its additive inverse, 7, we get:
-7 + 7 = 0
In fact, we can add any number to its additive inverse and get a sum of zero. This property is used in many mathematical applications, such as solving equations and balancing chemical reactions.
Learn more about additive inverse property here:
https://brainly.com/question/2870955
#SPJ1
1 .Find the Product of Prime factors 1352.
2. Find the L.C.M of 10 and 12.
Answers
The Prime Factors of 1352 are: 2, 2, 2, 13, 13.
What is Prime factors?The breakdown of a composite number into a sum of smaller integers is known as integer factorization in number theory. By repeatedly dividing a number by prime factors until the remainder equals one, the simplest method for determining a number's prime factors is to keep going till we have the answer. For instance, when we factor a number by its prime factors, we obtain 30/2 = 15, 15/3 = 5, and 5/5 = 1. It cannot be further factored because we received the leftover money.The Prime Factor Tree's Prime Factor Tree only uses prime numbers that are prime factors of 1352. The math is illustrated below:352 ÷ 2 = 676
676 ÷ 2 = 338
338 ÷ 2 = 169
169 ÷ 13 = 13
13 ÷ 13 = 1
Again, all the prime numbers you used to divide above are the Prime Factors of 1352. Thus, the Prime Factors of 1352 are: 2, 2, 2, 13, 13.
To learn more about Prime factors refer to:
https://brainly.com/question/18187355
#SPJ1
please help!
please

Answers
Answer:
8
Step-by-step explanation:
the table shows the function f(x) = 2x²
Rate of Change: [f(1) - f(3)] / 1-3
= 2(1²) - 2(3²) / -2
= 2 - 18 / -2
= -16/-2
= 8
A four day camping trip costs 800$. The camper rental fee was 340$. Also included were four camper tickets. How much was each ticket?
Answers
Answer:
$115 each
Step-by-step explanation:
(800 - 340)/4 = 115
2
Drag the tiles to the correct boxes to complete the pairs. Not all tiles will be used.
Find the average rate of change of each function over the interval [0, 3]. Match each representation with its respective average rate of change.

Answers
The average rate of change of a function over the interval (0, 3) is: 5
How to find the average rate of change over an interval?We are given the quadratic function as:
r(x) = x² + 2x - 5
The formula for calculating the average rate of change of a function over an interval (a, b) is:
f'(x) = [f(b) - f(a)] / (b - a)
Applying that to our function over the interval (0, 3)
r(0) = 0² + 2(0) - 5 = -5
r(3) = 3² + 2(3) - 5
r(3) = 10
Thus:
r'(x) = (10 - (-5))/(3 - 0)
r'(x) = 15/3
r'(x) = 5
Read more about average rate of change at: https://brainly.com/question/17131025
#SPJ1
can you please answer the question?

Answers
The trigonometric expression \(\frac{\tan^{2} \alpha}{\tan \alpha - 1} + \frac{\cot^{2} \alpha}{\cot \alpha - 1}\) is equivalent to the trigonometric expression \(\sec \alpha \cdot \csc \alpha + 1\).
How to prove a trigonometric equivalence
In this problem we must prove that one side of the equality is equal to the expression of the other side, requiring the use of algebraic and trigonometric properties. Now we proceed to present the corresponding procedure:
\(\frac{\tan^{2} \alpha}{\tan \alpha - 1} + \frac{\cot^{2} \alpha}{\cot \alpha - 1}\)
\(\frac{\tan^{2}\alpha}{\tan \alpha - 1} + \frac{\frac{1}{\tan^{2}\alpha} }{\frac{1}{\tan \alpha} - 1 }\)
\(\frac{\tan^{2}\alpha}{\tan \alpha - 1} - \frac{\frac{1 }{\tan \alpha} }{\tan \alpha - 1}\)
\(\frac{\frac{\tan^{3}\alpha - 1}{\tan \alpha} }{\tan \alpha - 1}\)
\(\frac{\tan^{3}\alpha - 1}{\tan \alpha \cdot (\tan \alpha - 1)}\)
\(\frac{(\tan \alpha - 1)\cdot (\tan^{2} \alpha + \tan \alpha + 1)}{\tan \alpha\cdot (\tan \alpha - 1)}\)
\(\frac{\tan^{2}\alpha + \tan \alpha + 1}{\tan \alpha}\)
\(\tan \alpha + 1 + \cot \alpha\)
\(\frac{\sin \alpha}{\cos \alpha} + \frac{\cos \alpha}{\sin \alpha} + 1\)
\(\frac{\sin^{2}\alpha + \cos^{2}\alpha}{\cos \alpha \cdot \sin \alpha} + 1\)
\(\frac{1}{\cos \alpha \cdot \sin \alpha} + 1\)
\(\sec \alpha \cdot \csc \alpha + 1\)
The trigonometric expression \(\frac{\tan^{2} \alpha}{\tan \alpha - 1} + \frac{\cot^{2} \alpha}{\cot \alpha - 1}\) is equivalent to the trigonometric expression \(\sec \alpha \cdot \csc \alpha + 1\).
To learn more on trigonometric expressions: https://brainly.com/question/10083069
#SPJ1
the owner of a property has 2000 yd. of fencing to enclose a rectangular area situated along a straight portion of a river. if fencing is not required along the river, what are the dimensions of the largest area that can be enclosed?
Answers
We need to use the fact that a rectangle with a fixed Dimension will have the largest area when it is a square. Let's assume that the length of the rectangle runs parallel to the river, so we only need to fence the other three sides.
If the width of the rectangle is "x," then the length of the rectangle is (2000 - 2x) because we subtract the length of the two sides parallel to the river.
To find the area, we multiply the length by the width, so the area is A = x(2000 - 2x).
To find the value of x that maximizes the area, we need to take the derivative of A with respect to x and set it equal to zero:
A' = 2000 - 4x
2000 - 4x = 0
x = 500
So the width of the rectangle should be 500 yards, and the length should be (2000 - 2(500)) = 1000 yards.
Therefore, the dimensions of the largest area that can be enclosed with 2000 yards of fencing along a straight portion of the river are 500 yards by 1000 yards.
Takes into account the dimensions of the rectangle, the perimeter of the fencing, and the process for finding the maximum area.
To know more about Dimensions visit :
https://brainly.com/question/30997214
#SPJ11
Solve for b: 2b-9=d please I need help
Answers
Answer:
Short answer, we need a value for d, longer answer is \(b = \frac{d}{2} + 4.5\) (I think)
Step-by-step explanation:
[1] Find b:
2b-9=d
[2] First we need to get b alone:
- \(\frac{b-9}{2}\)=\(\frac{d}{2}\)
- \(b - 4.5 = \frac{d}{2}\)
[3] Then continue
- Add 4.5 to both sides
- \(b = \frac{d}{2} + 4.5\)
Darryl deposits $1,500 into a savings account that has a simple interest rate of 2.7%.
Lori deposits $1,400 into a savings account that has a simple interest rate of 3.8%.
If no other transactions are made, who will have more money in their account after 10 years? How much more step by step explanation please
Answers
Lori will make more than Darryl, then the after 10 years Lori will have make $27.00 more in their account.
Darryl deposits into a savings = $1,500
Account that has a simple interest rate = 2.7%.
Lori deposits into a savings = $1,400
Account that has a simple interest rate = 3.8%.
Let us assume,
\(P_{1}\) = $1,500
\(r_{1}\) = 2.7%.
\(r_{1}\) = 2.7/100
\(r_{1}\) = 0.027
After 10 years = t = 10years
The formula to calculate simple interest is,
\(A_{1}\) = \(P_{1} (1+r_{1} t)\) (Equation-1)
\(P_{1}\) = Principal amount
\(r_{1}\) = rate of interest ( in decimal )
t = time
First we calculate Darryl's deposit,
So we can substitute values in the equation-1,
\(A_{1}\) = 1,500(1 + 0.027×10)
\(A_{1}\) = 1,500 ( 1+0.27 )
\(A_{1}\) = 1,500 × 1.27
\(A_{1}\) = $1905
Now we will calculate Lori's deposit,
\(A_{2}\) = \(P_{2} (1+r_{2} t)\) (Equation-2)
\(P_{2}\) = $1,400
\(r_{2}\) = 3.8%.
\(r_{2}\) = 3.8/100
\(r_{2}\) = 0.038
So we can substitute values in the equation-2,
\(A_{2}\) = 1,400 ( 1 + 0.038 × 10 )
\(A_{2}\) = 1,400 ( 1 + 0.38 )
\(A_{2}\) = 1,400 × 1.38
\(A_{2}\) = $1,932
Darryl will make money after 10 years = $1905
Lori will make money after 10 years = $1,932
Hence,
Lori will make more than Darryl,
Then the difference will be = 1,932 - 1,905 = $27
Therefore,
After 10 years Lori will have make $27.00 more in their account.
To learn more about information visit Deposit problems :
brainly.com/question/11607089
#SPJ1
Question 7:
g is 60% of h
f is a third of g
Write the ratio f g h in its simplest form.
Answers
The ratio of f to g to h is:
1:3:5
How to write the ratio?
First, we know that g is the 60% of h, then we can write:
g = 0.6*h
Now we can write the 0.6 as a fraction, then 0.6 = 3/5.
g = (3/5)*h
So we can write:
5*g = 3*h
So the ratio of g to h is 3:5
Now, we know that f is a third of g:
f = (1/3)*g
3f = g
So the ratio of f to g is:
1:3
notice that the value that represents the proportion of g is 3 in both ratios, so we can just write:
The ratio of f to g to h is:
1:3:5
If you want to learn more about ratios:
https://brainly.com/question/2328454
#SPJ1
read the picture plsssssssssssss

Answers
If the expression be 23 x² + 3x + 8 then the constant exists 8.
What is meant by expression?The addition, subtraction, multiplication, and division arithmetic operators are used to write a group of numbers together to form a numerical statement in mathematics. The expression of a number can take on various forms, including verbal form and numerical form.
A mathematical expression is a finite combination of symbols that is well-formed in accordance with context-specific norms.
A mathematical expression is a phrase that includes at least two numbers or variables, at least one arithmetic operation, and the expression itself. Any one of the following mathematical operations can be used. A sentence has the following structure: Number/variable, Math Operator, Number/Variable is an expression.
During the course of a program's execution, a constant's value cannot change. As a result, the value is constant, as implied by its name. During the course of a program's execution, a variable's value can change. As a result, the value might change, as implied by its name.
Let the expression be 23 x² + 3x + 8
then the constant exists 8.
Therefore, the correct answer is option C. 8.
To learn more about expression refer to:
https://brainly.com/question/723406
#SPJ1
Figure A is a scale image of figure B.
Figure A
Figure B
16
Figure A maps to figure B with a scale factor of
4.
9
What is the value of 3?

Answers
Answer:
x = 36
Step-by-step explanation:
16 / (4/9) = 36
That's all there is to it. Hope I helped
the pithag tells us how the ____ lengths of ______ triangles are related
Answers
Pythagorean theorem tells us how the side lengths of right triangles are related.
The Pythagorean theorem, also known as the Pythagorean identity, states that the sum of the squares of the lengths of the two sides forming the right angle is equal to the square of the length of the hypotenuse in any right triangle (the side opposite the right angle). In other words, the Pythagorean theorem can be used to calculate the length of the hypotenuse given the lengths of the two sides of a right triangle.
This relationship can be written as the equation:
\(a^2 + b^2 = c^2\)
where a and b are the lengths of the legs, and c is the length of the hypotenuse. The Pythagorean Theorem is named after the ancient Greek mathematician Pythagoras, who is credited with its discovery.
For more question on Pythagorean theorem click on
https://brainly.com/question/343682
#SPJ4
Which of the following statements is false?
-(-5) = 5
|-5| = -5
-|-5| = -5
-|5| = -5
Answers
Answer:
i did the work its the third one
Step-by-step explanation: