Terry sees this offer.
Refurbished phone
35% OFF
Now only £78
How much was the phone before the discounted price?
Answers
Answer: The phone was £120 before the discount.
Related Questions
A scuba diver is 31 feet below the surface of the water. She dives down an additional 16 feet. How far would she have to rise to reach the surface of the water?
Answers
Answer: 47 feet
Step-by-step explanation:
Originally the scuba driver was 31 feet below the surface of the water.
She then dives down a further 16 feet. The depth below the surface is now:
= 31 + 16
= 47 feet
The Scuba driver is now 47 feet below the surface of the water so if she had to come back to the surface, she would have to rise that 47 feet to get to the surface.
Answer:
47 feet
Step-by-step explanation:
she is already 31 feet below and dives down 16 more so 31+16 equals 47.
A car salesperson earns a 18% commission on every car sold. The salesperson sells a car for $37,560. What is the commission?
Answers
100% = $37,560
18% = ?
(18 × 37560) ÷ 100
= 676,080 ÷ 100
= $6760.8 commission
for a perfectly symmetrical distribution with µ = 30, what is the mode?
Answers
In a perfectly symmetrical distribution with a mean (µ) of 30, there is no specific mode because all values occur with equal frequency.
In a perfectly symmetrical distribution, such as a symmetric bell-shaped curve or a uniform distribution, each value occurs with the same frequency. This means that there is no value that occurs more frequently than others, resulting in multiple modes or no mode at all.
The mode is typically used to identify the most common value in a dataset, but in a perfectly symmetrical distribution, all values have equal frequency, and there is no single mode. Instead, the distribution is characterized by its symmetry around the mean (µ). The mean represents the central tendency of the distribution, indicating the balance point of the data. In this case, with a mean of 30, the distribution is centered around this value, with equal numbers of observations on either side.
Learn more about mode here:
https://brainly.com/question/19435110
#SPJ11
Please help!!! thank you!

Answers
Answer:
30 again if I’m wrong then it’s 40
Step-by-step explanation:
most of the pizzas that are sold are in the 30’s or below
(-2,-4)
(-2,4)
(2,4)
(2, 4)

Answers
Answer:
(-2,-4) is in quadrant 3
(-2,4) is in quadrant 2
(2,4) is in quadrant 1
(2,-4) is in quadrant 4
Step-by-step explanation:
Quadrant 1 is Positive, Positive
Quadrant 2 is Negative, Positive
Quadrant 3 is Negative, Negative
Quadrant 4 is Positive, Negative
Feel free to ask if you have anymore questionsHope this helped :)Step-by-step explanation:
(-2,-4) in 3rd Quadrant
(-2,4) in 2nd Quadrant
(2,4) in 1st Quadrant
(2, -4) in 4th Quadrant
-TheUnknownScientist
!25 POINTS! (5 SIMPLE GEOMETRY QUESTIONS)
QUSTIONS BELOW
|
|
\/

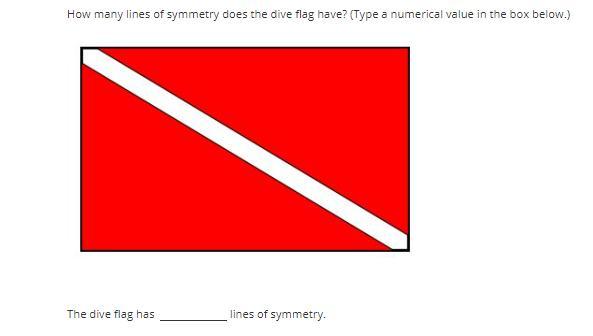


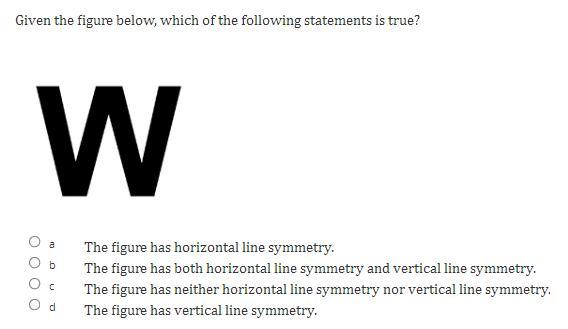
Answers
Answer:
1. The x-axis
2. No line of symmetry
3. 1 horizontal line of symmetry
4. y = -2
5. The figure has vertical line symmetry.
Step-by-step explanation:
A line of symmetry is a line that divides a shape into two parts that match exactly
1.
We can see that if we cut through point A we will be able to divide the shape into two equal parts.
So, the answer is the x-axis.
2.
The Flag has no line symmetry. It only has point symmetry about its center.
3.
1 horizontal line of symmetry
4.
We can see that if we cut through point R we will be able to divide the shape into two equal parts.
So, the answer is y = -2.
5.
The figure has vertical line symmetry.
when computing the correlation​ coefficient, what is the effect of changing the order of the variables on​ r?
Answers
The correlation coefficient (r) measures the strength and direction of the linear relationship between two variables. The value of r ranges from -1 to +1, where a value of -1 indicates a perfect negative correlation, +1 indicates a perfect positive correlation, and 0 indicates no correlation.
The correlation coefficient's sign will not change when the order of the variables in a correlation study is changed, but the coefficient's numerical value might. This is due to the symmetry of the correlation coefficient with regard to the two variables under comparison.
For instance, if you calculate the correlation coefficient between X and Y and the result is r = 0.7, it indicates that Y tends to rise as X does. The correlation coefficient between the variables Y and X will have the same sign (+ or -) but a different numerical value if the variables are computed in a different order.
For such more question on coefficient:
https://brainly.com/question/4219149
#SPJ4
Is 0.007 10 times the value of 0.07
Answers
The statement that 0.007 is 10 times the value of 0.07 is incorrect. Instead, 0.007 is 1/10th or one-tenth the value of 0.07.
To understand this, let's examine the decimal places in each number. In 0.007, the number is in the thousandths place, which means it represents 7/1000. On the other hand, in 0.07, the number is in the hundredths place, representing 7/100.
To compare the two numbers, we can write them as fractions:
0.007 = 7/1000
0.07 = 7/100
Now, let's calculate the value of 0.007 compared to 0.07:
0.007 = (7/1000) / (7/100)
= (7/1000) * (100/7)
= 1/100
So, 0.007 is equal to 1/100 or 0.01, which is 1/10th or one-tenth the value of 0.07.
No, 0.007 is not 10 times the value of 0.07. In fact, 0.007 is 1/10th or one-tenth the value of 0.07.
For more such questions on value visit:
https://brainly.com/question/24877689
#SPJ8
The area is square feet of a rectangular garden can be expressed as the product of the garden's length and
width, or A(x) = 2x2 - 21x + 40. If the width of the garden is (x - 8) feet, what is the length of the garden?
Answers
Answer:
(2x-5)feet
Step-by-step explanation:
The area of the rectangular garden A = Length × Width
Given the area A(x) = 2x² - 21x + 40
And the width =x-8 feet
To get the length, we will simply factorise the area function as shown;
You will just factorise the area
Factorizing 2x² - 21x+40
2x² − 21x + 40
2x² -16x - 5x + 40
2x(x - 8) -5(x - 8)
=(2x−5)(x−8)
Hence the length of the garden is
(2x-5)feet
Give an example of a vector such that together with forms a basis of.
Answers
we can solve the equation using the inverse of A:\(\[x = A^{-1}v = \begin{bmatrix} -1 & 2 & -5 \\ 5 & -9 & 20 \\ -1 & 2 & -4 \end{bmatrix} \begin{bmatrix} 1 \\ 1 \\ 1 \end{bmatrix} = \begin{bmatrix} -4 \\ 16 \\ -3 \end{bmatrix}\]Therefore, the four vectors:\[u_1 = \begin{bmatrix} 1 \\ -1 \\ 2 \end{bmatrix} , \; u_2 = \begin{bmatrix} 2 \\ 1 \\ 0 \end{bmatrix} , \; u_3 = \begin{bmatrix} -1 \\ 3 \\ -1 \end{bmatrix} , \; v = \begin{bmatrix} -4 \\ 16 \\ -3 \end{bmatrix}\]\)form a basis of .
To do that, we will solve the equation:\[Ax = v\]where x is a column vector of three unknowns. We want v to be linearly independent from the columns of A, so we need the equation to have a unique solution. We can achieve that by taking v to be any vector that is not in the column space of A. For example, we can take:\\([v = \begin{bmatrix} 1 \\ 1 \\ 1 \end{bmatrix}\]\)Then, we can solve the equation using the inverse of A:\(\[x = A^{-1}v = \begin{bmatrix} -1 & 2 & -5 \\ 5 & -9 & 20 \\ -1 & 2 & -4 \end{bmatrix} \begin{bmatrix} 1 \\ 1 \\ 1 \end{bmatrix} = \begin{bmatrix} -4 \\ 16 \\ -3 \end{bmatrix}\]Therefore, the four vectors:\[u_1 = \begin{bmatrix} 1 \\ -1 \\ 2 \end{bmatrix} , \; u_2 = \begin{bmatrix} 2 \\ 1 \\ 0 \end{bmatrix} , \; u_3 = \begin{bmatrix} -1 \\ 3 \\ -1 \end{bmatrix} , \; v = \begin{bmatrix} -4 \\ 16 \\ -3 \end{bmatrix}\]\)form basis of .
learn more about equation here;
https://brainly.com/question/23863621
#SPJ11
please help meeeeeeee <33

Answers
9 times 3 is 27 add 7 to that and you get 34
Answer:
f(5) = 22; f(9) = 34
Step-by-step explanation:
f(5) = 3(5) +7
f(5) = 22
f(9) = 3(9) +7
f(9) = 34
can someone answer both of these question and show work for both of them
question 15. You are sending a package in the mail. The box is a cube measuring 14 inches on all sides. How many cubic inches is the package.
question 16. You sent an email to 8 friends. They each forwarded the email to 8 friends, and those friends each forwarded the email to 8 friends. The chain of emails is represented by the expression \(8+8^{2}+8^{3}\). How many people were sent your email.
i will give brainliest if you answer both and show work for both the 2 question are in the picture also

Answers
Answer:
15) 2744 in³16) 584 peopleStep-by-step explanation:
Question 15Find the volume of the cube
V = a³ = (14 in)³ = 2744 in³Question 16Find the value of the expression
8 + 8² + 8³ = 8 + 64 + 512 = 584Answer:
15) 2744 in³
16) 584
Step-by-step explanation:
Question 15Volume of a cube
\(\sf V=s^3\)
(where s is the side length)
Given:
s = 14 inSubstitute the given value into the formula and solve for V:
\(\implies \sf V=14^3\)
\(\implies \sf V=14 \cdot 14 \cdot 14\)
\(\implies \sf V=196 \cdot 14\)
\(\implies \sf V=2744\:\:in^3\)
Question 16Given expression:
\(\sf 8 + 8^2+8^3\)To solve:
\(=\sf 8 + (8 \cdot 8) + (8 \cdot 8 \cdot 8)\)
\(\sf = 8 + 64 + 512\)
\(\sf = 72+512\)
\(= \sf 584\)
Therefore, 584 people were sent your email.
27) Suppose the price elasticity of supply for shampoo is 20. If the price of shampoo increases by 0.7%, what would we expect to happen to the quantity of shampoo supplied?
a) Increase by 27%
b) Increase by 14%)
e) Increase by 13%
d) Decrease by 13%
28)
e) Decrease by 27%
If pasta is a Giffen good, then....
a) pasta is also a normal good.
b) pasta is also a luxury good.
e) an decrease in the price of pasta will increase the quantity demanded. d) an increase in the price of pasta will increase the quantity demanded. e) pasta must make up a small portion of consumers' total expenditures.
20)
An inferior good in which the income effect dominates the substitution effect is called....
a) a normal good.
b) a luxury good.
30)
a) a Giffon good.
d) a mass-produced good.
e) a favored good.
The cross elasticity of demand measures the responsiveness of the quantity demanded of a particular good to changes in the prices of
a) its complements but not its substitutes.
b) Its substitutes but not ita complements.
c) its substitutes and its complements.
d) neither its substitutes nor its complements. e) None of the above..
Answers
In question 27, the price elasticity of supply for shampoo is given as 20, and the price of shampoo increases by 0.7%. The expected change in the quantity of shampoo supplied can be determined using the concept of price elasticity of supply. However, the specific percentage change in quantity supplied is not provided, so a precise answer cannot be given based on the given information.
In question 20, an inferior good in which the income effect dominates the substitution effect is referred to as a Giffen good. It is not classified as a normal good, luxury good, mass-produced good, or favored good.
In question 30, the cross elasticity of demand measures the responsiveness of the quantity demanded of a particular good to changes in the prices of its substitutes and complements. The correct answer is that the cross elasticity of demand measures the responsiveness to changes in both substitutes and complements.
In question 27, without the specific percentage change in quantity supplied, we cannot determine the exact outcome based on the given information. The price elasticity of supply of 20 suggests that the quantity supplied is highly responsive to changes in price, but the specific percentage change in quantity supplied cannot be calculated without additional data.
In question 28, the relationship between pasta being a Giffen good and other characteristics is not specified. While pasta being a Giffen good indicates that the quantity demanded increases as the price increases, it does not imply whether pasta is a normal good, luxury good, or how price changes affect quantity demanded.
To learn more about mass-produced good: -
2.2kg= measurment in lb?
Answers
Answer:
4.85100 lb
Step-by-step explanation:
1 kg = 2.205 lb
2.2 kg
= 2.2 x 2.205
= 4.85100 lb
Frankie says that it
costs $12.50 to make a
wreath.
The table below shows the cost to
make a wreath. How much does it
Sost to make one wreath?
3
5
WREATH, w
COST, a
7
$87.50
9
$112.50
$37.50
$62.50
Fiona says that it costs
$13.50 to make a
wreath.

Answers
Answer:
Frainkie is correct it takes $12.50
Step-by-step explanation:
37.50 divided by 3 to find out one wreath's cost which is 12.50
hope this helps
plz mark me brainliest.
(ó﹏ò。) hey uh- a lil' help here pls
As a young girl, Carmen enjoyed watching her father make things in his woodworking shop. Now that she's grown up, Carmen builds wooden furniture for a living. Her most popular product is a cedar porch swing.
There is a proportional relationship between the time (in days) Carmen spends making porch swings, x, and the number of swings she builds, y.
x (days) y (swings)
12 6
16 8
18 9
20 10
What is the constant of proportionality? Write your answer as a whole number or decimal.
swings per day
Answers
Answer:
Try using slope formula
Step-by-step explanation:
You can use the formula of m= ((y2)-(y1)/((x2)-(x1))
I hope this helps
Well, it's really simple actually. You don't need to focus on all those extra words on the top, focus on days and swings
In 20 days, Carmen swings 10 times.
In 10 days, Carmen swings 5 times.
If you notice, Y is multipled 0.5 of the original, so half
This means, 1 day (X) is swung 0.5 (Y)
I think Carmen is a possesed little girl for pulling that off
If you have any questions, feedback, or problems, let me know
(1 point) Let \( z=3 e^{x^{3} y^{2}} \) \( \frac{\partial z}{\partial x} \) \( \frac{\partial z}{\partial y} \)
(1 point) Let \( z=-\frac{x y}{4 x^{2}+2 y^{2}} \) \( \frac{\partial}{\partial} \) \( \
Answers
For the first question:
\(\(\frac{\partial z}{\partial x} = 3x^2y^2e^{x^3 y^2}\) and \(\frac{\partial z}{\partial y} = 3x^3 y e^{x^3 y^2}\).\)
For the second question:
\(\(\frac{\partial z}{\partial x} = \frac{-2y^3}{(4x^2 + 2y^2)^2}\) and \(\frac{\partial z}{\partial y} = \frac{-4x^3}{(4x^2 + 2y^2)^2}\).\)
For the first question:
Given \(\(z = 3e^{x^3 y^2}\),\) we need to find \(\(\frac{\partial z}{\partial x}\) and \(\frac{\partial z}{\partial y}\).\)
Using the chain rule, we have:
\(\(\frac{\partial z}{\partial x} = \frac{\partial}{\partial x}(3e^{x^3 y^2}) = 3y^2 e^{x^3 y^2} \cdot \frac{\partial}{\partial x}(x^3) = 3x^2y^2e^{x^3 y^2}\)\)
Similarly,
\(\(\frac{\partial z}{\partial y} = \frac{\partial}{\partial y}(3e^{x^3 y^2}) = 3x^3 y e^{x^3 y^2}\)\)
Therefore, \(\(\frac{\partial z}{\partial x} = 3x^2y^2e^{x^3 y^2}\) and \(\frac{\partial z}{\partial y} = 3x^3 y e^{x^3 y^2}\).\)
For the second question:
Given \(\(z = -\frac{xy}{4x^2 + 2y^2}\), we need to find \(\frac{\partial z}{\partial x}\) and \(\frac{\partial z}{\partial y}\).\)
To find the partial derivative with respect to \(x\), we differentiate \(\(z\)\)with respect to \(\(x\)\) while treating \(\(y\)\) as a constant:
\(\(\frac{\partial z}{\partial x} = -\frac{\partial}{\partial x}\left(\frac{xy}{4x^2 + 2y^2}\right) = -\frac{y(4x^2 + 2y^2) - x(8x)}{(4x^2 + 2y^2)^2} = \frac{-2y^3}{(4x^2 + 2y^2)^2}\)\)
To find the partial derivative with respect to \(\(y\)\), we differentiate \(\(z\)\) with respect to\(\(y\)\) while treating\(\(x\)\) as a constant:
\(\(\frac{\partial z}{\partial y} = -\frac{\partial}{\partial y}\left(\frac{xy}{4x^2 + 2y^2}\right) = -\frac{x(4x^2 + 2y^2) - y(4y)}{(4x^2 + 2y^2)^2} = \frac{-4x^3}{(4x^2 + 2y^2)^2}\)\)
\(Therefore, \(\frac{\partial z}{\partial x} = \frac{-2y^3}{(4x^2 + 2y^2)^2}\) and \(\frac{\partial z}{\partial y} = \frac{-4x^3}{(4x^2 + 2y^2)^2}\).\)
To know more about chain rule refer here
https://brainly.com/question/48504877#
#SPJ11
a tour bus normally leaves for its destination at 5 : 00 p.m. for a 350 mile trip. this week however, the bus leaves at 6 : 10 p.m. to arrive on time, the driver drives 10 miles per hour faster than usual. what is the bus` usual speed? the bus' usual speed is miles an hour.
Answers
The usual speed of the bus is 60 km/hr.
Define speed.Speed is the rate of change in location of an item, expressed in meters per second.
Given,
Let x be the usual speed.
Speed = Distance/ Time
Time = Distance/Speed
Bus usually travels 350 miles.
It takes time for it to get there = 350/x h
The bus departs this week at 6:10, though.
The 70-minute bus delay = 70/60 = 7/6 h
The bus's current speed is (x+10) miles per hour.
The new window of time for getting there is \(\frac{350}{x+10}\)
\(\frac{350}{x}\) + \(\frac{350}{x+10}\) = \(\frac{7}{6}\)
350(\(\frac{x+10-x}{x(x+10)}\) ) = \(\frac{7}{6}\)
350 ( \(\frac{10}{x^{2} +10x}\) )= \(\frac{7}{6}\)
7(x² +10x) = 6(350)(10)
x² + 10x = 3000
x² + 10x - 3000 = 0
x² -60x + 50x - 3000 = 0
x( x - 60) + 50(x - 60) = 0
(x + 50)(x - 60) = 0
x = -50 , x = 60
The usual speed of the bus is 60 km/hr.
To learn more about speed, visit:
https://brainly.com/question/15203844
#SPJ4
Find the mean of the following numbers.
14, 17, 21, 28, 40
Answers
14 + 17 + 21 + 28 +40 / 5
120 / 5
24
The curved surface area of a cylinder is twice the area of base and the sum of radius and height is 28 cm find the volume of the cylinder
Answers
The volume of Cylinder is 8624 \(cm^{2}\) and the radius and height of cylinder is 14 cm as per the given question.
Curved surface area of cylinder =2 x(area of the base) [as stated in the question]----Eq 1
Sum of radius and height = 28cm [r +h=28cm]-----Eq A
Curved surface area of cylinder = \(2\pi rh\)----Eq2
Area of base = \(\pi r^{2}\)---Eq3
Putting Eq 2 and 3 in Eq 1
\(2\pi rh\)=\(2\pi r^{2}\)
\(h=r\)
Thus from this we get that the height and radius of cylinder is equal.
Now Using Equation A
r + h =28cm
2r =28 cm
r=14 cm = h
so the height and radius of cylinder is 14 cm.
Volume of Cylinder = \(\pi r^{2} h\)
=22/7 x 14x14x14
=22 x 2 x14x 14
=8624 \(cm^{2}\)
You can find more about Volume:
https://brainly.com/question/6204273
#SPJ9
In ΔCDE, the measure of ∠E=90°, DE = 84 feet, and EC = 13 feet. Find the measure of ∠C to the nearest tenth of a degree.
Answers
Answer: 81.2
Step-by-step explanation:
Determine whether VY | ZW . Justify your answer.
WX=31,YX=21, , and Z X=4 Z V
Answers
The relationship between variables VY and ZW cannot be determined based on the given information.
In order to determine the relationship between VY and ZW, we need to have direct information about their relationship or some indirect information that can be used to establish a connection between them. However, the provided information only includes the values of WX, YX, and ZX. These values alone do not provide any direct or indirect evidence of the relationship between VY and ZW.
To establish a relationship between VY and ZW, we would need either the values of VY and ZW themselves or additional information that can be used to infer their relationship. Without any further data or context, it is not possible to determine whether VY and ZW are related or not. Therefore, based on the given information, we cannot make any conclusions about the relationship between VY and ZW.
learn more about variables -
https://brainly.com/question/33216668
#SPJ11
$55 is what percent of $55?
Answers
100%
Step-by-step explanation:$55 = $55. Or in other terms:
100% = 100%
$55 is the maximum number of the problem, which would be 100%. So, they are asking what the maximum is. Which is 100%.
Solution:100%
I hope my answer helped you! If you need more information or help, comment down below and I will be sure to respond if I am online. Have a wonderful rest of your day!
Answer: %100
Step-by-step explanation:
55 is the number you are using and the highest number is 55 so it will be %100
Suppose that 3 ≤ f '(x) ≤ 5 for all values of x. What are the minimum and maximum possible values of f(7) − f(3)? ____Your answer is incorrect. ≤ f(7) − f(3) ≤___
Answers
Using the Mean Value Theorem to find values for the inequality below, we get : Answer: 12 ≤ f(2) - f(-2) ≤ 20
By the Mean Value Theorem, if a function f(x) is continuous on the closed interval [a, b] and differentiable on the open interval (a, b), then there exists at least one value c in the interval (a, b) such that:
\(\[f'(c) = \frac{f(b) - f(a)}{b - a}\]\)
In this case, we are given that 3 ≤ f'(x) ≤ 5 for all values of x.
Let's apply the Mean Value Theorem to the interval [-2, 2]. Since f(x) is continuous on [-2, 2] and differentiable on (-2, 2), there exists a value c in (-2, 2) such that:
\(\[f'(c) = \frac{f(2) - f(-2)}{2 - (-2)} = \frac{f(2) - f(-2)}{4}\]\)
Given that 3 ≤ f'(x) ≤ 5 for all values of x, we can rewrite this inequality using the value of c:
3 ≤ f'(c) ≤ 5
Multiplying both sides of the inequality by 4, we get:
12 ≤ 4f'(c) ≤ 20
Since f'(c) = (f(2) - f(-2))/4, we can substitute this expression back into the inequality:
\(\[12 \leq 4 \cdot \frac{f(2) - f(-2)}{4} \leq 20\]\)
Simplifying, we have:
12 ≤ f(2) - f(-2) ≤ 20
Therefore, the values for the inequality are:
12 ≤ f(2) - f(-2) ≤ 20
To know more about the Mean Value Theorem refer here,
https://brainly.com/question/29145312#
#SPJ11
Complete question :
Suppose that 3≤f′(x)≤5 for all values of x. Use the Mean Value Theorem to find values for the inequality below.
Answer:_______ ≤f(2)−f(−2)≤ ________
A right rectangular prism has a length of 12 ft, width of 5 1/2 ft, and height of 10 1/2 ft. What is the volume of the prism? Enter the answer in the box.
Answers
Answer: The answer is: 693 ft^3 or 693ft cubed
What is the domain of √ 1 x²?
Answers
A domain has [-1, 1]
Now, According to the question:
Let's know:
How do you find the domain?
Let y = f(x) be a function with an independent variable x and a dependent variable y. If a function f provides a way to successfully produce a single value y using for that purpose a value for x then that chosen x-value is said to belong to the domain of f.
A square root with a negative number under the root cannot produce a real number. This means that \(1-x^2\) must be greater than or equal to zero.
Squaring a real number will always produce a positive number, so \(x^{2}\)must be equal to or smaller than 1. This means x is between 0 and 1, giving the domain of [0,1].
Learn more about Domain at:
https://brainly.com/question/28135761
#SPJ4
The complete question is this ;
What is the domain of \(\sqrt{1-x^2}\)
Which equation represents the slope-intercept form of the line below

Answers
An equation represents the slope-intercept form of the given line is y= 1/2 x+8. Therefore, option D is the correct answer.
Given that, y-intercept = (0, 8) and slope = 1/2.
The general equation of a straight line is y=mx+c, where m is the gradient, and y = c is the value where the line cuts the y-axis. This number c is called the intercept on the y-axis.
Substitute m=1/2 and c=8 in y=mx+c, we get
y= 1/2 x+8
Therefore, option D is the correct answer.
To learn more about the equation of a line visit:
https://brainly.com/question/2564656.
#SPJ1
PLEASE HELP ME. I NEED TO PRESENT THIS QUESTION IN CLASS TMRW!!

Answers
1. Given that AD is perpendicular to BC, this tells us angle ADC and ADB are both right angles and are therefore congruent.
2. We know AD = AD, since it's the same line segment.
3. Since AD bisects angle BAC, we know angle CAD and BAD are congruent.
Based on Angle-Side-Angle, triangles ADC and ADB are congruent.
Please help if you can
39n +1 = -49
Answers
Answer:
Step-by-step explanation:
39n = -49-1
39n = -50
n = - 1.28
Hey there!
39n + 1 = -49
39n = -49 - 1
39n = -50
n = -50 ÷ 39
n = -1.28
Hope it helps ya!
What is the answer to this?

Answers
Answer:
Y = 28.31
Z = 13.29
Angle A = 28
Step-by-step explanation:
For Angle A:
Remember a triangle has a total of 180 degree angles. So set up your formula as
180 - 62 -90 = Angle A
For Y:
The sine of an angle is equal to the ratio of the opposite side to the hypotenuse.
sin (B) = opp/hyp or b/c or (y)
sinB = 62
b = 25
Rewrite as c=b/2SinB -> c=25/sin62 -> Y = 28.31
For Z:
Use the Pythagorean theorem to find the unknown side.
\(z^{2} = 28.31^{2} - 25^{2}\)
z = 13.29