Suppose we have 5 colors, without replacement, from 14 distinct colors.1. If the order is not taken into consideration how many ways can this be done?2. If the order is taken into consideration how many ways?
Answers
Answer:
1) 240,240 ways
2) 2,002 ways
Explanation:
Here, we want to calculate the number of ways we can make a selection:
1. With the order not taken into consideration, we use the permutation formula as follows:
\(^nP_r=\frac{n!}{(n-r)!}_{}\)With respect to the given question, n is 14 and 5 is r
Thus, we have it as:
\(^{14}P_5\text{ = }\frac{14!}{(14-5)!}\text{ = }\frac{14!}{9!}\text{ = 240,240 ways}\)2. With the order taken into consideration, we use the combination formula as follows:
\(^nC_r\text{ = }\frac{n!}{(n-r)!r!}\)Thus, we have it as:
\(^{14}C_{5\text{ }}=\text{ }\frac{14!}{(14-5)!5!}\text{ = }\frac{14!}{9!5!}\text{ = 2,002 ways}\)Related Questions
I need help on question 3

Answers
Vector v is multiplied by a scalar resulting in u. What can be the interval that contains the scalar? less than -1 between -1 and 0 between 0 and 1 greater than 1

Answers
The interval that contains the scalar for this problem is given as follows:
Between -1 and 0.
How to multiply a vector of the scalar?When a vector is multiplied by a scalar, each component of the vector is multiplied by the scalar.
For this problem, we have that the direction of the vector u changed when it was multiplied by the scalar, hence the scalar is a negative number.
As vector v is smaller than vector u, the scalar has an absolute value that is less than 1, hence the number is between -1 and 0.
More can be learned about vectors at https://brainly.com/question/25705666
#SPJ1
plzzzzzzzz help for a brainly

Answers
Answer:
(3×3-5)³-19
(9-5)³-19
4³-19
64-19
=45
what is the property of 3x(5x7)=(3x5)7
Answers
The property you are referring to is called the associative property of multiplication. According to this property, when multiplying three numbers, the grouping of the numbers does not affect the result. In other words, you can change the grouping of the factors without changing the product.
In the equation you provided: 3x(5x7) = (3x5)7
The associative property allows us to group the factors in different ways without changing the result. So, whether we multiply 5 and 7 first, or multiply 3 and 5 first, the final product will be the same.
Use the law of detachment to determine what you can conclude from the given information

Answers
In mathematical logic, the Law of Detachment says that if the following two statements are true:
( 1 ) If p, then q.
( 2 ) p
Then we can derive a third true statement:
( 3 ) q.
In our question, we have
( 1 ) If 0º< A <90º, then A is an acute angle.
( 2 ) The measure of A is 58º.
Then, from the first statement, we can affirm
( 3 ) A is an acute angle.
Find the perimeter of ABC

Answers
Answer:
36 ft
Step-by-step explanation:
\(\triangle ABC\sim\triangle DEF\) (Given)Perimeters and corresponding sides of similar triangles are in proportion\(\implies\frac{P( \triangle ABC)}{P(\triangle DEF)}=\frac{BC}{EF}\) \(\implies\frac{P( \triangle ABC)}{12}=\frac{15}{5}\) \(\implies\frac{P( \triangle ABC)}{12}=3\) \(\implies P( \triangle ABC) =12(3)\) \(\implies P( \triangle ABC) =36 \: ft\)Simplify this expression: 3(2p + 1)
Answers
Answer:
6p + 3
General Formulas and Concepts:
Pre-Algebra
Distributive PropertyStep-by-step explanation:
Step 1: Define expression
3(2p + 1)
Step 2: Expand
Distribute 3: 6p + 3Explanation:
3 x 2p = 6
3 x 1 = 3
John bought a shirt at ksh 500 and marked it at Ksh 600. A customer bought it at ksh 550. What was the percentage discount?
Answers
Answer:
The original price of the shirt was Ksh 500 and it was sold for Ksh 550.
The discount is the difference between the original price and the selling price, which is Ksh 500 - Ksh 550 = Ksh -50.
To find the percentage discount, we can use the formula:
percentage discount = (discount ÷ original price) × 100%
percentage discount = (-50 ÷ 500) × 100%
percentage discount = -10%
Therefore, the percentage discount is 10%.
write v as a linear combination of u1, u2, and u3, if possible. (enter your answer in terms of u1, u2, and u3. if not possible, enter impossible.
Answers
The v can be written as a linear combination of u1, u2, and u3 as:
v = -(1/9)u1 + (5/3)u2 - (4/3)u3
To find the linear combination of u1, u2, and u3 that equals v, we can use the method of solving a system of linear equations.
The equation for v in terms of u1, u2, and u3 is:
v = a1u1 + a2u2 + a3u3
where a1, a2, and a3 are the coefficients of the linear combination.
Plugging in the given values for v, u1, u2, and u3:
(-1,7,2) = a1(3,2,8) + a2(1,-3,-1) + a3(-2,1,-3)
We can solve this system of equations by equating the corresponding entries of the two vectors:
-1 = 3a1 + a2 - 2a3
7 = 2a1 - 3a2 + a3
2 = 8a1 - a2 - 3a3
We can then use any method to solve the system of equations, such as substitution, elimination or Cramer's rule.
By solving the system of equations we get:
a1 = -(1/9), a2 = (5/3), a3 = -(4/3)
Therefore, v can be written as a linear combination of u1, u2, and u3 as:
v = -(1/9)u1 + (5/3)u2 - (4/3)u3
It is possible to write v as a linear combination of u1, u2, and u3.
For more questions like Linear combination click the link below:
https://brainly.com/question/30341410
#SPJ4

can someone please help me solve the problem

Answers
Given:
The exponential function is:
\(y=630(0.94)^x\)
To find:
The percentage rate of increase or decrease.
Solution:
The general exponential function is:
\(y=a(1+r)^x\) ...(i)
Where, a is the initial value, |r| is the growth rate in decimals if r>0 and |r| is the decay rate in decimal if r<0.
The exponential function is:
\(y=630(0.94)^x\)
It can be written as:
\(y=630(1-0.06)^x\)
\(y=630(1+(-0.06))^x\) ...(ii)
On comparing (i) and (ii), we get
\(r=-0.06\)
Since r is negative, therefore the given function is a decreasing or decay function.
Now,
\(|r|=|-0.06|\)
\(|r|=0.06\)
So, the rate of decrease is 0.06. Multiply this number by 100 to get the percentage.
\(0.06\times 100=6\%\)
Therefore, the rate of decrease is 6%.
Two identical lines are graphed below. How many solutions are there to the
system of equations?
5
A. Infinitely many
B. Zero
C. One
D. Two

Answers
Two identical lines have, A. Infinitely many solutions.
What are the three types of solutions for a system of linear equations?If a system of equations only contains two linear equations with two variables,
The system's equation can be graphed, the graph will have two straight lines, and the intersection point(s) of those lines will be the system's solution.
There are only three matching forms of solution for a given system of equations because there are only three different ways that two straight lines in the plane can graph.
Given are two identical lines,
Now we know the solution of two lines is where they intersect.
We also know that a line is made up of infinite points, So if two lines are identical every point of one line lies with every other point of the other line.
Therefore they have an infinite number of solutions.
learn more about types of solutions system of linear equations here :
https://brainly.com/question/19549073
#SPJ7
4c=3 solve for c please
Answers
Step-by-step explanation:
Given equation is:-
4c=3Dividing both sides by 4, we get
4c/4=3/4By doing simply, we get
c=¾Hence, the value of c will be ¾.
Which of the following expressions is equivalent to……

Answers
Answer:
Box 4
Step-by-step explanation:
negative divided by negative is positive so both negatives cancel out and u get (5/6) / (1/3)
maths question, been stuck on for ages
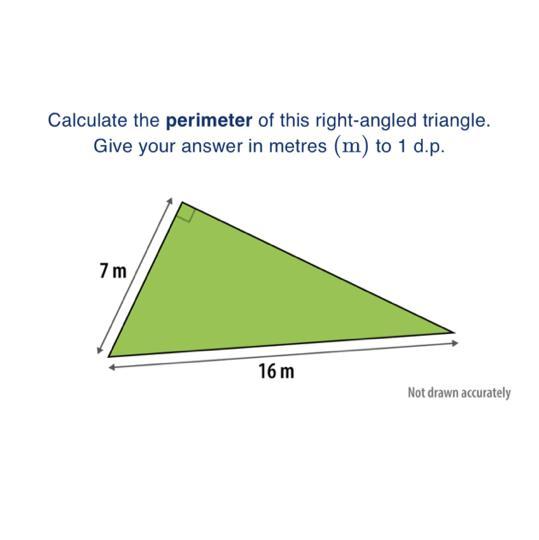
Answers
Answer:
14.4
Step-by-step explanation:
1) 16^2-7^2=207
2) square root of 207 is 14.4 (to 1 decimal place).
For a géometric sequence, if a1=9 and a5
=2304, what is the value of r?
Answers
The value of common ration r is 4.
Given that,
a₁ = 9
a₅ = 2304
We know that,
A geometric sequence is a sequence in which each term (except from the first term) is multiplied by a fixed amount to obtain the following term.
The following term in the geometric sequence must be obtained by multiplying it by a set term (referred to as the common ratio), and the previous term in the sequence can be obtained by dividing it by the same common ratio.
Since we know that nth term of GP is,
\(a_{n}\) = a₁ \(r^{n-1}\)
Therefore,
a₅ = a₁ \(r^{4}\) = 2304
⇒ 9 x \(r^{4}\) = 2304
⇒ \(r^{4}\) = 256
⇒ r = 4
Hence, r = 4
To learn more about GP visit:
https://brainly.com/question/29323941
#SPJ1
The histogram represents the time it takes employees to get to work each morning.
Which statement correctly describes the data?
The data is skewed left because the time it takes employees to get to work tails off to the left.
The data is skewed left because the time it takes employees to get to work tails off to the right.
The data is skewed right because the time it takes employees to get to work tails off to the right.
The data is skewed right because the time it takes employees to get to work tails off to the left.
Answers
Answer:the answer is B ,
Step-by-step explanation: just took the test .
Triangles Q R S and X Y Z are shown. Angles Q S R and X Z Y are right angles. Angles Q R S and X Y Z are congruent. The length of Y Z is 9, the length of X Z is 12, and the length of hypotenuse X Y is 15.
Given △QRS ~ △XYZ, what is the value of tan(Q)?
Three-fifths
Three-fourths
Four-fifths
Answers
Answer:three-fourths
Step-by-step explanation:
because my dad said it was right
A fence 6 feet tall runs parallel to a tall building at a distance of 6 feet from the building. We want to find the the length of the shortest ladder that will reach from the ground over the fence to the wall of the building. Here are some hints for finding a solution: Use the angle that the ladder makes with the ground to define the position of the ladder and draw a picture of the ladder leaning against the wall of the building and just touching the top of the fence. If the ladder makes an angle 0.82 radians with the ground, touches the top of the fence and just reaches the wall, calculate the distance along the ladder from the ground to the top of the fence. equation editorEquation Editor The distance along the ladder from the top of the fence to the wall is equation editorEquation Editor Using these hints write a function L(x) which gives the total length of a ladder which touches the ground at an angle x, touches the top of the fence and just reaches the wall. L(x) = equation editorEquation Editor . Use this function to find the length of the shortest ladder which will clear the fence. The length of the shortest ladder is equation editorEquation Editor feet.
Answers
Answer:
12√2 feet ≈ 16.97 feet
Step-by-step explanation:
For the dimensions shown in the attached diagram, the distance "a" along the ladder from the ground to the fence is ...
a = (6 ft)/sin(x) = (6 ft)/sin(0.82) ≈ 8.206 ft
The distance along the ladder from the top of the fence to the wall is ...
b = (6 ft)/cos(x) = (6 ft)/cos(0.82) ≈ 8.795 ft
__
In general, the distance along the ladder from the ground to the wall is ...
L(x) = a +b
L(x) = 6/sin(x) +6/cos(x)
This distance will be shortest for the case where the derivative with respect to x is zero.
L'(x) = 6(-cos(x)/sin(x)² +sin(x)/cos(x)²) = 6(sin(x)³ -cos(x)³)/(sin(x)²cos(x²))
This will be zero when the numerator is zero:
0 = 6(sin(x) -cos(x))(1 -sin(x)cos(x))
The last factor is always positive, so the solution here is ...
sin(x) = cos(x) ⇒ x = π/4
And the length of the shortest ladder is ...
L(π/4) = 6√2 + 6√2
L(π/4) = 12√2 . . . . feet
_____
The ladder length for the "trial" angle of 0.82 radians was ...
8.206 +8.795 = 17.001 . . . ft
The actual shortest ladder is ...
12√2 = 16.971 . . . feet

Which type of parent function is f(x) = |x|?
O A. Cube root
B. Square root
C. Linear
D. Absolute value
Answers
Answer:
D. Absolute value
Step-by-step explanation:
There are absolute value bars around x, so the function is absolute value.
Hope this helps...have a great day ^^
Find the product of ¾ and 1⅓ a)0 (b)1 (c)12 (d)1⁵/7

Answers
The value of the product of the number as given in the task content is; Choice B; 1.
What is the value big the product of the values; ¾ and 1⅓?It follows from the task content that the numbers whose product are to be determined are; ¾ and 1⅓.
To effectively multiply, we must convert the mixed number to a fraction as follows;
1⅓ = 4/3.
Hence, the product is; 4/3 × 3/4 = 12/12 = 1.
Read more on product of numbers;
https://brainly.com/question/10873737
#SPJ1
Find the slope of the line graphed

Answers
Answer:
1. 5/-2
2. 0/1
3. 6/2
Step-by-step explanation:
rise/run when calculating slope
I would like anyone to help me with this, please!

Answers
Answer:
the sencond and the last one
Answer:
125 • 125
Step-by-step explanation:
5⁶ = 5 × 5 × 5 × 5 × 5 × 5 = 15,625
125 • 125 = 15,625
They give the same result so both expressions are equivalent to each other.
14 Calculate the mode from the following data: 7,8, 6, 5, 10, 11, 4, 5,2 b. 5: а. 3.' 4 6 с. d: 6
Answers
MODE IS THE NUMBER THAT IS REPEATED THE HIGHEST TIME..
HERE, IN YOUR QUESTION 5CAME 2 TIMES i.e. it is repeated highest time .so mode=5....
Consider an experiment in which the number of pumps in use at each of two nine-pump gas stations was determined. Call the two gas stations Station A and Station B. Define rv's X, Y, and U by. x= the total number of pumps in use at the two stations. y=the difference between the number of pumps in use at Station A and the number in use at Station B. U=the maximum of the numbers of pumps in use at the two stations
Another random variable could be V- the difference between the maximum and minimum number of pumps in use at the two stations. What are the possible values of V?
A. 1, 2, 3, 4, 5, 6,7, 8,9
B. -8,-7,-6,-5,-4,-3,-2,-1,0
C. 0,1,2,3,4,5,6,7,8
D. -9, -8,-7,-6,-5,-4,-3,-2,-1,0
E. -9, -8,-7,-6,-5,-4,-3,-2,-1,0, 1,2,3,4,5,6,7,8,9
Answers
The possible values of V is E. -9, -2, -1, 0, 1, 2, 3, 4, 5, 6, 7, 8, 9.
To find the possible values of V, we need to consider the maximum and minimum number of pumps in use at the two stations. Let's call the maximum number of pumps used at either station as M, or the minimum number of pumps used as m. Then, the possible values of V would be from -(M-m) to (M-m).
Since each gas station has nine pumps, the maximum number of pumps in use at either station can be 9, and the minimum number of pumps in use at either station can be 0 (if all pumps are idle). Therefore, the possible values of M-m can range from 0 to 9, which means the possible values of V can range from -9 to 9.
The correct answer is E. -9, -2, -1, 0, 1, 2, 3, 4, 5, 6, 7, 8, 9.
To know more about Gas Stations:
https://brainly.com/question/14867812
#SPJ4
Please take a look at the picture

Answers
Answer: C
Step-by-step explanation:
can someone tell me how to find this problem?

Answers
The composition of functions f and g is:
(f o g) =6x^2 + 15x + 2
How to find the composition of functions?Here we have two known functions:
f(x) = 3x + 2
g(x) = 2x^2 + 5x
We want to find the composition:
(f o g)
That just means that we need to evaluate function f(x) in x = g(x), we wikk get:
(f o g) = 3*g(x) + 2
Now we can replace g(x) there to get:
(f o g) = 3*(2x^2 + 5x) + 2
= 6x^2 + 15x + 2
That is the composition.
Learn more about composition of functions at:
https://brainly.com/question/10687170
#SPJ1
For questions 1-2, use the Reciprocal and Quotient Identities to find each value.
Answers
By using the reciprocal identity the value \(Sin \theta\) will be \(\dfrac{5}{27}{\)
What is Reciprocal and Quotient Identities?In trigonometry, quotient identities refer to trig identities that are divided by each other.
So here we have
\(Cosec\theta = \dfrac{27}{5}\)
Now we have to find the reciprocal identity,
\(Sin\theta=\dfrac{1}{Cosec\theta}\)
\(Sin\theta}=\dfrac{1}{\dfrac{27} { 5} }\)
\(Sin\theta=\dfrac{5}{27}\)
hence by using the reciprocal identity the value \(Sin \theta\) will be \(\dfrac{5}{27}{\)
To know more about Reciprocating identity follow
https://brainly.com/question/15769816
#SPJ1
what the mean of 1,3,5,5, 1, 3, 0, 0, 5
Answers
1+3+5+5+1+3+5+0+0
4+5+5+1+3+5
9+5+1+3+5
14+1+3+5
15+3+5
18+5
23
23/9
the mean is 2 and 5/9
1+3+5+5+1+3+0+0+5=23
23/9=2.55555
Makesha lost 58.5 pounds in 18 weeks. Find her rate of loss in pounds per week.
Answers
Can someone please give me the answer to this?

Answers
Answer:
About 36.87
Step-by-step explanation:
The sine of an angle in a right triangle is the length of the opposite side divided by the length of a hypotenuse, which in this case is 3/5. Therefore, the measure of the angle is the arcsin of 3/5 or about 36.87 degrees. Hope this helps!