Suppose that p and q are statements so that p →q is false. Find the truth values of each of the following. (a) ~p → 9 True False (b) p v q True False (c) q → p True False
Answers
Given that p → q is false, this means that either p is true and q is false or both p and q are false. Let's use a truth table to find the truth values of the given statements:
p q p → q
T F F
F T T
F F T
T T T
(a) ~p → 9:
p ~p ~p → 9
T F T
F T T
Since p → q is false, p must be true and q must be false. Therefore, ~p is false, and any statement of the form false → q is true regardless of the truth value of q. So, ~p → 9 is true.
(b) p v q:
p q p v q
T F T
F T T
F F F
T T T
Since p → q is false, we know that at least one of p and q must be false. If p is false and q is true, then p v q is true. Otherwise, if both p and q are false, then p v q is false. Therefore, p v q is true if q is true and false if both p and q are false.
(c) q → p:
p q q → p
T F T
F T F
F F T
T T T
Since p → q is false, we know that p must be true and q must be false. Therefore, q → p is false, since false → true is false.
For more details about values click here:
https://brainly.com/question/30145972#
#SPJ11
Related Questions
Write an equation to find the nth term for: 7,13,19,25…
Answers
Answer:
\(a_{n} = a_{1} + d(n - 1) \\ d = 6 \\ a_{n} = 7 + 6(n - 1)\)
Given a normal distribution with mu equals 100 and sigma equals 10 comma complete parts (a) through (d). LOADING... Click here to view page 1 of the cumulative standardized normal distribution table. LOADING... Click here to view page 2 of the cumulative standardized normal distribution table. a. What is the probability that Upper X greater than 70? The probability that Upper X greater than 70 is .0016 nothing. (Round to four decimal places asneeded.) b. What is the probability that Upper X less than 80? The probability that Upper X less than 80 is nothing. (Round to four decimal places as needed.) c. What is the probability that Upper X less than 95 or Upper X greater than 125? The probability that Upper X less than 95 or Upper X greater than 125 is nothing.(Round to four decimal places as needed.) d. 99% of the values are between what two X-values (symmetrically distributed around the mean)? 99% of the values are greater than nothing and less than nothing.
Answers
a Probability that Upper X 0.0013 ,
b. Upper X less than 80 is 0.0228
c Upper X less than 95 or Upper X greater than 125 is 0.6853.
d 99% of the values are between 76.7 and 123.3 (symmetrically distributed around the mean).
Given a normal distribution with mu equals 100 and sigma equals 10, we can use the cumulative standardized normal distribution table to complete the following parts:
a. What is the probability that Upper X greater than 70?
Using the cumulative standardized normal distribution table, we find the z-score for 70 as (70-100)/10 = -3. We then look up the probability for a z-score of -3, which is 0.0013. Therefore, the probability that Upper X greater than 70 is 0.0013. (Round to four decimal places as needed.)
b. What is the probability that Upper X less than 80?
Using the cumulative standardized normal distribution table, we find the z-score for 80 as (80-100)/10 = -2. We then look up the probability for a z-score of -2, which is 0.0228. Therefore, the probability that Upper X less than 80 is 0.0228. (Round to four decimal places as needed.)
c. What is the probability that Upper X less than 95 or Upper X greater than 125?
Using the cumulative standardized normal distribution table, we find the z-score for 95 as (95-100)/10 = -0.5 and the z-score for 125 as (125-100)/10 = 2.5. We then find the probabilities for each of these z-scores, which are 0.3085 and 0.0062, respectively. To find the probability that Upper X is either less than 95 or greater than 125, we add these two probabilities and subtract from 1 (to account for the overlap): 1 - (0.3085 + 0.0062) = 0.6853. Therefore, the probability that Upper X less than 95 or Upper X greater than 125 is 0.6853. (Round to four decimal places as needed.)
d. 99% of the values are between what two X-values (symmetrically distributed around the mean)?
To find the z-score corresponding to the 99th percentile, we look up the probability of 0.99 in the cumulative standardized normal distribution table, which is 2.33 (rounded to two decimal places). Using this z-score, we can find the corresponding X-values using the formula z = (X - mu)/sigma. Solving for X, we get: X = z*sigma + mu = (2.33)(10) + 100 = 123.3 and X = (-2.33)(10) + 100 = 76.7. Therefore, 99% of the values are between 76.7 and 123.3 (symmetrically distributed around the mean).
Learn more about Probability there:
https://brainly.com/question/30034780
#SPJ11
Please help me find the answer

Answers
Answer:
x = 0
y = 2
Step-by-step explanation:
We can find the value of y using the steps on the image
Then we will use that to find x
2x + 3y = 6 replace y with 2
2x + 3*2 = 6
2x + 6 = 6 subtract 6 from both sides
2x = 0 and x = 0

It costs $7.15 to rent a kayak for 1 hour at a local state park. The price per hour stays the same for up to 5 hours of rental. After 5 hours, the cost decreases to $5.75 per hour.
How much would it cost to rent a kayak for 6 hours?
Answers
Answer:
7.15 times 5 plus 5.75=$41.5
Step-by-step explanation:
Answer:
$41.50
Step-by-step explanation:
Multiply 7.15 by 5. This represents how much it costs for the first 5 hours.Add 5.75 to the product. This is the extra hour with the reduced price.Which equation represents a line which is parallel to the line 2x+7y=-56
Answers
hi everyone help my math pls
show ur solutions

Answers
On solving the provided question, we can say that - the area of the provided circle is A = \(113.04 cm^2\)
What is circle?Every point in the plane that is a certain distance away from a certain point forms a circle (center). It is, thus, a curve formed by points moving in the plane at a fixed distance from a point. At every angle, it is also rotationally symmetric about the center. A circle is a closed two-dimensional object where every pair of points in the plane are equally spaced out from the "center." A line that goes through the circle creates a specular symmetry line. At every angle, it is also rotationally symmetric about the center.
here,
radius, r = 6 cm
Area of circle = \(\pi X r^2 = 3.14 X 6 X 6 \\\)
A = \(113.04 cm^2\)
To know more about circle visit:
https://brainly.com/question/29142813
#SPJ1
James wants to invest $12,000. He can invest the money at 7.2% simple interest for 30 yr or he can invest at 6.8% with interest compounded continuously for 30 yr. Which option results in more total interest?
Answers
We have to find which option results in more total interest. For the first option, the simple interest is given by: I = P × r × t Where,
P = Principal amount,
r = rate of interest,
t = time in years.
The simple interest that James will earn on the investment is given by:
I₁ = P × r × t
= $12,000 × 0.072 × 30
= $25,920
For the second option, the interest is compounded continuously. The formula for calculating the amount with continuously compounded interest is given by:
A = Pert Where,
P = Principal amount,
r = rate of interest,
t = time in years.
The amount that James will earn on the investment is given by:
= $49,870.83
Total interest in the second case is given by:
A - P = $49,870.83 - $12,000
= $37,870.83
James will earn more interest in the second case where he invests $12,000 at 6.8% with interest compounded continuously for 30 years. He will earn a total interest of $37,870.83.
To know more about invest visit:
https://brainly.com/question/10908938
#SPJ11
Suppose there are two samples from populations A and B. The sample from population A is of size 18 and the sample from population B is of size 21. The sample from population A has a sample variance of 8490 and the sample from population B has a sample variance of 6330. Find the pooled sample variance.
Round your answer to 2 decimal places.
Answers
The pooled sample variance, rounded to 2 decimal places, is 7,316.22.
To find the pooled sample variance of the two samples from populations A and B, follow these steps,
1. Calculate the weighted variance for each sample:
- For sample A: (Sample size A - 1) * Sample variance A = (18 - 1) * 8490 = 17 * 8490 = 144,330
- For sample B: (Sample size B - 1) * Sample variance B = (21 - 1) * 6330 = 20 * 6330 = 126,600
2. Calculate the total degrees of freedom:
- Total degrees of freedom = (Sample size A - 1) + (Sample size B - 1) = 17 + 20 = 37
3. Calculate the pooled sample variance:
- Pooled sample variance = (Weighted variance A + Weighted variance B) / Total degrees of freedom = (144,330 + 126,600) / 37 ≈ 7,316.22
The pooled sample variance, rounded to 2 decimal places, is 7,316.22.
Learn more about "variance": https://brainly.com/question/9304306
#SPJ11
If the allele frequency of the dominant allele is 0.4, what value is used for the term p^2 in the equation p^2+ 2pq + q^2 = 1?
Answers
The value is used for the term p^2 in the equation p^2+ 2pq + q^2 = 1 is 0.16.
According to the statement
we have given that the allele frequency of the dominant allele is 0.4, and we have to find that the value of p^2 in the equation p^2+ 2pq + q^2 = 1.
So, For this purpose, we know that the
The allele frequency represents the incidence of a gene variant in a population. Alleles are variant forms of a gene that are located at the same position, or genetic locus, on a chromosome.
And here
allele frequency is the 0.4 and represent the value of P.
So, The value of p is 0.4 and the
Then p^2 = (0.4)^2
so, the value becomes
p^2 = (0.4)^2
p^2 = 0.16.
So, The value is used for the term p^2 in the equation p^2+ 2pq + q^2 = 1 is 0.16.
Learn more about allele frequency here
https://brainly.com/question/6139888
#SPJ4
solve for x. round to nearest hundredth. PLS HELP. WILL AWARD BRAINLIEST

Answers
Answer:
16.52
Step-by-step explanation:
Use Pyth theorem
4^2 + b^2 = 17^2
16 + b^2 = 289
b^2 = 273
b = 16.52
elementary statistics, a step-by-step approach, 10th ed., bluman
Answers
The subject is taught in a non-theoretical manner in Al Bluman's Elementary Statistics. Today's world speaks statistics, and Bluman's market-leading Step-by-Step Approach makes it simple to learn and comprehend.
Bluman gives your students all the assistance they need to understand the foundations of statistics and draw that link by assisting them in transitioning from the computational to the conceptual.
Elementary Statistical Methods are the collection, analysis, presentation, and interpretation of data, and probability. The analysis includes descriptive statistics, correlation and regression, confidence intervals, and hypothesis testing.
Thus, The subject is taught in a non-theoretical manner in Al Bluman's Elementary Statistics. Today's world speaks statistics, and Bluman's market-leading Step-by-Step Approach makes it simple to learn and comprehend.
To know more about Elementary Statistics visit: brainly.com/question/30144742
#SPJ4
Please solve this! NO ROBOTS!

Answers
Answer: 54° or 40°, depending on my interpretation of the problem.
Step-by-step explanation:
I do not understand the drawing. I see a right angle with a line bisecting it at 36 degrees. A right angle is 90°, so the other angle should be (90 - 36) or 54°. But what's shown is (14 - 1)°, and I don't know how to interpret that. Is the "1" supposed to be an "x"? If so, x = 40°
Use part 1 of the fundamental theorem of calculus to find the derivative of the function. g(x) = x e5t2 − 4t dt 4 g'(x) =
Answers
The derivative of the given equation is \(\frac{dg(x) }{dx} = {e^{5x^{2} - 4x} } \,\).
According to the statement
we have given that the statement and we have to find the derivative of that term.
So, We know that the
The given equation is
\(g(t) = \int\limits^x_4 {e^{5t^{2} - 4t} } \, dt\)
Now find the derivative of that term
We find the derivative of the given term is with the help of the FTC.
And then
\(\frac{dg(x) }{dt} = {e^{5x^{2} - 4x} } \, \frac{dx}{dx}\)
Then the equation become is
\(\frac{dg(x) }{dx} = {e^{5x^{2} - 4x} } \,\)
So, this is the derivative of the given equation.
So, The derivative of the given equation is \(\frac{dg(x) }{dx} = {e^{5x^{2} - 4x} } \,\).
Learn more about derivative here
https://brainly.com/question/23819325
#SPJ4
the fact that only 63% of high school graduates have broadband internet access at home while almost 90% of college graduates do is an example of
Answers
By seeing the percentages given, it can be concluded that
the fact that only 63% of high school graduates have broadband internet access at home while almost 90% of college graduates do is an example of Digital divide
What is percentage?
Suppose there is a number and the number has to be expressed as a fraction of 100. That fraction is called percentage.
For example 2% means \(\frac{2}{100}\)
Here 2 is expressed as a fraction of 100
Here, only 63% of high school graduates have broadband internet access at home while almost 90% of college graduates have broadband internet access. Here limited group of high school graduate student has access to broadband internet access at home while almost unlimited group of college graduate students has access to broadband internet access at home. So there is a huge difference of the distribution of broadband internet access at home between high school graduate and college graduate. So this is a case of Digital divide.
So the fact that only 63% of high school graduates have broadband internet access at home while almost 90% of college graduates do is an example of Digital divide
To learn more about percentage, refer to the link-
brainly.com/question/24877689
#SPJ4
Please I beg of you I need it right now with answer and full explanation 35 points for it.

Answers
Answer:
Step-by-step explanation:
i think the solution is (-2,0) because that is where they touch at the x axis
Solutions are (-2, 0) and (0, -4)
a population of 10 scores has a mean of 50 and a standard deviation of 5. what is the population variance?
Answers
The value of the Population Variance is 25.
Given:
The standard deviation of a population is 5
The mean of a population is 50
The size of a population is 10
\(Variance = (Standard \ deviation)^{2}\)
\(Variance = 5^{2}\)
σ = 25.
So, The value of the Population Variance is 25.
Population variance is a metric for gauging how dispersed a set of data points is. It calculates the typical squared deviation from the mean. Therefore, the variance will be smaller if all of the data points are close to the mean than it will be if the data points are dispersed widely.
We are aware that the population variance describes the distribution of data points within a population by averaging the squared distances between each data point and the mean.
For such more question on variance:
brainly.com/question/28137083
#SPJ4
Which rational number is NOT greater than point A?
A. 214
B. 1.95
C. 2.05
D. 1624
Answers
Step-by-step explanation:
) Every positive rational number is greater than 0.
(ii) Every negative rational number is less than 0.
(iii) Every positive rational number is greater than every negative rational number.
(iv) Every rational number represented by a point on the number line is greater than every rational number represented by points on its left.
(v) Every rational number represented by a point on the number line is less than every rational number represented by paints on its right
b
A model rocket is launched from the roof of a building. It’s height can be found by using h(t)= -5t^2 + 30t + 9 where h is its height in meters and t is the time after the launch in seconds, as shown in the graph. Find the maximum height of the rocket. Show work

Answers
Answer:
The vertex of the parabola is found by setting the derivative of the function equal to zero and solving for t. The derivative of h(t) is h'(t) = -10t + 30. Setting this equal to zero and solving for t, we get t = 3.
Substituting t = 3 into h(t), we get h(3) = -5(3)^2 + 30(3) + 9 = 55 meters.
Copy and complete each of the equalities
below using the options given.
a) sin-¹)=30° 45° 60°
(b) cos-¹) = 30° 45° 60°
C) tan-¹)=30° 45° 60°
Answers
Completing the equalities using the given options, we have:
\(a) sin^(-1)(1) = 90°\\b) cos^(-1)(1/2) = 60°\\c) tan^(-1)(√3) = 60°\)
a) \(sin^(-1)(1) = 90°\)
The inverse sine function, \(sin^(-1)(x)\)gives the angle whose sine is equal to x. In this case, we are looking for the angle whose sine is equal to 1. The angle that satisfies this condition is 90 degrees, so\(sin^(-1)(1) = 90°\).
b) \(cos^(-1)(1/2) = 60°\)
The inverse cosine function, cos^(-1)(x), gives the angle whose cosine is equal to x. Here, we are looking for the angle whose cosine is equal to 1/2. The angle that satisfies this condition is 60 degrees, so \(cos^(-1)(1/2)\)= 60°.
c) \(tan^(-1)(√3) = 60°\)
The inverse tangent function, tan^(-1)(x), gives the angle whose tangent is equal to x. In this case, we are looking for the angle whose tangent is equal to √3. The angle that satisfies this condition is 60 degrees, so tan^(-1)(√3) = 60°.
Completing the equalities using the given options, we have:
\(a) sin^(-1)(1) = 90° b) cos^(-1)(1/2) = 60°c) tan^(-1)(√3) = 60°\)
For more such questions on Equalities
https://brainly.com/question/30339264
#SPJ8
a) The completed equalities are:
sin-¹(x) = 30°, sin-¹(x) = 45°, sin-¹(x) = 60°
b) The completed equalities are:
cos-¹(x) = 30°, cos-¹(x) = 45°, cos-¹(x) = 60°
c) The completed equalities are:
tan-¹(x) = 30°, tan-¹(x) = 45°, tan-¹(x) = 60°. C.
a) sin-¹(x) = 30°, 45°, 60°
The inverse sine function, sin-¹(x), gives the angle whose sine is equal to x.
Let's find the angles for each option given:
sin-¹(x) = 30°:
If sin-¹(x) = 30°, it means that sin(30°) = x.
The sine of 30° is 0.5, so x = 0.5.
sin-¹(x) = 45°:
If sin-¹(x) = 45°, it means that sin(45°) = x.
The sine of 45° is √2/2, so x = √2/2.
sin-¹(x) = 60°:
If sin-¹(x) = 60°, it means that sin(60°) = x.
The sine of 60° is √3/2, so x = √3/2.
The completed equalities are:
b) cos-¹(x) = 30°, 45°, 60°
The inverse cosine function, cos-¹(x), gives the angle whose cosine is equal to x.
Let's find the angles for each option given:
cos-¹(x) = 30°:
If cos-¹(x) = 30°, it means that cos(30°) = x.
The cosine of 30° is √3/2, so x = √3/2.
cos-¹(x) = 45°:
If cos-¹(x) = 45°, it means that cos(45°) = x.
The cosine of 45° is √2/2, so x = √2/2.
cos-¹(x) = 60°:
If cos-¹(x) = 60°, it means that cos(60°) = x.
The cosine of 60° is 0.5, so x = 0.5.
Therefore, the completed equalities are:
c) tan-¹(x) = 30°, 45°, 60°
The inverse tangent function, tan-¹(x), gives the angle whose tangent is equal to x.
Let's find the angles for each option given:
tan-¹(x) = 30°:
If tan-¹(x) = 30°, it means that tan(30°) = x.
The tangent of 30° is 1/√3, so x = 1/√3.
tan-¹(x) = 45°:
If tan-¹(x) = 45°, it means that tan(45°) = x.
The tangent of 45° is 1, so x = 1.
tan-¹(x) = 60°:
If tan-¹(x) = 60°, it means that tan(60°) = x.
The tangent of 60° is √3, so x = √3.
The completed equalities are:
tan-¹(x) = 30°, tan-¹(x) = 45°, tan-¹(x) = 60°. c)
For similar questions on completed equalities
https://brainly.com/question/29097867
#SPJ8
If the expression ___ is written in the form _____ then what is the product of a, b, and c?

Answers
Answer:
\( \frac{ {x}^{ - 2} {y}^{ \frac{1}{2} } }{ \sqrt{36x {y}^{2} } } = \frac{ \sqrt{y} }{ {x}^{2} \sqrt{36x {y}^{2} } } = \frac{ \sqrt{y} }{6 {x}^{2}y \sqrt{x} } = \frac{1}{6 {x}^{ \frac{5}{2} } {y}^{ \frac{1}{2} } } = \frac{1}{6} {x}^{ - \frac{5}{2} } {y}^{ - \frac{1}{2} } \)
\( \frac{1}{6} \times - \frac{5}{2} \times - \frac{1}{2} = \frac{5}{24} \)
which expression is equivalent to square root of 8x^7y^6? assume x is greater than or equal to 0
Answers
Answer:
\(2x^3y^3\sqrt{2x}\)
Step-by-step explanation:
= \(\sqrt{8x^7y^6}\)
= \(\sqrt{2^2 *2*x^6*x*y^6\)
= \(2x^3y^3\sqrt{2x}\)
Can someone help on this please? Thank youu;)
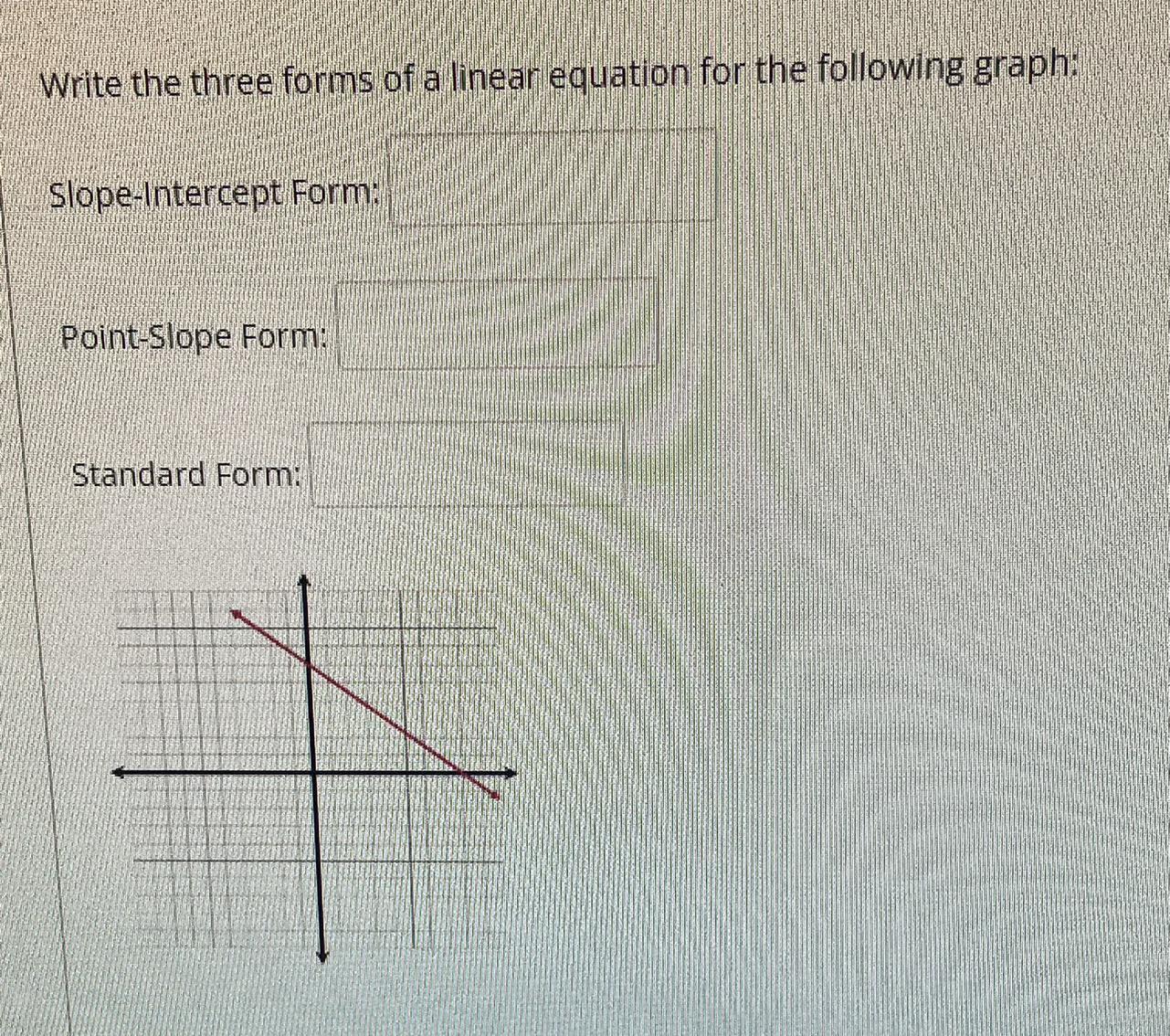
Answers
The equation of the line is written in the different forms
slope-intercept form: y = (-3/4)x + 6point slope form: y - 6 = (-3/4)xstandard form: 3x + 4y = 24How to write the equation of the lineCalculate the slope (m) using the formula:
m = (0 - 6) / (8 - 0)
m = -6 / 8
m = -3/4
Plug in the slope (m) and one of the given points (x1, y1) into the slope-intercept form to find the y-intercept (b):
y = mx + b
6 = (-3/4)(0) + b
6 = b
Substitute the y-intercept (b) into the equation:
y = (-3/4)x + 6
In point-slope form:
y - y1 = m(x - x1)
Using the point (0, 6):
y - 6 = (-3/4)(x - 0)
y - 6 = (-3/4)x
In standard form:
To convert the equation to standard form, we can manipulate the equation to have the form Ax + By = C, where A, B, and C are constants.
y - 6 = (-3/4)x
Multiply both sides by 4 to eliminate the fraction:
4(y - 6) = -3x
4y - 24 = -3x
Rearrange the equation to have x and y on the same side and a constant on the other side:
3x + 4y = 24
This is the equation in standard form.
Learn more about equation of line at
https://brainly.com/question/18831322
#SPJ1
Based on the information given, can you determine that the quadrilateral must be a parallelogram? Explain.
Given: XN = NZ and NY = NW

Answers
Answer:
Because N is the midpoint of the two diagonals so the quadrilateral must be a parallelogram
Answer:
Step-by-step explanation:
XN = NZ & NY = NW
This shows that diagonals XZ & WY bisect each other.
So, this quadrilateral is a parallelogram.
no L'Hopital's Rule, Product Rule, Quotient Rule, Chain Rule
Use the limit definition of the derivative to find f′(x) for f(x)=7/(5x−3)
Answers
Answer: The derivative of f(x) is f '(x) = -35/[5x - 3]^2.
Given the function f(x) = 7/(5x - 3), we have to find the derivative of this function by using the limit definition of the derivative without using L'Hopital's Rule, Product Rule, Quotient Rule, Chain Rule.
Derivative using the limit definition is given as f '(x) = lim(h → 0) [f(x + h) - f(x)]/h
We have to apply this formula to find the derivative of f(x) = 7/(5x - 3).
We substitute f(x) into the formula for f(x+h), we get: f (x+h) = 7/[5(x+h) - 3]
The derivative of f(x) isf '(x) = lim(h → 0) [f(x + h) - f(x)]/h
= lim(h → 0) [7/{5(x + h) - 3} - 7/{5x - 3}]/h
Taking the LCM of the denominator, we get the following expression f '(x) = lim(h → 0) [7(5x - 3) - 7(5x + 5h - 3)]/h[5(x + h) - 3][5x - 3][5(x + h) - 3]
Taking 7 as a common factor, we getf '(x) = lim(h → 0) [-35h]/[h(5x + 5h - 3)(5x - 3)]
Now, we cancel out h from both the numerator and denominator, we getf '(x) = lim(h
→ 0) [-35]/[(5x + 5h - 3)(5x - 3)]
Taking the limit as h → 0, we getf '(x) = -35/[5x - 3]^2
Therefore, the derivative of f(x) is f '(x)
= -35/[5x - 3]^2.
To know more about derivative visit:
https://brainly.com/question/29144258
#SPJ11
Let f(x) = x2 - 16 and g(x) = x + 4. Find (f = g)(x).

Answers
Answer:
3rd option is correct for answer
Step-by-step explanation:
(x^2-16)/x+4
(x)^2-(4)^4/ x+4
(x-4)(x+4)/(x+4)
(x-4)
so (x-4) ,when x not equal to 4 is the answer
Answer:
\((x-4)\), \((x\neq -4)\)
Step-by-step explanation:
Given,
\(f(x)=x^2 - 16\\g(x) = x + 4\\\)
Find,
\((f\)÷ \(g)(x)\)
1. Approach
The easiest way to solve the problem is to set up the problem as a fraction. Then factor both the numerator (value on top of the fraction bar), and the denominator (value under the fraction bar). After doing so, simplify the fraction. Remember to take note of the value eliminated from the denominator, for this value will serve as the domain of the expression.
2. Divide the two functions
The two given functions are the following:
\(f(x)=x^2 - 16\\g(x) = x + 4\\\)
Set up the division problem,
\(\frac{f(x)}{g(x)}\)
Substitute,
\(\frac{x^2-16}{x+4}\)
Factor,
\(\frac{(x-4)(x+4)}{(x+4)}\)
Simplify,
\((x-4)\)
3. Find the domain of the expression
The value that was removed from the denominator is the following:
\((x+4)\)
Set this equal to zero and solve,
\(x+4=0\\\\x=-4\)
This expression is true as long as (\(x\neq -4\)).
What is: 8x + 7 - 3x
Answers
Answer: 5x + 7
Step-by-step explanation:
Combine like terms
How many and what type of solutions does 5x2−2x+6 have?
1 rational solution
2 rational solutions
2 irrational solutions
2 nonreal solutions
Answers
Answer:
2 nonreal solutions
Step-by-step explanation:
given a quadratic equation in standard form
ax² + bx + c = 0 (a ≠ 0 )
then the nature of the roots are determined by the discriminant
b² - 4ac
• if b² - 4ac > 0 then 2 real and irrational solutions
• if b² - 4ac > 0 and a perfect square then 2 real and rational solutions
• if b² - 4ac = 0 then 2 real and equal solutions
• if b² - 4ac < 0 then no real solutions
5x² - 2x + 6 = 0 ← in standard form
with a = 5 , b = - 2 , c = 6
b² - 4ac
= (- 2)² - (4 × 5 × 6)
= 4 - 120
= - 116
since b² - 4ac < 0
then there are 2 nonreal solutions to the equation
what is the slop of 2x+3
Answers
Answer:
2
Step-by-step explanation:
The slope, or rate of change in any function is the number multiplied by the independent variable (in this case x).
The slope of the line is the value of
m
, and the y-intercept is the value of b
Sketch AABC in which A = 43°, B = 101° and a = 7.5 cm.
Find b and c, in cm correct to two decimal places.
Answers
We know the angles A and B and the length of side a we found the lengths of sides b = 10.79 cm and c = 6.46 cm :
Start by drawing a line segment of length 7.5 cm as side a.
At one end of side a, draw an angle of 43°, which is angle A.
At the other end of side a, draw an angle of 101°, which is angle B. Make sure the angle is wide enough to intersect with the other side.
The intersection of the two angles will be point C, completing the triangle.
To find the lengths of sides b and c, you can use the law of sines. The law of sines states that the ratio of the length of a side to the sine of its opposite angle is the same for all sides of a triangle.
Using the law of sines: b / sin(B) = a / sin(A)
b / sin(101°) = 7.5 cm / sin(43°)
Now, you can solve for b: b = sin(101°) * (7.5 cm / sin(43°))
b = 10.79 cm
Similarly, you can find c using the law of sines: c / sin(C) = a / sin(A)
c / sin(180° - A - B) = 7.5 cm / sin(43°)
Solve for c: c = sin(180° - A - B) * (7.5 cm / sin(43°))
c = 6.46 cm
LEARN MORE ABOUT triangle here: brainly.com/question/2773823
#SPJ11
a rectangle has a perimeter of 128 inches. the length is four less than twice the width. what is the length of the rectangle?
Answers
The length of the rectangle is approximately 41.34 inches.
Let's assume the width of the rectangle is represented by the variable w. According to the given information, the length of the rectangle is four less than twice the width, which can be expressed as 2w - 4.
The perimeter of a rectangle is calculated by adding the lengths of all four sides. In this case, the perimeter is given as 128 inches. Since a rectangle has two pairs of equal sides, we can set up the equation:
2w + 2(2w - 4) = 128.
Simplifying the equation, we get:
2w + 4w - 8 = 128,
6w - 8 = 128,
6w = 136,
w = 22.67.
So, the width of the rectangle is approximately 22.67 inches. To find the length, we can substitute this value back into the expression 2w - 4:
2(22.67) - 4 = 41.34.
Therefore, the length of the rectangle is approximately 41.34 inches.
In summary, the length of the rectangle is approximately 41.34 inches. This is determined by setting up a system of equations based on the given information: the perimeter of the rectangle being 128 inches and the length being four less than twice the width.
By solving the system of equations, we find that the width is approximately 22.67 inches, and substituting this value back, we obtain the length of approximately 41.34 inches.
To know more about length refer here:
https://brainly.com/question/2497593
#SPJ11