Solve the equation. (List your answers counterclockwise about the origin starting at the positive real axis. Express θ in radians.)
z^3 + 3 = -3i
Answers
Expressing the angles θ in radians, the solutions are: z1 ≈ 1.229 * \(e^{(-\pi i/12)\), z2 ≈ 1.229 * \(e^{(7\pi i/12)\) and z3 ≈ 1.229 * \(e^{(11\pi i/12)\). These solutions can be plotted counterclockwise about the origin starting at the positive real axis on the complex plane.
To solve the equation, we can rewrite it in exponential form using Euler's formula:
z³ + 3 = -3i
z³ = -3 - 3i
Now, let's convert -3 - 3i to polar form:
-3 - 3i = 3√2 * (-1/√2 - i/√2)
= 3√2 * \(e^{(-i\pi /4)\)
We can write z³ as r³ * \(e^{(i\theta3)\), where r is the magnitude of z and θ3 is the argument of z³.
So, we have:
r³ * e^(iθ3) = 3√2 * \(e^{(-i\pi /4)\)
Comparing the real and imaginary parts of both sides, we get:
r³ = 3√2
e^(iθ3) = \(e^{(-i\pi /4)\)
From the first equation, we can solve for r:
r = (3√2)¹/³
r ≈ 1.817
From the second equation, we know that θ3 = -π/4.
Now, let's find the three cube roots of r * \(e^{(i\theta)\):
z1 = r¹/³ * \(e^{(i\theta/3)\)
z1 ≈ 1.229 * \(e^{(-i\pi /12)\)
z2 = r¹/³ * \(e^{(i(\theta/3 + 2\pi /3))\)
z2 ≈ 1.229 * \(e^{(i7\pi /12)\)
z3 = r¹/³ * \(e^{(i(\theta/3 + 4\pi /3))\)
z3 ≈ 1.229 * \(e^{(i11\pi /12)\)
So, the solutions to the equation z³ + 3 = -3i are approximately:
z1 ≈ 1.229 * \(e^{(-i\pi /12)\)
z2 ≈ 1.229 * \(e^{(i7\pi /12)\)
z3 ≈ 1.229 * \(e^{(11\pi i/12)\)
Therefore, expressing the angles θ in radians, the solutions are: z1 ≈ 1.229 * \(e^{(-i\pi /12)\), z2 ≈ 1.229 * \(e^{(7\pi i/12)\)and z3 ≈ 1.229 * \(e^{(11\pi i/12)\). These solutions can be plotted counterclockwise about the origin starting at the positive real axis on the complex plane.
To know more about equations check the below link:
https://brainly.com/question/30396383
#SPJ4
Related Questions
NEED HELP FAST
A restaurant plans to use a new food delivery service. The food delivery service charges $4.98 for every 2 meals delivered, plus a $2.00 service fee. What is the slope of this situation?
2.00
2.49
4.49
4.98
Answers
Answer:
The slope of this situation would be $4.98/2 = $2.49 per meal delivered.
Step-by-step explanation:
The slope in this situation represents the cost per meal delivered. To find the cost per meal, we can divide the total cost by the number of meals.
Let's say the restaurant plans to deliver n meals. The cost of delivering n meals is given by:
Cost = (n/2) x 4.98 + 2.00
The first term represents the cost of delivering n meals in pairs (since the service charges for every 2 meals delivered), and the second term represents the flat service fee.
To find the cost per meal, we can divide both sides of the equation by n:
Cost/n = [(n/2) x 4.98 + 2.00]/n
Simplifying, we get:
Cost/n = 2.49 + 2.49/n
So the slope of this situation is 2.49, which represents the cost per meal delivered.
A gardener wants to divide a square piece of lawn in half diagonally. What is the length of the diagonal if the side of the square is ? Leave your answer in simplest radical form.Viruses
a. 16√8
b. 2√8
c. 8√8
d. 4
Answers
The correct response is c. 8√2 . The length of the diagonal if the side of the square is 8√2.
A polygon's opposed vertices (or corners) are connected by a line segment known as a diagonal. Or to put it another way, a diagonal is a line segment joining two polygonal vertices that are not neighbouring. With the exception of the figure's edges, it connects a polygon's vertices. For instance, a diagonal line or movement runs slopingly from one corner of a square to the other corner. a type of straight line called a diagonal. Straight up, down, or across are not possible on a diagonal line. It is a line that joins the corners of a form at two points. Instead of running straight up or across, a diagonal is formed by a straight line that is positioned at an angle.
Right isosceles triangles with both legs 8 feet long would make up each half.
The hypotenuse that both of those triangles would share is the diagonal.
We must determine the hypotenuse's length, d, in feet.
According to the Pythagorean theorem, the lengths of the legs' squares sum up to the length of the hypotenuse's square, so
\(8^{2} +8^{2} =8\sqrt{2}\)
To know more about diagonal here
https://brainly.com/question/25740584
#SPJ4
A gardener wants to divide a square piece of lawn in half diagonally. What is the length of the diagonal if the side of the square is 8ft? Leave your answer in simplest radical form.
a. 16√8
b. 2√8
c. 8√2
d. 4
One evening in snowed in several towns the number of inches of snow received are shown in the list below
Answers
Answer:
Please find the required line chart created with MS Excel
Step-by-step explanation:
Question:
The given data are;
3/4, 1 1/2, 2, 1 1/4, 3/4, 2, 1 1/2, 1 1/4, 1 1/2, 1/2, 1
In the question, it is required to create a line plot to represent the data
The given data can be sorted in ascending order in decimal form as follows;
0.5, 0.75, 0.75, 1, 1.25, 1.25, 1.5, 1.5, 1.5, 2
The line chart created with MS Excel is attached.

Answer:
3/4, 1 1/2, 2, 1 1/4, 3/4, 2, 1 1/2, 1 1/4, 1 1/2, 1/2, 1
In the question, it is required to create a line plot to represent the data
The given data can be sorted in ascending order in decimal form as follows;
0.5, 0.75, 0.75, 1, 1.25, 1.25, 1.5, 1.5, 1.5, 2
Step-by-step explanation:
been having this question for a while and this app wont help

Answers
The answer is B
When there is a solid point that means it is included in the graph, so you'd use a greater/less than or equal to symbol (the one with a line under). If it's an unfilled point then it means that it is not included in the graph, so you'd use a greater/less than sign.
The x² equation is less than 2 while the linear equation is greater than or equal to 2.
We know it's the x² equation that is less than two because it has a curve in it.
3. Point P(2, -1) is the image of point
P(3, 4) under a translation. What
is the image of (5,2) under the
same translation?
A(4, -1)
B (6,-3)
C(4, -3)
D (-3, 4)
Answers
A pump dispenses 15 gallons of water per minute. How many quarts per second can
the pump dispenses
(1 gal = 4 qts)
Answers
Answer:
1
Step-by-step explanation:
I beileve it would be 1 because if you grab the 15 gallons and mutiply them by 4, then you would get 60. There is 60 seconds in a minute therefore, I would take the quarts and divde them by the seconds. Then I would get my answer of 1 quart per second.
Hope this helped. If you still do not understand I will gladly explain it more. If im wrong, feel free to correct me. :)
ASAP
-WILL MARK BRIANiST

Answers
Answer:
5/18
Step-by-step explanation:
(5/6)/3
5/6 X 1/3
5/18
5/6 x 1/2 = 5/12
An 11 pound turkey costs $15.00, while the 16 pound turkey is on sale for $20.00. How much would you save with the sale price?
Answers
Answer:
I think the answer is 11.7333
In a university, if a student is a business major, then there is 70% chance that he/she will be employed immediately after graduation. And if a student is not a business major, then there is a 35% chance that hel she will be employed immediately after graduation. We also know that 40% of students are business majors and 60% of students are not business majors. What is the probability that a student is a business major given that he or she is employed immediately after graduaton?
Answers
P(Business Major | Employed) = P(Employed | Business Major) * P(Business Major) / P(Employed)
We are given the following probabilities:
P(Employed | Business Major) = 0.70
P(Business Major) = 0.40
P(Employed | Not Business Major) = 0.35
P(Not Business Major) = 0.60
First, we need to find the probability of being employed (P(Employed)):
P(Employed) = P(Employed | Business Major) * P(Business Major) + P(Employed | Not Business Major) * P(Not Business Major)
P(Employed) = (0.70 * 0.40) + (0.35 * 0.60) = 0.28 + 0.21 = 0.49
Now, we can use Bayes' theorem to find the probability that a student is a business major given that they are employed immediately after graduation:
P(Business Major | Employed) = (0.70 * 0.40) / 0.49 ≈ 0.5714
So, the probability that a student is a business major given that they are employed immediately after graduation is approximately 57.14%
the drying times for a certain type of cement are normally distributed with a standard deviation of 62 minutes. a researcher wishes to estimate the mean drying time for this type of cement. find the least sample size needed to assure with 90% confidence that the sample mean will not differ from the population mean by more than 5 minutes.
Answers
The least sample size needed to assure with 90% confidence that the sample mean will not differ from the population mean by more than 5 minutes will be 416.16.
What is normal distribution?A normal distribution is a data set design in which the majority of values cluster around the middle of the range and the remainder taper off symmetrically toward either end. Data in a normal distribution are symmetrically distributed and have no skew. The majority of values cluster around a central location, with values decreasing as one moves out from the center. In a normal distribution, the measures of central tendency (mean, mode, and median) are all the same. The normal distribution, like any other probability distribution, defines how the values of a variable are distributed. Because it properly captures the distribution of values for many natural occurrences, it is the most important probability distribution in statistics.
Here,
The z value at the 90 percent confidence interval is 1.645.
t ≥ z*s/√n
5≥1.645*62/√n
√n≥20.398
√n≥20.4
n≥416.16
The smallest sample size required to ensure that the sample mean does not deviate from the population mean by more than 5 minutes with 90% confidence is 416.16.
To know more about normal distribution,
https://brainly.com/question/17199694
#SPJ4
x(t) = Find a plane containing the point (-5,6,-6) and the line y(t) =
{x(t) = 7 - 5t
{y(t) = 3 - 6t
{z(t) = -6 -6t
Answers
To find a plane containing the point (-5, 6, -6) and the line defined by parametric equations x(t) = 7 - 5t, y(t) = 3 - 6t, and z(t) = -6 - 6t, we can use the point-normal form of the equation of a plane.
The equation of a plane in point-normal form is given by Ax + By + Cz + D = 0, where (A, B, C) is the normal vector to the plane, and (x, y, z) are the coordinates of a point on the plane. We can determine the normal vector by taking the cross product of two direction vectors in the plane.
The direction vector of the line can be obtained by taking the coefficients of t in the parametric equations, which gives us (-5, -6, -6). We can choose any two non-parallel direction vectors in the plane, for example, (1, 0, 0) and (0, 1, 0). Taking the cross product of these two vectors, we get the normal vector (0, 0, -1).
Now, we can substitute the values of the point (-5, 6, -6) and the normal vector (0, 0, -1) into the point-normal form equation. This gives us 0*(-5) + 0*6 + (-1)*(-6) + D = 0, which simplifies to D = -6. Thus, the equation of the plane containing the point (-5, 6, -6) and the given line is 0*x + 0*y - z - 6 = 0, or simply -z - 6 = 0.
Learn more about point here:
https://brainly.com/question/32083389
#SPJ11
Which of the following equations represents a line that is perpendicular toy = -2x+4 and passes through the point, (4, 2)?
A. y=-3x +2
B. y - x
O C. y - 3x+4
O D. y = -2x
Answers
The point-slope form is y - y₁ = m(x - x₁), where (x₁, y₁) represents the given point and m is the slope. The equation of the line that is perpendicular to y = -2x + 4 and passes through the point (4, 2) is given by option D: y = -2x.
To determine which equation represents a line perpendicular to y = -2x + 4 and passes through the point (4, 2), we need to consider the slope of the given line. The equation y = -2x + 4 is in slope-intercept form (y = mx + b), where the coefficient of x (-2 in this case) represents the slope of the line.
Since we are looking for a line that is perpendicular to this given line, we need to find the negative reciprocal of the slope. The negative reciprocal of -2 is 1/2. Therefore, the slope of the perpendicular line is 1/2.
Now, we can use the point-slope form of a line to find the equation. The point-slope form is y - y₁ = m(x - x₁), where (x₁, y₁) represents the given point and m is the slope.
Substituting the values (4, 2) for (x₁, y₁) and 1/2 for m, we get:
y - 2 = (1/2)(x - 4).
Simplifying this equation, we find:
y - 2 = (1/2)x - 2.
Rearranging the terms, we obtain:
y = (1/2)x.
Therefore, option D, y = -2x, represents the equation of the line that is perpendicular to y = -2x + 4 and passes through the point (4, 2).
Learn more about point-slope form here:
https://brainly.com/question/29503162
#SPJ11
The five values for a data set are: minimum = 0 lower quartile = 2 median = 3. 5 upper quartile = 5 maximum = 10 Bruno created the box plot using the five values. What error did he make? The right whisker should go from 3. 5 to 10. The left whisker should go from 0 to 2. The box should go from 2 to 3. 5. The box should go from 3. 5 to 5
Answers
The five values for a data set are: minimum = 0 lower quartile = 2 median = 3. 5 upper quartile = 5 maximum = 10 Bruno created the box plot using the five values. Bruno made error. The left whisker should go from 0 to 2.
About quartileQuartiles is a type of quartile that divides data into four parts with approximately the same number. The first quartile or lower quartile (Q1) is the middle value between the smallest value and the median of the data group. The first quartile is a marker that the data in that quartile is 25% below the data group.
The second quartile (Q2) is the median data which marks 50% of the data (dividing the data in half). The third or upper quartile (Q3) is the middle value between the median and the highest value of the data set. The third quartile is a marker that the data in that quartile is 75% below the data group. Quartiles are a form of an ordered statistic because to determine quartiles, data needs to be sorted from smallest to largest value first.
Learn more about quartile at https://brainly.com/question/24329548.
#SPJ4
my question needs an answer, and quick!

Answers
Answer:
If he worked 7 hours on Thursday, he would earn 63 dollars.
Step-by-step explanation:
4 hours : 36 + 9 = 45
5 hours: 45 + 9 = 54
6 hours: 54 + 9 = 63
63 dollars is your answer.
Answer:
Hope you don't mind me answering your latest question here. One of those fake link people answered and I cant answer now ugh but I believe the answer is 1.1 I dont know for sure though so please answer at your own risk Hope that helps!
PLEASE ANSWER ASAP !!!

Answers
Answer:
the midpoint would be:(0,5)
Step-by-step explanation:
How to reduce decimals to fractions.
Answers
Decimals can be written in fraction form. To convert a decimal to a fraction, place the decimal number over its place value. For example, in 0.6, the six is in the tenths place, so we place 6 over 10 to create the equivalent fraction, 6/10. If needed, simplify the fraction.
Calculate the future value of a three year uneven cash flow given below, using 11% discount rate:
Year 0 Year 1 Year 2 Year 3
0 $600 $500 $400
Answers
Therefore, the future value of a three-year uneven cash flow given below, using an 11% discount rate is $1,238.82.
To calculate the future value of a three-year uneven cash flow given below, using an 11% discount rate, we need to use the formula;
Future value of uneven cash flow = cash flow at year 1/(1+discount rate)¹ + cash flow at year 2/(1+discount rate)² + cash flow at year 3/(1+discount rate)³ + cash flow at year 4/(1+discount rate)⁴
Given the cash flows;
Year 0: $0
Year 1: $600
Year 2: $500
Year 3: $400
Then the Future value of uneven cash flow
= $600/(1+0.11)¹ + $500/(1+0.11)² + $400/(1+0.11)³
= $600/1.11 + $500/1.23 + $400/1.36
=$540.54 + $405.28 + $293.00
=$1,238.82
Therefore, the future value of a three-year uneven cash flow given below, using an 11% discount rate is $1,238.82.
To know more about discount rate visit:
https://brainly.com/question/13660799
#SPJ11
Can someone answer asapppppp!!!!

Answers
Answer:
1°
Step-by-step explanation:
There are 360 degrees in a circle.
Thus, if a circle turns x degrees, then it will represent x/360 of the entire circle.
Since the rotation represented 1/360 of the entire circle, this means that the measure of the angle must be only 1 degree.
Answer:180
Step-by-step explanation:
An angle turns through n one degree angles is said to have an angle measure of n degrees
for each of the following, determine whether the item would be on the asset side of the feds balance sheet
Answers
Determining whether an item belongs on the asset side of the Federal Reserve's balance sheet depends on the nature of the item. Assets that would be found on the Federal Reserve's balance sheet include cash, securities, loans, and property.
If it represents a valuable resource that the Federal Reserve owns, controls, or expects to receive economic benefits from in the future, it is likely to be classified as an asset. Examples of assets that would be found on the Federal Reserve's balance sheet include cash, securities, loans, and property.
The Federal Reserve's balance sheet is a financial statement that shows its assets, liabilities, and capital, and is used to monitor the financial health of the institution. The assets side of the balance sheet represents the resources that the Federal Reserve owns, controls, or expects to receive economic benefits from in the future. Examples of assets that would be found on the Federal Reserve's balance sheet include cash, securities, loans, and property.
Cash is a liquid asset that the Federal Reserve holds to meet the liquidity needs of the banking system. It includes both physical currency and electronic reserves held by banks at the Federal Reserve. Securities represent investments that the Federal Reserve holds in various forms, including Treasury securities, mortgage-backed securities, and agency debt. Loans are assets that the Federal Reserve makes to depository institutions, such as banks, in order to support their lending activities. Lastly, property represents the real estate and other physical assets that the Federal Reserve owns, such as buildings and equipment.
Overall, whether an item belongs on the asset side of the Federal Reserve's balance sheet depends on the nature of the item and whether it represents a valuable resource that the institution owns, controls, or expects to receive economic benefits from in the future.
Complete Question:
For each of the following, determine whether the item would be on the asset side of the feds balance sheet.
Learn more about Balance:
brainly.com/question/28699225
#SPHJ11
In each room shown, you plan to put down carpet and add a wallpaper border around the ceiling. Which room needs more carpeting? more wallpaper?
Room A or B needs more carpeting.
Room A or B needs more wallpaper.



Answers
Answer:
See below ~
Step-by-step explanation:
Room A
Area (carpeting) = 10 × 11 + 6 × 4 = 110 + 24 = 134 ft²Perimeter (wallpaper) = 10 + 2(11) + 2(4) + 6 = 16 + 22 + 8 = 46 ftRoom B
Area (carpeting) = 12 × 8 + 1/2 × 3.14 × 6² = 96 + 3.14 × 18 = 96 + 56.52 = 152.52 ft²Perimeter (wallpaper) = 12 + 2(8) + 3.14 × 6 = 28 + 18.84 = 46.84 ftRoom A needs more carpeting.
Room A needs more wallpaper.
Let f(x) = (x^2 - 4x)e^z on [0,4]
F (0) = F (4) = Find c such that f'(c) = 0 or if Rolle's Theorem does not apply, enter DNE.
c =
Answers
There exists a value c = 2 such that f'(c) = 0 according to Rolle's Theorem.
To find the value of C:
Let f(x) = (x^2 - 4x)e^z on [0,4].
We are asked to find c such that f'(c) = 0 according to Rolle's Theorem, or if it does not apply, enter DNE.
First, let's verify if Rolle's Theorem applies.
The conditions for Rolle's Theorem are:
1. The function is continuous on the closed interval [0, 4].
2. The function is differentiable on the open interval (0, 4).
3. f(0) = f(4).
Since f(x) = (x^2 - 4x)e^z is a product of a polynomial and an exponential function,
it is continuous and differentiable on its entire domain.
Thus, conditions 1 and 2 are satisfied.
Now, let's check condition 3:
f(0) = (0^2 - 4*0)e^z = 0
f(4) = (4^2 - 4*4)e^z = (16 - 16)e^z = 0
Since f(0) = f(4), all conditions for Rolle's Theorem are satisfied.
Now, we need to find f'(x) and set it equal to 0.
Step 1: Differentiate f(x) using the product rule, which states that (uv)' = u'v + uv'.
u = x^2 - 4x
v = e^z
u' = 2x - 4
v' = 0 (since z is a constant)
f'(x) = (2x - 4)e^z + (x^2 - 4x)*0 = (2x - 4)e^z
Step 2: Set f'(x) equal to 0 and solve for x.
(2x - 4)e^z = 0
2x - 4 = 0
2x = 4
x = 2
Thus, there exists a value c = 2 such that f'(c) = 0 according to Rolle's Theorem.
To know more about Rolle's Theorem:
https://brainly.com/question/13972986
#SPJ11
A 2-ft wide circular track for a camera dolly is set up for a movie scene. The two rails of the track form concentric circles. The radius of the
inner circle is 50 ft. How much farther does a wheel on the outer rail travel than a wheel on the inner rail of the track in one turn?
The wheel on the outer rail travels ___ feet farther than the wheel on the inner rail of the track in one turn.
(Round to the nearest tenth as needed.)
Answers
In a single round, the wheel on the outer rail of the track moves 12.56 feet farther than the wheel on the inner rail.
Calculating the distance between the circumferences of the two circles will allow us to determine how much further a wheel on the outer rail moves than a wheel on the inner rail does in a single turn.
The formula provides the circumference of a circle. C = 2πr, where C is the circumference and r is the radius of the circle.
The circumference of the inner circle with a radius of 50 feet is:
C_inner = 2π(50) = 100π ft.
By multiplying the radius of the inner circle by the track's width (2 feet), we can determine the radius of the outer circle:
Radius_outer = 50 + 2 = 52 ft.
The outer circle's circumference is thus:
C_outer = 2π(52) = 104π ft.
By deducting the inner circle's circumference from the outer circle's circumference, we may calculate the difference in distance travelled:
C_diff = C_outer - C_inner
= 104π - 100π
= 4π ft.
To get the numerical value, we can approximate π to 3.14:
C_diff ≈ 4(3.14)
= 12.56 ft.
For more such question on round. visit :
https://brainly.com/question/24326172
#SPJ8
Find the modulus, argument and principal value of
\(z = \sqrt{ \frac{1 + i}{1 - i} } \)
Answers
The modulus is 1, argument is π/4 and the principal value is π/4 mod 2π
How to determine the modulus, argument and principal valueFrom the question, we have the following parameters that can be used in our computation:
\(z = \sqrt{ \frac{1 + i}{1 - i}\)
Rationalize the expression
So, we have
\(z = \sqrt{ \frac{1 + i}{1 - i} = \frac{(1 + i)(1 + i)}{(1 - i)(1 + i)} = \frac{1 + 2i + i^2}{1 - i^2} = \frac{1 + 2i - 1}{1 + 1} = \frac{2i}{2} = i\)
This means that
z = √i
The modulus of a complex number is calculated as
|z| = √(a^2 + b^2)
where z = a + bi. In this case, a = 0 and b = 1
So, we have
|z| = √(0^2 + 1^2)
|z| = 1
The argument of a complex number is calculated as
\(\arg(z) = \tan^{-1} \left( \frac{b}{a} \right)\)
So, we have
\(\arg(z) = \tan^{-1} \left( \frac{1}{0} \right)\)
This gives
arg(z) = π/4
The principal value of the argument of z is
arg(z) mod 2π
So, we have
π/4 mod 2π
Read more about complex numbers at
https://brainly.com/question/28007375
#SPJ1
Solve by factoring: x2+3x-18=0
Answers
Answer:
x^2 +3x- 18=0
x^2 +6x -3x - 18 =0
x( x+6) - 3(x +6) =0
(x-3) + ( x+6) =0
therefore, x= -6,3
Solve the equations X = -6 X = 3 The equation has 2 solutions Solution
2 X + 6x-3x- 18 = 0 Factor the expressions xx (x + 6) -3 (x + 6) = 0 Factor the expression (x + 6) x (x-3) = 0 Separate into possible cases X + 6 = 0 X-3 = 0 Solve the equations X = -6 X = 3 The equation has 2 solutions Solution X4 = -6, x, = 3>

PLS HELP WILL MARK BRAINLIEST

Answers
The correct answer is A because 5 divided by $1.50 gives you 30 cents! if you divide any of the number of goldfish by the price it gives you $.30. Please mark brainliest
What is the range of the function f(x) = |x – 3| + 4?
R: {f(x) ∈ ℝ | f(x) ≥ 4}
R: {f(x) ∈ ℝ | f(x) ≤ 4}
R: {f(x) ∈ ℝ | f(x) > 7}
R: {f(x) ∈ ℝ | f(x) < 7}
Answers
Answer: R: {f(x) ∈ ℝ | f(x) ≥ 4}
Step-by-step explanation:
\(|x-3| \geq 0\) for all real x, so the range is \(f(x) \geq 4\).
A baker has 130 cookies to decorate for a charity event. He can decorate 10 cookies per hour. Which equation represents the number of cookies, y, that the baker still needs to decorate after x hours?
A. y = 10x - 130
B. y = 10x +130
C. y = -10x - 130
D. y = -10x + 130
Answers
solve x+8=3x wefsdf eferc4frtvtgervgbeg4t
Answers
Answer:
x = 4
Step-by-step explanation:
x + 8 = 3x
8 = 2x
x = 4
So, the answer is x = 4
X - 3x = -8
Collect terms
-2x= -8
Solution:
X= 4
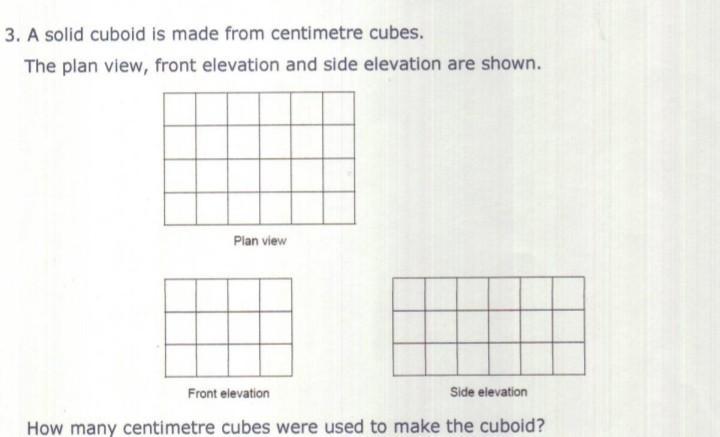
a solid cuboid is made from centimeter cubes how many centimeter cubes were used to make the cuboid
Answers
This question is incomplete because it lacks the appropriate diagram.
Please find attached this answer the appropriate diagram.
Kindly note that: The diagram was obtained from or referenced from Planes and Elevation (F) Version 3 January 2016.
Answer:
72 centimeter cubes
Step-by-step explanation:
Looking at the attached diagram, we are given 3 views of the cuboid
a) The plan view: The is the view of the cuboid from the top or a very high position
b) Front Elevation view: This is the view of the cuboid from the front
c) Side elevation view: This is the view of the cuboid from the side.
In the referenced and attached diagram,
The plan view shows us the top of the cuboid with a length of 4 and breadth of 6
The front elevation view shows us the front of the cuboid with a length of 3 and breadth of 4
The side elevation view shows us the side of the cuboid with a length is 3 and breadth of 6
We are told to find the number of centimeter cubes were used to make the cuboid. This means we are to find the volume of the cuboid.
Volume of a cuboid = Length × Width × Height
Combining the 3 views together,
The Length of the cuboid = 4
Width of the cuboid = 6
Height of the cuboid = 3
Volume of the cuboid = 4 × 6 × 3
= 72
Therefore, the number of centimeter cubes that were used to make the cuboid is 72.
Apartide moving along the z-axs has is postion deteribed by the function x=(5t
3
+2t+5)m, where fishs. At t−4 n, what is the partices position? You may want to reviow (Pogns 56−461 Expeess your answer to three slaniticant figures and inclode the approperate units. For help wath math veils you may want to review. View Anvallable 1fint(s) Disseentiation of Pohnowitationctions Part B Expess your answer to three slanificant figures sod inchade the appropriate units. - View Avaliable Hineiso) - Part C o Viow Areilable Hine(v)
Answers
A. At t = -4, the particle's position is -323 meters.
B: The velocity of the particle at t = -4 is 242 m/s.
C: The acceleration of the particle at t = -4 is -120 m/s².
Part A: The position of the particle is given by the function \(x = 5t^3 + 2t + 5\). We need to find the particle's position at t = -4.
Substituting t = -4 into the position function:
\(x = 5(-4)^3 + 2(-4) + 5 \\x = 5(-64) - 8 + 5 ] \\x = -320 - 8 + 5 \\x = -323\)
Therefore, at t = -4, the particle's position is -323 meters.
Part B: Find the velocity of the particle at t = -4.
To find the velocity, we need to take the derivative of the position function with respect to time (t).
Given position function: \(x = 5t^3 + 2t + 5\)
Taking the derivative:
\(v = dx/dt = d/dt(5t^3 + 2t + 5) \\v = 15t^2 + 2\)
Substituting t = -4 into the velocity function:
\(v = 15(-4)^2 + 2 \\v = 15(16) + 2 \\v = 240 + 2\\v = 242\)
Therefore, the velocity of the particle at t = -4 is 242 m/s.
Part C: Find the acceleration of the particle at t = -4.
To find the acceleration, we need to take the derivative of the velocity function with respect to time (t).
Given velocity function: \(v = 15t^2 + 2\)
Taking the derivative:
\(a = dv/dt = d/dt(15t^2 + 2) \\a = 30t\)
Substituting t = -4 into the acceleration function:
\(a = 30(-4) \\a = -120\)
Therefore, the acceleration of the particle at t = -4 is -120 \(m/s^2\).
Learn more about velocity and accelaration: https://brainly.com/question/25876659
#SPJ11