round to the nearest hundredth . 7.428
Answers
Answer:
7.43
hope this helps
have a good day :)
Step-by-step explanation:
Answer:
7.43
Step-by-step explanation:
So lets go over a few things:
In decimal form, you start of in the tenths.
So in this case that is 4.
After tenths, is hundreths, thousandths, ten thousandths, etc.
Since hundreths comes after the tenths, that will be 2.
Any number 5 or greater will be rounded up, and any number below 5 is rounde down.
Now, to find if we raise or lower the hundreths(which is 2), we must see if the thousandth splace is above or lower than 5.
We can see that our thousandths is 8.
Since 8 is higher than 5, the 2 in our hundreths place will go up to 3.
So we get:
7.43
Hope this helps!
Related Questions
a game is played with a circular spinner that contains 7 different colors. the design of the spinner is the order in which the colors are arranged. how many ways can this spinner be designed
Answers
Answer:
This spinner can be designed in 5040 ways.
Step-by-step explanation:
Number of possible arrangements:
The number of possible arrangements of n elements is given by:
\(A_{n} = n!\)
In this question:
7 colors, so:
\(A_{7} = 7! = 5040\)
This spinner can be designed in 5040 ways.
The word isometric can be broken into two parts. The prefix "iso-” means "of the same,” and "-metric” means "measure.” How does the meaning of the word isometric relate to determining if an isometric transformation occurred? Include the defining characteristics of angle measure and line segments in your response.
Answers
The term "isometric" has the Greek roots "isos," which means "same," and "metron," which means "measure." The definition of an isometric transformation is one in which the original figure and its transformed equivalent have the same shape, size, and orientation.
When we speak about geometric figures, the concept of shape, size, and orientation come into play.The defining characteristics of angle measure and line segments play a critical role in determining whether an isometric transformation has occurred. In geometry, angle measures are the measurements of angles in a geometric figure. An angle is formed by two line segments that share a common endpoint. It is a unit used to calculate the measure of a plane figure's interior or exterior, such as a polygon. In other words, the size of the angle doesn't change during an isometric transformation.Line segments are the building blocks of geometric figures. They are used to construct geometric figures such as polygons, triangles, and rectangles, among others. In an isometric transformation, the length of the line segments remains constant because the shape and size of the original figure and its transformed equivalent remain the same.In conclusion, the word "isometric" implies that the transformation has the same measurements of the original figure. It is a transformation that retains the original geometric figures' shape, size, and orientation. The defining characteristics of angle measure and line segments remain unchanged during the isometric transformation. This means that if an isometric transformation occurs, the original and transformed figures have the same measurements of angles and line segments.For such more question on isometric
https://brainly.com/question/14486229
#SPJ8
Represent 2x + 3y = 6 by a graph. Write the coordinates of the point where it meets: (a) x-axis
Answers
The point where the line 2x + 3y = 6 intersects the x-axis is (3, 0). This means that when x is equal to 3, y is equal to 0.
To graph the equation 2x + 3y = 6, we can rewrite it in the slope-intercept form, y = mx + b, where m represents the slope and b represents the y-intercept.
Starting with the given equation, we isolate y to one side:
3y = -2x + 6
y = (-2/3)x + 2
Now, we have the equation in slope-intercept form, y = (-2/3)x + 2. The slope is -2/3, and the y-intercept is (0, 2).
To find the point where the graph intersects the x-axis, we need to determine the coordinates where y is equal to zero. This occurs when the line crosses the x-axis.
Setting y = 0 in the equation, we have:
0 = (-2/3)x + 2
(-2/3)x = -2
x = (-2)(-3/2) = 3
Therefore, the point where the line 2x + 3y = 6 intersects the x-axis is (3, 0). This means that when x is equal to 3, y is equal to 0, indicating the point of intersection with the x-axis on the graph.
To more on Graph:
https://brainly.com/question/19040584
#SPJ8
5/8 = x : 8 (Any / means fraction)
Answers
Answer:
x=5
Step-by-step explanation:
I think you are looking for the value of x and you just say that
8*x=5*8
8x=40
x=5
Theprofit(indollars)earnedbysellingwappletreesisrepresentedby w2 − 4w + 14. The profit (in dollars) earned by selling w pear trees is
represented by 2w + 10. Write a polynomial that represents how much more profit is earned by selling w apple trees than w pear trees.
Answers
\(w^2 - 6w + 4\) is a polynomial that represents how much more profit is earned by selling w apple trees than w pear trees.
The profit earned by selling w pear trees is 2w + 10
To find the difference in profit, we need to subtract the profit earned by selling w pear trees from the profit earned by selling w apple trees:
\((w^2 - 4w + 14) - (2w + 10)\)
Simplifying the expression:
\(w^2 - 6w + 4\)
Therefore, the polynomial that represents how much more profit is earned by selling w apple trees than w pear trees is \(w^2 - 6w + 4\).
Learn more about expression here:
https://brainly.com/question/14083225
#SPJ1
Use the to the right the value of PT. T is the midpoint of PQ
PT=7x+5 and TQ=9x-9
Answers
By using the figure below, the value of line segment PT is equal to 54 units.
How to determine the midpoint of a line segment?In Mathematics, the midpoint of a line segment with two endpoints can be calculated by adding each point on a line segment together and then divide by two (2).
Since T is the midpoint of PQ, we have the following:
Line segment PT = Line segment TQ
7x + 5 = 9x - 9
9x - 7x = 9 + 5
2x = 14
x = 14/2
x = 7.
Now, we can determine the value of PT:
PT = 7x + 5
PT = 7(7) + 5
PT = 54 units.
Read more on midpoint here: https://brainly.com/question/17918978
#SPJ1
Complete Question:
Use the figure to the right to find the value of PT. T is the midpoint of PQ PT=7x+9 and TQ=9x-5

A person jogs 1/5 mile in 1/40 hour. The person's speed is (blank) miles per hour
Answers
Answer:
8 miles per hour
Step-by-step explanation:
1/5 x 40 = 8
Decide whether the given number is a solution of the given equation.
Is - 7 a solution of x + 12 = 19?
Answers
Answer:
No
Step-by-step explanation:
-7+12=5
Answer:
\(x=7\)
-7 is not a solution for \(x+12=19\)
Step-by-step explanation:
\(x +12 = 19\)
Subtract 12 from both sides
\(x = 19-12\)
Subtract 12 from 19 to get 7
\(x = 7\)
It would be -7 if it was \(-x+12=19\)
Phyllis invested 32000 dollars, a portion earing a simple interest rate of 5 percent per year and the rest earning a rate of 6 percent per year. After
one year the total interest earned on these investments was 1820 dollars. How much money did she invest at each rate?
Answers
9514 1404 393
Answer:
$10,000 at 5%$22,000 at 6%Step-by-step explanation:
Let x represent the amount invested at 6%. Then 32000-x is the amount invested at 5%, and the total interest earned is ...
0.06x +0.05(32000 -x) = 1820
0.01x +1600 = 1820 . . . . simplify
0.01x = 220 . . . . . . . . . . subtract 1600
x = 22,000 . . . . . . . . . . . multiply by 100
32000 -x = 10,000
Phyllis invested $22,000 at 6% and $10,000 at 5%.
Congruent triangle proofs
Answers
Answer:
SSS (Side Side Side)
The simplest way to prove that triangles are congruent is to prove that all three sides of the triangle are congruent. When all the sides of two triangles are congruent, the angles of those triangles must also be congruent. This method is called side-side-side, or SSS for short.
SAS (Side-Angle-Side)
A second way to prove the congruence of triangles is to show that two sides and their included angle are congruent. This method is called side-angle-side. It is important to remember that the angle must be the included angle--otherwise you can't be sure of congruence. When two sides of a triangle and the angle between them are the same as the corresponding parts of another triangle there is no way that the triangles aren't congruent. When two sides and their included angle are fixed, all three vertices of the triangle are fixed. Therefore, two sides and their included angle is all it takes to define a triangle; by showing the congruence of these corresponding parts, the congruence of each whole triangle follows.
3 1/6 times 1 4/7 in simplest form
Answers
Answer:
Its about 5
Brainliest?
Answer:
209/42
Step-by-step explanation:
3 1/6=19/6
1 4/7=11/7
----------------
(19/6)(11/7)=209/42
i cannot figure this out for the life of me. any help?
Find f(3) for the
piece-wise function.
f(x) =
x+1
X
if x > 0
if x ≤ 0
f(3) = [?]

Answers
Answer:
f(3) = 3 + 1 = 4
Step-by-step explanation:
Since 3 > 0, we need to focus on the part of the function where x > 0. So:
f(3) = 3 + 1 = 4.
2/5 of today's total production run will be bought by
Wal-Mart. 2/3 of the Wal-Mart amount will be sold
trough Sam's Club stores. If the total production
run was 150,000 pounds how many pounds will be
sold through Sam's Club stores?
Answers
Answer:
40,000 pounds
Step-by-step explanation:
2/5 * 150,000 = 60,000
2/3 * 60,000 = 40,000
Find the slope of the line graphed below.
ů
m
5
•
0

Answers
ANSWER: y=-7x+4
In Mr. Thompsons class, 6 of the students are student ambassadors and the other 18 students are not. What is the ratio of Student Ambassador to total Students in Mr. Thompsons class?
Answers
The ratio of Student Ambassadors to total Students in Mr. Thompson's class is 1:4.
Ratios and proportions are two fundamental concepts in mathematics that are used to compare quantities and express relationships between them.
A ratio is a comparison of two quantities that are expressed in the same units. Ratios are typically written in the form of "a:b" or "a to b", where a and b are two quantities being compared. For example, the ratio of the number of boys to the number of girls in a class of 20 students might be 3:2, indicating that there are 3 boys for every 2 girls.
A proportion is a statement that two ratios are equal. Proportions are used to solve problems involving ratios by setting up an equation that equates two ratios.
The total number of students in Mr. Thompson's class is 6 + 18 = 24.
The ratio of Student Ambassadors to total Students is 6:24, which can be simplified by dividing both the numerator and denominator by 6 to get 1:4.
Therefore, the ratio of Student ambassadors to total Students in Mr. Thompson's class is 1:4.
To know more about ratios and proportions follow
https://brainly.com/question/28823316
#SPJ1
what are the x and y intercepts of the linear function given by the equation 2x+5y=-10
Answers
In order to find the x-intercept of the function, you need to evaluate the function for y = 0, so:
\(\begin{gathered} 2x+5y=-10 \\ y=0 \\ 2x+5(0)=-10 \\ 2x=-10 \\ x=-\frac{10}{2} \\ x=-5 \end{gathered}\)So, the x-intercept is x = -5
In order to find the y-intercept of the function, you need to evaluate the function for x = 0, so:
\(\begin{gathered} 2x+5y=-10 \\ x=0 \\ 2(0)+5y=-10 \\ 5y=-10 \\ y=-\frac{10}{5} \\ y=-2 \end{gathered}\)Therefore, the y-intercept is y = -2
In Detroit the high temperatures in degrees Fahrenheit for
five days in January were -12°, -8°, -3°, 6°, -15°. What was the
average temperature for these five days?
Answers
Answer:
-6.4 degrees.
Step-by-step explanation:
The average temperature
= (-12 -8 -3 + 6 - 15) / 5
= -32/5
= -6.4 degrees.
Which choice could be modeled by a linear function?
A. The amount, y, of radioactive material remaining after x years when decay occurs at rate 30% each year.
B. The amount of money, y, In an account after x years earning 4% interest compounded annually.
C. The height, y, of a ball after bouncing x times, if each bounce reaches 2/3 the previous height.
D. The monthly cost, y, to use a cell phone for x minutes at a rate of 4 cents per minute.
Answers
Answer:
D
Step-by-step explanation:
D is linear because it increases the same amount each time
Help please can someone solve this
a = 165/360 × pi10^2
Answers
Answer:
Use a TI84 calculator.
Step-by-step explanation:
There is no way to solve this by hand on paper as the answer is an indefinite number.
P (n), given below, is the price in dollars for n grams of vitamins. P(n)=0.5n+6.4 Complete the following statements. Let P¹ be the inverse function of P. Take x to be an output of the function P. That is, x=P (n) and n=P-¹(x). (a) Which statement best describes P-¹(x)? The reciprocal of the price (in dollars) for x grams of vitamins. The amount of vitamins (in grams) for a price of x dollars. The price (in dollars) for x grams of vitamins. The ratio of the price (in dollars) to the number of grams, x. -1 (b) P(x) = - 1 (c) P (9.2) = log 010 X log No solution 0=0 Ś
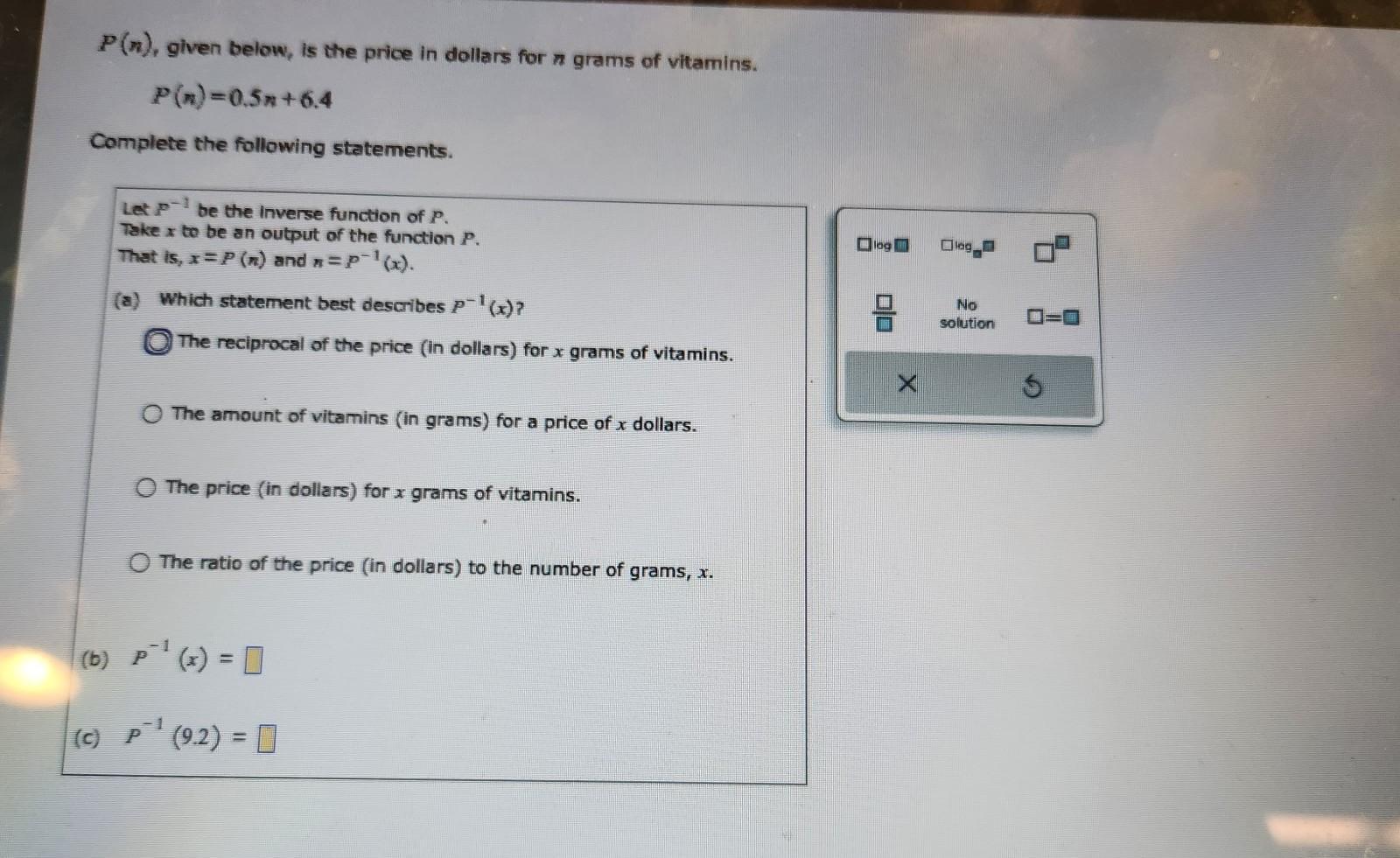
Answers
The number of vitamins (in grams) for a price of x dollars. Then the correct option is B. The inverse function is P⁻¹(x) = (x - 6.4) / 0.50. And at x = 9.2, the value P⁻¹(x) will be 5.4 grams.
What is the inverse function?Let the function f is given as
y = m(x + a) + c
Then the inverse function of the function f will be given by swapping x with y and y with x.
The function is given below.
P(n) = 0.50n + 6.4
The inverse of the function P(n) is given as,
x = 0.50P⁻¹(x) + 6.4
P⁻¹(x) = (x - 6.4) / 0.50
At x = 9.2, then we have
P⁻¹(9.2) = (9.2 - 6.4) / 0.50
P⁻¹(9.2) = 5.6 grams
The number of vitamins (in grams) for a price of x dollars.
More about the inverse function is given below.
https://brainly.com/question/2541698
#SPJ1
The diagram was constructed with straightedge and compass tools.
Select all segments that have the same length as segment AC.
Answers
Answer:
AB and AC
Step-by-step explanation:
Because they are the same length
1500 as a mixed number, please help QUICK
Answers
Answer:
divid 1500 to 50
Step-by-step explanation:
Answer: 1500 0/0
Step-by-step explanation:
Recall that with base-ten blocks: 1 long 10 units, 1 flat 10 longs, and 1 block 10 flats. What is the fewest number of multibase blocks that can be used to represent the corresponding numeral in the given base?
a. 20 longs in base seven
b. 10 longs in base three
Answers
a. The answer is: The fewest number of multibase blocks required to represent 20 longs in base seven is 2 flats.
b. The answer is: The fewest number of multibase blocks required to represent 10 longs in base three is 3 flats and 1 unit.
a. To represent 20 longs in base seven, we need to find the fewest number of multibase blocks required.
In base seven, we have the following conversions:
1 long = 1 unit
1 flat = 10 units
1 block = 10 flats
To represent 20 longs, we can use 2 flats (each flat representing 10 units) and 0 units since there are no remaining units.
So, the fewest number of multibase blocks required would be 2 flats.
Therefore, the answer is: The fewest number of multibase blocks required to represent 20 longs in base seven is 2 flats.
b. To represent 10 longs in base three, we need to find the fewest number of multibase blocks required.
In base three, we have the following conversions:
1 long = 1 unit
1 flat = 3 units
1 block = 3 flats
To represent 10 longs, we can use 3 flats (each flat representing 3 units) and 1 unit since there is one remaining unit.
So, the fewest number of multibase blocks required would be 3 flats and 1 unit.
Therefore, the answer is: The fewest number of multibase blocks required to represent 10 longs in base three is 3 flats and 1 unit.
for such more question on fewest number
https://brainly.com/question/859564
#SPJ8
After drawing a square on the board, Mr. Hinkle asked his class to identify the shape. Timothy stated that Mr. Hinkle had drawn a rhombus. Which of the following statements is true?
Answers
Juliana is taking out an $8,700, 3-year loan with an APR of 2.31%. What will be the monthly payment for this loan? Round to the nearest cent.
Answers
Answer: $250.37
Step-by-step explanation: no clue teacher told me because she doesn't know
Simplify: 3√50+ √24 - 2√72
A. 3√6 +2√2
B. 3√9+2√5
C. 3√2+2√6
D. 10√2+2√6
Answers
Answer:
Step-by-step explanation:
13+(-3)^2+4(-3)+1- {-10-(-6)}
_________________________
[4+5}/ [4^2-3^2(4-3)-8]+12
15/3=5
Given: PS=RT, PQ=ST
Prove: QS=RS
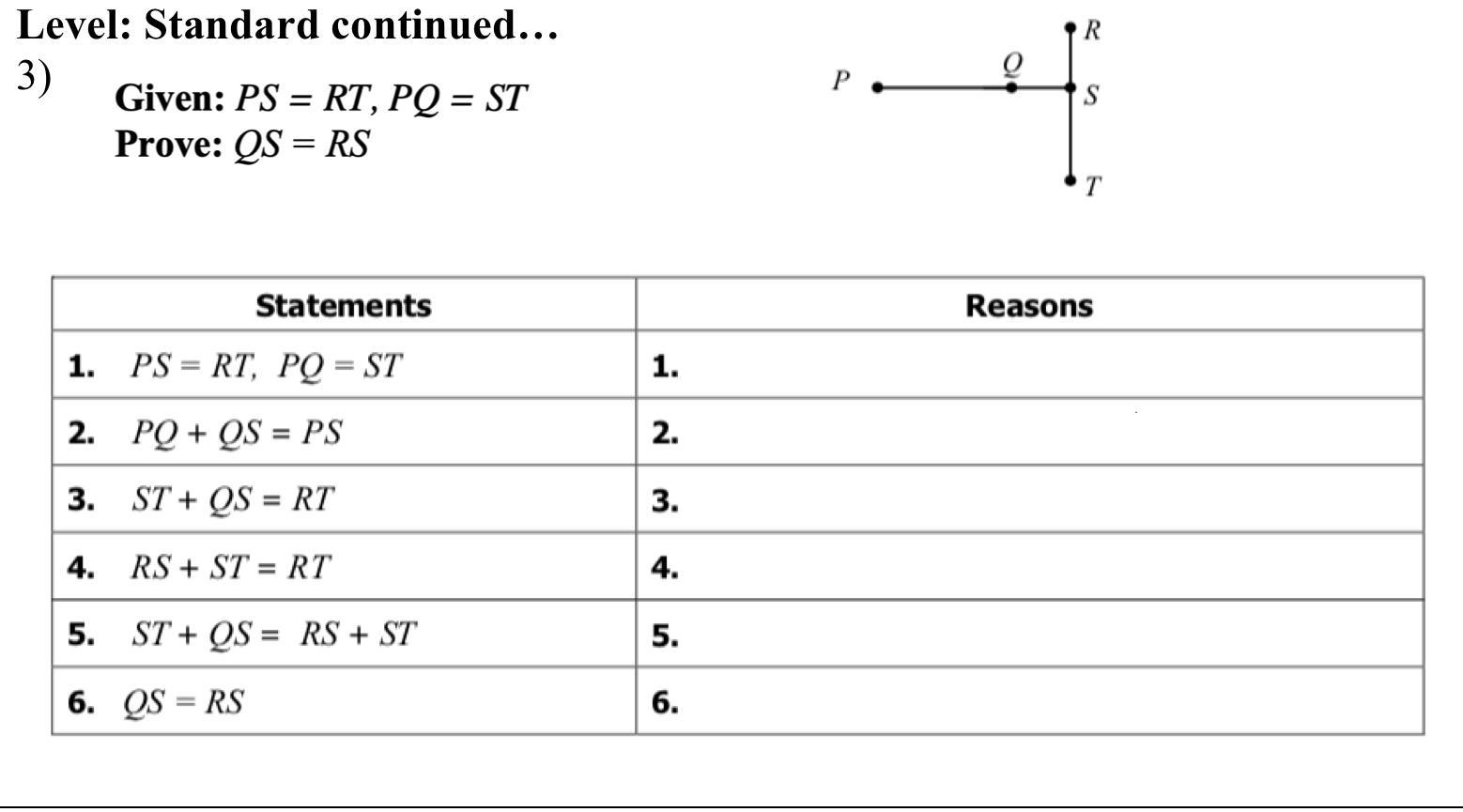
Answers
Answer:
I) Eq(1) reason: sum of segments of a straight line
II) Eq(2) reason: Given PQ = ST & PS = RT
III) Eq(3) reason: sum of segments of a straight line
IV) Eq(4) reason: Same value on right hand sides of eq(2) and eq(3) demands that we must equate their respective left hand sides
V) Eq(5) reason: Usage of collection of like terms and subtraction provided this equation.
Step-by-step explanation:
We are given that;
PS = RT and that PQ = ST
Now, we want to prove that QS = RS.
From the diagram, we can see that from concept of sum of segments of a straight line we can deduce that;
PQ + QS = PS - - - - (eq 1)
Now, from earlier we saw that PQ = ST & PS = RT
Thus putting ST for PQ & PS for RT in eq 1,we have;
ST + QS = RT - - - - (eq 2)
Again, from the line diagram, we can see that from concept of sum of segments of a straight line we can deduce that;
RS + ST = RT - - - - -(eq 3)
From eq(2) & eq(3) we can see that both left hand sides is equal to RT.
Thus, we can equate both left hand sides with each other to give;
ST + QS = RS + ST - - - (eq 4)
Subtracting ST from both sides gives;
ST - ST + QS = RS + ST - ST
This gives;
QS = RS - - - - (eq 5)
Thus;
QS = RS
Proved
what is the perimeter of a parallelogram with length 26cm , 24cm and base 25cm?
Answers
Answer:
Step-by-step explanation:
P=2(a+b)
2(26+25)
=102 ans
Answer:
102 cm
Step-by-step explanation:
P=2(a+b)
P = 2(26+25)
= 2 * 51
= 102
Find the mean, median, and mode(s) of the data. Choose the measure that best represents the data. 31, 35, 2, 32, 27, 35
Please Answer Quickly :^)
Answers
median : 31.5
mode : 35
If f(x) = 2x + 3 and g(x) =4x - 1, find f(4).
Answers
Solve the "f" function with substitute 4 and solve the "g" function with what we get for the "f" function.
f(4) = 2(8) + 3
f(4) = 16 + 3
f(4) = 19
g(19) = 4(19) - 1
g(19) = 76 - 1
g(19) = 75
Best of Luck!