Prove that for any n,m∈N,(10n−1) is not a divisor of (10m+1)
Answers
For any n, m ∈ N, (10n-1) is not a divisor of (10m+1).
To prove this, we can assume the opposite and show that it leads to a contradiction. Suppose (10n-1) is a divisor of (10m+1). This implies that there exists an integer k such that (10n-1)k = (10m+1). Rearranging the equation, we have 10nk - k = 10m + 1.
Looking at the left-hand side of the equation, we notice that 10nk is divisible by 10. However, the term -k is not divisible by 10 since k is an integer. Therefore, the left-hand side of the equation cannot be divisible by 10.
On the other hand, the right-hand side of the equation, 10m + 1, has a remainder of 1 when divided by 10, indicating that it is not divisible by 10.
This contradiction shows that our initial assumption was incorrect. Therefore, (10n-1) cannot be a divisor of (10m+1) for any n, m ∈ N.
Hence, we have proven that for any n, m ∈ N, (10n-1) is not a divisor of (10m+1).
Learn more about divisor here:
https://brainly.com/question/26086130
#SPJ11
Related Questions
3 What is the slope of the line that contains the coordinate points (8,-3) and (-2, 7)?
A -1
B -9/11
C -5/3
D -2/5

Answers
Answer:
c
Step-by-step explanation:
3a=12; a =
b+3.3=8.9; b =
1=14c; c =
512=d+14; d =
2e=6.4; e =
help? Please!
Answers
Answer:
a=4
Step-by-step explanation:
3a=12
a=12/3
a=4
b+3.3=8.9
b=8.9-3.3
b=5.6
1=14c
1/14=14c/14
1/14=c
512=d+14
512-14=d
498=d
2e=6.4
2e/2=6.4/2
e=3.2
A quadrilateral has congruent side lengths. 2 opposite angles have measures of 100 degrees. The other 2 opposite angles have measures of 70 degrees and (5 x) degrees. What is the value of x
Answers
Answer: 18
Step-by-step explanation:
Angles of a quadrilateral add to 360 degrees, so
\(100+100+70+5x=360\\\\270+5x=360\\\\5x=90\\\\x=18\)
Arnold has a picture frame with a width of 8 inches and a height of 6 inches. Which proportion could be used to calculate the dimensions of a smaller frame with a width of 5 inches, that is
similar to the larger one?
8
6
5
2
Answers
Answer:
2
Step-by-step explanation:
5 inches width is 3 less then the original 8. 3 less than 5 is 2. i would say 3 but its not an answer choice.
8. player 1 runs to first base at a speed of 20 ft/s while player 2 runs from second base to third base at a speed of 15 ft/s. let s be the distance between the two players. how fast is s changing when player 1 is 30 ft from home plate and player 2 is 60 ft from second base?
Answers
In linear equation, 90 ft is the distance between the two players .
What in mathematics is a linear equation?
A linear equation is a first-order (linear) term plus a constant in the algebraic form y=mx+b, where m is the slope and b is the y-intercept.Sometimes, the aforementioned is referred to as a "linear equation of two variables," where x and y are the variables.Let player 1 distance from home plate = x ft
so, dx/dt = 20 ft/sec
let player 2 distance from 2nd base = y ft
dy/dt = 15 ft/sec
we know that baseball around is of square type with length 90 ft .
we have to find change in s.
x = 40 ft , y = 50 ft
applying Pythagoras .
S² = (90)² + ( 90 - ( x + y ) )²
S² = ( 90)² + ( 90 - ( 50 + 40 ))²
S² = 90²
S = 90
Learn more about linear equation
brainly.com/question/11897796
#SPJ4
Test the series below for convergence using the Ratio Test. ∑[infinity] to n=1 10^n÷n! The limit of the ratio test simplifies to limn→[infinity]∣f(n)∣ where f(n)=∣a^n+1∣÷∣an∣ f(n)= The limit is: (enter oo for infinity if needed) Based on this, the series Question Help:
Answers
The limit of the ratio test for the series ∑[infinity] to n=1 10^n÷n! is infinity (∞).
The ratio test is used to determine the convergence or divergence of a series. It involves taking the limit of the absolute value of ratio of consecutive terms. If the limit is less than 1, the series converges. If the limit is greater than 1 or infinity (∞), the series diverges. If the limit is exactly 1, the test will be inconclusive.
In this case, we have f(n) = ∣(10^n+1)÷(10^n)∣ = ∣10∣ = 10. The limit of f(n) as n approaches infinity is 10.
Since the limit of f(n) is greater than 1, the series fails the ratio test. This means that the series ∑[infinity] to n=1 10^n÷n! diverges. The ratio test suggests that the series does not have a finite sum and continues indefinitely.
To learn more about divergence click here, brainly.com/question/30726405
#SPJ11
Use -2,-1,0, 1, and 2 for x and find the corresponding values of f(x) for the following exponential function. Then, choose which graph represents the exponentialfunction.f(x)=3(x-1)For each value of x, find the corresponding value for f(x).Xf(x)=3(x-1)-2-1012

Answers
when x = -2, f(x) = 1/27
when x = -1, f(x) = 1/9
when x = 0, f(x) = 1/3
when x = 1, f(x) = 1
when x = 2, f(x) = 3
Explanation:Given:
\(f(x)\text{ = 3}^{x-1}\)To find:
to get the y values for x = -2, -1, 0, 1, and 2
To determine the corresponding values of f(x), we will substitute each of the values into the given function
\(\begin{gathered} f(x)\text{ = 3}^{x-1} \\ when\text{ x = -2} \\ f(x)\text{ = 3}^{-2-1}\text{ = 3}^{-3} \\ f(x)\text{ = }\frac{1}{3^3}\text{ } \\ f(x)\text{ = }\frac{1}{27} \\ \\ when\text{ x = -1} \\ f(x)\text{ = 3}^{-1-1}\text{ = 3}^{-2} \\ f(x)\text{ = }\frac{1}{3^2} \\ f(x)\text{ = }\frac{1}{9} \end{gathered}\)\(\begin{gathered} when\text{ x = 0} \\ f(x)\text{ = 3}^{0-1}\text{ = 3}^{-1} \\ f(x)\text{ = }\frac{1}{3^1} \\ f(x)\text{ = }\frac{1}{3} \\ \\ when\text{ x = 1} \\ f(x)\text{ = 3}^{1-1}\text{ = 3}^0 \\ f(x)\text{ = 1} \end{gathered}\)\(\begin{gathered} when\text{ x = 2} \\ f(x)\text{ = 3}^{2-1}\text{ = 3}^1 \\ f(x)\text{ = 3} \end{gathered}\)Help plz it’s urgent

Answers
Answer:
3. 18 + 18 + 7 + 7 = 50
4. 120 degrees
Step-by-step explanation:
CAN SOMEONE HELP ME PLEASE ASAP!?

Answers
Answer:
false
Step-by-step explanation:
Dilations are transformations that generate an enlargement or a reduction. Translations are congruence transformations that move an object, without changing its size or shape.
this transformation here is a translation.
all points have been moved by +1 in x direction and +2 in y direction.
for a dilation the should have been a multiplication factor and not adding constants.
Olivia planted 25 tomato plants but only 20 produce tomatoes what percentage of the plants did not produce tomatoes?
Answers
answer:
1.25 %
explanation:
25 - 20 = 5 (5 plants didn't produce)
25 ÷ 100 × 5 = 1.25 %
Substitution:
2x - y = 6
x + y = -3
Answers
Answer:
4 and for x+y is 2+1
Step-by-step explanation:
hi! please help i’ll give brainliest

Answers
Answers:
deposition of eroded materials
Step-by-step explanation:
PLEASE HELP... PLEASE

Answers
Answer:
So I am honestly not sure what THEY though when they did this.
Step-by-step explanation:
So when you look at this, we see that:
3x+3y=10
2x+3y=20
Lets just compare these two.
There is only two differences
There is 1 less x, or -1x in the bottom equation.
The number value is 10 more, or +10, in the bottom equation.
This must mean that -x=10
Or
x=-10
This is my way of doing it, their way of doing this is:
Combining the two equations 3x+3y=10 and 2x+3y=20
lets work through this their way and find our mistake.
So combining them we get:
5x-6y=30
Oh...
Well, I see their mistake, do you?
This is our equation:
5x+6y=30
Their equation:
5x=30
The difference is that they though they needed to subtract 3 y from 3y, canceling the two y variables out. When really, you need to combine the two y values.
So this is our REASONING.
Now, you cannot solve it this way further, since you have two variables.
However, using the method above, we were able to find that x=-10
Hope this helps! :)
Discrete math
Prove or disprove each statement:
a) If g: X→Y and h: Y→Z, then if h ◦ g is onto, then g must be
onto.
b) If g: X→Y and h: Y→Z, then if h ◦ g is onto, then h must be
onto.
Answers
h◦g is not onto,
Discrete Math: Prove or Disprove Statementa) If g: X→Y and h: Y→Z, then if h ◦ g is onto, then g must be onto.If h◦g is onto, then h is onto. Therefore, g may not be onto. This statement is false and can be disproven by using the counterexample: let X={1,2} and Y={2,3} and Z={3,4}.
Define g: X→Y by g(1)=2 and g(2)=3, and h: Y→Z by h(2)=3 and h(3)=4. We can show that h◦g is onto by verifying that for all z∈Z, there exists x∈X such that (h◦g)(x)=h(g(x))=z.For instance, when z=4, we need to find x∈X such that (h◦g)(x)=4. We observe that there is no such x, since (h◦g)(1)=h(2)=3 and (h◦g)(2)=h(3)=4.
Therefore, h◦g is onto, but g is not onto.b) If g: X→Y and h: Y→Z, then if h ◦ g is onto, then h must be onto.Similar to Part (a), we can disprove this statement by providing a counterexample. Let X={1,2} and Y={1,2,3}, and Z={1,2,3,4}. Define g: X→Y by g(1)=1 and g(2)=2, and h: Y→Z by h(1)=2, h(2)=3, and h(3)=4.
We can show that h◦g is onto by verifying that for all z∈Z, there exists x∈X such that (h◦g)(x)=h(g(x))=z. For instance, when z=4, we need to find x∈X such that (h◦g)(x)=4. We observe that there is no such x, since (h◦g)(1)=h(1)=2 and (h◦g)(2)=h(2)=3. Therefore, h◦g is not onto, and the statement is disproven.
Know more about Discrete Math here,
https://brainly.com/question/27990704
#SPJ11
Look at the following graph and determine the slope of the line. AY 10 7 5 3 2 1 х 1 2 3 4 5 6 7 8 9 8 9 10
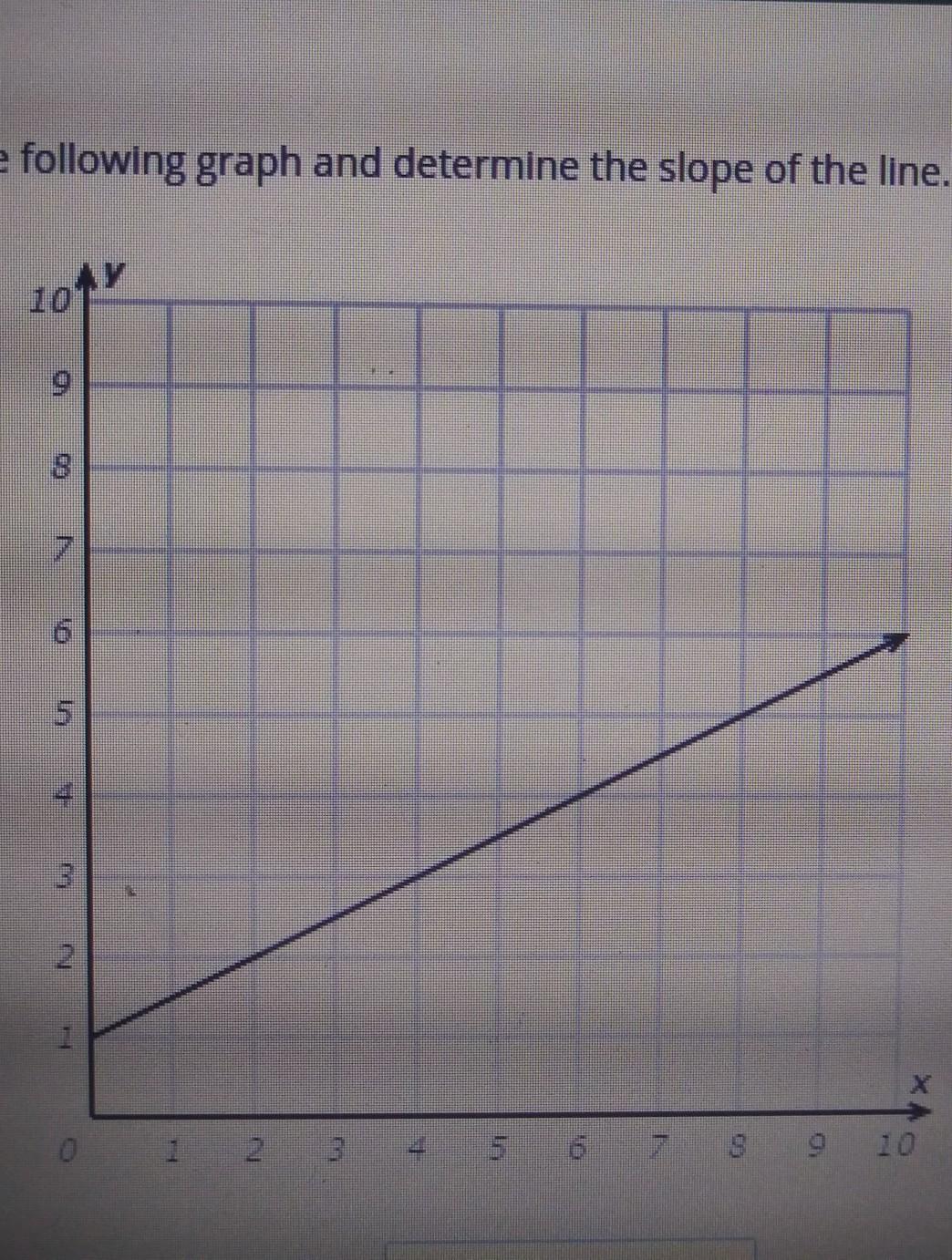
Answers
Answer:
I believe the slope is 2/3.
A boat leaves the dock and travels 9 miles due south, then 12 miles due west. How far is the boat from the dock A. 15 mi B. 21 mi C. 3 mi D. 225 mi
Answers
Answer:
21 miles which is b
Step-by-step explanation:
no other answer makes sense besides that one.
The equation of an ellipse is
4 x²+9 y²+8 x-54 y+49=0
a. Write the equation in standard form. Show your work.
Answers
The equation of the given ellipse, 4x² + 9y² + 8x - 54y + 49 = 0, can be transformed into standard form by completing the square for both the x and y terms.
The standard form of an ellipse equation is (x-h)²/a² + (y-k)²/b² = 1, where (h, k) represents the center of the ellipse, and 'a' and 'b' are the lengths of the major and minor axes.
To convert the equation 4x² + 9y² + 8x - 54y + 49 = 0 into standard form, we need to complete the square for both the x and y terms. Let's begin by rearranging the equation:
4x² + 8x + 9y² - 54y + 49 = 0
Next, we focus on completing the square for the x terms. We take half the coefficient of x (which is 4) and square it, then add and subtract that value inside the parentheses:
4(x² + 2x + 1) + 9y² - 54y + 49 - 4 = 0
Simplifying further:
4(x + 1)² + 9y² - 54y + 45 = 0
Now, we complete the square for the y terms. We take half the coefficient of y (which is -54/9 = -6) and square it, then add and subtract that value inside the parentheses:
4(x + 1)² + 9(y² - 6y + 9) + 45 - 36 = 0
Simplifying once more:
4(x + 1)² + 9(y - 3)² + 9 = 0
To obtain the standard form of an ellipse equation, we divide the entire equation by the constant on the right side (which is 9):
(x + 1)²/9 + (y - 3)²/1 = 1
Thus, the equation is now in standard form, where the center of the ellipse is (-1, 3), the length of the major axis is 2 times the square root of 9 (which is 6), and the length of the minor axis is 2 times the square root of 1 (which is 2).
Learn more about Ellipse here :
brainly.com/question/20393030
#SPJ11
A vector field F has the property that the flux of F out of a small cube of side 0.01 centered around the point (2, 7, 9) is 0.0015. Estimate divF at the point (2, 7, 9).
Answers
By the Divergence Theorem, the flux of a vector field F through a closed surface S is equal to the volume integral of the divergence of F over the region enclosed by S. That is,
∬S F · dS = ∭V (div F) dV
where ∬S denotes the surface integral over S, and ∭V denotes the volume integral over V.
In this problem, we are given that the flux of F out of a small cube of side 0.01 centered around the point (2, 7, 9) is 0.0015. Let's call this cube C. Then, by the Divergence Theorem,
∬S F · dS = ∭V (div F) dV
where S is the boundary surface of C, and V is the volume enclosed by C.
Since the cube C is small, we can approximate its volume as (0.01)^3 = 0.000001. We are also given that the flux of F out of C is 0.0015. Therefore,
∭V (div F) dV = 0.0015
We want to estimate div F at the point (2, 7, 9). Let's call this point P. We can choose C to be a small cube centered around P, say with side length 0.1. Then, by the Divergence Theorem,
∬S F · dS = ∭V (div F) dV
where S is the boundary surface of C, and V is the volume enclosed by C.
Since C is small, we can assume that the value of div F is approximately constant over the region enclosed by C. Therefore,
(div F) ∭V dV ≈ (div F) V
where V is the volume of C. We can use this approximation to estimate div F at P as follows:
(div F) ≈ ∬S F · dS / V
where S is the boundary surface of C.
Since C is centered at (2, 7, 9) and has side length 0.1, its vertices are at the points (1.95, 6.95, 8.95), (2.05, 6.95, 8.95), (1.95, 7.05, 8.95), (2.05, 7.05, 8.95), (1.95, 6.95, 9.05), (2.05, 6.95, 9.05), (1.95, 7.05, 9.05), and (2.05, 7.05, 9.05). We can use these points to estimate the surface integral ∬S F · dS as follows:
∬S F · dS ≈ F(P) · ΔS
where ΔS is the sum of the areas of the faces of C, and F(P) is the value of F at P. Since C is small, we can assume that F is approximately constant over the region enclosed by C. Therefore,
F(P) ≈ (1/8) ∑ F(xi)
where the sum is taken over the eight vertices xi of C.
We are not given the vector field F explicitly, so we cannot compute this sum. However, we can use the fact that the flux of F out of C is 0.0015 to estimate the value of ∬S F · dS. Specifically, we can assume that F is approximately constant over the region enclosed by C, and that its value is equal to the flux density.
Learn more about flux here:
https://brainly.com/question/14527109
#SPJ11
Solve the next problem. (Round your answers to two decimal places). Find the critical value z(alpha/2) that corresponds to a 93% confidence level. 1.66 02.11 O 1.42 1.81
Answers
The critical value z(alpha/2) that corresponds to a 93% confidence level is 1.81. This means that when constructing a confidence interval, the margin of error will be determined by the value of 1.81.
To explain further, a confidence level of 93% indicates that we are confident that the true population parameter lies within the calculated confidence interval 93% of the time in repeated sampling.
The critical value z(alpha/2) represents the number of standard deviations from the mean that encompasses the desired confidence level. For a two-tailed test like this, we divide alpha (1 - confidence level) by 2 to find the tail area for each side of the distribution.
Looking up this tail area in a standard normal distribution table, we find the critical value of 1.81, which captures 93% of the area under the curve.
Learn more about Standard deviations click here :brainly.com/question/25639778
#SPJ11
what is the largest possible area for a right triangle in which the sum on the lengths of the two shorters sides is 1q00 in
Answers
The largest possible area for the right triangle is 1250 in² in which the sum on the lengths of the two shorter sides is 100 in.
we know that in a right triangle the two shorter sides are the base and the perpendicular. Here we have to find the largest possible area of the triangle in which the sum of the shorter sides is 100 in.
Let the base and perpendicular be x and y respectively.
Therefore,
x+y = 100
y = 100 -x
Also, Area A
= 1/2 xy
= 1 x(100-x)/z
= 100x-x²/2
so the coordinate of vertex = -b/2a
= - 50/2(1-1/2)
= 50
Also,
y = 100-50 [Putting the value of x in equation 1]
= 50
Therefore Maximum, area:
= 1/2 (50)(50)
= 1250 in²
Hence we get the required maximum area.
Learn more about Right triangle here:
brainly.com/question/2217700
#SPJ4
What is the simplified product? 2 3/8×5
Answers
Answer:
Simplify the expression.
Exact Form: 95 /8
Decimal Form: 11.875
Mixed Number Form: 11 and 7 /8
Step-by-step explanation:
Answer:
11 7/8
Step-by-step explanation:
Asymmetric encryption algorithm can be used to ensure the integrity of a file's contents. T/F
Answers
False. Asymmetric encryption algorithm can be used to ensure the integrity of a file's contents.
Asymmetric encryption algorithms, such as RSA, are primarily used for data confidentiality and authentication, not for ensuring the integrity of a file's contents. They provide a way to securely exchange encrypted messages between parties and verify the authenticity of the sender.
To ensure the integrity of a file's contents, techniques such as cryptographic hash functions or digital signatures are used. Cryptographic hash functions generate a fixed-size hash value that uniquely represents the file's contents. Comparing the hash value before and after transmission can verify if the file has been tampered with. Digital signatures, on the other hand, use asymmetric encryption to provide a means of verifying the integrity and authenticity of a file by attaching a digital signature created with the sender's private key.
Know more about encryption algorithm here:
https://brainly.com/question/28283722
#SPJ11
anybody know this at all?
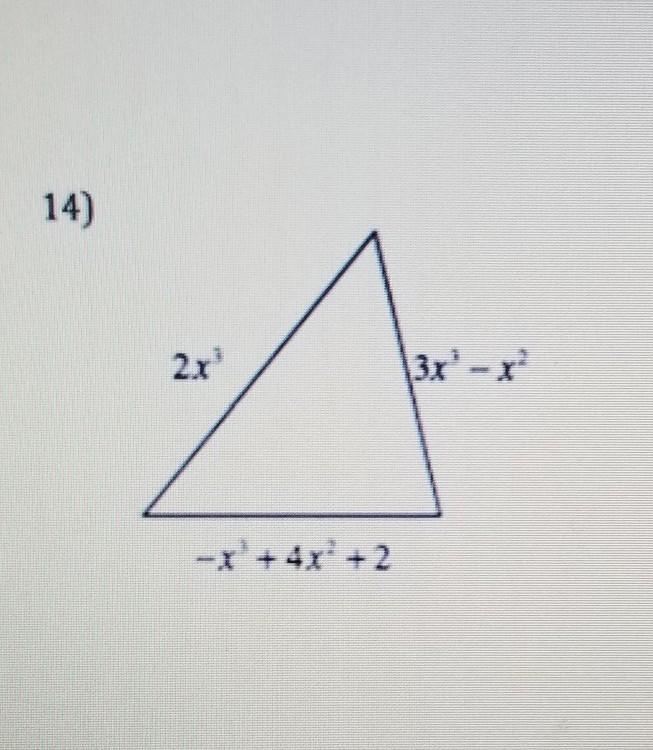
Answers
Answer:
perimeter = 4x³ + 3x² + 2
Step-by-step explanation:
perimeter = sum of the lengths of the sides
perimeter = 2x³ + 3x³ - x² - x³ + 4x² + 2
perimeter = 4x³ + 3x² + 2
The ratio of juice to sparkling water in a recipe is 5:3. If juan uses 25 ounces of juice, how many total ounces of punch did he make?
Answers
Answer:
144 oz
Step-by-step explanation:
What happens if the rate of change is not constant in a function?
Answers
Answer:
time will be a straight line, and you can find the rate of change by calculating the slope of the line. If the rate of change is not constant, a graph of the measured quantity vs. time will be curved instead of straight
Step-by-step explanation:
Answer:
When the rate of change is constant, the graph results in a straight line. When the rate of change is not constant, the graph will include a curved line.
Step-by-step explanation:
Please help 60 points for a rapid answer-In the figure below which of the following is true in circle E?


Answers
Answer:
all 3 options are true : A, B, C
Step-by-step explanation:
warning : it has come to my attention that some testing systems have an incorrect answer stored as right answer for this problem.
they say that A and C are correct.
but I am going to show you that if A and C are correct, then also B must be correct.
therefore, my given answer above is the actual correct answer (no matter what the test systems say).
originally the information about the alignment of the point F in relation to point E was missing.
therefore, I considered both options :
1. F is on the same vertical line as E.
2. F is not on the same vertical line as E.
because of optical reasons (and the - incomplete - expected correct answers of A and C confirm that) I used the 1. assumption for the provided answer :
the vertical line of EF is like a mirror between the left and the right half of the picture.
A is mirrored across the vertical line resulting in B. and vice versa.
the same for C and D.
this leads to the effect that all 3 given congruence relationships are true.
if we consider assumption 2, none of the 3 answer options could be true.
but if the assumptions are true, then all 3 options have to be true.
now, for the "why" :
remember what congruence means :
both shapes, after turning and rotating, can be laid on top of each other, and nothing "sticks out", they are covering each other perfectly.
for that to be possible, both shapes must have the same basic structure (like number of sides and vertices), both shapes must have the same side lengths and also equally sized angles.
so, when EF is a mirror, then each side is an exact copy of the other, just left/right being turned.
therefore, yes absolutely, CAD is congruent with CBD. and ACB is congruent to ADB.
but do you notice something ?
both mentioned triangles on the left side contain the side AC, and both triangles in the right side contain the side BD.
now, if the triangles are congruent, that means that each of the 3 sides must have an equally long corresponding side in the other triangle.
therefore, AC must be equal to BD.
and that means that AC is congruent to BD.
because lines have no other congruent criteria - only the lengths must be identical.
Find the total surface area of the figure below:

Answers
Answer:
95
Step-by-step explanation:
Formula -
Triangle Area - 1/2bh
Square - l x w
Triangle - 5 x 7 = 35/2 = 17.5
Since there are four triangle, we will multiply 17.5 x 4 which is equal to 70
Square - 5 x 5 = 25
Now we add both 70 and 25 which is 95
So the total surface area is 95
Answer:
95
Step-by-step explanation:
Area of the triangles:
A = (base × height) ÷ 2A = (5 × 7) ÷ 2A = (35) ÷ 2A = 17.5Because there are 4 triangles, we can do our answer of one triangle × 4So, 17.5 × 4 = 70Area of a square:
The sides of squares are the sameA = L × WA = 5 × 5A = 25Put them together:
Area of triangles + area of the square = surface area70 + 25 = suface area70 + 25 = 95I hope this helps!
그 ]
1 pts
A catering company has small tables and large tables. Small tables (s) seat 4 people and
large tables (L) seat 6. They are planning a party for more than 100 guests. How many of
each size table do they need?
Answers
Answer:
100 / 4 is 25 so the need like 25 and more of the small tables
Now, 100/6 is 16.67 approximately 17 seats are needed for the large seats.
You have created a 95% confidence interval for μ with the result
10 ≤ μ ≤ 15. What decision will you make if you test H0: μ=12
versus H1: μ≠12 at α = 0.05?
Do not reject H0 in favour
Answers
in this scenario, we would not reject the null hypothesis H0: μ = 12. The null hypothesis does not imply that the null hypothesis is true; rather, it means that we do not have enough evidence to reject it based on the available data.
Based on the given 95% confidence interval for μ as 10 ≤ μ ≤ 15 and performing a hypothesis test at α = 0.05 with the null hypothesis H0: μ = 12 and the alternative hypothesis H1: μ ≠ 12, we can make a decision regarding the null hypothesis.
Since the confidence interval for μ (10 ≤ μ ≤ 15) includes the value specified in the null hypothesis (12), we fail to reject the null hypothesis in favor of the alternative hypothesis.
In hypothesis testing, if the null hypothesis value falls within the confidence interval, it suggests that the null hypothesis is plausible, and there is insufficient evidence to reject it. Therefore, in this scenario, we would not reject the null hypothesis H0: μ = 12.
This decision implies that, at a significance level of α = 0.05, we do not have enough evidence to conclude that the true population mean μ is different from 12. It is important to note that failing to reject the null hypothesis does not imply that the null hypothesis is true; rather, it means that we do not have enough evidence to reject it based on the available data.
Remember that hypothesis testing provides a framework for making statistical decisions, and the conclusion is based on the evidence and the chosen significance level.
Learn more about hypothesis here
https://brainly.com/question/606806
#SPJ11
what is the radius of the semicircle

Answers
20
Step-by-step explanation:
Hint: Use the Pythagorean Theorem
———————————————————
We’ll just listen to what the hint says
Pythagorean theorem: a^2 + b^2 = c^2
where a = 16 b = 12 and c = radius
16^2 + 12^2 = c^2
256 + 144 = c^2
c^2 = 400
square root 400
sqrt rt 400 = 20
The radius of the semicircle is 20