Answers
Answer:
i dont understand russian
Step-by-step explanation:
Related Questions
A wooden beam is (3y2 + 7y+1) meters long. If a piece of length (y2 - 10) meters is cut off, express the length of the
remaining piece of beam as a polynomial in y.
Answers
Answer:
2y^2 +7y +11
Step-by-step explanation:
The remaining length is the difference between the original and the length cut off:
remaining = whole length - piece length
remaining = (3y^2 +7y +1) -(y^2 -10)
= 3y^2 +7y +1 -y^2 +10 = (3 -1)y^2 +7y +(1 +10)
remaining = 2y^2 +7y +11
Look at this graph: What is the slope? Simplify your answer and write it as a proper fraction, improper fraction, or integer.

Answers
Answer:
1/2
Step-by-step explanation:
Pick two points on the graph that are easy to read. A point is easiest to read if it lies on the intersection of grid lines.
For example, pick (10, 50) and (30, 60) which are easy to read.
slope = rise/run
rise = change in y
run = change in x
To go from (10, 50) to (30, 60), you go 20 units right. "20 units right" is a change of +20 units in x. The run is 20.
To go from (10, 50) to (30, 60), you go 10 units up. "10 units up" is a change of +10 units in y. The rise is 10.
slope = rise/run = 10/20
slope = 1/2
Tim dug a hole that was 16 1/2 inches deep. He left a pile of dirt next to the at was inches high. Show how you could use subtraction to find the e from the top of the pile of dirt to the bottom of the hole. 8 3/4 Tim dug a hole that hole that was distance from the
Answers
Answer: 2 inches
Step-by-step explanation:
14 inches
Step-by-step explanation:
Length of the hole = 16(½) inches
Length of the dirt = 8(¾)inches
The distance between the top of the pile to the bottom of the hole = ?
Since the dirt is beside the hole and not inside the hole, we can find it's length using addition.
Distance from the top to the bottom = length of dirt + length of the hole
Length of dirt = 8×¾ = 6 inches
Length of the hole = 16 × ½ = 8 inches
Distance from the top of the dirt to the bottom of the hole = (6 + 8) inches
Distance from the top of the dirt to the bottom of the hole = 14 inches
Note: the only way we can use subtraction here is if the hole was filled with dirt, what's the distance that's left
Distance left = length of hole - length of dirt
Distance left = 8 - 6 = 2 inches .
i need help. Can u help me solve for x?

Answers
Answer:
\( x = \sqrt {40}\)
Step-by-step explanation:
Given is an isosceles triangle, dotted line is the bisector of top angle which is also perpendicular bisector of the base of the triangle. Hence, by Pythagoras theorem:
\( {x}^{2} = {6}^{2} + ({ \frac{4}{2} })^{2} \\ = 36 + 4 \\ = 40 \\ \therefore \: x = \sqrt{40} \\ \)
Answer:
D. x = sqrt(52).
Step-by-step explanation:
Since the line measuring 6 units bisects the top angle, there are two right angles. We can use the Pythagorean Theorem to solve for x.
a^2 + b^2 = x^2
4^2 + 6^2 = x^2
16 + 36 = x^2
52 = x^2
x = sqrt(52)
x = sqrt(2 * 2 * 13)
x = 2sqrt(13)
x = 7.211102551.
Hope this helps!
490,612 round to the nearest hundred thousand
Answers
Answer:
500,000
Step-by-step explanation:
If the number is under 5 it rounds down and over 5 rounds up
Explanation:
7.
Wendy is paid $12 per hour and plans to work between 30 and 35 hours per week. Identify the independent and dependent quantity in the situation. Find reasonable domain and range values.
A. weekly pay; hours worked; $360 to $420; 30 to 35 hours
B. hours worked; weekly pay; 30 to 35 hours; $360 to $420
C. weekly pay; hours worked; 30 to 35 hours; $360 to $420
D. hours worked; weekly pay; 30 to 35 hours; $0 to $420
Answers
Answer: I'm gonna go with B. hours worked; weekly pay; 30 to 35 hours; $360 to $420, because I had something similar to this
Wendy is paid $9 per hour and plans to work between 20 and 25 hours per week. Identify the independent and dependent quantity in the situation. Find reasonable domain and range values.
weekly pay; hours worked; 20 to 25 hours; $180 to $225
hours worked; weekly pay; 20 to 25 hours; $180 to $225
weekly pay; hours worked; $180 to $225; 20 to 25 hours
hours worked; weekly pay; 20 to 25 hours; $9 to $225
Step-by-step explanation:
The independent quantity is her pay and the dependent quantity is the hours she works in a week.
I believe the range would be 30 to 35 hours and the domain would be $360 to $450.
...................................................................................................................................................
Answer:
Part a) The independent quantity is the variable x (the number of hours) and the dependent quantity is the variable y (total paid)
Part b) Domain
Part c) Range
Step-by-step explanation:
Let
x------> the number of hours
y-----> total paid
The linear equation that represent this situation is
so
Part a)
The independent quantity is the variable x (the number of hours)
The dependent quantity is the variable y (total paid)
Part b) Find the domain
The domain is the interval-------->
All real numbers greater than or equal to hours and less than or equal to hours
Part c) Find the range
For
find the value of y
For
find the value of y
The range is the interval------->
All real numbers greater than or equal to and less than or equal to
...............................................................................................................................................
The dependent quantity would be the $12 per hour, while the independent quantities are 30 hrs/ week to 35 hours/ week.
The $12 per hour would become $360/week to $420/week.
The domain of the problem, with the unit of hrs/week, would be :30,31,32,33,34,35.
The Range of the problem, with the unit of $/week, would be : 360, 372, 384, 396, 408, 420.
...............................................................................................................................................
Help me please!!!!!!!

Answers
Answer:
E = 100M/L
Step-by-step explanation:
we could cross-multiply to get:
E·L = 100M
now divide each side by L to get:
E = 100M/L
Coefficient of determination is a value between a) 0 and 1 b) \( -1 \) and 0 c) 1 and 100 d) \( -1 \) and 1
Answers
The coefficient of determination is a value between 0 and 1 (option a).
The coefficient of determination, denoted as \(R^{2}\) , is a statistical measure that represents the proportion of the variance in the dependent variable that can be explained by the independent variable(s) in a regression model. It ranges from 0 to 1, where 0 indicates that the independent variable(s) cannot explain any of the variability in the dependent variable, and 1 indicates that the independent variable(s) can completely explain the variability in the dependent variable.
\(R^{2}\) represents the goodness-of-fit of a regression model. A value close to 1 indicates a strong relationship between the independent and dependent variables, suggesting that the model provides a good fit to the data. On the other hand, a value close to 0 suggests that the model does not effectively explain the variability in the dependent variable.
Learn more about coefficient of determination here:
https://brainly.com/question/29581430
#SPJ11
Find a general solution to the Cauchy-Euler equation x3y" - 7x2y"' +8xy' - 8y = x? x>0, given that (x,4x In (4x),x®) is a fundamental solution set for the corresponding homogeneous equation. y(x) = 0 (Simplify your answer.)
Answers
The general solution is y(x) = c_1x + c_2x ln(x) + c_3x^-4 + (1/8)x, where c_1, c_2, and c_3 are constants.
To find the general solution to the Cauchy-Euler equation x^3y" - 7x^2y"' +8xy' - 8y = x, we first need to find a particular solution to the non-homogeneous equation.
We can try a particular solution of the form y_p(x) = Ax + B. Substituting this into the equation, we get:
x^3(0) - 7x^2(0) + 8x(1) - 8(Ax + B) = x
Simplifying this, we get:
8Ax - 8B = x
Comparing coefficients, we see that A = 1/8 and B = 0. Therefore, our particular solution is y_p(x) = (1/8)x.
Now, we can find the general solution by adding the particular solution to the homogeneous solution.
Since (x, 4x ln(4x), x^r) is a fundamental solution set for the corresponding homogeneous equation, we can write the homogeneous solution as:
y_h(x) = c_1x + c_2x ln(x) + c_3x^-4
where c_1, c_2, and c_3 are constants.
Therefore, the general solution is:
y(x) = y_h(x) + y_p(x)
y(x) = c_1x + c_2x ln(x) + c_3x^-4 + (1/8)x
where c_1, c_2, and c_3 are constants.
To learn more about the Cauchy-Euler equation visit: brainly.com/question/12976304
#SPJ11
Please help fast for 10 points and bro list!! And it’s multiple choice!!! ANSWER ONLY IF YOU KNOW!!

Answers
Answer:
C. x - 5y - 30 = 0
Step-by-step explanation:
Y = x/5 - 30
y + 30 = x/5
you multiply both sides by 5
5y + 30 = x
you subtract x from both sides
- x + 5y + 30 = 0
which is the same as
x - 5y - 30 = 0
Three dice are thrown simultaneously, the probability that sum being 3 is * (2 Points) \( 3 / 215 \) \( -12^{2} \)
Answers
The probability of getting 3 is P(A) = 1/216. The probability that sum being 3 is 1 / 216.
When three dice are thrown simultaneously, the probability that the sum is 3 is 1/216. A dice can show a minimum of one and a maximum of six dots, for a total of six sides, giving it a total of 6 * 6 * 6 = 216 possible outcomes.
:When three dice are thrown simultaneously, the probability that the sum is 3 is 1/216. The probability can be calculated as follows:
Let A be the event that the sum is 3. The number of ways to get 3 is 1, i.e., (1, 1, 1). The total number of possible outcomes is 6³,
since each die can have 6 possible outcomes.
Therefore, the probability of getting 3 is P(A) = 1/216.
In conclusion, the probability that sum being 3 is 1 / 216.
To know more about probability visit:
brainly.com/question/31828911
#SPJ11
2. The diagram shows a circle inside a rectangle. Work out the shaded region,
Give your answer to 3 significant figures.
8 cm
17 cm
32 cm

Answers
Answer:
342.938 cm²
Step-by-step explanation:
Area of shaded region = area of rectangle - area of circle
= (length*width) - (π*radius²)
= (32*17) - (π8²)
= 544 - 201.06192983
= 342.93807017 ≈ 342.938 cm²
PLEASE HELPPPPP GEOMETRY
WILL GIVE BRAINLIEST

Answers
Answer:
B
Step-by-step explanation:
Here are the first four terms of a quadratic sequence
3 10 21 36
Work out an expression for the nth term
Please please answers this question ill give u like and mark as brainlist
Answers
Answer:
2n² + n
Step-by-step explanation:
Given sequence:
3, 10, 21, 36, ...
Work out the first differences between terms:
\(3 \underset{+7}{\longrightarrow} 10 \underset{+11}{\longrightarrow} 21 \underset{+15}{\longrightarrow} 36\)
As the first differences are not the same, work out the second differences.
\(7 \underset{+4}{\longrightarrow} 11 \underset{+4}{\longrightarrow} 15\)
As the second differences are the same, the sequence is quadratic and will contain an n² term.
The coefficient of n² is always half of the second difference. Therefore, as the second difference is 4, the coefficient of n² is 2.
To work out the nth term of the sequence, write out the numbers in the sequence 2n² and compare with the given sequence:
\(\begin{array}{|l|c|c|c|c|}\cline{1-5} n & 1 & 2 & 3 & 4 \\\cline{1-5} 2n^2 & 2 & 8 & 18 & 32\\\cline{1-5} \sf Operation & +1 & +2& +3& +4\\\cline{1-5} \sf Sequence & 3 & 10 & 21 & 36\\\cline{1-5}\end{array}\)
Therefore, we need to add n to 2n² to match the sequence.
Therefore, the nth term of this sequence is 2n² + n.
Learn more about sequences here:
https://brainly.com/question/27953040
https://brainly.com/question/27775450
A frame designer is making a triangular frame. She has two sides of length 18 inches and 27 inches. What are the possible lengths for the third side?.
Answers
The possible lengths( in whole elevation) for the third side is
9 elevation< x< 45 elevation, i.e in between 9 and 45 elevation.
For the below question, we've a rule from the properties of triangle, the sum of the length of any two sides of the triangle must be lesser than the length of the third side.
Hence
She has two sides of length 18 elevation and 27 elevation
Let the third side = x
Hence
a) 18 27> x
45> x
b) 18 x> 27
x> 27- 18
x> 9
thus, the possible lengths( in whole elevation) for the third side is
elevation< x< 45 elevation
Learn more about properties of triangle here :
https://brainly.com/question/30166682
#SPJ4
Which relationship in the triangle must be true?
A
с
a
sin(B) = sin(A)
Osin(B) = cos(90 - B)
O cos(B) = sin(180-B)
Ocos(B) = cos(A)
B

Answers
The relationship in the triangle that must be true is sin B = cos (90 - B).
option B.
What are complementary angles?Complementary angles are two angles that add up to 90 degrees. In other words, if you have two angles that are complementary, the measure of one angle added to the measure of the other angle will equal 90 degrees.
In the given diagram, angle A is complementary to angle B.
90 - B = A
From the diagram, Sin B = b/c
Cos (90 - B) = Cos A = b/c
So Cos (90 - B) is equal to sin B because the two angles are complementary.
Learn more about complementary angles here: https://brainly.com/question/16281260
#SPJ1
In VWX, X ≈ W, WX = 17 and VW = 16. Find XV. HELPPPPP

Answers
Answer:
XV = 16
Step-by-step explanation:
Triangle VWX is an isosceles triangle. Since the base angles are the same, the sides opposite the angle are also the same. See image. They gave VW so XV is the same as VW.

The answer would be
A bag only contains 4 red and 6 blue marbles. What is the probability of drawing a green marble?
NO LINKS OR TROLL
THIS IS YOUR WARNING
Answers
Answer:
0% chance
Step-by-step explanation:
there are no green marbles
Marbles no
red=4blue=6green=0There is no green marbles
So it will never occur(impossible event)
Probability is 0What is the output of y=23x−4 when the input is 18?
Answers
Answer:
y = 410
General Formulas and Concepts:
Pre-Algebra
Order of Operations: BPEMDAS
Brackets Parenthesis Exponents Multiplication Division Addition Subtraction Left to RightStep-by-step explanation:
Step 1: Define
y = 23x - 4
x = 18
Step 2: Evaluate
Substitute x: y = 23(18) - 4Multiply: y = 414 - 4Subtract: y = 410Answer:
y = 410
Step-by-step explanation:
y = 23x - 4 If x = 18
y = (23*18) - 4
y = 414 - 4
y = 410
The restaurant Frank’s Burger Shack is offering a lunch special this week. Customers who order lunch will receive 25% off the original total price of their lunch order. The original total price of a lunch order is represented by the variable p .
Which of the expressions below represent the price of the lunch special.
Select all that apply.
A
p−0.25p
B
p−0.25
C
0.25p
D
0.75p
Answers
The expressions below represent the price of the lunch special are:
A. p-0.25p
D. 0.75p
What are linear expressions?A term in an algebraic expression that is referred to as a linear expression is either a constant or a variable raised to the first power. The fundamental equation used to represent and solve for an unknown quantity is a linear expression in one variable. It is simply depicted graphically and is always a straight line. A simple way to convey a mathematical assertion is with a linear expression.
In the given question,
The original total price of a lunch order be the variable p.
Now 25% off the original price = 25% × p
= 0.25p
Now after the discount the price of the lunch special is:
p-0.25p
= 0.75p
Therefore, the expressions below represent the price of the lunch special are:
A. p-0.25p
D. 0.75p
To know more about linear expressions, visit:
https://brainly.com/question/30112998
#SPJ1
A card is drawn from a deck of cards, Then the card is replaced, the deck is reshuffled, second card is drawn. What is the probability of getting.
1. a 3 on the first draw and a red card on the second draw?
2. an ace on the first draw and a heart on the second draw?
3. a queen on the first draw and a king on the second draw
4. a jack on the first draw and multiple of 5 on the second draw
Answers
Answer:
https://socratic.org/questions/a-card-is-drawn-from-a-standard-deck-a-second-card-is-drawn-without-replacing-th-1#131182
Step-by-step explanation:
:)
hellllllllllllllllllllpppppppppppppppppppppppppppppppppp

Answers
= 115/10
= 11.5
median= middle number when numbers are arranged in order from least to greatest
2,3,5,5,5,5,10,10,20,50
since the amount of numbers is even there is no middle number so you take the two middle numbers and find the average of them.
In this case they are 5 and 5 so you add them and divide by 2 which just gives you 5.
mode= the number that is most common so 5
Express the inequality −1.8≤x≤−0.03 using interval notation.
Answers
Answer:
[-1.8,0.03]
Step-by-step explanation:
This is how you use interval notation, square brackets means including the numbers, circular brackets means not including
Answer:
(-1.3,1.8]
Step-by-step explanation:
The inequality −1.3<x≤1.8 means "all numbers between −1.3 and 1.8." In interval notation, we express this as (−1.3,1.8]. Notice that we use a parenthesis to show that the number −1.3 is not included and a bracket to show that the number 1.8 is included.
The temperature F, in degrees Fahrenheit, of a cup of coffee t minutes after it is poured is given by F(t)= 72 + 118e^-0. 093t. To the nearest degree, what is the average temperature of the coffee between t= 0 and t= 10 minutes?
A. 93 degrees
B. 119 degrees
C. 146 degrees
D. 149 degrees
E. 154 degrees
Answers
The average temperature of the coffee between t = 0 and t = 10 minutes is approximately 146 degrees.
Option C is the correct answer.
We have,
To find the average temperature of the coffee between t = 0 and t = 10 minutes, we need to calculate the average value of the temperature function F(t) over that interval.
The formula for the average value of a function f(x) over an interval [a, b] is given by:
Average value = (1 / (b - a)) x ∫[a to b] f(x) dx
In this case,
We want to find the average temperature over the interval [0, 10] minutes, so our formula becomes:
Average temperature = (1 / (10 - 0)) x ∫[0 to 10] F(t) dt
To find the integral of F(t), we need to calculate the antiderivative of F(t):
∫ F(t) dt = ∫ \((72 + 118e^{-0.093t}) dt\)
Using the power rule of integration and the fact that the integral of \(e^x\) is \(e^x\), we can evaluate the integral:
∫ F(t) dt =\(72t - (118 / 0.093) \times e^{-0.093t}\)
Now,
Average temperature = (1 / 10) x ∫[0 to 10] F(t) dt
Average temperature = (1 / 10) x (72(10) - (118 / 0.093) x \(e^{-0.093(10)}\) - (72(0) - (118 / 0.093) x \(e^{-0.093(0)}))\)
Simplifying further:
Average temperature = (1 / 10) x (720 - (118 / 0.093) x \(e^{-0.93}\) - 0)
Using a calculator, we find:
Average temperature ≈ 146 degrees
Therefore,
The average temperature of the coffee between t = 0 and t = 10 minutes is approximately 146 degrees.
Learn more about average temperature here:
https://brainly.com/question/28041542
#SPJ1
The product of the sum of 3 and 6 and the sum of 2 and 7
Answers
Identify which of the following measurements could be accurate for the given scenarios if the given lengths create right triangles. Select the two scenarios that apply. PLEASE HURRY:(
1. A 17-foot ladder is leaning against a building so that the top of the ladder is 15 feet up the side of the building. The distance from the base of the building to the bottom of the ladder is 7 feet.
2. A small bicycle ramp is 17.9 inches long. The ramp is 7.5 inches off the ground, and the distance along the ground is 17.7 inches.
3. Tom is looking out the window from a height of 124.8 feet and sees his friend William on the ground. The straight-line distance from Tom to William is 130 feet, and the distance from William to the base of the building is 36.4 feet.
4. An airplane is in the air 30,000 feet directly above you. You are 40,000 feet from where the plane took off, and the plane has traveled 50,000 feet along a straight-line path from the takeoff point.
5. A tree that is 20 feet tall casts a 17-foot-long shadow along the ground. The straight-line distance from the top of the tree to the tip of the shadow is 26 feet.
6. A lighthouse is 65 feet tall and casts a beam of light that measures 100 feet from the top of the lighthouse to the point where it hits the ground. The distance from where the light hits the ground to the bottom of the lighthouse is 75 feet.
Answers
Two scenarios that create right angle are 3 and 4.
In both the situations, Pythagoras theorem get satisfy with the sides which theorem is only applicable when there is right angle in the triangle.
Here's the explanation using 3 as an example :
From the statement we can assume that height of the window as the perpendicular(P) and distance from base(B) of the building to William as base of the triangle and line distance from Tom to William as hypotenuse(H) of the triangle.
Therefore, H = 130, B= 36.4 and P = 124.8
By Pythagoras theorem,
H² = P² + B²;
130² = 124.8² + 36.4²,
16900 = 15575.04 + 1324.96,
16900 = 16900
Hence satisfy the Pythagoras theorem, therefore triangle is right angle.
Similarly for the 4 statement.
To know about more Pythagoras theorem https://brainly.com/question/8564112
#SPJ1
Find the area bounded by the parametric curve x=cost,y=et;0≤t≤π/2, and the lines y=1andx=0
Answers
The given parametric curve x=cost, y=et; 0≤t≤π/2, intersects the line y=1 at t=0, and intersects the line x=0 at t=π/2. Therefore, we need to find the area bounded by the curve and the lines y=1 and x=0, between t=0 and t=π/2. We can use the formula for area enclosed by a curve given by A=∫(y.dx) from a to b, where y is the function of x. In this case, we need to express x in terms of y, so we can use x=arccos(y) and substitute it in the formula. The final result is A=e-1/2.
The given parametric curve x=cost, y=et; 0≤t≤π/2, intersects the line y=1 at t=0, and intersects the line x=0 at t=π/2. Therefore, we need to find the area bounded by the curve and the lines y=1 and x=0, between t=0 and t=π/2. To do so, we can use the formula for area enclosed by a curve given by A=∫(y.dx) from a to b, where y is the function of x. In this case, we need to express x in terms of y, so we can use x=arccos(y) and substitute it in the formula. The final result is A=e-1/2.
The area bounded by the parametric curve x=cost, y=et; 0≤t≤π/2, and the lines y=1 and x=0 is e-1/2. This can be found using the formula for area enclosed by a curve given by A=∫(y.dx) from a to b, where y is the function of x. We need to express x in terms of y, so we can use x=arccos(y) and substitute it in the formula. The curve intersects the line y=1 at t=0 and the line x=0 at t=π/2, which defines the boundaries for the integral.
To know more about parametric curve visit:
https://brainly.com/question/15585522
#SPJ11
PLEASEEE I CAN'T DO ITT
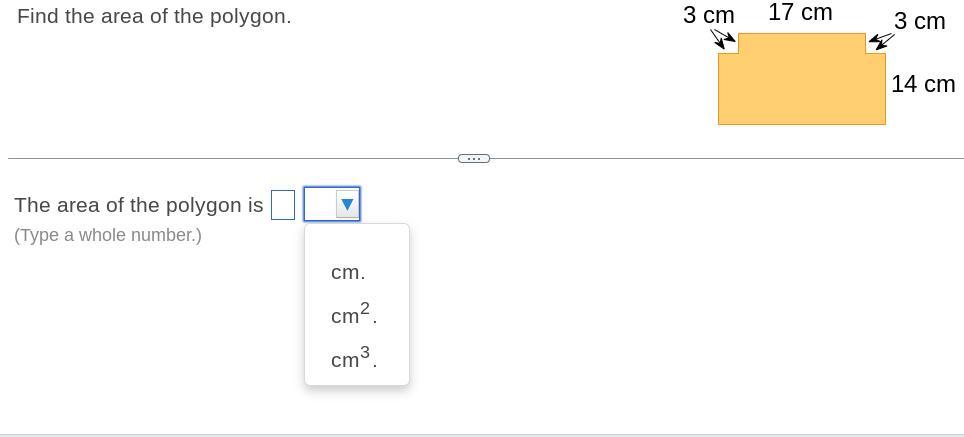
Answers
Answer:
answer is cm² ok
Step-by-step explanation:
because it is saying area ,in area we have to put cm2
Can someone help me with this please?

Answers
Answer:
16.49
Step-by-step explanation:
21^2 - 13^2 = 272
square root 272 = 16.49
Rafael has 118 baseball cards arranged in an album. Each page of the album can hold 9 cards. How many pages are full and how many cards are on the last page?
Answers
Answer:
Dear user,
Answer to your query is provided below
Pages full = 13
Card on last page = 1
Step-by-step explanation:
Rafael has 118 baseball cards.
Each album can hold 9.
So, using multiples of 9 or by dividing 118/9
That 1 is remainder.
So, cards on last page is 1
Verify ,
Pages full = (118-1)/9 = 13
