PLSSSSSSSSSSSSS HELp VERY URGENT The graph of F(x), shown below, resembles the graph of G(x) = x^2, but it has been stretched somewhat and shifted. Which of the following could be the equation of F(x)?


Answers
Answer:
Option B
Step-by-step explanation:
Function 'g' is,
g(x) = x²
Since, leading coefficient of this function is positive, parabola is opening upwards.
From the graph attached,
Function 'f' is opening upwards leading coefficient of the function will be positive.
Since, the graph of function 'f' is vertically stretched, equation will be in the form of f(x) = kx²
Here, k > 1
Since, function 'f' is formed by shifting the graph of function 'g' by 1 unit upwards,
f(x) = g(x) + 1
Combining all these properties, equation of the function 'f' should be,
f(x) = 4x² + 1
Option B will be the correct option.
Related Questions
Which of the following algebraic representations shows a dilation that is an enlargement? A.(13x,13y) B.(.1x, .1y) C.(58x, 58x) D.(52x, 52y)
Answers
Answer:
A
Step-by-step explanation: yes
Answer: C
Step-by-step explanation:
Rosa is saving money for a tripShe has already saved $250 and plans to save $40 per week. This situation can be represented by the function f(x) = 250 + 40x , where x is the number of weeks. How much money has Rosa saved after 6 weeks? (5 points ) 1 ) $ 490 2 ) $ 480 3 ) $ 250 4 ) $ 240
The answer is $490..
just trying to help people get points
Answers
Answer:
490
Step-by-step explanation:
Thanks!
500.00
-319.45 = m
Solve for m
Answers
Answer:
STo solve for m in the equation -319.45 = m, we can isolate the variable m by adding 319.45 to both sides of the equation:
-319.45 + 319.45 = m + 319.45
This simplifies to:
0 = m + 319.45
Finally, we can subtract 319.45 from both sides to solve for m:
0 - 319.45 = m + 319.45 - 319.45
-319.45 = m
Therefore, the value of m is -319.45.tep-by-step explanation:
Easy , but whats the answer ???

Answers
Answer:
cant see it.................
Step-by-step explanation:
Answer:
im sorry but you said it was ages ago
Step-by-step explanation:
The standard normal curve is graphed below. Shade the region under the standard normal curve to the left of z=0.50

Answers
Given:
A graph is given with standard normal curve to the left of z=-0.50
Required:
Shade the region under the standard normal curve to the left of z=0.50
Explanation:
The region under the standard normal curve to the left of z=-0.50 is shown right for the area under the standard curve is 1
\(\begin{gathered} P(z\leq-0.50)=\phi(-0.50)=\text{ 1-}\phi(0.5) \\ \\ =1-0.6915=0.3085 \end{gathered}\)Required answer:
Shaded region shown in above graph

In Tokyo, construction workers earn an average of ×420,000 (yen) per month with a standard deviation of ×20,000, while in Hamburg, Germany, construction workers earn an average of €3,200 (euros) per month with a standard deviation of €57. A worker making ×460,000 per month in Tokyo is earning relatively the same as one earning €3,300 per month in Hamburg?
Answers
Answer:
Since they have different z-scores, they are not earning relatively the same.
Step-by-step explanation:
We use z-scores to solve this question.
In a set with mean \(\mu\) and standard deviation \(\sigma\), the zscore of a measure X is given by:
\(Z = \frac{X - \mu}{\sigma}\)
In this question:
They will be earning relatively the same if they have the same z-score.
Tokyo:
Earns 460, mean 420, standard deviation 20. So \(X = 460, \mu = 420, \sigma = 20\). The z-score is:
\(Z = \frac{X - \mu}{\sigma}\)
\(Z = \frac{460 - 420}{20}\)
\(Z = 2\)
Hamburg:
Earns 3300, mean 3200, standard deviation 57. So \(\mu = 3300, \sigma = 3200, \sigma = 57\).
The z-score is:
\(Z = \frac{X - \mu}{\sigma}\)
\(Z = \frac{3300 - 3200}{57}\)
\(Z = 1.75\)
Since they have different z-scores, they are not earning relatively the same.
How can u find missing angles measurements in supplementary angles?

Answers
First, let's remember the definition of a supplementary angle
A supplementary angle is an angle with which a straight angle is formed. That is, two angles are supplementary if their sum is 180º
We have two angles, the angle
The angle \(\begin{gathered} <\text{ABD}+<\text{CBD}=180 \\ <\text{ABD}+92.1=180- \\ <\text{ABD}=180-92.1 \\ <\text{ABD}=87.9 \end{gathered}\)
In conclusion, the answer is 87.9°
The lines are what form the angles for us, where the supplementary angle, in this case, the angle

9. Find BD if AABC~AXYZ
A
BD and
and
B
12
A
D
C X
YW
are medians.
Answers
We have found that: AB = (BD/2) * (XZ/AY) and we can solve for BD:
BD = 2 * AB * AY / XZ
What is triangle?
A triangle is a three-sided polygon with three angles. It is a fundamental geometric shape and is often used in geometry and trigonometry.
Since BD is a median of triangle ABC, it divides side AC into two equal parts. Let's label the midpoint of AC as M. Similarly, YW is a median of triangle XYZ and it divides side XZ into two equal parts, with the midpoint labeled as N.
Since AABC~AXYZ, we know that the corresponding sides are proportional. Thus,
AB/AX = AC/AY = BC/BZ
Since AB = BC (given that B is a vertex of both triangles), we have:
AB/AX = AC/AY
We can rewrite this equation as:
AB/AC = AX/AY ----(1)
Since BD is a median of triangle ABC, we have:
BD = 1/2 AC ----(2)
Similarly, since YW is a median of triangle XYZ, we have:
YW = 1/2 XZ ----(3)
From equation (1), we have:
AB/AC = AX/AY
Multiplying both sides by AC, we get:
AB = AX * (AC/AY)
Now, using equation (2), we can substitute AC/2 for BD:
AB = AX * BD/AY
Finally, using equation (3), we can substitute XZ/2 for YW:
AB = (BD/2) * (XZ/AY)
Therefore, we have found that:
AB = (BD/2) * (XZ/AY)
Hence, we can solve for BD:
BD = 2 * AB * AY / XZ
Note that we need to know the actual values of AB, AY, and XZ to find BD.
To learn more about triangle from the given link:
https://brainly.com/question/2773823
#SPJ1
Si tienes gripe y debes tomar tres cápsulas 207 al día, ¿cuántas horas
pasarán entre toma y toma
Answers
The equivalent expression value when {a} = 2, {b} = -3, {c} = -1 is 19.
What is division?Division is a process of splitting a number into two or more equal parts.
Given is that you have the flu and must take three 207 capsules a day
There are total 24 hours in a day.
Total number of capsules to be taken in a day = 3.
We can write the number of hours between elapsing as -
{n} = 24/3
{n} = 8
Therefore, the equivalent expression value when {a} = 2, {b} = -3, {c} = -1 is 19.
To solve more questions on division, visit the link below-
https://brainly.com/question/15381501
#SPJ9
{Question in english -
If you have the flu and must take three 207 capsules a day, how many hours will elapse between takings?}
VERY EASY, WILL GIVE 50 POINTS FOR CORRECT ANSWER ASAP AND WILL GIVE BRAINLIEST.

Answers
Hope this helped! :)
35 miles per hour to kilometers per hour
Answers
Answer:
35 mph into kilometers per hour is 56.327 kph
Does each equation represent exponential decay or exponential growth?
Drag and drop the choices into the boxes to correctly complete the table.

Answers
Answer:
decay: a, b
growth: c, d, e, f
neither: g
Step-by-step explanation:
A function is exponential if it has the independent variable in an exponent. g(x) is a linear function, not an exponential function.
__
An exponential function is a growth function if the coefficient of the variable is positive and the base of the exponent is greater than 1. It is a decay function if the exponent is positive and the base is less than 1.
The first two functions are decay functions. The remaining four exponential functions are growth functions.
Find the Distance between the two points . (2,5) and (-4,7).

Answers
Answer:
6.32
Step-by-step explanation:
They gave you everything you need in the question - just plug the x and y values in where they showed you. (2, 5) is your (x1, y1) and (-4, 7) is your (x2, y2)
0.40 - 2.55
5
please answer this
Answers
Answer:
-2.15
Step-by-step explanation: I was kind of confused on what you asked. If you were asking for 0.4-2.55, then that is the answer above.
1/2x+4=2/3x+1, solve for x
Answers
Answer:
x=18
Step-by-step explanation:
Step 1: Subtract 2/3x from both sides.
1/2x+4=2/3x+1
-2/3x -2/3x
= -1/6x+4=1
Step 2: Subtract 4 from both sides.
-1/6x+4=1
-4 -4
= -1/6x=-3
Step 3: Multiply both sides by 6/(-1).
-1/6x=-3
*6/(-1) * 6/(-1)
x=18
Kylan had 1 ⅜ kilograms of fertilizer. He used some to fertilize a flower bed, and he only had ⅔ kilograms left. How much fertilizer was used in the flower bed?
Answers
Answer: 17/24
Step-by-step explanation:
let x be the fertilizer used in the flower bed
to find how much he has left
1 3/8 - x = 2/3
the fraction 1 3/8 we can write as 11/8
11/8 - x = 2/3
11/8 -2/3= x
we need the common denominator between 8 and 3, which is 24
33/24-16/24=17/24
PLEASE QUICK TIMED !!!
What is the end behavior of the polynomial function?

Answers
Answer:
As X -> ∞, then y -> - ∞
Step-by-step explanation:
If you look at the right-most part of the graph, you'll see X is increasing, while the Y value is decreasing
1. On the same day, Parkers friend, Peggy, was charged $5 for 1 1/2lb of gummy candy. Explain in terms of the graph why this most be a mistake.
Answers
3 A system of two linear equations is graphed on a coordinate plane. If the system of
equations has infinitely many solutions, which statement must be true?
a. On the graph, there are no points (x, y) that satisfy both equations.
b. On the graph, there is exactly one point (x, y) that satisfies both equations.
c. On the graph, any point (x, y) that satisfies one of the equations cannot satisfy the
other equation.
d.
On the graph, any point (x, y) that satisfies one of the equations must also satisfy
the other equation.
Answers
Answer:
d. On the graph, any point (x, y) that satisfies one of the equations must also satisfy the other equation.----------------------
If the system of linear equations has infinitely many solutions, it means the two lines overlap.
In other words, each point of one of the lines also belongs to the second line.
Choices a, b, c give us one or no solutions and therefore not the answer.
Choice d is reflecting the infinitely many solutions and hence is the correct one.
some students are painting a mural on the side of a building. they have enough paint for a 1000-square-foot area triangle. if two sides of the triangle measure 60 feet and 120 feet, then what angle (to the nearest degree) should the two sides form to create a triangle that uses up all the paint
Answers
The angle is 16 degrees or 164 degrees.
Any triangle that is not a right triangle is said to be oblique. There are two types of triangles: acute triangles, in which all three angles are less than right angles, and obtuse triangles (one of the three angles is greater than a right angle). Actually, right triangles could equally well be included in the category of "oblique triangles" for trigonometric purposes. In that case, studying oblique triangles is basically studying all triangles.
Given, the area of an oblique triangle which is going to be used as an area of the formula backward to find a missing measure.
We know two side lengths of the triangular region 60 ft and 1 20 ft
Area = 1000-square-foot
A= 1/2*60*120=1000
A=3600=1000
Dividing both sides with 3600,
We get an angle of 16 degrees.
But it is also possible that the area will be the same. If we use this in supplementary angle as well we get,
180-16 = 164 degrees.
To know more about triangles visit: brainly.com/question/2773823
#SPJ4
x + 7y = 21
x + 4y = 15
Solve the simultaneous equations
Answers
Answer:
x = 7, y = 2
Explanation:
equations given:
x + 7y = 21
x + 4y = 15
make "x" the subject:
x + 7y = 21
x = 21 - 7y .........equation 1
x + 4y = 15
x = 15 - 4y .......equation 2
Solving both the equations simultaneously:
15 - 4y = 21 - 7y
-4y + 7y = 21 - 15
3y = 6
y = 6 ÷ 3
y = 2
If y is 2
Then x = 21 -7y;
x = 21 - 7(2)
x = 21 - 14
x = 7
x + 7y= 21
7 goes to RHS = X+ y = 21/7-3
x + 4y = 15
4 goes to RHS= x+y = 15/4 = 3.75
Suppose that 45% of students in a college have a smart phone. If you select three students at random, what is the probability that all three have a smart phone? Give your answer as a decimal (to at least 3 places) or fraction
Answers
Answer:
\( P(X=3)\)
And we can use the probability mass function and we got:
\(P(X=3)=(3C3)(0.45)^3 (1-0.45)^{3-3}=0.091\)
Then the probability that all three have a smart phone is 0.091
Step-by-step explanation:
Let X the random variable of interest "number of students with smartphone", on this case we now that:
\(X \sim Binom(n=3, p=0452)\)
The probability mass function for the Binomial distribution is given as:
\(P(X)=(nCx)(p)^x (1-p)^{n-x}\)
Where (nCx) means combinatory and it's given by this formula:
\(nCx=\frac{n!}{(n-x)! x!}\)
And we want to find this probability:
\( P(X=3)\)
And we can use the probability mass function and we got:
\(P(X=3)=(3C3)(0.45)^3 (1-0.45)^{3-3}=0.091\)
Then the probability that all three have a smart phone is 0.091
Kuta Software - Infinite Algebra 2 Factoring By Grouping Factor ench completely. 1) 12a-9a? +40 - 3 Name Sarn on the ears Date : 120, 37443 4ac3a ²+1)-3(3u341) ta 2373 (403) 2) 2p'+ 5p² +6p+ 15 apstept sport 2plpa

Answers
Factor the expression.
\(\begin{gathered} 90au-36av-150yu+60yv=90au-150yu+60yv-36av \\ =30u(3a-5y)-12v(3a-5y) \\ =(3a-5y)(30u-12v) \\ =(3a-5y)(6\cdot5u-6\cdot2v) \\ =6(3a-5y)(5u-2v) \end{gathered}\)So answer is,
\(6(3a-5y)(5u-2v)\)I=\int\limits{\frac{x}{x^{4} -8x^{2} +16} } \,
Answers
Answer:
Step-by-step explanation:
2 Points
These dot plots show the lengths (in feet) from a sample of crocodiles and
alligators
Crocodi
0 1 2 3 4 5 6 7 8 9 10 11 12 13 14 15 16 17 18 19 20
Alligator
0 1 2 3 4 5 6 7 8 9 10 11 12 13 14 15 16 17 18 19 20
Length
What are the differences between the centers and spreads of these
distributions?
Select two choices: one for the centers and one for the spreads
D A Centers: The crocodiles have a lower median length than the
alligators
B. Centers: The crocodiles have a greater median length than the
alligators
c. Spreads: The lengths of the alligators are more spread out
D. Spreads. The lengths of the crocodiles are more spread out
PREVIOUS
SEO
Answers
Answer:
(B) Centers: The crocodiles have a greater median length than the alligators
(C) Spreads: The lengths of the alligators are more spread out
Step-by-step explanation:
The question is incomplete without the diagram. Find attached the diagram used in solving the question
Centers: this is the median of the distribution
Spread: this is the variation of the data distribution. The range can be used to find the spread. If the range is large, the spread is larger and If the range is small, the spread is smaller.
Range = highest value - lowest value
From the diagram:
Median of crocodile = 17
Median of the alligator = 9
Therefore, for Centers: The crocodiles have a greater median length than the alligators (option B)
Spread for crocodile data = from 15 to 19
Range = 19-15 = 4
Spread for alligator data = from 7 to 15
Range = 15-7 = 8
For Spreads: The lengths of the alligators are more spread out.

Answer: b and c
Step-by-step explanation:
rewrite (21+31).4 using the disbributive property of multiplication over addition
Answers
Answer: evaluate it is 52
Step-by-step explanation:
solve the equation
a) y''-2y'-3y= e^4x
b) y''+y'-2y=3x*e^x
c) y"-9y'+20y=(x^2)*(e^4x)
Answers
Answer:
a) To solve the differential equation y''-2y'-3y= e^4x, we first find the characteristic equation:
r^2 - 2r - 3 = 0
Factoring, we get:
(r - 3)(r + 1) = 0
So the roots are r = 3 and r = -1.
The general solution to the homogeneous equation y'' - 2y' - 3y = 0 is:
y_h = c1e^3x + c2e^(-x)
To find the particular solution, we use the method of undetermined coefficients. Since e^4x is a solution to the homogeneous equation, we try a particular solution of the form:
y_p = Ae^4x
Taking the first and second derivatives of y_p, we get:
y_p' = 4Ae^4x
y_p'' = 16Ae^4x
Substituting these into the original differential equation, we get:
16Ae^4x - 8Ae^4x - 3Ae^4x = e^4x
Simplifying, we get:
5Ae^4x = e^4x
So:
A = 1/5
Therefore, the particular solution is:
y_p = (1/5)*e^4x
The general solution to the non-homogeneous equation is:
y = y_h + y_p
y = c1e^3x + c2e^(-x) + (1/5)*e^4x
b) To solve the differential equation y'' + y' - 2y = 3xe^x, we first find the characteristic equation:
r^2 + r - 2 = 0
Factoring, we get:
(r + 2)(r - 1) = 0
So the roots are r = -2 and r = 1.
The general solution to the homogeneous equation y'' + y' - 2y = 0 is:
y_h = c1e^(-2x) + c2e^x
To find the particular solution, we use the method of undetermined coefficients. Since 3xe^x is a solution to the homogeneous equation, we try a particular solution of the form:
y_p = (Ax + B)e^x
Taking the first and second derivatives of y_p, we get:
y_p' = Ae^x + (Ax + B)e^x
y_p'' = 2Ae^x + (Ax + B)e^x
Substituting these into the original differential equation, we get:
2Ae^x + (Ax + B)e^x + Ae^x + (Ax + B)e^x - 2(Ax + B)e^x = 3xe^x
Simplifying, we get:
3Ae^x = 3xe^x
So:
A = 1
Therefore, the particular solution is:
y_p = (x + B)e^x
Taking the derivative of y_p, we get:
y_p' = (x + 2 + B)e^x
Substituting back into the original differential equation, we get:
(x + 2 + B)e^x + (x + B)e^x - 2(x + B)e^x = 3xe^x
Simplifying, we get:
-xe^x - Be^x = 0
So:
B = -x
Therefore, the particular solution is:
y_p = xe^x
The general solution to the non-homogeneous equation is:
y = y_h + y_p
y = c1e^(-2x) + c2e^x + xe^x
c) To solve the differential equation y" - 9y' + 20y = x^2*e^4x, we first find the characteristic equation:
r^2 - 9r + 20 = 0
Factoring, we get:
(r - 5)(r - 4) = 0
So the roots are r = 5 and r = 4.
The general solution to the homogeneous equation y" - 9y' + 20y = 0 is:
y_h = c1e^4x + c2e^5x
To find the particular solution, we use the method of undetermined coefficients. Since x^2*e^4x is a solution to the homogeneous equation, we try a particular solution of the form:
y_p = (Ax^2 + Bx + C)e^4x
Taking the first and second derivatives of y_p, we get:
y_p' = (2Ax + B)e^4x + 4Axe^4x
y_p'' = 2Ae^4x +
2. The box and whisker plot below shows the starting salaries for 120 graduates of a small college.
a) What is the range of the starting salaries?
b) About 30 graduates make below what amount?
c) How many graduates have a salary above $33,000 ?
d) $25 of the graduates make above what amount?
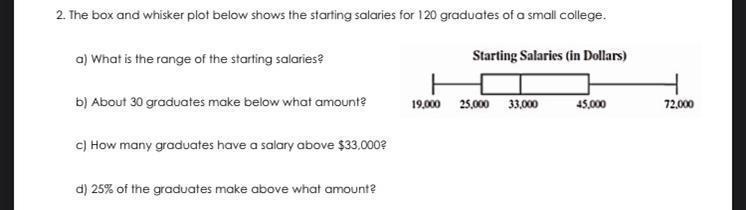
Answers
The range of the starting salaries is 53,000
Given,
The box and whisker plot below shows the starting salaries
The number of graduates in a small college = 120
We have to find the range of the starting salaries;
Range of a data;
The difference between the highest and lowest values for a given data collection is the range in statistics. For instance, the range will be 10 - 2 = 8 if the given data set is 2, 5, 8, 10, and 3. As a result, the range may alternatively be thought of as the distance between the highest and lowest observation.
Here,
Lowest value = 19,000
Highest value = 72,000
Then,
Range of the set = 72,000 - 19,000 = 53,000
That is,
The range of the given data set is 53,000
Learn more about range here;
https://brainly.com/question/1634693
#SPJ4
If a car is worth 28,000 in 2019 and the value depreciates at8.5% per year, what will it be worth in 2030? The answer is 10,538.77 I just need help finding how to get that answer.
Answers
Answer:
Step-by-step explanation:
just take 8.5% of 28,020 off for ever year until you get to 2030.
8.5% is 2381.70
Take 2381.70 of 28,020 each year
Find the value of this expression if x=-9.
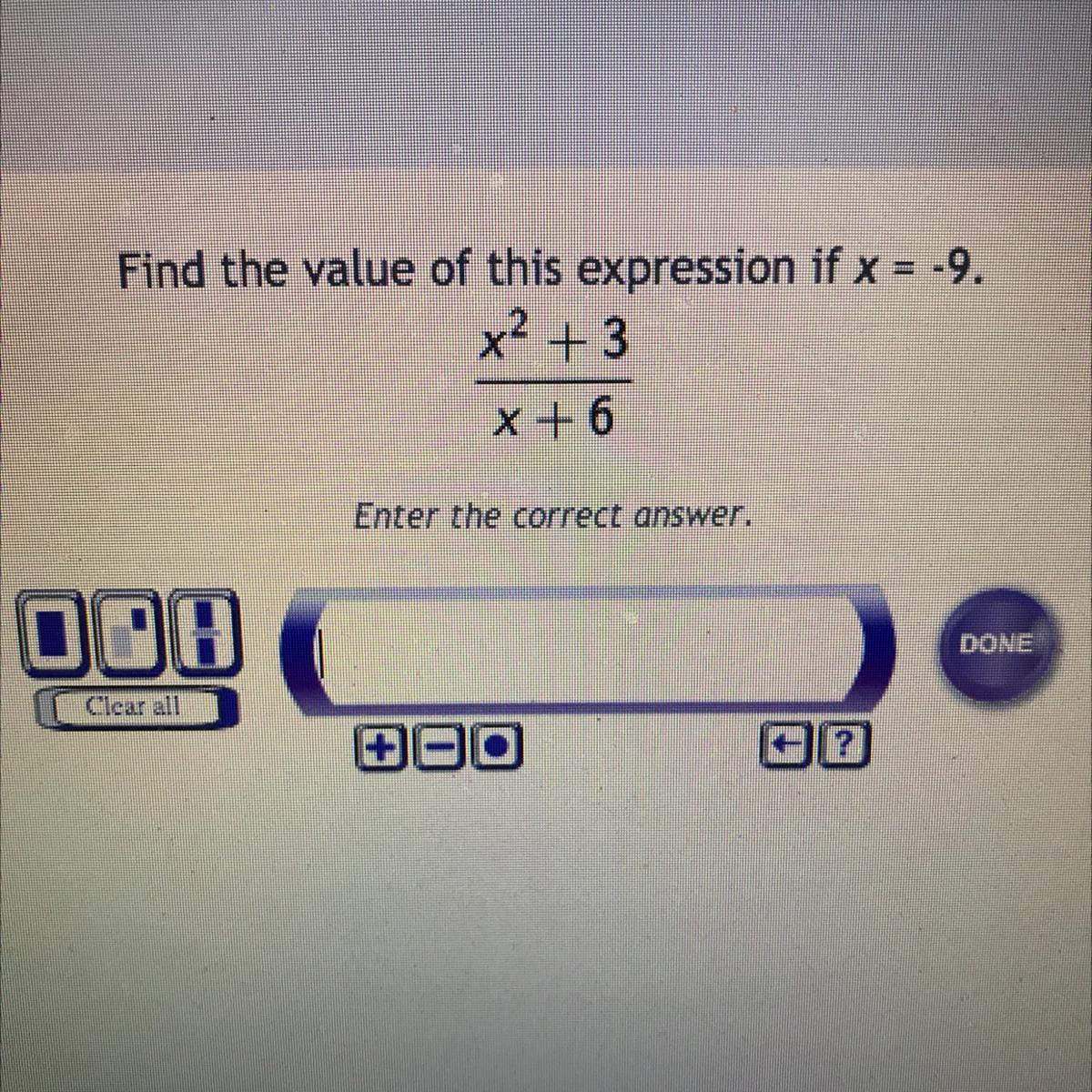
Answers
Answer:
if x = -9
then, (-9)×(-9) + 3
= 81 +3
= 84
(-9) + 6
= -3
so, ans is = 84/(-3)
= (-84)/3