Answers
Answer:
Option A:\( {y}^{5} + 13x + 12\)Step-by-step explanation:
As,
A trinomial contains 3 terms which the above option has,
and here 12 is the constant term .
Hence,
Required option is A.
Related Questions
A store purchased a chair for $10.00 and sold it to a customer for 50% more than the purchase price. The customer was charged 7% tax when the chair was sold. What was the customer's total cost for the chair? I
Answers
Answer:
$16.05
Step-by-step explanation:
50% of 10.00
0.50 x 10.00 = 5.00
10.00 + 5.00 = 15.00
7% of 15.00
0.07 x 15.00 = 1.05
15.00 + 1.05 = 16.05
solve each system by substitution. y=2x-1 3x+8y=11
Answers
X=1 y=1
Answer:
y=2x-1 ---(1)
3x+8y=11 ---(2)
sub (1) into (2)
3x+8(2x-1)=11
3x+16x-8=11
19x=19
X= 1
sub X= 1 into (1)
y= 2(1)-1
y=1
One month before an election, a poll of 630 randomly selected voters showed 55% planning to vote for a certain candidate. A week later it became known that he had had an extramarital affair, and a new poll showed only 53% of 1010 voters supporting him. Do these results indicate a decrease in voter support for his candidacy?
Determine the test statistic. z= (Round to two decimal places as needed.)
Find the P-value.
estimate that difference, p1−p2, with a 95% confidence interval
Answers
The statistics are as follows:
- Test Statistic: The calculated test statistic is approximately 1.02.
- P-value: The P-value associated with the test statistic of 1.02 is approximately 0.154.
- Confidence Interval: The 95% confidence interval for the difference in proportions is approximately -0.0186 to 0.0786.
To solve the problem completely, let's go through each step in detail:
1. Test Statistic:
The test statistic can be calculated using the formula:
z = (p1 - p2) / √[(p_cap1 * (1 - p-cap1) / n1) + (p_cap2 * (1 - p_cap2) / n2)]
We have:
p1 = 0.55 (proportion in the first poll)
p2 = 0.53 (proportion in the second poll)
n1 = 630 (sample size of the first poll)
n2 = 1010 (sample size of the second poll)
Substituting these values into the formula, we get:
z = (0.55 - 0.53) / √[(0.55 * (1 - 0.55) / 630) + (0.53 * (1 - 0.53) / 1010)]
z = 0.02 / √[(0.55 * 0.45 / 630) + (0.53 * 0.47 / 1010)]
z ≈ 0.02 / √(0.0001386 + 0.0002493)
z ≈ 0.02 / √0.0003879
z ≈ 0.02 / 0.0197
z ≈ 1.02 (rounded to two decimal places)
Therefore, the test statistic is approximately 1.02.
2. P-value:
To find the P-value, we need to determine the probability of observing a test statistic as extreme as 1.02 or more extreme under the null hypothesis. We can consult a standard normal distribution table or use statistical software.
The P-value associated with a test statistic of 1.02 is approximately 0.154, which means there is a 15.4% chance of observing a difference in proportions as extreme as 1.02 or greater under the null hypothesis.
3. Confidence Interval:
To estimate the difference in proportions with a 95% confidence interval, we can use the formula:
(p1 - p2) ± z * √[(p_cap1 * (1 - p_cap1) / n1) + (p_cap2 * (1 - p_cap2) / n2)]
We have:
p1 = 0.55 (proportion in the first poll)
p2 = 0.53 (proportion in the second poll)
n1 = 630 (sample size of the first poll)
n2 = 1010 (sample size of the second poll)
z = 1.96 (for a 95% confidence interval)
Substituting these values into the formula, we get:
(0.55 - 0.53) ± 1.96 * √[(0.55 * (1 - 0.55) / 630) + (0.53 * (1 - 0.53) / 1010)]
0.02 ± 1.96 * √[(0.55 * 0.45 / 630) + (0.53 * 0.47 / 1010)]
0.02 ± 1.96 * √(0.0001386 + 0.0002493)
0.02 ± 1.96 * √0.0003879
0.02 ± 1.96 * 0.0197
0.02 ± 0.0386
The 95% confidence interval for the difference in proportions is approximately (0.02 - 0.0386) to (0.02 + 0.0386), which simplifies to (-0.0186 to 0.0786).
To know more about confidence intervals, refer here:
https://brainly.com/question/32546207#
#SPJ11
Which table of values represents a linear function?
A
�
x
�
y
1
1
3
3
3
3
0
0
7
7
−
5
−5
9
9
−
8
−8
B
�
x
�
y
−
2
−2
−
6
−6
0
0
−
5
−5
3
3
−
4
−4
5
5
−
3
−3
C
�
x
�
y
−
4
−4
−
8
−8
−
2
−2
−
5
−5
0
0
−
2
−2
2
2
1
1
D
�
x
�
y
3
3
6
6
4
4
4
4
5
5
1
1
6
6
−
1
−1
Answers
The table of values that represents a linear function include the following: C. table C.
What is a linear function?In Mathematics and Geometry, a linear function refers to a type of function whose equation is graphically represented by a straight line on the xy-plane or cartesian coordinate.
This ultimately implies that, a linear function has the same (constant) slope and it is typically used for uniquely mapping an input variable to an output variable, which both increases simultaneously.
In this context, we have the following table C with constant slopes:
Slope = (1 + 2)/(-1 + 3) = (4 - 1)/(0 + 2)
Slope = 3/2
Read more on linear function here: brainly.com/question/27325295
#SPJ1
Missing information:
The question is incomplete and the complete question is shown in the attached picture.

Ellen plans to leave an 18 percent tip with her $23.75 lunch bill. Which of these expressions could she use to determine her total bill? Check all that apply.
Ellen plans to leave an 18 percent tip with her $23.75 lunch bill. Which of these expressions could she use to determine her total bill? Check all that apply.
Answers
You probably forgot to mention the options. After a little research, I was able to get the complete question with the mentioned options you should have mentioned. The potential complete question is:
Ellen plans to leave an 18 percent tip with her $23.75 lunch bill. Which of these expressions could she use to determine her total bill?
Check all that apply.
(1.18)(23.75) 23.75 – [(0.18)(23.75)] 23.75 + [(0.18)(23.75)] (0.18)(23.75)
Answer:
The expression \(23.75\:+\:\left[\left(0.18\right)\left(23.75\right)\right]\) will determine her total bill.
Step-by-step explanation:
Total lunch bill = $23.75
As he pans to 18% tip of the total bill, so the expression will be:
\(=23.75\:+\:\left(\:18\%\:\:of\:\:23.75\:\right)\)
\(=23.75\:+\:\left(\:\frac{18}{100}\:\times \:23.75\:\right)\)
\(=23.75\:+\:\left(\:0.18\times \:23.75\:\right)\)
or
\(=23.75\:+\:\left[\left(0.18\right)\left(23.75\right)\right]\)
Therefore, the expression \(23.75\:+\:\left[\left(0.18\right)\left(23.75\right)\right]\) will determine her total bill.
Answer:
(1.18)(23.75)
23.75 + [(0.18)(23.75)]
Step-by-step explanation:
ur welcome
What condition has no solution?
Answers
A condition that is impossible to satisfy has no solution.
Mathematics can be a powerful tool for solving many problems, but there are some issues that can't be solved.
In particular, any condition that is impossible to satisfy, such as the logical statement ‘this statement is false’, has no solution in mathematics. This is because the statement can’t be proven true or false, and so it can’t be solved.
An unsolvable condition is one that cannot be solved using any combination of logical and mathematical steps.
This means that no matter how much time and effort is put in, a solution to the problem cannot be discovered.
To learn more about solution, click here:
https://brainly.com/question/25785824
#SPJ4
The U. S. Coast Guard surveyed a simple random sample of 300 small boats in the Cape Cod area found 120 in violation of one or more safety regulations. Construct and interpret a 99. 8% confidence for p, the proportion of all unsafe small boats
Answers
We are 99.8% confident that the true proportion of all unsafe small boats in the Cape Cod area lies between approximately 0.3161 and 0.4839.
To construct a confidence interval for the proportion of all unsafe small boats (p), we can use the formula for a confidence interval for a proportion:
Confidence interval = \(\hat{p}\) ± z * √((\(\hat{p}\) * (1 - \(\hat{p}\))) / n)
Where:
\(\hat{p}\) is the sample proportion of unsafe small boats (120/300 = 0.4)
z is the z-score corresponding to the desired confidence level (99.8% corresponds to a z-score of approximately 2.967)
n is the sample size (300)
Now we can plug in the values and calculate the confidence interval:
Confidence interval = 0.4 ± 2.967 * √((0.4 * (1 - 0.4)) / 300)
Simplifying the expression within the square root:
Confidence interval = 0.4 ± 2.967 * √(0.24 / 300)
Confidence interval = 0.4 ± 2.967 * √(0.0008)
Confidence interval = 0.4 ± 2.967 * 0.0283
Confidence interval = 0.4 ± 0.0839
Confidence interval ≈ (0.3161, 0.4839)
Interpretation:
We are 99.8% confident that the true proportion of all unsafe small boats in the Cape Cod area lies between approximately 0.3161 and 0.4839. This means that, based on the sample data, we can estimate with high confidence that the proportion of unsafe small boats in the Cape Cod area falls within this range.
Learn more about confidence interval here
https://brainly.com/question/32546207
#SPJ4
in a class of 50 students, 28 participate in mathcounts, 21 participate in science club, and 6 students participate in neither. how many students participate in both mathcounts and science club?
Answers
Answer:
5
Step-by-step explanation:
28+21+6=55
Answer:55-50
what is 247x126 pllzzz answer quick
Answers
Answer:
31122
Step-by-step explanation:
Hope this helps.
Answer:
the answer is 31,122
Two identical squares of sides 17 cm overlap to form a rectangle as shown below. The shaded part is the overlapping region

Answers
To start with, we are told that the two shapes are squares. This means that they have the same length and breadth. From the diagram, we can see that one of the sides of the squares is 17 cm. This means that the squares are a square of 17cm in length.
If the original length is 17, and the overlapped length of the figure we have is 30 cm. Then we can say that since there exist 2 squares, 30/2 = 15 cm. If the overlapped length is 15 cm, this means that the part that is shaded is 2 cm in length.
Applying area of a rectangle formula
length * breadth = 17 * 2 = 24 cm²to learn more about area of shaded regions, see here https://brainly.com/question/10090807
5+|6-5x|=14 Absolute Value
Answers
Answer:
3/5
Step-by-step explanation:
5+6+5x=14
11+5x=14
5x=3
THEREFORE X=3/5
Answer:
3/5 s the answer hope this help
Step-by-step explanation:
What is a discrete probability distribution? What are the two conditions that determine a probability distribution?
Answers
Discrete probability distribution is the events are those with a finite number of outcomes and the two conditions are that the each value is between 0 and 1, and the sum of all values is 1.
According to the statement
we have to explain all about the discrete probability distribution and its two conditions which determine that the this probability is a probability distribution.
So, For this purpose, we know that the
Discrete events are those with a finite number of outcomes
And this is other way that we defines that the A discrete probability distribution counts occurrences that have countable or finite outcomes. This is in contrast to a continuous distribution, where outcomes can fall anywhere on a continuum.
The main example of this is Binomial.
And the two conditions of the probability distribution is :
The probability of each value of the discrete random variable is between 0 and 1, inclusiveand the sum of all the probabilities is 1.
So, Discrete probability distribution is the events are those with a finite number of outcomes and the two conditions are that the each value is between 0 and 1, and the sum of all values is 1.
Learn more about discrete probability distribution here
https://brainly.com/question/17145091
#SPJ4
2 Bill is shopping for new shoes. He found 3 pairs of shoes that he likes, while he is in line he finds a pair of socks for $7 that he also purchases. If all three pair of shoes are the same price and he spends $70 total for the 3 pairs of shoes and 1 pair of socks. How much does each pair of shoes cost? Your answer
Answers
Answer: Each pairs of shoes cost $21.
I NEED HELP!! WILL MARK BRAINLIEST!!!

Answers
Question 8: The slope of line (m) = \(\frac{7}{4}\)
The y-intercept will be b = 1
The equation is \(7x-4y +1 =0\)
Define the term line equation?the equation of a line is a formula that describes the relationship between the x and y coordinates of points on the line. The most commonly used form of the equation of a line is the slope-intercept form: y = mx + b, where m is the slope of the line and b is the y-intercept.
Given: Points \((x_{1}, y_{1} ) and (x_{2}, y_{2} )\) are (4, 8) and (12, 22)
We know the slope of line (m) = \(\frac{y_{2}-y_{1} }{x_{2}-x_{1}}\)
So, the slope of line (m) = \(\frac{22-8}{12-4}\) = \(\frac{14}{8} = \frac{7}{4}\)
the slope of line (m) = \(\frac{7}{4}\)
Equation of line, y = mx + b,
here b is the y-intercept, y = \(y_{1}\) = 8, and x = \(x_{1}\) = 4,
so, \(8=\frac{7}{4}*4 +b\)
Therefore, y-intercept by b = 1.
Equation \(y=\frac{7}{4}x +1\)
Therefore, the equation is \(7x-4y +1 =0\)
To know more about equation, visit:
https://brainly.com/question/2228446
#SPJ1
Subtract - 7x - 9 from -4c2 + 3x - 5.
Answers
Answer:
this is the real answer
\( \frac{664}{5} \)
Solution -4x2+3x-5(-7x-9)
Rationalizing factor of cube root 5
Answers
Answer:
You cube your answer that is the numerator and denominatorJorge gives an equal number of marbles to 6 friends. Which could be the total number of marbles he gave to his friends?

Answers
Answer:
D. 60
Step-by-step explanation:
To find an equal number of numbers the sum has to be equal to 6*x.
Answer:
If he gave it all to his friends then its D. If it is counting him too then C.
Step-by-step explanation:
A gardener is installing fence around his garden. Let x represent the width of the garden, in feet. The perimeter of the garden is 8x + 8. Which expression represents the length of the garden? A. 2x + 2 B. 3x + 4 C. 6x + 8 D. 8x + 8 – 2x
Answers
Answer:
B. 3x + 4
Step-by-step explanation:
Perimeter garden = 8x + 8
Perimeter = 2 * length + 2 * width
Width = x
8x + 8 = 2 * length + 2x
8x - 2x + 8 = 2* length
6x + 8 = 2 * length
3x + 4 = length
what’s the answer ??

Answers
Answer:
Left box: 1/2n
Right box = 2n - 22
Step-by-step explanation:
Since we want one-half the number and n represents the unknown number, we have 1/2n on the left-hand side of the equation.
Thus, you want to put 1/2n in box on the left.
Twice the number and 22 less than this is given by: y: 2n - 22 less than this means we subtract. Thus, we have 2n - 22 as the numerator on the right hand side of the equation.
Thus, you want to put 2n - 22 in the box on the right.
y² + 7y-8
Please answer this and show work
Answers
The factorization of y²+7y-8 is (y+8)(y-1).
y²+7y-8
=y²+8y-y-8
=y(y+8)-1.(y+8)
=(y+8)(y-1)
The factors are (y+8), (y-1).
The method we are following here is factorize by grouping.
To learn more about factorization,
brainly.com/question/26791528
The correct question is-
Factor y²+7y-8.
What is the maximum possible value of the greatest common divisor of two consecutive terms of the sequence $a_n = n! + n$, where $n \ge 0$?
Answers
Answer:
2
Step-by-step explanation:
You want the largest possible greatest common divisor of consecutive terms of the sequence an = n! +n.
SequenceThe sequence starts off 2, 4, 9, 28, 125, ...
The first two terms have a GCD of 2. The remaining pairs of terms have a GCD of 1.
The maximum possible GCD of adjacent terms is 2.
<95141404393>
4x-5y>-5
Please solve
Answers
Answer:
x-intercept(s):
(−54,0)
y-intercept(s): (0,−1)
Step-by-step explanation:
Please help fill in the blanks, will give brainlist if correct
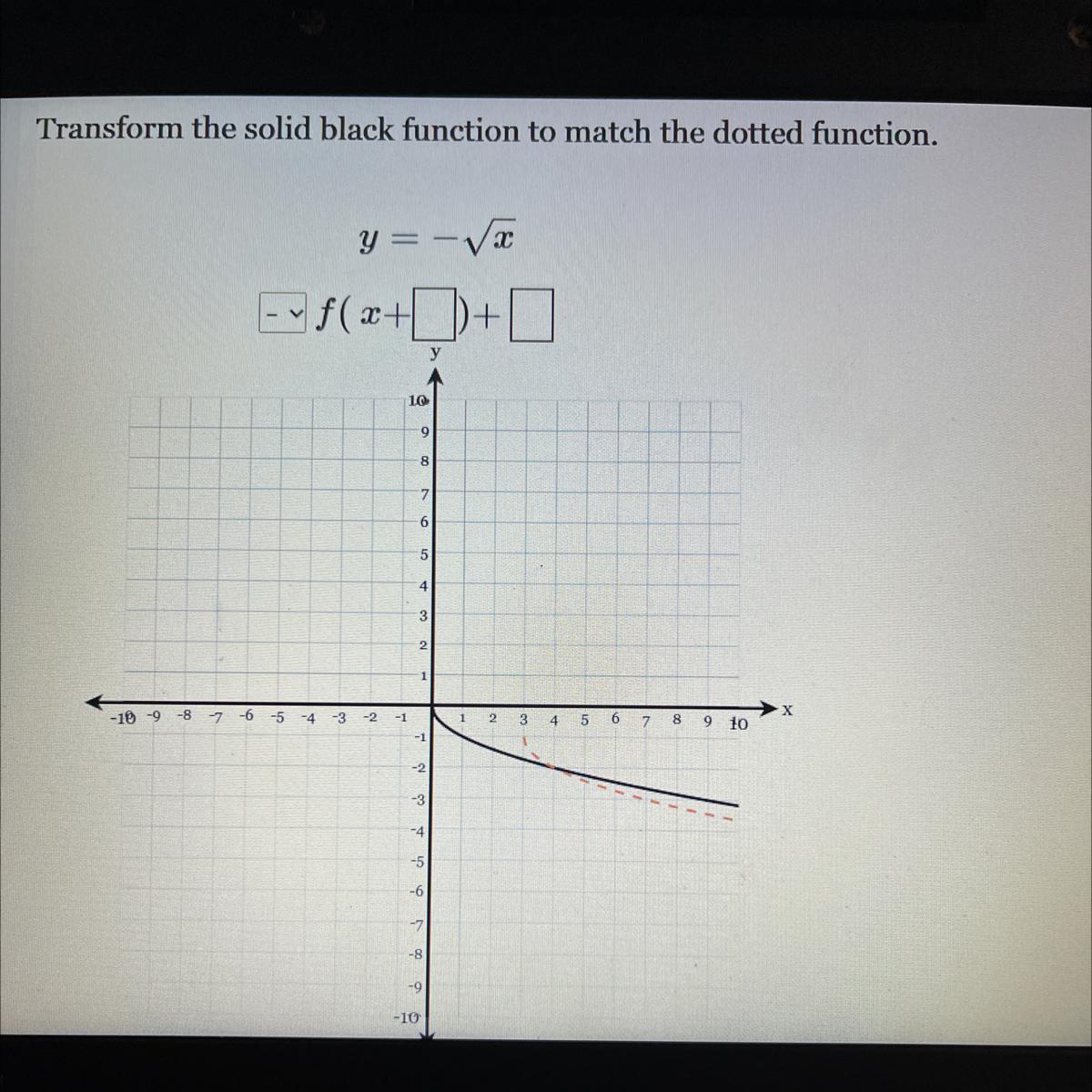
Answers
Answer:
Ayan na po
Nagpatulong lang ako sa gauthmath


How far can you travel at an average speed of 54mph over 3hrs?
Answers
Answer:
162 miles
Step-by-step explanation:
54(3) = 162
Answer:
162 miles?
Step-by-step explanation:
What is the midline equation of y = 10 cos (6x – 1) – 4?
Y=

Answers
Answer: -4
Step-by-step explanation: ur welc
Someone explain please

Answers
Answer:
SA = 94 ft²
Step-by-step explanation:
To find the surface area of a rectangular prism, you can use the equation:
SA = 2 ( wl + hl + hw )
SA = surface area of rectangular prism
l = length
w = width
h = height
In the image, we are given the following information:
l = 4
w = 5
h = 3
Now, let's plug in the information given to us to solve for surface area:
SA = 2 ( wl + hl + hw)
SA = 2 ( 5(4) + 3(4) + 3(5) )
SA = 2 ( 20 + 12 + 15 )
SA = 2 ( 47 )
SA = 94 ft²
If this answer helped you, please leave a thanks!
Have a GREAT day!!!
The population, P, of a city is changing at a rate dP/dt = 0.012P, in people per year. Approximately how many years will it take for the population to double? 57.762 58.108 83.333 166.667
Answers
The population, P, of a city is changing at a rate dP/dt = 0.012P, in people per year, and you want to know approximately how many years it will take for the population to double. To solve this problem, we can use the formula for exponential growth:P(t) = P₀ * e^(kt)
Here, P₀ is the initial population, P(t) is the population at time t, k is the growth rate, and e is the base of the natural logarithm (approximately 2.718).Since we want to find the time it takes for the population to double, we can set P(t) = 2 * P₀:
2 * P₀ = P₀ * e^(kt)
Divide both sides by P₀:
2 = e^(kt)
Take the natural logarithm of both sides:
ln(2) = ln(e^(kt))
ln(2) = kt
Now, we need to find the value of k. The given rate equation, dP/dt = 0.012P, tells us that k = 0.012. Plug this value into the equation:
ln(2) = 0.012t
To find t, divide both sides by 0.012:
t = ln(2) / 0.012 ≈ 57.762 years
So, it will take approximately 57.762 years for the population to double.
Learn more about population here
https://brainly.com/question/29885712
#SPJ11
Pweaseee Helps! 30 Pts!

Answers
Answer:
a) 6x³ − 5x² − 39x + 35
b) no
Step-by-step explanation:
(2x^3 − 5) (3x² + 5x − 7)
Distribute:
3x² (2x − 5) + 5x (2x − 5) − 7 (2x − 5)
Distribute again:
6x³ − 15x² + 10x² − 25x − 14x + 35
Combine like terms:
6x³ − 5x² − 39x + 35
5x − 2 is the opposite of 2x − 5, so you would get the negative of the answer from part (a).
What would you choose as x in the given series of clicks to calculate formulas automatically: file < options < x < automatic?
Answers
We should choose Formulas as X in the given series of clicks to calculate formulas automatically.
File < Options < (A) Formulas < Automatic
What are Formulas?In science, a formula is a concise way of symbolically expressing information, such as a mathematical formula or a chemical formula. In science, the term formula refers to the general construct of a relationship between given quantities. In mathematics, a formula is an identity that equates one mathematical expression to another, the most important of which are mathematical theorems. A formula (also known as a well-formed formula) is a logical entity that is constructed using the symbols and formation rules of a given logical language.We should choose Formulas as X in the given series of clicks to calculate formulas automatically.
Therefore, File < Options < (A) Formulas < Automatic
Know more about Formulas here:
https://brainly.com/question/21140562
#SPJ4
The complete question is given below:
What would you choose as X in the given series of clicks to calculate formulas automatically: File < Options < X < Automatic?
a. Formulas
b. Language
c. Proofing
d. Advanced
Write the equation of the tangent line to the graph of y = (6x² − 6x + 3)(1 + 2x) at x = 1. Check the reasonableness of your answer by graphing both the function and the tangent line.
Answers
To find the equation of the tangent line to the graph of y = (6x^2 − 6x + 3)(1 + 2x) at x = 1, we need to find the slope of the tangent line at that point and use the point-slope form of a line equation. Here's a summary of the solution:
Calculate the derivative of the function y = (6x^2 − 6x + 3)(1 + 2x) using the product rule and simplify the expression.
Evaluate the derivative at x = 1 to find the slope of the tangent line.
Substitute the coordinates of the point (1, f(1)) into the point-slope form of the line equation, where f(1) is the value of the function at x = 1.
Simplify the equation to obtain the equation of the tangent line.
To check the reasonableness of the answer, graph both the function and the tangent line using a graphing tool or software. Plot the function and the tangent line on the same graph to visually verify that the line is indeed tangent to the function at x = 1.
By taking the derivative of the function, evaluating it at x = 1, and applying the point-slope form, you can determine the equation of the tangent line. Graphing both the function and the tangent line together allows you to visually confirm the tangent relationship at x = 1.
To learn more about tangent click here:
brainly.com/question/10053881
#SPJ11
