Answers
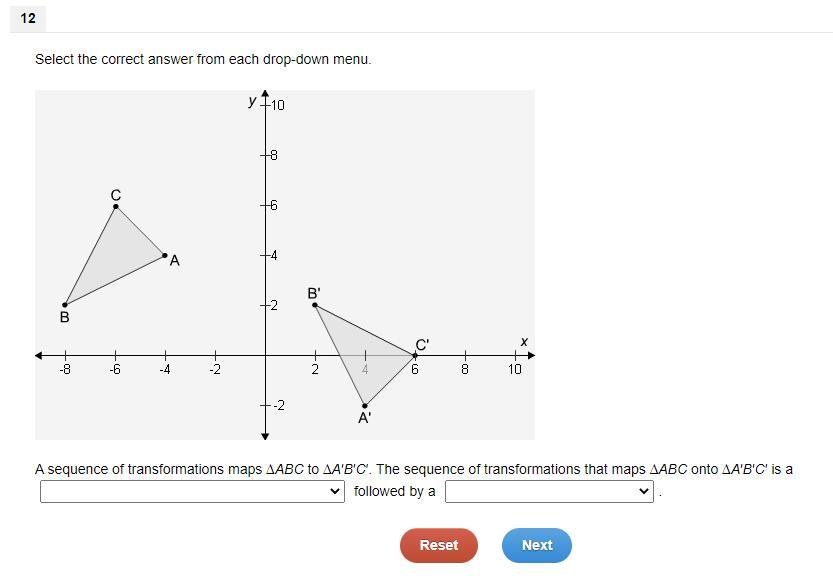
A sequence of transformations maps ∆ABC to ∆A′B′C′. The sequence of transformations that maps ∆ABC onto ∆A′B′C′ is a reflection across the line x = -3 followed by a reflection across the line y = x.
What is the explanation of the above transformation?Given that both triangles are congruent, we can infer or deduce that there were no dilations or contractions. Hence, since we are moving in a clockwise direction, we can submit that this was not a rotation but a reflection.
Hence the first sequence of reflection was such that:
∆ABC reflects onto ∆A′B′C′ across the line x = -3; followed by
a reflection across the line y = x.
Note that in geometry, reflection refers to the mirror image of an image. The line through which an image reflects is called the line of reflection.
Learn more about transformation:
https://brainly.com/question/11709244
#SPJ1
Related Questions
How does 899,451 compare to 756,451
Answers
899,452 is greater than 756,451
899,452 > 756,451
Given,
899,451
756,451
We need to compare these numbers.
What is the place value of a large number?Consider 967543
3 - ones
4 - tens
5 - hundreds
7 - thousands
6 - ten thousands
9 - hundred thousands
Find the highest place value in 899,452.
- 8 hundred thousands
We can read this as eight hundred ninety-nine thousand four hundred fifty-two.
Find the highest place value in 756,451.
- 7 hundred thousands
Seven hundred fifty-six thousand four hundred fifty one
Thus,
899,452 is greater than 756,451
899,452 > 756,451
Learn more about place value here:
https://brainly.com/question/21467438
#SPJ1
5x + 13
36
Q
Р
30
6x-2
R
find x
Answers
so what is 36 Q P 30 and R for? or is the question 5x+13×36QP×30×6x-2×R?
Pablo solved the polynomial equations given in the table. Determine whether each polynomial is correct. Select Correct or Incorrect for each equation.
Equation
(b²+7b-9)+(4b-6b²) = -8b² + 14b-9
(4a+6)-(3a²-9a+1)=-3a²+ 13a +5
(12c-8c²)+(5c²- 10c) = -3c²+2c
Answers
The first equation is incorrect.
The second equation is correct.
The third equation is correct.
Let's go through each equation and determine whether it is correct or incorrect:
(b²+7b-9)+(4b-6b²) = -8b² + 14b-9
To determine if this equation is correct, we need to simplify both sides and check if they are equal.
On the left side:
(b²+7b-9)+(4b-6b²) = b² - 6b² + 7b + 4b - 9 = -5b² + 11b - 9
On the right side:
-8b² + 14b - 9
Comparing both sides, we can see that -5b² + 11b - 9 is not equal to -8b² + 14b - 9. Therefore, the equation is incorrect.
(4a+6)-(3a²-9a+1)=-3a²+ 13a +5
Again, we need to simplify both sides of the equation and check if they are equal.
On the left side:
(4a+6)-(3a²-9a+1) = 4a + 6 - 3a² + 9a - 1 = -3a² + 13a + 5
On the right side:
-3a² + 13a + 5
Comparing both sides, we can see that -3a² + 13a + 5 is equal to -3a² + 13a + 5. Therefore, the equation is correct.
(12c-8c²)+(5c²- 10c) = -3c²+2c
Again, let's simplify both sides and compare them.
On the left side:
(12c-8c²)+(5c²- 10c) = 12c - 8c² + 5c² - 10c = -3c² + 2c
On the right side:
-3c² + 2c
Comparing both sides, we can see that -3c² + 2c is equal to -3c² + 2c. Therefore, the equation is correct.
For more such questions on equation visit:
https://brainly.com/question/17145398
#SPJ8
Antonio is in a bowling league. His goal is to score an average of at least 100 points per game. His scores so far are 105,95,97,82, and 110. How many points can Antonio score in his next game to reach his goal? Show your work.
Please show work I need it so please have step by step I will give brainly thanks!
Answers
Antonio needs to score at least 111 points in his next game to reach his goal of an average of at least 100 points per game.
What are basic arithmetic operations?
Basic arithmetic operations are fundamental mathematical calculations that involve manipulating numerical values using a set of basic operations.
To find out how many points Antonio needs to score in his next game to reach an average of at least 100 points per game, we can use the following formula:
Total points needed = (number of games + 1) × average score goal - total points scored so far
In this case, Antonio has played 5 games, so the number of games he will have played after his next game is 6. His goal is an average of 100 points per game, so his average score goal is 100. The total points he has scored so far is:
105 + 95 + 97 + 82 + 110 = 489
Substituting these values into the formula, we get:
Total points needed = (6) × (100) - 489
Total points needed = 600 - 489
Total points needed = 111
Hence, Antonio needs to score at least 111 points in his next game to reach his goal of an average of at least 100 points per game.
To learn more about arithmetic operations, visit:
https://brainly.com/question/4721701
#SPJ1
solve the equation. check your solution
x - 7 = 87
Answers
Answer:
94
Step-by-step explanation:
x - 7 = 87
x - 7 + 7= 87 + 7
x = 94
LS check
x - 7
94 - 7
87
RS check
87
LS = RS
A large tank is filled to capacity with 700 gallons of pure water. Brine containing 3 pounds of salt per gallon is pumped into the tank at a rate of 7 gal/min. The well-mixed solution is pumped out at the same rate. Find the number A(t) of pounds of salt in the tank at time t.
Answers
Answer:
The number A(t) of pounds of salt in the tank at time 't' is;
\({A(t)}= -2,100 \times e^{\dfrac{-t}{100} } + 2,100\)
Step-by-step explanation:
In the question, we have;
The volume of pure water initially in the tank = 700 gal
The concentration of brine pumped into the tank = 3 pounds per gallon
The rate at which the brine is pumped into the tank, = 7 gal/min
The rate at which the well mixed solution is pumped out = The same 7 gal/min
The number of pounds of salt in the tank at time 't' is found as follows;
The rate of change in A(t) with time = The rate of salt input - The rate of salt output
\(The \ rate \ of \ change \ in \ A(t) \ with \ time = \dfrac{dA}{dt}\)
The rate of salt input = 7 gal/min × 3 lbs/gal = 21 lbs/min
The rate of salt output = (A(t)/700) lb/gal × 7 gal/min = (A(t)/100) lb/min
Therefore, we have;
\(\dfrac{dA}{dt} = 21 - \dfrac{A(t)}{100}\)
Therefore;
\(\dfrac{dA}{dt} + \dfrac{A(t)}{100}= 21\)
The integrating factor is \(e^{\int\limits {\frac{1}{100} } \, dx } = e^{\frac{x}{100} }\)
\(e^{\dfrac{t}{100} } \times \dfrac{dA}{dt} + e^{\dfrac{t}{100} } \times\dfrac{A(t)}{100}= e^{\dfrac{t}{100} } \times21\)
\(\dfrac{d}{dt} \left[ e^{\dfrac{t}{100} } \times{A(t)}{} \right]= e^{\dfrac{t}{100} } \times21\)
Using an online tool, we get;
\({A(t)}= c_1 \times e^{\dfrac{-t}{100} } + 2,100\)
At time t = 0, A(t) = 0
We get;
\(0= c_1 \times e^{\dfrac{-0}{100} } + 2,100\)
c₁ = -2,100
Therefore, the number A(t) of pounds of salt in the tank at time 't' is given as follows;
\({A(t)}= -2,100 \times e^{\dfrac{-t}{100} } + 2,100\)
Write an equation in slope - intercept form for the line that passes through the given paint andis parallel to the given equation.4.(-4, 2), y = -1/2x+ 6
Answers
When two equation are parallel it means they have the same slope.
You have the equation:
\(y=-\frac{1}{2}x+6\)You can identify the slope(m) as the number next to the x in equation that follow the next form:
\(y=mx+b\)Then, the equation parallel to the given equation has an slope of:
m= - 1/2Now, if we have a point of the equation we can find the value of the b (y-intercept) in the slope - intercept form of a lineal equation:
\(y=mx+b\)The given point is ( -4 , 2)
You have the next values:
m= - 1/2
y= 2
x= -4
Then we substitute that values:
\(2=-\frac{1}{2}(-4)+b\)And clear the b:
\(2=\frac{4}{2}+b\)\(2=2+b\)\(2-2=2-2+b\)\(0=b\)Now, we get the value of the slope (m= - 1/2) and the y-intercept (b=0)
We can write the equation in slope - intercept form:
\(y=-\frac{1}{2}x+0\)or:
\(y=-\frac{1}{2}x\)[10 Points] In the standard numbering (shortlex ordering) of strings over the alphabet {a,b,c} what number corresponds to string cbbca cba cbc ?
Answers
The number corresponds to the given string is 237880
What is common ratio?
In a geometric sequence, ratio of each term to its immediately preceding term is always constant and is known as common ratio.
The shortlex ordering places the shortest sequences at the front of the list of sequences by cardinality (length), while sequences of the same length are arranged lexicographically.
Given: cbbca cba cbc
length =11
We need to find the number of strings that precede the given string over {a,b,c}
Number of strings of length 0 = 1
Number of strings of length 1 = 3
Number of strings of length 2 = 3²
'
'
'
'
'
Number of strings of length 10 = 3¹⁰
In general, number of strings formed withy length n = 3ⁿ
Add all the strings of length 0 to 10
1+3+3²+......................+3⁻¹⁰
By simple geometric sum formula ,
we have
= (3¹¹⁻¹)/(3-1) = 88573
Number of strings of length 11 starting with either 'a' or 'b' = 2*3¹⁰
Number of strings of length 11 starting with 'ca' = 3⁹
Number of strings of length 11 starting with 'cba' = 3⁸
Number of strings of length 11 starting with 'cbba' or 'cbbb' = 2*3⁷
Number of strings of length 11 starting with 'cbbcaa' or 'cbbcab' = 2*3⁵
Number of strings of length 11 starting with 'cbbcaca' = 3⁴
Number of strings of length 11 starting with 'cbbcacbab' or 'cbbcacbaa' = 2*3²
Number of strings of length 11 starting with 'cbbcacbaca' = 3
Number of strings of length 11 starting with 'cbbcacbacba' or 'cbbcacbacbb' = 2
Total number of strings of length 11 preceding the given string is :
2+3+2*3² +3⁴+ 2*3⁵+ 2*3⁷+3⁸+3⁹ + 2*3¹⁰
= 149306
The number corresponds to the given string
= 88573 + 149306 +1
= 237880
To learn more about the common ratio from the given link
https://brainly.com/question/28762774
#SPJ4
(X+2b)(x-4b) simplified
Answers
Answer:
I will ASSUME X = x
x² + 2bx - 4bx - 8b²
x² - 2bx - 8b²
Answer:
\(\\\\\\-8b^2-2bx+x^2\)
Step-by-step explanation:
\((x+2b)(x-4b)\\=(x+2b)(x+(-4b)\\=(x)(x)+(x)(-4b)+(2b)(x)+(2b)(-4b)\\\\x^2-4bx+2bx-8b^2\\\\-8b^2-2bx+x^2\)
Find the set of the solutions of each of the following absolute value inequalities. Check your answers. |(x+1)/2 - x/2|<1/2
Answers
Thus, the solution set of the given absolute value inequalities is found as: 0 ≥ x ≥ -1
Explain about the absolute value inequalities:The comparison of the connection here between variable and its value is a compound inequality. In a compound inequality, a variable is compared across two ranges which may provide the variable's range by satisfying either one or both of the limitations .
The given absolute value inequalities :
|(x+1)/2 - x/2| < 1/2
Removing the modulus sign will for 2 equations:
(x+1)/2 - x/2 < 1/2 and (x+1)/2 - x/2 < - 1/2
Simplifying each:
(x+1)/2 - x/2 < 1/2
x/2 + 1/2 - x/2 < 1/2
Both values will get cancelled:
0 < 0
But 0 = 0
and (x+1)/2 - x/2 > - 1/2
x/2 + 1/2 - x/2 > -1/2
0 > -1 (correct)
here also the terms are getting completely cancelled to
Thus, the solution set of the given absolute value inequalities is found as: 0 ≥ x ≥ -1
Know more about the absolute value inequalities
https://brainly.com/question/27307313
#SPJ1
Complete question:
Find the set of the solutions of the following absolute value inequalities.
|(x+1)/2 - x/2| < 1/2
The number of people employed as medical assistants in a country was 373 thousand in 2003. By the year 2013, this number is expected to rise to 591 thousand. Let y be the number of medical assistants (in thousands) employed in the country in the year x, where x=0 represents 2003. a. Write a linear equation that models the number of people (in thousands) employed as medical assistants in the year x. b. Use this equation to estimate the number of people who will be employed as medical assistants in the year 2012.
Answers
a) The linear function is: \(y = 21.8x + 373\)
b) The estimate for the number of people employed in 2012 is of 569.2.
-----------------------------
A linear function has the following format:
\(y = mx + b\)
In which
m is the slope, that is, how much y changes when x changes by 1.b is the y-intercept, which is the value of y when x = 0.-----------------------------
Item a:
During the first year of observation, the number of people, in thousands of dollars, was of 373. Thus, the y-intercept is \(b = 373\)In 10 years, it changed from 373 to 591, thus, the slope is:\(m = \frac{591 - 373}{10} = 21.8\)
The function is:
\(y = 21.8x + 373\)
-----------------------------
Item b:
2012 is 9 years after 2003, thus, this is \(y(9)\)
\(y(9) = 21.8(9) + 373 = 569.2\)
The estimate for the number of people employed in 2012 is of 569.2.
A similar problem is given at https://brainly.com/question/24742848
1/5(5x-5)+3x=-9(1/3x+4)
Answers
The solution to the equation, 1/5(5x - 5) + 3x = -9(1/3x + 4), is solved as x = -5.
How to Solve an Equation?When we are given an algebraic equation that contains a variable, to solve for the variable means to find the value of that variable that will make the equation true.
Given the equation, 1/5(5x - 5) + 3x = -9(1/3x + 4), apply the distributive property to open the parentheses:
1/5(5x) + 1/5(-5) + 3x = -9(1/3x) -9(+4)
x - 1 + 3x = -3x - 36
Combine like terms:
x - 1 + 3x = -3x - 36
4x - 1 = -3x - 36
4x + 3x = 1 - 36
7x = -35
Divide both sides by 7:
7x/7 = -35/7
x = -5
Check to confirm the solution by plugging in the value of x into the equation:
1/5(5(-5) - 5) + 3(-5) = -9(1/3(-5) + 4)
1/5(-30) - 15 = -9(-5/3 + 4)
-21 = -21
Therefore, the solution to the equation is x = -5.
Learn more about the solution of an equation on:
https://brainly.com/question/25678139
#SPJ1
Cómo resolver con cambio de variable

Answers
The solution of the integral by variable change is equal to (1 / 6) · √(2 · x + 3)³ - (3 / 2) · √(2 · x + 3).
How to solve an integral by variable change
In this problem we find the definition of an integral, whose solution must be found by variable change, that is, a kind of algebraic substitution. First, write the complete expression:
∫ [x / √(2 · x + 3)] dx
Second, use algebraic substitution to simplify the expression:
u = 2 · x + 3
x = (u - 3) / 2
dx = du / 2
(1 / 2) ∫ [(u - 3) / (2 · √u)] du
(1 / 4) ∫ [(u - 3) / √u] du
(1 / 4) ∫√u du - (3 / 4) ∫ du / √u
Third, solve the integral:
(1 / 6) · √u³ - (3 / 2) · √u
Fourth, revert the algebraic substitution:
(1 / 6) · √(2 · x + 3)³ - (3 / 2) · √(2 · x + 3)
To learn more on indefinite integrals: https://brainly.com/question/28036871
#SPJ1
Differentiate y=x4 -x
Answers
Answer:
Step-by-step explanation:
To differentiate the function y = x^4 - x, we will use the power rule of differentiation. The power rule states that if f(x) = x^n, then the derivative of f(x) is f'(x) = nx^(n-1).
So, for y = x^4 - x, we can find the derivative as follows:
y' = 4x^3 - 1
So, the derivative of the function y = x^4 - x is y' = 4x^3 - 1.
Name 4 fractions that are equivalent to 9/12. Show your work
Answers
Answer:
3/4
6/8
12/15
15/18
Step-by-step explanation:
Answer:
3/4, 18/24, 27/36, 36/48
Step-by-step explanation:
9 and 12 can both be divided by 3 to equal 3/4
9/12 times 2 is 18/24
9/12 times 3 is 27/36
9/12 times 4 is 36/48
hope this helps:)
Will someone please help me solve this problem.

Answers
Answer:
A
Step-by-step explanation:
The answer is 64 since it can be a multiple of the numbers
Write an equation of the line that passes through the given points.
(-2,5) and (3,0)
Answers
9514 1404 393
Answer:
y = -x +3
Step-by-step explanation:
The 2-point form of the equation of a line is useful when 2 points are given.
y = (y2 -y1)/(x2 -x1)(x -x1) +y1
y = (0 -5)/(3 -(-2))(x -(-2)) +5
y = -5/5(x +2) +5
y = -x +3
(5+6) + what = 5 + (6+2)
Answers
Answer:
total is 13. so 5+6 = 11 and total is 13 so 13 - 11 = 2
Christine received a $1500 bonus. She decided to invest it in a 5-year certificate of deposit (CD) with an annual interest rate of 1.44% compounded annually. Answer the questions below. Do not round any intermediate computations, and round your final answers to the nearest cent. If necessary, refer to the list of financial formulas. (a) (b) Assuming no withdrawals are made, how much money is in Christine's account after 5 years? How much interest is earned on Christine's investment after 5 years? X S
Answers
a) Assuming Christine makes no withdrawals, her $1,500 investment in a 5-year certificate of deposit with an annual interest rate of 1.44% compounded annually, will be worth (future value of) $1,611.16 after 5 years.
b) The total interest earned on Christine's investment after 5 years is $111.16.
What is the future value?The future value represents the compounded present value.
The future value can be computed using an online finance calculator.
N (# of periods) = 5 years
I/Y (Interest per year) = 1.44%
PV (Present Value) = $1,500
PMT (Periodic Payment) = $0
Results:
Future Value (FV) = $1,611.16
Total Interest = $111.16
Learn more about the future value at https://brainly.com/question/27979326
#SPJ1
3/5 + 7/8 + 3/10 = plssss ghelp
Answers
Answer:
\(\frac{71}{40}\)
or
71/40
Explanation:
\(\frac{3}{5} + \frac{7}{8} + \frac{3}{10}\)
LCM (Least Common Multiple) of 5, 8, and 10 = 40
5 × 8 = 40
8 × 5 = 40
10 × 4 = 40
(8 × \((\frac{3}{5} )\) ) + (5 × \((\frac{7}{8} )\) ) + (4 × \((\frac{3}{10} )\) )
\(\frac{24}{40} + \frac{35}{40} + \frac{12}{40}\)
\(\frac{71}{40}\)
17. the shaft is supported at its ends by two bearings a and b and is subjected to the forces applied to the pulleys fixed to the shaft. determine the resultant internal loadings acting on the cross section at point d. the 400-n forces act in the -z direction and the 200-n and 80-n forces act in the y direction. the journal bearings at a and b exert only y and z components of force on the shaft.
Answers
The resultant normal force at point D along the x-direction is \(F_D_x=0\) and the resultant shear force at point D along the y-direction is \(F_D_y=154.29N\)
Consider the equilibrium of the shaft.
Resolve the forces along the y-direction.
\(R_A_y+R_B_y=200+200+80+80R_A_y+R_B_y=560-----(1)\)
Take moments about point B and about the z-axis:
\(R_A_y*1.4=2*100*0.7+2*80*0.4\\\\R_A_y=245.71\)
Substitute the above value in equation (1).
\(245.71+R_B_y=560\\\\R_B_y=314.29N\)
Resolve the forces along the z-direction.
\(R_A_z+R_B_z=385+385R_A_z+R_B_z=770-----(2)\)
Take moments about point B and about the y-axis:
\(R_A_z*1.4=2*385*1.1\\\\R_A_z=605N\)
Substitute the above value in equation (2).
\(605+R_B_z=770\\\\R_B_z=165N\)
a) The resultant normal force at point D along the x-direction is
\(F_D_x=0\)
b) The resultant shear force at point D along the y-direction is
\(F_D_y-R_B_y+2*80=0\\\\F_D_y-314.29+160=0\\F_D_y=154.29N\)
To know more about the resultant normal forces:
https://brainly.com/question/14292810
#SPJ4

11) What is probability of not being born in a month that starts with avowel?
Answers
total number of months: 12
Months that don't start with a vowel: 9
January
February
March
May
June
July
September
November
December
probability of not being born in a month that starts with a
vowel ( or being born in a month that starts with a consonant)
9/12 = 0.75
No file, guessing, or random answers
a population has a standard deviation of 20.2. what is the standard error of the sampling distribution if the sample size is 121? round your answer to two decimal places.
A) 1.84
B) 1.44
C) 1.55
D) 1.68
Answers
Answer:
A) 1.84
Step-by-step explanation:
I just took the quiz
The standard error of the sampling size is 121 is 1.84
What is standard error?"a measure of the statistical accuracy of an estimate, equal to the standard deviation of the theoretical distribution of a large population of such estimates."
"In the standard error formula, the factor sqrt[ (N - n ) / (N - 1) ] is called the finite population correction or fpc. When the population size is very large relative to the sample size, the fpc is approximately equal to one; and the standard error formula can be approximated by:
σx = σ / sqrt(n)."
Given,
Standard deviation of the sample is 20.2
and sample size is = 121
Standard error is = \(\sigma/\sqrt(n)\)
i.e. standard error = \(20.2/\sqrt(121)\) = 20.2/ 11 = 1.84
Hence, option A is true.
To know more about standard error here
https://brainly.com/question/13179711
#SPJ2
Please please please help me
I really need to pass this I will give brainliest and a lot of points please just help me solve this correctly

Answers
The length of side AB is about 5.87 units.
How to find the side of a right triangle?The triangle ABC is a right angle triangle. A right angle triangle is a triangle that has one of its angles as 90 degrees.
Therefore, let's find the length AB in the right triangle.
Using trigonometric ratios,
cos 33 = adjacent / hypotenuse
Therefore,
Adjacent side = AB
hypotenuse side = 7 units
cos 33° = AB / 7
cross multiply
AB = 7 cos 33
AB = 7 × 0.83867056794
AB = 5.87069397562
AB = 5.87 units
learn more on right triangle here: https://brainly.com/question/30966657
#SPJ1
how many red apple are in the jar?
Answers
Answer:
2
Step-by-step explanation:
A bag contains colored tiles.
• 3 tiles are red
• 6 tiles are green
• 3 tiles are blue
A tile will be randomly selected from the bag.What is the probability in decimal form that the tile selected will
be green?
Answers
0.5 or 50% because there are 12 tiles and 6 are green. 6/12 probability simplifies to 1/2 = 0.5 = 50%
pls help pls help pls help pls help

Answers
Answer:
12 inches
Step-by-step explanation:
Look at the figure below: an image of a right triangle is shown with an angle labeled y degrees If sin y° = s divided by 8 and tan y° = s divided by t, what is the value of cos y°?
cos y° = 8s
cos y° = 8t
cos y°= t / 8
cos y°=8 / t
Answers
Answer:
Cos y = t / 8
Step-by-step explanation:
Using the hints given in the question, the omitted tribagke will look like the triangle attached on the picture ;
From trigonometry :
Sin y = opposite / hypotenus
Sin y = s / 8
Opposite side = s ; hypotenus = 8
Tan y = opposite / Adjacent
Tan y = s / t
Adjacent side = t
Then ;
Cos y = Adjacent / hypotenus
Hence,
Cos y = t / 8

Answer:
the answer is :
cos y°= t / 8
Step-by-step explanation:
I promise! I got this right, and.....you are welcome.
40 POINTS PLEASE HELP ASAP YOU CAN SAVE MY LIFE WILL GIVE BRANLIEST
Which expression is equivalent to 8\sqrt(6)
A.\sqrt(14)
B.\sqrt(48)
C.\sqrt(96)
D.\sqrt(384)
Answers
Answer:
D. \sqrt(384)
Step-by-step explanation:
All you have to do is to expand the root the way I have done. I hope you understand and rate this answer well.
Don't forget 8 is the same as root(8) * root(8) which will give root(64) and the square root of 64 is 8.

Evaluate x^2 y for x = -5 and y = 3.
-75
-15
75
Answers
\(x = - 5\)
\(y = 3\)
_________________________________
\( {x}^{2} \times y = ({ - 5})^{2} \times 3 = 25 \times 3 = 75 \\ \)
Done♥️♥️♥️♥️♥️