Answers
Answer:
5.5
Step-by-step explanation:
57-29.5 / 10-5 = 5.5
Answer:
5.5
Step-by-step explanation:
The rate of change of a relationship is the ratio of corresponding changes in value. Symbolically, we indicate a change with the greek letter Δ (pronounced "delta"). A change in x is written Δx ("delta eks") and a change in y is written Δy ("delta why"). We're looking for the ratio Δy/Δx ("delta y over delta eks").
Mathematically, Δx and Δy are the differences between two corresponding x an y values. To pick these, we can take any two rows in our table. Let's pick rows 4 and 2, nice whole numbers:
\(\Delta{x}=20-10=10\\\Delta{y}=112-57=55\\\Delta{y}/\Delta{x}=55/10=11/2=5.5\)
We can confirm that this holds for every other pair, too - picking rows 2 and 1 as an example:
\(\Delta{x}=10-5=5\\\Delta{y}=57-29.5=27.5\\\Delta{y}/\Delta{x}=27.5/5=275/50=11/2=5.5\)
Related Questions
Can someone check this?
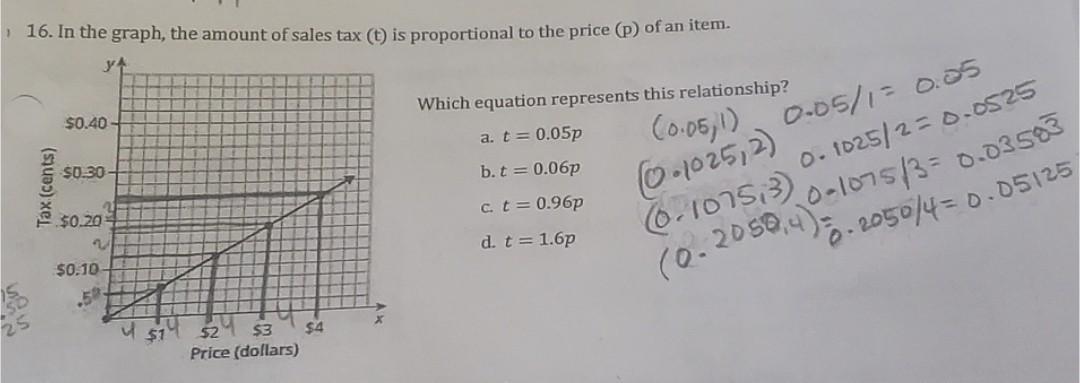
Answers
t = 0.06p
================================================
Explanation:
If we plugged in p = 2, then,
t = 0.06*p = 0.06*2 = 0.12
Showing that the point (2, 0.12) is on the diagonal line of the equation t = 0.06p
This matches with what the graph shows.
The y axis is incrementing by $0.025, aka 2.5 cents, each tickmark upward. Counting up 5 tickmarks will get you to $0.125 or 12.5 cents which is fairly close to 12 cents.
When you divided the x and y values to find the constant of variation, you divided in the wrong order. You should do y/x instead of x/y.
Fran baked 12 lemon tarts for her son, Bob. He gobbled up 4 tarts. What fraction of lemon tarts did bob eat?
Answers
Answer:
One third or 1/3
Step-by-step explanation:
5. Match the steps for constructing a congruent line segment to their pictures.
▼
▼
1. Step 2: Draw a second line
segment that is longer than the
first.
2. Step 4: That intersection is the
final endpoint of the copied
segment.
3. Step 1: Put the point of the
compass on one endpoint of the
segment to be copied. Change the
compass setting so that the
pencil end is just touching the
other endpoint, make a small arc
to see this.
4. Step 3: Without changing the
compass setting, put the
compass point on the new
endpoint on a new line. Make a
small arc that intersects the line.

Answers
We can see here that matching the steps for constructing a congruent line segment to their pictures, we have:
Step 1 - Picture a
Step 2 - Picture b
Step 3 - Picture c
Step 4 - Picture c.
What is a line segment?Any portion of a line with two ends and a set length is referred to as a line segment. It differs from a line that has neither a beginning nor an end and can be stretched in both directions.
The endpoints of a line segment can be used to define it. The portion of the line that begins at point A and finishes at point B, for instance, is known as the line segment AB.
A ruler or measuring tape can be used to determine the length of a line segment. The distance between two line segments' endpoints is the segment's length.
Learn more about line segment on https://brainly.com/question/280216
#SPJ1
Answer:
Step-by-step explanation:
Evaluate the expression if p=1/2 and S + 3 1/2
3P+2S+ 6 1/3
Answers
The Value of the expression with p = 1/2 and s = 3 1/2 is 87/6.
To evaluate the expression with p = 1/2 and s = 3 1/2, we substitute these values into the given expression:
3p + 2s + 6 1/3
Substituting p = 1/2 and s = 3 1/2:
3(1/2) + 2(3 1/2) + 6 1/3
We first simplify the expression within parentheses:
3/2 + 2(7/2) + 6 1/3
Next, we perform the multiplication:
3/2 + 14/2 + 6 1/3
The denominators are already the same, so we can add the fractions:
3/2 + 14/2 + 6 1/3 = (3 + 14)/2 + 6 1/3
Now, we need to find a common denominator for the fraction and the whole number:
(3 + 14)/2 + 6 1/3 = 17/2 + 6 1/3
To add the fractions, we need a common denominator of 6:
17/2 + 6 1/3 = 17/2 + 18/3
Now, we can find a common denominator for the fractions:
17/2 + 18/3 = 51/6 + 36/6
Adding the fractions:
51/6 + 36/6 = 87/6
Therefore, the value of the expression with p = 1/2 and s = 3 1/2 is 87/6.
To know more about Value .
https://brainly.com/question/13085451
#SPJ8
a gymnast practices 6 days each week.She practices the same number of ours each day.If she practices 120 hours in a 4 week period how many hours each day does she practice?
Answers
Answer: She practices 5 hours a day
Step-by-step explanation: 6 days a week x 4 weeks = 24 days
120 hours of practice / 24 days = 5 hours of practice a day
Answer:
5hrs
Step-by-step explanation:
120 ÷ 4 = 30. 30 ÷ 6 = 5. She practices for 5hrs a week.
The first three terms of a sequence are given. Round to the nearest thousandth (if necessary).
9,16,23,...
Find the 46th term.
Answers
The 46th term of the sequence is 324.
We have,
To find the 46th term of the sequence, we need to determine the pattern or rule that generates the terms.
Since the difference between consecutive terms is a constant value of 7, we know that this is an arithmetic sequence with a common difference
of 7.
To find the 46th term, we can use the formula for the nth term of an arithmetic sequence:
a(n) = a(1) + (n - 1) d
where a(1) is the first term, d is the common difference, and n is the term number we want to find.
Using the given information, we have:
a(1) = 9
d = 7
n = 46
Plugging these values into the formula, we get:
a (46) = 9 + (46 - 1)7
a (46) = 9 + 45 (7)
a(46) = 9 + 315
a(46) = 324
Therefore,
The 46th term of the sequence is 324.
Learn more about arithmetic sequence here:
https://brainly.com/question/10396151
#SPJ1
If you toss 7 fair coins in how many ways can you obtain at least two heads
Answers
When you toss 7 fair coins, the number of ways that it is possible to obtain at least two heads is 120 ways.
How to find the number of ways?First, find the number of outcomes when tossing 7 fair coins:
= 2 x 2 x 2 x 2 x2 x 2 x 2
= 128 outcomes
The total number of ways you can attain at least two heads is:
= Total outcomes - Outcomes where only 1 head is obtained - Outcomes where no heads are obtained
= 128 - 7 - 1
= 120 outcomes
Find out more on tossing a fair coin at https://brainly.com/question/24224393
#SPJ1
You might need: CalculatorZA=Round your answer to the nearest hundredth.СоMY?ProPro6TeaB4

Answers
∠A = 41.81°
Explanation:To get measure of angle A, we would apply trigonometry ratio SOHCAHTOA:
opposite = side opposite the angle = 4
hypotenuse = 6
adjacent = base = AC = ?
Since we have been given the opposite and the hypotenuse, we would use the sine ratio:
Sin A = opposite/hypotenuse
Sin A = 4/6
sin A = 0.6667
\(A=sin^{-1}(0.6667)\)A = 41.8129 degrees
To the nearest hundredth, ∠A = 41.81°
HELPP !
How many times does the graph of y=x2- 4 cross the x-axis?
Answers
Answer: 9
Step-by-step explanation:
Y=x2=9
You go on a bicycle trip of 210 miles in the mountains and 3/19 of the trip is Downhill, how many miles of the trip are not downhill?
Answers
Answer:
176.842105263
Step-by-step explanation:
multiply 210 by 3/19 to get the miles downhill and subtract that from 210 to get the uphill
On a bicycle trip of 210 miles in the mountains and 3/19 of the trip is Downhill, Number of miles of the trip are not downhill is, 33 miles.
What is the ratio?A ratio in mathematics is a comparison of two or more numbers that shows how big one is in comparison to the other. The dividend or number being divided is referred to as the antecedent, while the divisor or number that is dividing is referred to as the consequent.
Given that,
Total bicycle trip of 210 miles in the mountains
3/19 of the trip is Downhill
Downhill = 3/19(210)
Downhill = 33 miles
Miles of the trip are not downhill = 210-33
= 177
Hence, the not downhill distance is 177 miles
To know more about Ratio check:
https://brainly.in/question/7996583
#SPJ2
Consider the equation below.
f(x) = 2x^3 + 3x^2 − 18x
(a) Find the interval on which f is increasing. (Enter your answer in interval notation.)
Find the interval on which f is decreasing. (Enter your answer in interval notation.)
(b) Find the local minimum and maximum values of f.
local minimum
local maximum
(c) Find the inflection point.
(x, y) =
Find the interval on which f is concave up. (Enter your answer in interval notation.)
Find the interval on which f is concave down. (Enter your answer in interval notation.)
Answers
Answer:
\(\textsf{(a)} \quad \textsf{Increasing}: \quad \left(-\infty, \dfrac{-1-\sqrt{13}}{2}\right) \cup \left(\dfrac{-1+\sqrt{13}}{2}, \infty\right)\)
\(\textsf{Decreasing}: \quad \left(\dfrac{-1-\sqrt{13}}{2} < x < \dfrac{-1+\sqrt{13}}{2}\right)\)
\(\textsf{(b)} \quad \textsf{Minimum}: \quad \left(\dfrac{-1-\sqrt{13}}{2},\dfrac{19+13\sqrt{13}}{2}\right)\)
\(\textsf{Maximum}: \quad \left(\dfrac{-1+\sqrt{13}}{2},\dfrac{19-13\sqrt{13}}{2}\right)\)
\(\textsf{(c)} \quad \textsf{Point of inflection}: \quad \left(-\dfrac{1}{2}, \dfrac{19}{2}\right)\)
\(\textsf{Concave up}: \quad \left(-\dfrac{1}{2}, \infty\right)\)
\(\textsf{Concave down}: \quad \left(- \infty,-\dfrac{1}{2}\right)\)
Step-by-step explanation:
Given function:
\(f(x) = 2x^3 + 3x^2-18x\)
Part (a)\(\textsf{A function is \textbf{increasing} when the \underline{gradient is positive}}\implies f'(x) > 0\)
\(\textsf{A function is \textbf{decreasing} when the \underline{gradient is negative}} \implies f'(x) < 0\)
Differentiate the given function:
\(\implies f'(x)=3 \cdot 2x^{3-1}+2 \cdot 3x^{2-1}-18x^{1-1}\)
\(\implies f'(x)=6x^2+6x-18\)
Complete the square:
\(\implies f'(x)=6(x^2+x-3)\)
\(\implies f'(x)=6\left(x^2+x+\dfrac{1}{4}-3-\dfrac{1}{4}\right)\)
\(\implies f'(x)=6\left(x^2+x+\dfrac{1}{4}\right)-\dfrac{39}{2}\)
\(\implies f'(x)=6\left(x+\dfrac{1}{2}\right)^2-\dfrac{39}{2}\)
Increasing
To find the interval where f(x) is increasing, set the differentiated function to more than zero:
\(\implies f'(x) > 0\)
\(\implies 6\left(x+\dfrac{1}{2}\right)^2-\dfrac{39}{2} > 0\)
\(\implies 6\left(x+\dfrac{1}{2}\right)^2 > \dfrac{39}{2}\)
\(\implies \left(x+\dfrac{1}{2}\right)^2 > \dfrac{39}{12}\)
\(\implies \left(x+\dfrac{1}{2}\right)^2 > \dfrac{13}{4}\)
\(\textsf{For\;\;$u^n > a$,\;\;if\;$n$\;is\;even\;then\;\;$u < -\sqrt[n]{a}$\;\;or\;\;$u > \sqrt[n]{a}$}.\)
Therefore:
\(x+\dfrac{1}{2} < -\sqrt{\dfrac{13}{4}}\implies x < \dfrac{-1-\sqrt{13}}{2}\)
\(x+\dfrac{1}{2} > \sqrt{\dfrac{13}{4}}\implies x < \dfrac{-1+\sqrt{13}}{2}\)
So the interval on which function f(x) is increasing is:
\(\left(-\infty, \dfrac{-1-\sqrt{13}}{2}\right) \cup \left(\dfrac{-1+\sqrt{13}}{2}, \infty\right)\)
Decreasing
To find the interval where f(x) is decreasing, set the differentiated function to less than zero:
\(\implies f'(x) < 0\)
\(\implies 6\left(x+\dfrac{1}{2}\right)^2-\dfrac{39}{2} < 0\)
\(\implies \left(x+\dfrac{1}{2}\right)^2 < \dfrac{13}{4}\)
\(\textsf{For\;\;$u^n < a$,\;\;if\;$n$\;is\;even\;then\;\;$-\sqrt[n]{a} < u < \sqrt[n]{a}$}.\)
Therefore:
\(x+\dfrac{1}{2} > -\sqrt{\dfrac{13}{4}}\implies x > \dfrac{-1-\sqrt{13}}{2}\)
\(x+\dfrac{1}{2} < \sqrt{\dfrac{13}{4}}\implies x < \dfrac{-1+\sqrt{13}}{2}\)
So the interval on which function f(x) is decreasing is:
\(\left(\dfrac{-1-\sqrt{13}}{2} < x < \dfrac{-1+\sqrt{13}}{2}\right)\)
Part (b)To find x-coordinates of the local minimum and maximum set the differentiated function to zero and solve for x:
\(\implies f'(x)=0\)
\(\implies 6\left(x+\dfrac{1}{2}\right)^2-\dfrac{39}{2} =0\)
\(\implies \left(x+\dfrac{1}{2}\right)^2 =\dfrac{13}{4}\)
\(\implies x=\dfrac{-1-\sqrt{13}}{2},\;\;\dfrac{-1+\sqrt{13}}{2}\)
To find the y-coordinates of the turning points, substitute the found values of x into the function and solve for y:
\(\implies f\left(\dfrac{-1-\sqrt{13}}{2}\right)=\dfrac{19+13\sqrt{13}}{2}\)
\(\implies f\left(\dfrac{-1+\sqrt{13}}{2}\right)=\dfrac{19-13\sqrt{13}}{2}\)
Therefore:
\(\textsf{Minimum}: \quad \left(\dfrac{-1-\sqrt{13}}{2},\dfrac{19+13\sqrt{13}}{2}\right) \approx (-2.30, 32.94)\)
\(\textsf{Maximum}: \quad \left(\dfrac{-1+\sqrt{13}}{2},\dfrac{19-13\sqrt{13}}{2}\right) \approx (1.30, -13.94)\)
Part (c)At a point of inflection, f''(x) = 0.
To find the point of inflection, differentiate the function again:
\(\implies f''(x)=12x+6\)
Set the second derivative to zero and solve for x:
\(\implies f''(x)=0\)
\(\implies 12x+6=0\)
\(\implies x=-\dfrac{1}{2}\)
Substitute the found value of x into the original function to the find the y-coordinate of the point of inflection:
\(\implies f\left(-\dfrac{1}{2}\right)=\dfrac{19}{2}\)
Therefore, the inflection point is:
\(\left(-\dfrac{1}{2}, \dfrac{19}{2}\right)\)
A curve y = f(x) is concave up if f''(x) > 0 for all values of x.
A curve y = f(x) is concave down if f''(x) < 0 for all values of x.
Concave up
\(\implies f''(x) > 0\)
\(\implies 12x+6 > 0\)
\(\implies x > -\dfrac{1}{2}\)
\(\implies \left(-\dfrac{1}{2}, \infty\right)\)
Concave down
\(\implies f''(x) < 0\)
\(\implies 12x+6 > 0\)
\(\implies x < -\dfrac{1}{2}\)
\(\implies \left(- \infty,-\dfrac{1}{2}\right)\)



Simplify 2x^2-7x-4/x^2

Answers
Answer:
Option 1
Step-by-step explanation:
\(\frac{2x^2 - 7x-4}{x^2 - 5x+4}=\frac{(x-4)(2x+1)}{(x-4)(x-1)}=\frac{2x+1}{x-1}\)
find the intersection (2,2) (2,4) (8,4) (8,2)
Answers
The intersection (2,2) (2,4) (8,4) (8,2) is (5,3).
On the coordinate plane, the four points that are given together form a rectangle. We must first locate the midway of both diagonals in order to determine where the diagonals will connect.
The midpoint of the diagonal that passes through the points (2,2) and (8,4) is ((2+8)/2, (2+4)/2). (5,3).
The midpoint of the diagonal that passes through the points (2,4) and (8,2) is ((2+8)/2, (4+2)/2). (5,3).
Consequently, the diagonals' point of intersection is (5,3).
Due to the fact that a rectangle's two diagonals meet here in the middle, this location also serves as the rectangle's centre.
For such more question on intersection:
https://brainly.com/question/30915785
#SPJ11
Two metal spheres of diameter 2.3cm and 3.86cm are melted. The molten material is used to cast equal cylindrical slabs of radius 8mm and length 70mm. If 1/2 of the metal is lost during casting. Calculate the number of complete slabs casted.
Answers
Answer:
4
Step-by-step explanation:
If 1/20 of the metal is lost during casting. Calculate the number of complete slabs casted. (4mks)
sat question I can’t seem to understand fully.

Answers
The value of m + n is given as follows:
A. 18.
How to obtain the value of m + n?The exponential expression for this problem is defined as follows:
\((x^5y^6)^{\frac{1}{5}}(x^3y^4}^{\frac{1}{4}}\)
Applying the power of power rule, we have that the exponents are given as follows:
x: 5/5 + 3/4 = 1 + 3/4 = 7/4.y: 6/5 + 4/4 = 6/5 + 1 = 11/5.Then the values of m and n are given as follows:
m = 7, n = 11.
Thus the sum is given as follows:
m + n = 7 + 11 = 18.
More can be learned about exponent rules at https://brainly.com/question/11975096
#SPJ1
What is the rate of change for the interval between 0 and
2 for the quadratic equation as f(x) = 2x² + x - 3
represented in the table?
O
23/1/2
04
05
O 10
Answers
\(\begin{array}{llll} f(x)~from\\\\ x_1 ~~ to ~~ x_2 \end{array}~\hfill slope = m \implies \cfrac{ \stackrel{rise}{f(x_2) - f(x_1)}}{ \underset{run}{x_2 - x_1}}\impliedby \begin{array}{llll} average~rate\\ of~change \end{array} \\\\[-0.35em] ~\dotfill\\\\ f(x)= 2x^2 + x -3 \qquad \begin{cases} x_1=0\\ x_2=2 \end{cases}\implies \cfrac{f(2)-f(0)}{2 - 0} \\\\\\ \cfrac{[2(2)^2 + (2) -3]~~ - ~~[2(0)^2 +(0) -3]}{2}\implies \cfrac{7-(-3)}{2}\implies \cfrac{7+3}{2}\implies \text{\LARGE 5}\)
The rate of change for the interval between 0 and 2 for the quadratic equation will be 5. Then the correct option is C.
What is the average rate change?It is the average amount by which the function varied per unit throughout that time period. It is calculated using the gradient of the line linking the interval's ends on the graph depicting the function.
Average rate = [f(x₂) - f(x₁)] / [x₂ - x₁]
The function is given below.
f(x) = 2x² + x - 3
The rate of change of the function for the interval between 0 to 2 is calculated as,
Average rate = [f(2) - f(0)] / [2 - 0]
Average rate = [(2(2)² + 2 - 3) - (2(0)² + 0 - 3)] / 2
Average rate = 10 / 2
Average rate = 5
The rate of change for the interval between 0 and 2 for the quadratic equation will be 5. Then the correct option is C.
More about the average rate change link is given below.
https://brainly.com/question/28744270
#SPJ5
^look at picture *answer quick please!*

Answers
Answer:2
Step-by-step explanation:
What is the quotient of 3÷1/6 multiply 3 by
Answers
Answer:
18
Step-by-step explanation:
Change 3 into a fraction.
Which gives you 3/1
multiply 3/1 by the inverse of 1/6
3/1x1/6
Then you get 1 :)
A scientist needs 60 milliliters of buffer solution for each of 13 experiments. He has a bottle that
contains 971 milliliters of buffer solution. Is there enough buffer solution in the bottle for all 13
experiments? Explain
Answers
Answer:
Yes
Step-by-step explanation:
60 × 13 = 780 milliliters
due to 971 > 780
therefore there is enough buffer solution in the bottle for all 13 experiments
Penelope goes out to lunch. The bill, before tax and tip, was $16.05. A sales tax of 3% was added on. Penelope tipped 18% on the amount after the sales tax was added. How much tip did she leave? Round to the nearest cent.
Answers
Answer:
2.98
Step-by-step explanation:
First find 3% of 16.05 which is 0.48.
Then add 0.48 to 16.05 which is 16.53.
Then multiply .18 by 16.53 which gets you 2.98
Hope it helps
Answer:
To find the amount of the sales tax, we need to multiply the bill amount by the tax rate of 3% or 0.03:
Sales tax = 0.03 x $16.05 = $0.48
To find the total amount of the bill after the sales tax was added, we need to add the bill amount to the sales tax:
Total bill = $16.05 + $0.48 = $16.53
To find the amount of the tip, we need to calculate 18% of the total bill after the sales tax was added:
Tip = 0.18 x $16.53 = $2.98
Rounding to the nearest cent, Penelope left a tip of $2.98.
Step-by-step explanation:
Big Orange Trucking is designing an information system for use in “in-cab” communications. It must summarize data from eight sites throughout a region to describe typical conditions. Compute an appropriate measure of central location for the variables wind direction, temperature, and pavement. (Note: Trace indicates slightly wet.)
Answers
Complete question
The complete question is shown on the first uploaded image
Answer:
Wind direction [Mode] is South west
The [Mean] temperature is \(T = 91\)
The [Bimodal] pavement is Dry Wet
Step-by-step explanation:
From the question the first question is to determine the wind direction
Generally from the wind direction would be the data with the highest frequency on the wind column i.e the mode of the data, this because this variable(wind direction ) is a nominal level type of level of measurement where the value assigned to the variable are for classification of the data (i.e telling what the data is all about )
So
The mode is South west
This means that the wind direction is South west
From the question the second question is to determine the temperature
Generally given that their are are no outlier that will skew the data we can obtain the temperature by calculating the mean for the temperature column
This is mathematically represented as
\(T = \frac{89 + 86 + \cdots + 93 + 93}{8}\)
\(T = 91\)
From the question the first question is to determine the pavement
Generally from the pavement would be the data with the highest frequency on the pavement i.e the mode of the data, this because this variable(pavement ) is a nominal level type of level of measurement where the value assigned to the variable are for classification of the data (i.e telling what the data is all about )
From the table we see that the pavement that the pavement column is bimodal
So the mode is Dry Wet
This mean that the pavement is Dry Wet

A truck can be rented from Company A for $110 a day plus $0.50 per mile. Company B charges $80 a day plus $0.75 per mile to rent the same truck. Part A: Write an equation to find the number of miles in a day at which the rental costs for Company A and Company B are the same. Part B: Solve the equation.
Answers
m=number of miles
A=$110+$0.50m
B=$80+$0.80m
$110+$0.50m=$80+$0.80m Subtract ($80+$0.50m)
$30=$0.30m Divide each side by $0.30
100=m ANSWER: More than 100 miles must be driven per day to make Company A a better deal.
35.99 mixed number simplest form
Answers
Answer:
33 \(\frac{99}{100}\)
Step-by-step explanation:
hope this helps :)
Answer:99/100
Step-by-step explanation:
i need help can someone help me right now!!!!!!

Answers
(a) | BD | bisects | AC | (reason : Given)
(b) |AD| ≅ |CD| (reason: |BD| is the perpendicular bisector of segment AC).
(c) ∠ABD ≅ ∠CBD (reason: | BD | bisects angle ABC)
(d) ∠A ≅ ∠ C (reason: complementary angles of a right triangle)
What is the complete proof of the congruent angles?Congruent angles are the angles that have equal measure. So all the angles that have equal measure will be called congruent angles.
From the first statement, we will complete the flow chart as follows;
line BD bisects line AC (reason : Given)
line AD is congruent to line CD (reason: line BD is the perpendicular bisector of segment AC)
Angle ABD is congruent to angle CBD (reason: line BD bisects angle ABC)
Angle A is congruent to angle C (reason: angle ABD = angle CBD, and both triangles ABD and CBD are right triangles).
Learn more about congruent angles here: https://brainly.com/question/28262429
#SPJ1
A fitness company conducted an experiment on multiple volunteers for their 15-day "beach body" weight loss program. Their before and after weights were taken and the results are listed below.Before After247.7 232.9177.8 169.7152.7 151.6115.9 119.1242.5 241121.7 126.2241.3 234.5212.8 217.7182.5 176.7231.3 222.8225.9 217.2155.3 154.4187.9 177.5149.4 139.3187.7 194.1176.4 174.3197.7 191.8Required:a. What is the average before weight of the group?b. What is the average after weight of the group?c. Using an appropriate confidence interval, determine if the program was effective over the 15-days in helping people lose weight.
Answers
Answer:
a. Mb = 188.6
b. Ma = 184.8
c. The upper-bound 95% confidence interval for the mean is (-∞, -1.3).
As the upper bound of the confidence interval is negative, we are 95% confident that the true mean difference is negative and the treatment is effective.
Step-by-step explanation:
The matched-pair sample data we have is:
Before After Difference
247.7 232.9 -14.8
177.8 169.7 -8.1
152.7 151.6 -1.1
115.9 119.1 3.2
242.5 241 -1.5
121.7 126.2 4.5
241.3 234.5 -6.8
212.8 217.7 4.9
182.5 176.7 -5.8
231.3 222.8 -8.5
225.9 217.2 -8.7
155.3 154.4 -0.9
187.9 177.5 -10.4
149.4 139.3 -10.1
187.7 194.1 6.4
176.4 174.3 -2.1
197.7 191.8 -5.9
We can calculate the mean and standard deviation of the before weight as:
\(M_b=\dfrac{1}{n}\sum_{i=1}^n\,x_i\\\\\\M_b=\dfrac{1}{17}(247.7+177.8+152.7+. . .+197.7)\\\\\\M_b=\dfrac{3206.5}{17}\\\\\\M_b=188.6\\\\\\s_b=\sqrt{\dfrac{1}{n-1}\sum_{i=1}^n\,(x_i-M_b)^2}\\\\\\s_b=\sqrt{\dfrac{1}{16}((247.7-188.6)^2+(177.8-188.6)^2+(152.7-188.6)^2+. . . +(197.7-188.6)^2)}\\\\\\s_b=\sqrt{\dfrac{27057.6}{16}}\\\\\\s_b=\sqrt{1691.1}=41.1\\\\\\\)
We can calculate the mean and standard deviation of the after weight as:
\(M_a=\dfrac{1}{n}\sum_{i=1}^n\,x_i\\\\\\M_a=\dfrac{1}{17}(232.9+169.7+151.6+. . .+191.8)\\\\\\M_a=\dfrac{3140.8}{17}\\\\\\M_a=184.8\\\\\\s_a=\sqrt{\dfrac{1}{n-1}\sum_{i=1}^n\,(x_i-M_a)^2}\\\\\\s_a=\sqrt{\dfrac{1}{16}((232.9-184.8)^2+(169.7-184.8)^2+(151.6-184.8)^2+. . . +(191.8-184.8)^2)}\\\\\\s_a=\sqrt{\dfrac{23958}{16}}\\\\\\s_a=\sqrt{1497.4}=38.7\\\\\\\)
As this is a matched-paired data, we can test the effectiveness of the program using the sample data for the difference of each pair.
First, we calculate the mean and standard deviation of the pair differences:
\(M_d=\dfrac{1}{n}\sum_{i=1}^n\,x_i\\\\\\M_d=\dfrac{1}{17}((-14.8)+(-8.1)+(-1.1)+. . .+(-5.9))\\\\\\M_d=\dfrac{-65.7}{17}\\\\\\M_d=-3.9\\\\\\s_d=\sqrt{\dfrac{1}{n-1}\sum_{i=1}^n\,(x_i-M_d)^2}\\\\\\s_d=\sqrt{\dfrac{1}{16}((-14.8-(-3.9))^2+(-8.1-(-3.9))^2+(-1.1-(-3.9))^2+. . . +(-5.9-(-3.9))^2)}\\\\\\s_d=\sqrt{\dfrac{607.7}{16}}\\\\\\s_d=\sqrt{38}=6.2\\\\\\\)
We have to calculate a 95% confidence interval for the paired difference. We are only interested in the upper bound, so the lower bound is not calculated.
If the upper bound is negative, that means that we are 95% confident that the mean difference is negative or significantly smaller than 0, which means that the treatment is effective.
The population standard deviation is not known, so we have to estimate it from the sample standard deviation and use a t-students distribution to calculate the critical value.
The sample mean is M=-3.9.
The sample size is N=17.
When σ is not known, s divided by the square root of N is used as an estimate of σM:
\(s_M=\dfrac{s}{\sqrt{N}}=\dfrac{6.2}{\sqrt{17}}=\dfrac{6.2}{4.123}=1.504\)
The degrees of freedom for this sample size are:
\(df=n-1=17-1=16\)
The t-value for an upper-bound 95% confidence interval and 16 degrees of freedom is t=1.746.
The margin of error (MOE) can be calculated as:
\(MOE=t\cdot s_M=1.746 \cdot 1.504=2.63\)
Then, the lower and upper bounds of the confidence interval are:
\(UL=M+t \cdot s_M = -3.9+2.63=-1.3\)
The upper-bound 95% confidence interval for the mean is (-∞, -1.3).
As the upper bound of the confidence interval is negative, we are 95% confident that the true mean difference is negative and the treatment is effective.
Correct answer please

Answers
Answer:
50.75
Step-by-step explanation:
We have:
\(E[g(x)] = \int\limits^{\infty}_{-\infty} {g(x)f(x)} \, dx \\\\= \int\limits^{1}_{-\infty} {g(x)(0)} \, dx+\int\limits^{6}_{1} {g(x)\frac{2}{x} } \, dx+\int\limits^{\infty}_{6} {g(x)(0)} \, dx\\\\= \int\limits^{6}_{1} {g(x)\frac{2}{x} } \, dx\\\\=\int\limits^{6}_{1} {(4x+3)\frac{2}{x} } \, dx\\\\=\int\limits^{6}_{1} {(4x)\frac{2}{x} } \, dx + \int\limits^{6}_{1} {(3)\frac{2}{x} } \, dx\\\\=\int\limits^{6}_{1} {8} \, dx + \int\limits^{6}_{1} {\frac{6}{x} } \, dx\\\\\)
\(=8\int\limits^{6}_{1} \, dx + 6\int\limits^{6}_{1} {\frac{1}{x} } \, dx\\\\= 8[x]^{^6}_{_1} + 6 [ln(x)]^{^6}_{_1}\\\\= 8[6-1] + 6[ln(6) - ln(1)]\\\\= 8(5) + 6(ln(6))\\\\= 40 + 10.75\\\\= 50.74\)
The graph below could be the graph of which exponential function?
||||
5
5
Answers
The graph could be the graph of exponential function of option b)
\(F(x) = 2 • (0.5)^{x} \)
The general form of an exponential function is
\(f(x) = {ab}^{x} \)
Here, a is the function's starting value and b is its growth factor.
F(x) is an increasing function if b > 1, and if b<1, then f(x) is a decreasing function
The function's initial value is 2 as can be seen from the provided graph. Therefore, a has a value of 2.
Given that the graph indicates that the function is decreasing, b must be less than 1.
It indicates that the necessary function is in the form of
\(f(x) = 2(b)^{x} \)
Where it is b<1
By checking option B, b=0.5<1. Hence, option B is the correct answer
To learn more about exponential function: https://brainly.com/question/30241796
#SPJ1
Note that the full question is:
(check the attached image)

coach Emanuel is trying to lose weight. the stars 2017 weighing 296 pounds. if he plans to burn enough calories to lose 3 pounds per week, then how many weeks will pass before Couch Emanuel is at most 251 pounds
Thank you to whoever answers this :)
Answers
Answer:
13 weeks because he loses 3 pounds per week
Step-by-step explanation:
269-251=39
39 divided by 3 = 13
13 weeks is your answer!
please mark brainliest please!!!
Positive numbers have negative square roots.
A. True
O B. False
Answers
Answer:
A. True
Step-by-step explanation:
Which triangle is △ABC similar to and why?
Answers
Answer:
can we get a picture
Step-by-step explanation:
