Make t the subject of this formula.
r = 7 (t + 3)
Answers
Answer:
t= r-21
7
Step-by-step explanation:
r = 7(t+3)
expanding the bracket
r = 7t + 21
bringing t to the left hand side
7t = r - 21
divide both sides by seven
t= r-21
7
Answer:
t = (r/7) - 3
Step-by-step explanation:
Given equation,
→ r = 7(t + 3)
Now the value of t will be,
→ r = 7(t + 3)
→ r = 7t + 21
→ r - 21 = 7t
→ 7t = r - 21
→ t = (r - 21)/7
→ t = (r/7) - 3
Hence, value of t is (r/7) - 3.
Related Questions
hey can someone pls help me asap?!

Answers
Hope this helps
Find the circumference of the object. Use 3.14 or 22 7 for ml. Diameter:9

Answers
Answer:
c = 28.26 cm
Step-by-step explanation:
equation for circumference of a circle is 2πr
r = d/2 so here r = 9/2 = 4.5
2 x 3.14 x 4.5 = 28.26
Answer:
C ≈ 28.26 cm
Step-by-step explanation:
The circumference (C) is calculated as
C = πd ( d is the diameter ) , then
C = π × 9 ≈ 3.14 × 9 = 28.26 cm ( to the nearest hundredth )
which parent functions do not have a domain of all real numbers?
Answers
Using domain concepts, it is found that the two most known functions that do not have a domain of all real numbers are fractions and even roots.
---------------------
The domain of a function is composed by all possible values that the input can assume.Functions such as linear functions and quadratic functions have no restrictions, thus, their domain is all real numbers.---------------------
In fractions, there are is a restriction, that the denominator cannot be zero, thus, values for the input which make the denominator zero are outside the domain, and the domain is not all real numbers.In even roots, there is a restriction, as the even roots of negative numbers do not exist in the real domain, and thus, values for the input which makes the number inside the root negative are outside the domain, and the domain is not all real numbers.Thus, the most known functions that do not have a domain of all real numbers are fractions and even roots.A similar problem is given at https://brainly.com/question/13136492
ASAP Please i need this done in the next 20 minutes
cos^2B-sin^2B=3cosB+1
Answers
the answer is "Using the trigonometric identity
sin²x + cos²x = 1 , then sin²x = 1 - cos²x
Consider the left side
sin²a - sin²b
= (1 - cos²a) - (1 - cos²b)
= 1 - cos²a - 1 + cos²b
= cos²b - cos²a
= right side , thus verified"
Due to bad weather, a fruit seller had to sell his fruits at a loss of 8%. If he bought t
Rs 225 a dozen, find the SP of 40
Answers
The SP of 40 fruits is Rs 690.
Given,
Due to bad weather, a fruit seller had to sell his fruits at a loss of 8%.
He bought the fruits for Rs 225 a dozen.
We have to find the SP of 40.
We have,
A dozen of fruits = Rs 225
12 fruits = Rs 225
Divide both sides by 12
1 fruit = Rs 225 / 12
1 fruit = Rs 18.75
He sold each fruit at a loss of 8%.
8% of Rs 18.75
= 8/100 x 18.75
= Rs 1.5
So,
The selling price of 1 fruit:
= 18.75 - 1.5
= Rs 17.25
The selling price of 40 fruits:
40 x Rs 17.25
= 690
Thus the SP of 40 fruits is Rs 690.
Learn more about the Selling price here:
https://brainly.com/question/28017453
#SPJ1
Find the values of x,y, and z and the perimeter

Answers
What is the area, measured in square meters, of the parallelogram shown?
Round to the nearest tenth, if necessary.
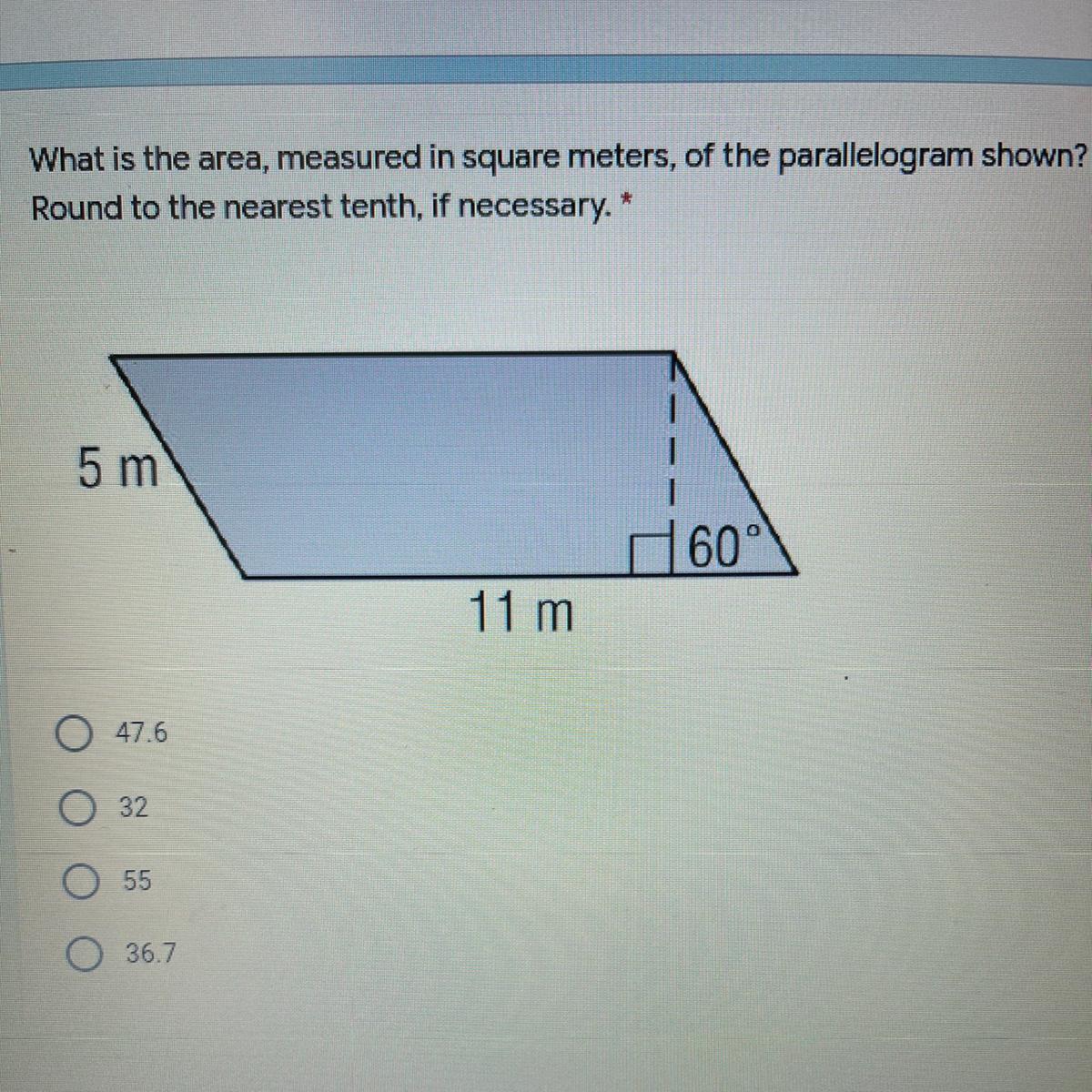
Answers
Answer:
A = (11 m)(4.33 m) = 47.6 m^2
Step-by-step explanation:
The width of this parallelogram is (5 m)(sin 60 degrees), or approximately 4.33. This is the length of the dashed line shown.
The area of this parallelogram is A = (length)(width), which here is:
A = (11 m)(4.33 m) = 47.6 m^2
Answer:
55
Step-by-step explanation:
i think
\(Given \: cotA = \sqrt{\dfrac{1}{3}}\)
Find all other trigonometric ratios.
Answers
\( \tt cotA = \sqrt{ \dfrac{1}{3}}\)
\( \tt \implies cotA = \dfrac{1}{\sqrt{3}}\)
To Find :All other trigonometric ratios, which are :
sinAcosAtanAcosecAsecASolution :Let's make a diagram of right angled triangle ABC.
Now, From point A,
AC = Hypotenuse
BC = Perpendicular
AB = Base
\( \tt We \: are \: given, \: cotA = \dfrac{1}{\sqrt{3}}\)
\( \tt We \: know \: that \: cot \theta = \dfrac{base}{perpendicular}\)
\( \tt \implies \dfrac{base}{perpendicular} = \dfrac{1}{\sqrt{3}}\)
\( \tt \implies \dfrac{AB}{BC} = \dfrac{1}{\sqrt{3}}\)
\( \tt \implies AB = 1x \: ; \: BC = \sqrt{3}x \: (x \: is \: positive)\)
Now, by Pythagoras' theorem, we have
AC² = AB² + BC²
\( \tt \implies AC^{2} = (1x)^{2} + (\sqrt{3}x)^{2}\)
\( \tt \implies AC^{2} = 1x^{2} + 3x^{2}\)
\( \tt \implies AC^{2} = 4x^{2}\)
\( \tt \implies AC = \sqrt{4x^{2}}\)
\( \tt \implies AC = 2x\)
Now,
\( \tt sin \theta = \dfrac{perpendicular}{hypotenuse}\)
\( \tt \implies sinA = \dfrac{BC}{AC} \)
\( \tt \implies sinA = \dfrac{\sqrt{3}x}{2x} \)
\( \tt \implies sinA = \dfrac{\sqrt{3}}{2} \)
\( \Large \boxed{\tt sinA = \dfrac{\sqrt{3}}{2}} \)
\( \tt cos \theta = \dfrac{base}{hypotenuse}\)
\( \tt \implies cosA = \dfrac{AB}{AC} \)
\( \tt \implies cosA = \dfrac{1x}{2x} \)
\( \tt \implies cosA = \dfrac{1}{2} \)
\( \Large \boxed{\tt cosA = \dfrac{1}{2}} \)
\( \tt tan \theta = \dfrac{perpendicular}{base}\)
\( \tt \implies tanA = \dfrac{BC}{AB} \)
\( \tt \implies tanA = \dfrac{\sqrt{3}x}{1x} \)
\( \tt \implies tanA = \sqrt{3}\)
\( \Large \boxed{\tt tanA = \sqrt{3}}\)
\( \tt cosec \theta = \dfrac{hypotenuse}{perpendicular}\)
\( \tt \implies cosecA = \dfrac{AC}{BC} \)
\( \tt \implies cosecA = \dfrac{2x}{\sqrt{3}x} \)
\( \tt \implies cosecA = \dfrac{2}{\sqrt{3}}\)
\( \Large \boxed{\tt cosecA = \dfrac{2}{\sqrt{3}}}\)
\( \tt sec \theta = \dfrac{hypotenuse}{base}\)
\( \tt \implies secA = \dfrac{AC}{AB} \)
\( \tt \implies secA = \dfrac{2x}{1x} \)
\( \tt \implies secA = 2\)
\( \Large \boxed{\tt secA = 2}\)
![[tex]Given \: cotA = \sqrt{\dfrac{1}{3}}[/tex]Find all other trigonometric ratios.](https://i5t5.c14.e2-1.dev/h-images-qa/answers/attachments/U9ntM9I1LfDar2Huoc5U94ZStCOXUryP.jpeg)
\(\setlength{\unitlength}{2mm}\begin{picture}(0,0)\thicklines\put(0,0){\line(3,0){2.5cm}}\put(0,0){\line(0,3){2.5cm}}\qbezier(12.4,0)(6.6,5)(0,12.4)\put(-2,13){\sf A}\put(13,-2){\sf C}\put(-2,-2){\sf B}\put(-3,6){\sf 1}\put(6,-3){\sf \sqrt3$}\put(7,7){\sf 2}\end{picture}\)
Solution :-Given ,
cotA = \(\sf \sqrt{\dfrac{1}{3}}=\dfrac{1}{\sqrt3}\)We need to find ,
All the trigonometric identitiesFirst finding the other side of the triangle using Pythagoras theorem .
Hypotenuse² = Base² + Height²
\(\to\sf Hypotenuse^2 = (1)^2 + (\sqrt3)^2\)
\(\to\sf Hypotenuse^2 = 1 + 3 \)
\(\to \sf Hypotenuse = \sqrt4\)
\(\to\bf Hypotenuse = 2\)
Now ,
\(\rm sinA = \dfrac{opposite}{hypotenuse}=\sf\dfrac{\sqrt3}{2}\)\(\rm cosA = \dfrac{adjacent}{hypotenuse}=\sf\dfrac{1}{2}\)\(\rm tanA = \dfrac{opposite}{adjacent}=\sf\dfrac{\sqrt3}{1}\)\(\rm cosecA=\dfrac{hypotenuse}{adjacent}=\sf\dfrac{2}{\sqrt3}\)\(\rm secA = \dfrac{Hypotenuse}{adjacent}=\sf\dfrac{2}{1}\)\(\rm cotA = Already\; given =\sf \dfrac{1}{\sqrt3}\)Find the slope show your work you don't have to do them all I just need some of them done please

Answers
Answer: 1. Slope equals 3/4 or 0.75, 2. Slope equals -5/2 or -2.5
Step-by-step explanation:
1. Subtract y coordinates 4 and -2 to get 6. Then subtract x coordinates 4 and -4 to get 8, so that is equal to 6/8. Then simplify to get 3/4 or 0.75
2. Subtract y coordinates 10 and -5 to get -15. Then subtract x coordinates 0 and -6 to get 6, so that is equal to-15/6. Then simplify to get -5/2 or -2.5.
Combine the like terms to create an equivalent expression:
−4p+(−6p)
Answers
THE ANSWER IS -10P.
Step-by-step explanation:
USING BY MULTIPLICATION RULE.
minus × plus = minus
then minus × minus = plus.
so we add the 4p+6p= -10p.
why -10p is come it means THE BIGGER NUMBER SIGN IS MINUS. So it's comes -10p.
HOPE IT HELP......
\(\huge\text{Hey there!}\)
\(\mathsf{-4p + (-6p)}\\\\\mathsf{= -4p - 6p}\\\\\large\text{COMBINE the LIKE TERMS:}\\\\\mathsf{= (-4p - 6p)}\\\\\mathsf{= -4p - 6p}\\\\\mathsf{-10p}\\\\\\\huge\textbf{Therefore, your answer should be:}\\\huge\boxed{\mathsf{-10p}}\huge\checkmark\)
\(\huge\text{Good luck on your assignment \& enjoy your day!}\)
~\(\frak{Amphitrite1040:)}\)
Write an expression using fractions to determine the amount that each person will pay then calculate each person's contributions showing all steps in long division
Answers
Answer:
Fractional Expression for how much each person pays = (64.04/4)
Each person pays $16.01.
Step-by-step explanation:
Complete Question
Write an expression using fractions to show how to determine the amount that each person will pay. Then calculate each person's contributions showing all steps in long division.
The total of the bill is $64.04 and there are 4 people splitting the bill.
Solution
Total amount to be shared equally by 4 persons = $64.04
Fractional Expression for how much each person pays = (64.04/4)
Long division method to do this division.
16.01
4 | 64.04
4
24
24
00
0
04
4
0
Each person pays $16.01.
Hope this Helps!!!
use the method of cylindrical shells to find the volume of the solid obtained by rotating the region bounded by f(x)=5cos(πx), the x-axis, the y-axis, and the line x=12 around the y-axis.
Answers
the volume of the solid obtained by rotating the region bounded by f(x)=5cos(πx), the x-axis, the y-axis, and the line x=12 around the y-axis. we can use the method of cylindrical shells is \(V = ∫[0,5] ∫[0,(1/π)cos^(-1)(y/5)] 2πx dx dy\).
To find the volume of the solid obtained by rotating the region bounded by the function f(x) = 5cos(πx), the x-axis, the y-axis, and the line x = 12 around the y-axis, we can use the method of cylindrical shells.
The region bounded by the given curves and lines is a curve that oscillates between the x-axis and a maximum height of 5. We want to rotate this region around the y-axis to form a solid.
Consider an infinitesimally thin strip or shell with height Δy and width Δx. The radius of this shell is x, and its volume can be approximated as the product of its height, width, and circumference:
ΔV ≈ 2πxΔxΔy.
To find the volume of the entire solid, we need to sum up the volumes of all these cylindrical shells. We integrate this expression with respect to y over the range of y-values for which the region exists.
The region exists for 0 ≤ y ≤ 5, and at any given height y, x varies between 0 and a corresponding x-value on the curve f(x). Since f(x) = 5cos(πx), we have:
\(x = (1/π)cos^(-1)(y/5).\)
Integrating ΔV = 2πxΔxΔy over this range and simplifying, we get:
\(V = ∫[0,5] ∫[0,(1/π)cos^(-1)(y/5)] 2πx dx dy.\)
Evaluating this double integral will give us the volume of the solid. The integral can be solved using standard integration techniques or numerical methods.
Note: The explanation provided is a general outline of the method of cylindrical shells and how it applies to this specific problem. Actual calculation and evaluation of the integral require detailed steps and calculations beyond the scope of this response.
To learn more about volume click here:
https://brainly.com/question/27710307#
#SPJ11
What are the coordinates of P?

Answers
The function h(x)=12x^8+49 is an even function. Which transformation of h(x) would result in a function that is neither even nor odd?
A) reflection over the x-axis
B) vertical stretch by a factor of 7
C) translation 8 units to the right
D) horizontal compression
Explanation of the correct answer would be nice so that I understand it better.
Answers
Answer:
The correct option is;
C) Translation 8 units to the right
Step-by-step explanation:
The function h(x) = 12·x⁸ + 49 is an even function
However a translation 8 units to the right would yield the following function;
h(x) = 12 × (x+ 8)⁸ + 49 = 12x⁸ + 768x⁷ + 21504x⁶ + 344064x⁵ + 3440640x⁴ + 22020096x³ + 88080384x² + 201326592x + 201326641
For h(1) = 516560701
Also h(-x) = 12 × (-x+ 8)⁸ + 49 = 12x⁸ - 768x⁷ + 21504x⁶ - 344064x⁵ + 3440640x⁴ - 22020096x³ + 88080384x² - 201326592x + 201326641
For h(-1) = 69177661
Therefore h(x) ≠ h(-x) after the translation 8 units to the right and the function is neither even nor odd.
Determine the value of y, if x is 9
y=\(x^{2}\)-8
Answers
Answer:
Step-by-step explanation:
y = x² - 8
put x= 9 you have y = (9)² - 8 =81-8 = 73
the manager of a shopping mall wishes to expand the number of shops available in the food court. she has a market research survey the first 110
Answers
The best way to remedy Ask customers throughout the day on both weekdays and weekends.
The cause of the bias in the survey is likely sampling bias. This means that the sample of 110 customers may not be representative of the entire population of food court shoppers. The bias can arise if the sample is not selected randomly or if certain groups of shoppers are overrepresented or underrepresented in the sample.
To remedy the sampling bias, the best way would be to Ask customers throughout the day on both weekdays and weekends.
By surveying customers at different times, including both weekdays and weekends, a more diverse and representative sample can be obtained. This will help to capture the preferences of a wider range of food court shoppers and reduce the bias introduced by sampling only weekday mornings.
Learn more about Sampling here:
https://brainly.com/question/14611759
#SPJ4
The manager of a shopping mall wishes to expand the number of shops available in the food court. He has a market researcher survey the first 110 customers who come into the food court during weekday mamings in distin what types of food the shoppers would like to see added to the food court,
Which of the following is the best way to remedy this problem?
OA cisne the sample size so that more people respond to the question OB Ask customers throughout the day on both weekdays and hands
OC Reward the question so that it is balanced
One half the sum of two numbers equals their difference. What are the numbers if their sum is 56
Answers
Answer:
28
Step-by-step explanation:
Answer:
28
Step-by-step explanation:
(1/2)56 = a - b
28 = a - b
a + 28 = 56
a = 28
Malik created a model of a rectangular flag. His model is 1010 inches long and the actual flag is 2525 inches long. How many times wider is the actual flag than malik's model?.
Answers
The actual flag is 2.5 times wider than Malik's rectangular model, meaning that it is 250% wider than the model he created.
Step 1: Calculate the width of Malik's model: 1010 inches
Step 2: Calculate the width of the actual flag: 2525 inches
Step 3: Divide the width of the actual flag by the width of Malik's model: 2525 / 1010 = 2.5
Malik created a model of a rectangular flag that was 1010 inches long. However, the actual flag was 2525 inches long. To figure out how many times wider the actual flag was than Malik's model, we needed to calculate the ratio of the widths. To do this, we divided the width of the actual flag (2525 inches) by the width of Malik's model (1010 inches). This gave us a ratio of 2.5, which means that the actual flag was 2.5 times wider than Malik's model. This can also be expressed as 250%, since 2.5 is the same as 250%. This means that the actual flag is 250% wider than the model created by Malik.
Learn more about rectangle here
https://brainly.com/question/29068457
#SPJ4
Can somebody help me please

Answers
Answer:
I will help you I. working on it just be pecient
Kiaya has to have at least $100 in her checking account to avoid a fee from the bank. She has $376 in her account now. Each week, she makes a $25 withdrawal. For how many weeks can she make this withdrawal and avoid a fee?
Answers
Answer:
$500 a week
Step-by-step explanation:
Hope I can help.
Kiaya can make a $25 withdrawal for 11 weeks without incurring a fee.
What is the division operation?In mathematics, divides left-hand operands into right-hand operands in the division operation.
Kiaya needs to have at least $100 in her checking account to avoid a fee from the bank. She has $376 in her account now, so she can make $376 - $100 = $276 worth of withdrawals before she will be charged a fee.
Each week, she makes a $25 withdrawal.
To determine the number of weeks she can make this withdrawal before being charged a fee, we divide the total amount she can withdraw ($276) by the amount she withdraws each week ($25).
So, Kiaya can make $276 / $25 = 11 withdrawals before she will be charged a fee.
Therefore, she can make a $25 withdrawal for 11 weeks without incurring a fee.
To learn more about the division operation click here :
brainly.com/question/25870256
#SPJ2
two dice are rolled at the same time and the numbers on the face up are read what is the probability of the numbers facing up and add up to 8
Answers
The probability of rolling two dice and getting a sum of 8 is 5/36.
There are a total of 36 possible outcomes when two dice are rolled at the same time. Each die has 6 possible outcomes (1, 2, 3, 4, 5, or 6). To calculate the probability of getting a sum of 8, we need to count the number of ways that the dice can add up to 8.
There are five possible combinations that add up to 8: (2, 6), (3, 5), (4, 4), (5, 3), and (6, 2). Each of these combinations has a probability of 1/36 (since there is only one way to roll each of these combinations).
Therefore, the probability of rolling two dice and getting a sum of 8 is the sum of the probabilities of each of these five outcomes, which is:
1/36 + 1/36 + 1/36 + 1/36 + 1/36 = 5/36
So the probability of the numbers facing up and adding up to 8 is 5/36.
Learn more about probability: https://brainly.com/question/30034780
#SPJ11
-1/3u-4=1/4u-1/4
Solve and check
Answers
9514 1404 393
Answer:
u = -45/7 = -6 3/7
Step-by-step explanation:
It can work well to eliminate fractions first. Here, we can do that by multiplying by the product of their denominators: 12.
-4u -48 = 3u -3
-45 = 7u . . . . . . . . add 3+4u
-45/7 = u . . . . . . divide by the coefficient of u
__
Check
(-1/3)(-45/7) -4 = (1/4)(-45/7) -1/4
15/7 -4 = -45/28 -7/28
-13/7 = -52/28 . . . true
help with this question

Answers
Answer:
option D is correct
x=O and x=-8
47:PLEASE HELP Find the y-intercept of -x +2y=20
Answers
Answer:
(0,10)
Step-by-step explanation:
-x + 2y = 20
2y = x +20
y = 1/2x + 10
This is slope-intercept form. The y-intercept is 10.
(0,10)
Answer:
(0, 10)
Step-by-step explanation:
Can be simplified to
2y = x+20 by adding x to both sides
then divide by 2 to get to slope intercept form: y = ax+b
b is the y-intercept
y = 1/2x + 10
so the answer is 10
Gabriella bought three melons for 7.50 how many melons can April buy if she has 17.50
Answers
Answer:
7 melons
Step-by-step explanation:
So we first figure out the unit price of each melon by dividing 7.5 with 3 and you get $2.5 per melon. Then you divide 17.5 by 2.5 and then you should get 7.
1 mile long to 800 feet
Answers
Answer:
0.1515
Step-by-step explanation:
1 mile = 5,280 feetTo convert 1 mile to 800 feet, we can set up a proportion:
\( \frac{1 mile}{5,280 feet} = \frac{x \: miles}{800 feet} \)
where x is the number of miles equivalent to 800 feet. To solve for x, we can cross-multiply:
\({5,280 feet}^{x miles} = {800 \: \: feet}^{1 mile}\)
Dividing both sides by 5,280 feet, we get:
\(x miles = \frac{800 feet}{5,280 feet} \\ x miles = 0.1515151515 miles\)
Therefore, 1 mile is equivalent to approximately 0.1515 miles or 800 feet is equivalent to approximately 0.1515 miles.
Complete the square. x² +14 x+ ____
Answers
The complete expression of the given quadratic function is:
x² + 14x + 49
What is an algebraic expression?An algebraic expression is a set of numbers and letters that make up an expression that has a meaning, the letters are variables and the numbers are coefficients or independent terms, algebraic expressions can be part of an equation and model mathematical processes.
To solve this problem we must complete square in the expression, it is necessary to note that perfect square trinomial has the form of:
(a + b)² = a² + 2ab + b²
x² + 14x + ?
where:
x² = a²14x = 2abb = ?Substitute and find b
14x = 2xb
2b = 14
b = 7
Let's complete the square
x² + 14x + 7²
x² + 14x + 49
Learn more about algebraic expression in:
brainly.com/question/21856579
#SPJ4
Pls helppp!!! Multiple choice questions

Answers
Answer:
Step-by-step explanation:
a
what percentage of the data values are greater than or equal to 52
Answers
Using the box-whisker plot approach, it is computed that 50% of the data values are more than 45.
In a box-whisker plot, as seen in the illustration, The minimum, first quartile, median, third quartile, and maximum quartiles are shown by a rectangular box with two lines and a vertical mark. In descriptive statistics, it is employed.
Given the foregoing, the box-whisker plot depicts a specific collection of data. A vertical line next to the number 45 shows that it is the 50th percentile in this instance and that 45 is the median of the data.
It indicates that 50% of the values are higher than 45 and 50% of the values are higher than 45.
Using this technique, we can easily determine the proportion of data for which the value is higher or lower. Data analysis and result interpretation are aided by it. Therefore, 50% of values exceed 45.
Note: The correct question would be as
The box-and-whisker plot below represents some data sets. What percentage of the data values are greater than 45?
0
H
10
20
30 40
50 60
70 80 90 100
For more questions on the box-whisker plot
https://brainly.com/question/1535617
#SPJ8
Un topógrafo usa un instrumento llamado teodolito para medir el ángulo de elevación entre el nivel del piso y la cumbre de una montaña. En un punto, se mide un ángulo de elevación de 41°. Medio kilómetro más lejos de la base de la montaña, el ángulo de elevación medido es de 37°. ¿Qué altura tiene la montaña?
Answers
Answer:
La altura de la montaña es 2848,80 m.
Step-by-step explanation:
Un topógrafo usa un instrumento llamado teodolito para medir el ángulo de elevación entre el nivel del piso y la cumbre de una montaña. En un punto, se mide un ángulo de elevación de 41°.
La función trigonométrica tangente se define como:
\(tan (angulo)=\frac{cateto opuesto}{cateto adyacente}\)
Entonces, siendo h altura de la montaña e y la distancia de la base de montaña a donde se realiza la medición, la distancia de esta primera observación del topógrafo entre el nivel del piso y la cumbre de una montaña es:
\(tan (41)=\frac{h}{x}\) Ecuación (A)
Medio kilómetro más lejos de la base de la montaña, el ángulo de elevación medido es de 37°. Entonces:
\(tan (37)=\frac{h}{x+500m}\) Ecuación (B)
Entonces, despejando h de la ecuación (A):
h= tan(41)* x
Reemplazando la expresión en la ecuación (B):
\(tan (37)=\frac{tan(41)*x}{x+500m}\)
Resolviendo:
tan(37)*(x+500)= tan(41)* x
0,754*( X +500) = 0,869*X
0,754*X + 377 = 0,869*X
377= 0,869*X -0,754*X
377= 0,115*x
377÷0.115= x
x= 3278,26 m
Entonces, reemplazando en la expresión h= tan(41)* x:
h= tan(41)* 3278,26 m
Resolviendo:
h = 0,869 *3278.26 m
h = 2848,80 m
La altura de la montaña es 2848,80 m.
En la imagen adjunta se puede observar una representación gráfica de las medidas utilizadas en el problema.
