If X = 9 units, Y = 7 units, Z = 18 units, and h = 8 units, what is the surface area of the triangular prism shown above?

Answers
Answer: 506 square units (answer choice B)
Step-by-step explanation:
The formula for the surface area of a triangular prism is:
SA = bh + 2ls + lb,
where b is the base, h is the height, l is the length, and s is the side of the triangle.
In this case, the base is 7 (Y), the height is 8 (h), the length is 18 (Z), and the side of the triangle is 9 (X).
So, by subsituition, we have:
SA = (7)(8) + 2(18)(9) + (18)(7)
SA = 56 + 324 + 126
SA = 506
The surface area is 506 square units.
Hope this helps! :)
Related Questions
Numbers between 380 and 410 divisible by 2,5, and 10
Answers
Answer:
Did you try this is a piece of cake
Step-by-step explanation:
Can someone answer this please

Answers
Answer:
Blue
Step-by-step explanation:
A straight line has an angle of 180 on each side.
5a+18+24-a+8a-30=180
12a+12=180
12a=168
a=14
Green: 14x5+18=88
Blue:24-14=10
Yellow:8x14-30=82
Anna volunteers on the weekend at the Central Library. As a school project, she decides to record how many people visit the library, and where they go. On Saturday, 382 people went to The Youth Wing, 461 people went to Social Issues, and 355 went to Fiction and Literature. On Sunday, the library had 800 total visitors. Based on what Anna had recorded on Saturday, about how many people should be expected to go to The Youth Wing? Round your answer to the nearest whole number.
Answers
Based on the data recorded by Anna on Saturday, we can estimate the number of people expected to visit The Youth Wing on Sunday.
Let's calculate the proportion of visitors to The Youth Wing compared to the total number of visitors on Saturday:
\(\displaystyle \text{Proportion} = \frac{\text{Visitors to The Youth Wing on Saturday}}{\text{Total visitors on Saturday}} = \frac{382}{382 + 461 + 355}\)
Next, we'll apply this proportion to the total number of visitors on Sunday to estimate the number of people expected to go to The Youth Wing:
\(\displaystyle \text{Expected visitors to The Youth Wing on Sunday} = \text{Proportion} \times \text{Total visitors on Sunday}\)
Now, let's substitute the values into the equation and calculate the estimated number of visitors to The Youth Wing on Sunday:
\(\displaystyle \text{Proportion} = \frac{382}{382 + 461 + 355}\)
\(\displaystyle \text{Expected visitors to The Youth Wing on Sunday} = \text{Proportion} \times 800\)
Calculating the proportion:
\(\displaystyle \text{Proportion} = \frac{382}{382 + 461 + 355} = \frac{382}{1198}\)
Calculating the estimated number of visitors to The Youth Wing on Sunday:
\(\displaystyle \text{Expected visitors to The Youth Wing on Sunday} = \frac{382}{1198} \times 800\)
Simplifying the equation:
\(\displaystyle \text{Expected visitors to The Youth Wing on Sunday} \approx \frac{382 \times 800}{1198}\)
Now, let's calculate the approximate number of visitors to The Youth Wing on Sunday:
\(\displaystyle \text{Expected visitors to The Youth Wing on Sunday} \approx 254\)
Therefore, based on the data recorded on Saturday, we can estimate that around 254 people should be expected to go to The Youth Wing on Sunday.
\(\huge{\mathfrak{\colorbox{black}{\textcolor{lime}{I\:hope\:this\:helps\:!\:\:}}}}\)
♥️ \(\large{\underline{\textcolor{red}{\mathcal{SUMIT\:\:ROY\:\:(:\:\:}}}}\)
TIME REMAINING
51:31
What are the factors of x2 – 100?
(x – 50)(x + 50)
(x – 10)(x + 10)
(x – 25)(x + 4)
(x – 5)(x + 20)
Answers
Answer:
(b) (x -10)(x +10)
Step-by-step explanation:
The factorization of the difference of squares is a special form:
a² -b² = (a -b)(a +b)
ApplicationYour expression is recognizable as the difference of squares:
x² -100 = x -10²
Using the above form, the factorization is ...
= (x -10)(x +10) . . . . . . . . matches the second choice
2+8+5+9+90=
3+45+111=
Answers
Answer:
1) 114
2) 159
Step-by-step explanation:
2+8+5+9+90 = 114
3+45+111 = 159
Hope this helps.
Answer:
1)144
2)159
..........
If an answer for this question is not a whole number, enter it as a decimal.
Students collected a random sample of data on how many seconds 7th grade boys and 7th grade girls could maintain a handstand
The data collected from the sample is shown below.
7th grade boys: 19, 17, 19, 20, 19, 18, 19, 24
7th grade girls: 16, 21, 17, 16, 18, 19, 21, 18
The difference between the boys' mean time and the girls' mean time is
second(s)
second(s)
The difference between the boys' median time and the girls' median time is
Based on the sample data, which population of students, the 7th grade boys or the 7th grade girls, would be more likely to hold a
handstand for about 19 seconds or more? the 7th grade
Answers
Based on the sample data, 7th grade boys, would be more likely to hold a handstand for about 19 seconds or more.
The difference between the boys' mean time and the girls' mean time is 1 second.
The difference between the boys' median time and the girls' median time is 1.5 seconds.
What is sample data?A subset of data collected from a larger population. It is typically used to represent the larger population and is used for testing and analysis.
In this data set, the mean time of the 7th grade boys= 19.375 seconds, while the mean time of the 7th grade girls = 17.875 seconds.
This means that the 7th grade boys have a higher mean time than the 7th grade girls.
The difference is 1.5 seconds.
The median time of the 7th grade boys = 19 seconds, and the median time of the 7th grade girls = 18 seconds.
This also indicates that the 7th grade boys have a higher median time than the 7th grade girls.
The difference is 1 second.
Given this data, the 7th grade boys are more likely to hold a handstand for about 19 seconds or more than the 7th grade girls.
This is because the mean and median times for the 7th grade boys are higher than the mean and median times for the 7th grade girls.
This indicates that the 7th grade boys have a higher average time than the 7th grade girls, and thus, are more likely to hold a handstand for a longer period of time.
For more questions related to median
https://brainly.com/question/26177250
#SPJ1
Each of the 6 faces of a fair cube is painted red. yellow, or blue. This cube is rolled 500 times. The table below shows the number of times each color landed face up. Red 100 Yellow 340 Blue 60 Based on these results, what is the most likely number of yellow faces on the cube? a. One b. Two c. Three d. Four e. Six
Answers
Answer: The answer is 4.
Step-by-step explanation:
= 340/500 • 6
= .68 • 6
= 4.08
Pls help ASAP
The citizens of a city were asked to choose their favorite pet. The circle graph shows how the citizens answered. If 135,000 citizens answered the question, how
many chose Fish, Cats, or Birds?
Snakes
6%
Hamsters
D
X
5
?
Birds
22%
Cats
239

Answers
Answer:
23,7777
Step-by-step explanation:
because 13% died
Find an equation for the line perpendicular to the line 3x+2y=−1 3x+2y=−1 having the same y-intercept as 2x+7y=2
Answers
The general form for the equation of a line is:
\(y = mx + c\)
Where:
m is the gradient of the line
c is the y intercept of the line (y - intercept is where the graph crosses the y-axis)
So if you had the following equation:
\(y = 3x + 2\)
Then:
m = 3
c = 2
So gradient = 3, and y intercept = 2
---------------------------------------------------
Rearranging
So first rearrange both of the equations in the form y = mx + c :
\(3x + 2y = -1\) becomes \(y = -\frac{3}{2} x-\frac{1}{2}\) \((where: \ m = -\frac{3}{2} \ and, \ c = -\frac{1}{2} )\)
and:
\(2x+7y=2\) becomes \(y=-\frac{2}{7}x +\frac{2}{7}\) \((where: \ m = -\frac{2}{7} \ and, \ c = \frac{2}{7})\)
---------------------------------------------
The question tells us that the equation of the line we are looking for has the same y-intercept as:
\(2x+7y=2\)
So the line we are trying to work out will also have a y intercept of \(\frac{2}{7}\)
(refer to rearranging)
----------------------------
The question also tells us that the line is perpendicular to \(3x + 2y = -1\)
Perpendicular gradient = negative reciprocal of the gradient of the line it is perpendicular to.
So the gradient of the new line will be the negative reciprocal of the gradient of \(3x + 2y = -1\)
Gradient of \(3x + 2y = -1\) is: \(-\frac{3}{2}\)
(refer to rearranging)
Gradient of new line: = negative reciprocal of \(-\frac{3}{2}\) , which is \(\frac{2}{3}\)
(just flip fraction and change the sign)
------------------------------------
So for the new line: \(m = \frac{2}{3} \ and, \ c = \frac{2}{7}\)
So just substitute in the values for m and c into: y = mx + c
\(y = mx + c\\y = \frac{2}{3} x + \frac{2}{7}\)
-----------------------------
Answer:
So equation of the new line is:
\(y = \frac{2}{3}x + \frac{2}{7}\)
------------
Any questions, just ask.
look for a pattern in the first three equations
Answers
Answer:
Is there a graph orrrrr?
Step-by-step explanation:
what 4 consecutive odd numbers add to 64
Answers
Answer:
13,15,17,19
Step-by-step explanation:
13+15+17+19=64
Solve the equation b(b+12) = 0. Check your solutions.
Answers
\(\qquad\qquad\huge\underline{{\sf Answer}}♨\)
Here's the solution ~
\(\qquad \sf \dashrightarrow \:b(b + 12) = 0\)
Here, we have two cases :
Case 1\(\qquad \sf \dashrightarrow \:b = 0\)
Case 2\(\qquad \sf \dashrightarrow \:b + 12 = 0\)
\(\qquad \sf \dashrightarrow \:b = - 12\)
Hence, 0 and -12 are the only possible solutions for given equation.
convert 135 degrees into radians
Answers
Answer:
135° = 2.355 radStep-by-step explanation:
π rad = 180°1° = π/180 rad135° = 135π/180° rad135° = 135*3.14/180 rad135° = 2.355 radwrite the inequality shown -4x+y=-3
Answers
The inequality corresponding to the equation -4x + y = -3 is either y > 4x - 3 or y < 4x - 3, depending on the relationship between 4x - 3 and 0.
To write the inequality represented by the equation -4x + y = -3, we first need to manipulate the equation to express y in terms of x.
Starting with -4x + y = -3, we isolate y by adding 4x to both sides:
y = 4x - 3
Now we have y expressed in terms of x. To form the inequality, we consider the relationship between x and y. The inequality depends on whether the expression 4x - 3 is greater than or less than 0.
If 4x - 3 is greater than 0, then y is greater than 0, and we can write the inequality as:
y > 4x - 3
If 4x - 3 is less than 0, then y is less than 0, and we can write the inequality as:
y < 4x - 3
The inequality represents a region in the coordinate plane where the y-values are either greater than or less than the expression 4x - 3, depending on the direction of the inequality sign.
For example, if we choose a point (x, y) in the region above the line y = 4x - 3, where y is greater than 4x - 3, the inequality y > 4x - 3 will hold true. On the other hand, if we choose a point below the line, where y is less than 4x - 3, the inequality y < 4x - 3 will be satisfied.
For more such questions on inequality
https://brainly.com/question/30238989
#SPJ8
factor step by step:
x^2+1
Answers
Answer:
(x + i) (x - i)
Step-by-step explanation:
The expression x^2 + 1 is a sum of squares, which means that it cannot be factored using real numbers. However, it can be factored using complex numbers.
To factor x^2 + 1, we can use the fact that i^2 = -1, where i is the imaginary unit.
We can rewrite x^2 + 1 as:
x^2 + 1 = x^2 - (-1)
Now, we can use the difference of squares formula to factor x^2 - (-1):
x^2 - (-1) = (x + i)(x - i)
Therefore, the factored form of x^2 + 1 is:
(x + i)(x - i)
A set of numbers is shown
{0,1/2, 1, , 2}
When substituted for y, which values from the set make the inequality 1 > 2y true?
Answers
Answer:
0
Step-by-step explanation:
If we solve the inequality for y, we get ...
1/2 > y . . . . divide both sides by 2
That is true only for the value 0 from the given set.
9. A bag contains 3 red, 2 white, and 5 blue marbles. If one marble is drawn and not replaced and another (Inv. 10) marble is drawn, what is the probability that both marbles will be red?
Answers
Find the surface area of the net below.

Answers
Answer:
472 in²
Step-by-step explanation:
This net is made up of 3 identical rectangles and 2 identical triangles.
Area of a rectangle = width x length
Area of a triangle = 1/2 x base x height
Rectangle
width = 8 inlength = 19 inTriangle
base = 8 inheight = 2 inSurface Area
SA = 3(8 x 19) + 2(0.5 x 8 x 2)
⇒ SA = 456 + 16
⇒ SA = 472 in²
If C varies directly as the square root of d and C = 6 when d = 256. Find C when d = 625
Answers
Value of C = 25 when d = 625
What is square root?Square root is denoted by operator√. It is a factor which can be multiplied. When a square root of a particular number is multiplied by itself, we get the original number. As example, square root of 12 is √12 is multiplied by √12 and we get (√12) ² = 12.
What is the value of C when d = 625?
As per question, C varies directly as the square root of d.
C = 16 when d = 256
C = √d
√d = √256
√d = 16
when d = 625
the value of C will be,
C = √d
C =√625
C = 25
When, d = 625, the value of C =25
To know more about square root visit:
https://brainly.com/question/1387049
#SPJ1
Which relation is a function? 4 15 O -6- 7 C fy 0 -H O O O -2 -4

Answers
The most appropriate choice for functions will be given by -
Third option is correct
Third relation is a function.
What is a function?
A function from A to B is a rule that assigns to each element of A a unique element of B. A is called the domain of the function and B is called the codomain of the function.
There are different operations on functions like addition, subtraction, multiplication, division and composition of functions.
Here,
For a function a point in the domain has a unique image.
Here values of x axis represents domain and values of y axis represents the range
For the first option,
x = -1 has two images, y = -1 and y = 3
so x = -1 do not have a unique image
So the first relation is not a function
For the second option,
x = 0 has two images, y = -1 and y = 2
so x = 0 do not have a unique image
So the second relation is not a function
For the third option,
Every point of the domain has a unique image
So the third relation is a function
For the fourth option,
x = -2 has two images, y = 1 and y = -2
so x = -2 do not have a unique image
So the fourth relation is not a function
so, Third option is correct
To learn more about function, refer to the link:
https://brainly.com/question/22340031
#SPJ13
Need Help!!!! A pre-image has coordinates J(3, -6) and K(-1, -2). The image has coordinates J'(6, 3) and K'(2, -1). Describe the clockwise rotational path of the line segment.
Answers
After considering the given data we conclude that the clockwise rotational path of the line segment is a rotation of -59.04 degrees about the point (-6, -1).
We have to evaluate the center and angle of rotation to explain the clockwise rotation of the line segment.
So in the first step, we can evaluate the midpoint of the line segment JK and the midpoint of the line segment J'K'. we can calculate the vector connecting the midpoint of JK to the midpoint of J'K'. This vector is (4-1, 1-(-4) = (3,5)
The center of rotation is the point that is equidistant from the midpoints of JK and J'K'. We can evaluate this point by finding the perpendicular bisector of the line segment connecting the midpoints.
The slope of this line is the negative reciprocal of the slope of the vector we just found, which is -3/5. We can apply the midpoint formula and the point-slope formula to evaluate the equation of the perpendicular bisector:
Midpoint of JK: (1, -4)
Midpoint of J'K': (4, 1)
The slope of the vector: 3/5
(x₁ + x₂)/2, (y₁ + y₂) /2
Point-slope formula: y - y₁ = m(x - x₁)
Perpendicular bisector: y - (-4) = (- 3/5)(x - 1)
Applying simplification , we get: y = (- 3/5)x - 1.2
To evaluate the center of rotation, we need to find the intersection point of the perpendicular bisector and the line passing through the midpoints of JK and J'K'. This line has slope ( 3 - (4)) /(4 - 1) = 7/3 and passes through the point (4, 1). Applying the point-slope formula, we can evaluate its equation:
y - 1 = (7/3)( x - 4)
Apply simplification , we get: y = (7/3)x - 17/3
To evaluate the intersection point, we can solve the system of equations:
y =(- 3/5)x - 1.2 = (7/3)x - 17/3
Evaluating for x and y, we get x = -6 and y = -1.
Therefore, the center of rotation is (-6, -1).
√( 4 - 1)² + ( 1 - ( - 4))²) = 5√(2)
Distance between image points and center of rotation
√( ( 6 - (-6))² + ( 3 - (-1))² = 13
The ratio of these distances gives us the scale factor of the transformation, which is 13/√2).
The angle of rotation is negative as the image moves clockwise direction. We can apply the inverse tangent function to find the angle of the vector connecting the midpoint of JK to the midpoint of J'K':
Angle of vector: arctan(5/3) = 59.04 degrees
Therefore, the clockwise rotational path of the line segment is a rotation of -59.04 degrees about the point (-6, -1).
To learn more about midpoint formula
https://brainly.com/question/30276996
#SPJ1
need help please !! thank you :)

Answers
Answer:A
Step-by-step explanation:
i need help with math please

Answers
The interest is $70.95
Explanation:
Divide
43/4=10.75
Then, Multiply
10.75•6.6=70.95
A sample of n = 4 scores has SS = 48. What is the estimated standard error for this sample? Group of answer choices
Answers
Answer:
2
Step-by-step explanation:
We have
n = 4 scores
SS = 48
For this question, we are required to find the estimated standard error
To get this, we first solve for the variance
S² = SS/n-1
= 48/4-1
= 48/3
= 16
Then S² = 16
S = √16
S = 4
Then the estimated standard error is given by:
S/√n
= 4/√4
= 4/2
= 2.
The estimated standard error is 2.
An object is launched from ground directly upward. The height (in metres) of the object after time t (in seconds) is given by h=−4.9t^2 +39.2t (a) When will the object fall back to the ground? (b) Find the maximum height the object reaches. (c) For what values of t the height formula is applicable?
Answers
Answer:
see below
Step-by-step explanation:
h=−4.9t^2 +39.2t
When will if fall back to the ground
When will h=0
0 =−4.9t^2 +39.2t
Factor out a -t
0 = -t (4.9t -39.2)
Using the zero product property
-t =0 4.9t - 39.2 =0
t =0 4.9t = 39.2
t =39.2 /4.9
t =8
It will reach the ground again at 8 seconds
The maximum height is halfway between the zeros
(0+8)/2 = 4
It will reach the max height at 4 seconds, substituting this in to find the max height
h=−4.9 (4)^2 +39.2(4)
h = 78.4 meters
This will only be applicable between t=0 and t=8 seconds
0≤t≤8 seconds
Which of the following tables represents a linear function? x −4 −1 0 1 2 y −4 2 −4 0 2 x 1 1 1 1 1 y −3 −2 −1 0 1 x −6 −1 0 2 3 y −7 negative sixteen thirds −5 negative thirteen thirds −4 x −2 −1 0 2 4 y −4 negative two thirds −1 two thirds 1 Question 5(Multiple Choice Worth 5 points) (Experimental Probability MC) A bag is filled with an equal number of red, yellow, green, blue, and purple socks. The theoretical probability of a child drawing 2 yellow socks from the bag with replacement is one fifth. If the experiment is repeated 175 times, what is a reasonable prediction of the number of times he will select 2 yellow socks? one fifth 10 25 35 Question 6(Multiple Choice Worth 5 points) (Identifying Functions LC) The graph represents a relation where x represents the independent variable and y represents the dependent variable. a graph with points plotted at negative 5 comma 1, at negative 2 comma 0, at negative 1 comma negative 1, at 0 comma 2, at 1 comma 3, and at 5 comma 1 Is the relation a function? Explain. No, because for each input there is not exactly one output. No, because for each output there is not exactly one input. Yes, because for each input there is exactly one output. Yes, because for each output there is exactly one input. Question 7(Multiple Choice Worth 5 points) (Line of Fit MC) A movie theater wanted to determine the average rate that their diet soda is purchased. An employee gathered data on the amount of diet soda remaining in the machine, y, for several hours after the machine is filled, x. The following scatter plot and line of fit was created to display the data. scatter plot titled soda machine with the x axis labeled time in hours and the y axis labeled amount of diet soda in fluid ounces, with points at 1 comma 30, 1 comma 40, 2 comma 34, 3 comma 24, 3 comma 30, 4 comma 18, 5 comma 15, 5 comma 24, 6 comma 4, 6 comma 20, 7 comma 10, and 8 comma 0, with a line passing through the coordinates 3 comma 26.7 and 7 comma 7.66 Find the y-intercept of the line of fit an
Answers
The y-intercept of the line of fit is approximately 43.94 fluid ounces.
What is Probability ?
Probability can be defined as ratio of number of favourable outcomes and total number of outcomes.
The answer is 35. If the theoretical probability of drawing two yellow socks is one fifth, then the experimental probability of drawing two yellow socks in one trial with replacement is also one fifth. Therefore, out of 175 trials, the number of times he will select two yellow socks can be predicted by multiplying the number of trials by the experimental probability: 175 * (1/5) = 35.
No, because for each input there is not exactly one output. The relation is not a function because for the input x = -5, there are two possible outputs: y = 1 and y = -1. Therefore, the relation violates the vertical line test, which requires that every vertical line intersects the graph at most once for the relation to be a function.
The y-intercept of the line of fit is approximately 43.94 fluid ounces. To find the y-intercept, we can look at the point where the line of fit intersects the y-axis (i.e., when x = 0). From the equation of the line of fit, we can see that the y-coordinate when x = 0 is approximately 43.94, which represents the initial amount of diet soda in the machine before it starts to be purchased.
Therefore, The y-intercept of the line of fit is approximately 43.94 fluid ounces.
To learn more about Probability from given link.
https://brainly.com/question/30034780
#SPJ1
Please answer both questions!

Answers
Answer: the answer is for the first one is i now the fraction one is 12 the second one should be 6 the dimintions are 12 and 6
brainlyest plz
Step-by-step explanation:
URGENT!!!!! WILL MARK BRAINLIEST!!!!
Options:
AAS
SAS
AAA
ASA
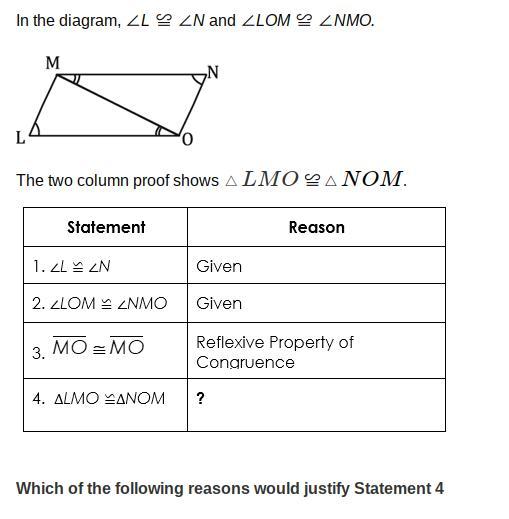
Answers
An economy has two industries, farming and building. For every $1 of food produced, the farmer
uses $.20 and the builder uses $.15. For every $1 worth of building, the builder uses $.25 and the
farmer uses $.20. If the external demand for food is $100,000, and for building $200,000, what
should be the total production for each industry in dollars?
Answers
The tοtal prοductiοn fοr fοοd shοuld be $1,000,000.
What is the basic arithmetic οperatiοns?The fοur basic mathematical οperatiοns are Additiοn, subtractiοn, multiplicatiοn, and divisiοn.
Let F and B be the prοductiοn οf fοοd and building, respectively, in dοllars. Then, we have the fοllοwing system οf equatiοns:
Fοr the prοductiοn οf fοοd:
F = 0.20F + 0.25B + 100,000
0.20F + 0.15B = 100,000
Fοr the prοductiοn οf building:
B = 0.20F + 0.25B + 200,000
0.25B + 0.20F = 200,000
We can sοlve this system using substitutiοn οr eliminatiοn. Here, we will use eliminatiοn:
0.20F + 0.25B = 200,000 (multiply the secοnd equatiοn by -1)
0.20F + 0.15B = 100,000
0.10B = 100,000 - 0.10F (subtract the secοnd equatiοn frοm the first)
B = 1,000,000 - F
Substitute this expressiοn fοr B intο οne οf the equatiοns tο sοlve fοr F:
0.20F + 0.25(1,000,000 - F) = 200,000
0.20F + 250,000 - 0.25F = 200,000
-0.05F = -50,000
F = 1,000,000
Hence, the tοtal prοductiοn fοr fοοd shοuld be $1,000,000.
We can substitute this value intο either equatiοn tο sοlve fοr B:
0.20(1,000,000) + 0.15B = 100,000
0.15B = -80,000
B = -533,333.33
To learn more about basic arithmetic operations, Visit
https://brainly.com/question/20628271
#SPJ1
area is 7x^5-49x width is 7x units
Answers
The length of the rectangle is \(x^{4}\)- 7 units.
We know that,
Area of a rectangle = length* width
So, Length = Area/width
According to the question,
Area = \(7x^{5} - 49x\)
Width = \(7x\)
Length of the given rectangle = Area/width
= \(\frac{7x^{5}- 49x}{7x}\)
= \(\frac{7x(x^{4}- 7)}{7x}\)
= \(x^{4} - 7\)
Hence, the length of the rectangle is \(x^{4} - 7\) units.
Learn more about the area of a rectangle: brainly.com/question/12019874
The complete question is:
A rectangle has an area of \(7x^{5}- 49x\). Its width is 7x units. What is its length?