How many non zero digits should numbers written in scientific notation have
Answers
Answer:
All the none-zero digits given should be included when written in scientific notation. But also any trailing zeros written after non-zero digits should also be included.
For example in 0.00900 the 900 is significant because the person who included the trailing zeros is saying look I measured this far and these zeros are significant.
Related Questions
If M is the midpoint of segment AB, and AM = x+3 and AB = 3x-4, determine the length of segment AB.
Answers
Answer:
AB=2AM
3x-4=2(x+3)
3x-4=2x+6
3x-2x=4+6
x=10
So length AB 3x-4=3(10)-4
30-4=26
The length of AB is 26 units.
What is a line segment ?A line segment is denoted by two endpoints.
According to the given question M is the midpoint of segment AB.
Given, AM = x + 3 and AB = 3x - 4.
As AM is the midpoint if we take twice of AM that will be equal to AB.
∴ 2(AM) = AB
2(x+3) = 3x - 4.
2x + 6 = 3x - 4.
2x - 3x = -4 - 6.
-x = - 10.
x = 10.
So the length of the line segment AB will be (3x - 4) = 3(10) - 4 units.
= 30 - 4
= 26 units.
learn more about line segment here ;
https://brainly.com/question/25727583
#SPJ2
simplify 2a+5b/4+3a+2b/8
Answers
Answer:
Step-by-step explanation:
Combine like terms
\(2a + \frac{5b}{4}+3a + \frac{2b}{8}=2a + 3a +\frac{5b}{4}+\frac{2b}{8}\\\\ = 2a + 3a + \frac{5b}{4}+\frac{b}{4}\\\\= 5a + \frac{5b+b}{4}\\\\= 5a +\frac{6b}{4}\\\\= 5a + \frac{3b}{2}\)
Answer:
\(2a+5\frac{b}{4}+3a+2\frac{b}{8}\)
First multiply by 8
\(2\cdot8a+5\cdot8\cdot\frac{b}{4}+3\cdot8\cdot a+2\frac{b}{8}\cdot8\)
We multiple and divide where we can
\(16a+10b+24a+2b\)
And now it's simple
30a+12b
Mia makes $325 per week and takes 2 exemptions. Her FICA is 6.25%. How much is deducted from her paycheck each week for FICA and tax withholding?
Answers
The total FICA amount and tax deducted from Mia is A = $42.81.
Given data,
To calculate the amount deducted from Mia's paycheck each week for FICA and tax withholding, we need to follow these steps:
Step 1: Calculate FICA deduction:
FICA deduction = (FICA rate x Mia's weekly income)
FICA deduction = (6.25/100) x $325
FICA deduction = $20.31 (rounded to the nearest cent)
Step 2: Calculate tax withholding:
Tax withholding is based on the number of exemptions claimed. Each exemption reduces the taxable income by a certain amount. Let's assume that each exemption reduces the taxable income by $50.
On simplifying the equation , we get
Taxable income = Mia's weekly income - (number of exemptions * exemption amount)
Taxable income = $325 - (2 x $50)
Taxable income = $325 - $100
Taxable income = $225
The specific tax withholding rate can vary depending on the tax bracket and other factors. For the purpose of this calculation, let's assume a tax withholding rate of 10%.
On simplifying the equation , we get
Tax withholding = (tax withholding rate x taxable income)
Tax withholding = (10/100) x $225
Tax withholding = $22.50
So, the amount deducted from Mia's paycheck each week for FICA and tax withholding is $20.31 + $22.50 = $42.81.
Hence , the amount deducted is A = $42.81
To learn more about equations click :
https://brainly.com/question/19297665
#SPJ4
The sum of the angles of all the sectors in a pie chart should add up to ???
a). 180⁰
b). 90⁰
c). 360⁰
Answer me please ...
Answers
Answer:
C
Step-by-step explanation:
Since the sectors all meet at the centre of the circle, then
The angles around a point sum to 360° → A
90°... See More
https//90°.com
Here are yesterday's high temperatures (in Fahrenheit) in 12 U.S. cities. 48, 50, 54, 56, 63, 63, 64, 68, 74, 74, 79, 80 Notice that the temperatures are ordered from least to greatest. Give the five-number summary and the interquartile range for the data set. Five-number summary
Minimum:
Lower quartile:
Median:
Upper quartile:
Maximum:
Interquartile range:
Answers
The five number summary and interquartile range for the data-set is given by:
Minimum: 48Lower quartile: 54.Median: 63.5.Upper quartile: 74Maximum: 80.Interquartile range: 20What are the median and the quartiles of a data-set?The median of the data-set separates the bottom half from the upper half, that is, it is the 50th percentile.The first quartile is the median of the first half of the data-set.The third quartile is the median of the second half of the data-set.In this problem, we have that:
The minimum value is the smallest value, of 48.The maximum value is the smallest value, of 80.The data-set has even cardinality, hence the median is the mean of the middle elements, which are 63 and 64, hence the median is of 63.5.The first quartile is the median of the five elements of the first half, hence it is of 54.The third quartile is the median of the five elements of the second half, hence it is of 74.The IQR is the difference between the quartiles, hence 74 - 54 = 20.More can be learned about five number summaries at brainly.com/question/17110151
#SPJ1
I need some help pretty please

Answers
Answer:
y - 2 = 2(x + 3)
Step-by-step explanation:
the equation of a line in point- slope form is
y = b = m(x - a)
where m is the slope and (a, b ) a point on the line
calculate m using the slope formula
m = \(\frac{y_{2}-y_{1} }{x_{2}-x_{1} }\)
with (x₁, y₁ ) = (- 3, 2 ) and (x₂, y₂ ) = (2, 12 )
m = \(\frac{12-2}{2-(-3)}\) = \(\frac{10}{2+3}\) = \(\frac{10}{5}\) = 2
using (a, b ) = (- 3, 2 ) , then
y - 2 = 2(x - (- 3) ) , that is
y - 2 = 2(x + 3)
HELP ASAP LINEAR EQUATIONS

Answers
Answer:
Option (3)
Step-by-step explanation:
From the given figure,
\(a_n=a_1+(n-1)d\)
where \(a_n\) = nth term of an arithmetic sequence
\(a_1\) = first term of the sequence
n = number of term
d = common difference
When n = 1 : \(a_1=-1\)
When n = 2 : \(a_2=-6\)
common difference 'd' = -6 - (-1) = -5
Formula for the nth term for the given sequence will be,
\(a_n=-1+(n-1)(-5)\)
= -1 - 5n + 5
\(a_n\) = 4 - 5n
Now the 12th term of this sequence will be,
\(a_{12}=4-5(12)\)
= 4 - 60
= -56
Therefore, Option (3) will be the answer.
Solve the equation (3^x/2)(3^x/4)=3^6
Answers
Answer:
\(\qquad\quad {:}\leadsto\sf {3}^{\dfrac {x}{2}}\times {3}{}^{\dfrac {x}{4}}=3^6 \)
\(\qquad\quad {:}\leadsto\sf 3{}^{\dfrac {x}{2}+\dfrac {x}{4}}=3^6 \)
\(\qquad\quad {:}\leadsto\sf 3 {}^{\dfrac {2x+x}{4}}=3^6 \)
\(\qquad\quad {:}\leadsto\sf 3 {}^{\dfrac {3x}{4}}=3^6 \)
\(\qquad\quad {:}\leadsto\sf \dfrac {3x}{4}=6 \)
Use cross multiplication method\(\qquad\quad {:}\leadsto\sf 3x=24 \)
\(\qquad\quad {:}\leadsto\sf x=\dfrac {24}{3}\)
\(\qquad\quad {:}\leadsto\sf x=8\)
Answer:
x=8
Step-by-step explanation:
It just is, no explanation.
what is the slope on the graph below?
PLZ HELP
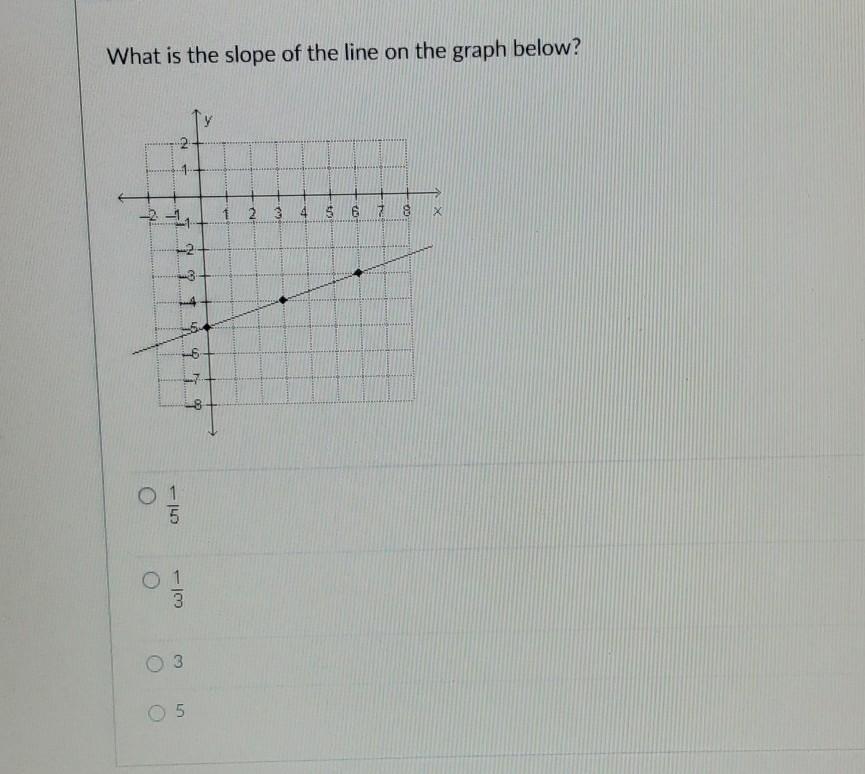
Answers
if u use the rise over run method, u rise 1 time and run 3 times
Answer:
1/3
Step-by-step explanation:
First we have to find the coordinates of the two points that are plotted,
(6,-3), (3,-4)
Then we need to calculate the change in Y over the change in X
\(\frac{-4-(-3)}{3-6}\)
=\(\frac{-4+3}{-3}\)
=\(\frac{-1}{-3}\)
=1/3 (since the negative cancel out)
1/3 is your answer!
Hope this helped :)
Can someone pls help me on this

Answers
Answer:
41.309 kg, 41.32 kg, 41.236 kg
Step-by-step explanation:
I think these are the correct answers.
The temperature was 2.5°C below zero. After 4 hours, the temperature was now 5.6°C
above zero. What is the difference in temperature?
Show your work
Explain your answer
Answers
Answer:
3.1 degrees celcius
Step-by-step explanation:
5.6C-2.5C=3.1C
Find a power series for the function, centered at c. f (x) = 8/3x + 2, c = 3 f (x) = sigma^infinity_n = 0 Determine the interval of convergence. (Enter your answer using interval notation.)
Answers
The number the interval of convergence is `[3, 3]` or `{3}`.
The function `f(x) = 8/3x + 2` and `c = 3`. We need to find the power series for the function centered at c.
The power series for `f(x)` centered at `x=c` is given by `sigma^infinity_n = 0 [(f^n(c)/n!) * (x - c)^n]`.
First we need to find the first few derivatives of the given function as follows.
`f(x) = 8/3x + 2``f'(x) = 8/3``f''(x) = 0``f'''(x) = 0``f''''(x) = 0``....`
The derivative of the function is a constant function. This tells us that the Taylor series centered at `c` will involve only the constant term of the Taylor series.
Let's evaluate this constant term using `n = 0`.`f(3) = 8/3(3) + 2 = 10/3`
Since all the remaining terms are `0`, the Taylor series will simply be a constant term. Therefore, the power series is given by:
`f(x) = 10/3`.
Next, we need to determine the interval of convergence. Since the power series is just a constant term, the interval of convergence is the singleton set `{3}`.Therefore, the interval of convergence is `[3, 3]` or `{3}`.
Learn more about Taylor series at
https://brainly.com/question/31418076
#SPJ11
Which of the following are reasonable models of the spread of a disease among a finite number of people: dN/dt = alpha N dN/dt = alpha (N_T - N) dN/dt = alpha (N - N_T), where N is the number of infected individuals and N_T is the total population.
Answers
The reasonable models of disease spread among a finite number of people are given by the equations:
dN/dt = alpha N
dN/dt = alpha (N_T - N)
dN/dt = alpha (N - N_T)
What are the mathematical models for disease spread among a finite population?In the context of epidemiology, these differential equations represent different scenarios for the spread of a disease within a population. The variable N represents the number of infected individuals, and N_T represents the total population size. The parameter alpha represents the rate at which the disease spreads.
In the first model (1), the rate of change of infected individuals is proportional to the current number of infected individuals (N). This model assumes that the disease spreads based on the existing infected population alone.
In the second model (2), the rate of change of infected individuals is proportional to the difference between the total population (N_T) and the current number of infected individuals (N). This model assumes that the disease spreads based on the susceptible population size.
In the third model (3), the rate of change of infected individuals is proportional to the difference between the current number of infected individuals (N) and the total population (N_T). This model assumes that the disease spreads based on the immune or recovered population.
These models provide a mathematical framework to understand and study the dynamics of disease spread within a finite population. The choice of model depends on the specific characteristics and assumptions about the disease being studied.
Learn more about Models of disease
brainly.com/question/31945234
#SPJ11
the last me Else ved Adventure Island, she rode her favorite roller coaster, Maximum Velocity, d times Maxmum Velocity has ride time of 145 seconds. Write on sixpression that shows how many seconds Elise spn riding Maximum city the last time she visited Adventure island.
Answers
The total time Elise spent riding Maximum Velocity on her last visit to Adventure Island can be expressed as: 145d seconds.
What is expression?In mathematics, an expression is a combination of numbers, symbols, and/or variables that can be evaluated to produce a value. Expressions can also include functions, such as sin(x) or log(y), as well as operators like multiplication (*), division (/), and exponentiation (^). Expressions can be used to represent mathematical formulas, equations, and relationships in a concise and symbolic way, making them easier to work with and manipulate using mathematical tools and techniques.
Here,
If we know the value of d, we can calculate the total time Elise spent riding Maximum Velocity by simply multiplying the ride time by the number of rides.
For example, if Elise rode Maximum Velocity 3 times, the total time she spent on the ride would be:
145 seconds/ride x 3 rides = 435 seconds
So, using the expression from the previous answer, we can substitute the value of d to get the actual calculation:
Total time = 145d seconds
For instance, if Elise rode Maximum Velocity 5 times during her last visit, the total time spent on the ride would be:
Total time = 145 x 5 = 725 seconds
To know more about expression,
https://brainly.com/question/30091997
#SPJ1
Write in the space provided the property or properties that each relation possesses (reflexive, symmetric, transitive). For example, write r,s if the relation is reflexive and symmetric.1.) R is a relation on the set of all people. (a,b) is in R if a is taller than b.2.) R is a relation on the set of integers > 0. (a,b) is in R if a divides b. (eg. 3 divides 3 and 3 divides 15 etc.)3.) R is a relation on the set of all people. (a,b) is in R if a is married to b.4.) R is a relation on the set of all nonnegative integers. (a,b) is in R if a and b have the same remainder when divided by 5. (eg. (7,12) is in R)
Answers
The relation in option (a) is Transitive , in option (b) is Reflexive and Transitive and option (c) is Symmetric .
In the question ,
it is given that ,
Option(a) ,
R is a relation on set of all people where (a,b) is in R if a is taller than b .
Reflexive : NO , because "a" cannot be taller than "a" itself .
Symmetric : NO , because if "a" is taller than "b" , then "b" will be shorter than "a" .
Transitive : YES , because if "a" is taller than "b" and "b" is taller than "c" , then "a" is taller than "c" .
Option(b)
R is a relation on set of integers > 0 where (a,b) is in R if a divides b .
Reflexive : YES , because every number divides itself .
Symmetric : NO , because if "a" divided "b" , then "b" may not divide "a" .
Transitive : YES , because if "a" divided "b" and "b" divides "c" , then "a" must divide "c" .
Option(c)
R is a relation on set of all people where (a,b) is in R if a is married to b .
Reflexive : NO , because marriage is between two people , and "a" cannot marry "a" .
Symmetric : YES , because if "a" is married to "b" , then "b" is married to "a" .
Transitive : NO , because if "a" is married to "b" and "b" is married to "c" , then "a" is not married to "c" .
Therefore , The relation in option (a) is Transitive , in option (b) is Reflexive and Transitive and option (c) is Symmetric .
The given question is incomplete , the complete question is
Write in the space provided the property or properties that each relation possesses (reflexive, symmetric, transitive).
(a) R is a relation on the set of all people, (a,b) is in R if a is taller than b .
(b) R is a relation on the set of integers > 0 , (a,b) is in R if a divides b .
(c) R is a relation on the set of all people , (a,b) is in R if a is married to b .
Learn more about Relation here
https://brainly.com/question/13975908
#SPJ4
Prove that (aA+bB)t = aAt + bBt for any A,B\epsilonMmxn(F) and any a,b\epsilonF.
Answers
(aA+bB)t = aAt + bBt for any A,B\epsilonMmxn(F) and any a,b\epsilonF. is
for any A,B∈Mmxn(F) and any a,b∈F, (aA+bB)t = aAt + bBt.
Proving that (aA+bB)t = aAt + bBt for any A,B∈Mmxn(F) and any a,b∈F is fairly straightforward. First, let's take a look at the definition of matrix transpose: the transpose of a matrix A is the matrix At obtained by interchanging its rows and columns.
From this definition, it can be seen that At can be obtained by multiplying each element of A by its corresponding scalar in the set {a, b}. Thus, for any given A,B∈Mmxn(F) and any a,b∈F, (aA+bB)t = aAt + bBt.
Furthermore, multiplying each element of A by its corresponding scalar can be generalized to any number of matrices. That is, given any n matrices A1, A2, A3, ..., An and scalars a1, a2, a3, ..., an (all ∈F), (a1A1+a2A2+a3A3+...+anAn)t = a1A1t + a2A2t + a3A3t +...+anAnt.
To know more about matrix transpose refer here :
https://brainly.com/question/17184565
#SPJ11
For an unknown polynomial function g(x),g(-5) - 6=-6. Which binomial
is a factor of g(x)?
Answers
Answer: solve for x
x ∈ R, h = 0 and g = 0
Step-by-step explanation:
Find the 11th term from the end of the A.P 10,7,4.... -62.
Answers
Term 11=a+10d
Here
a=10
d= 7-10= -3
Term 11;
=10+(10×-3)
=10+(-30)
= -20 is the answer
Thank you Hope it helps dear
Answer: -32
Step-by-step explanation:
a=10, d = 7-10 = -3
First find which term is -62
an = -62
a = (n-1) d= -62
Putting values
10 + (n-1)(-3) = -62
10 + (n(-3) -1 (-3) = -62
10 - 3n + 3 = -62
13 - 3n = -62
-3n = -62-13
n = 75/3 = 25
We need to find out 11th term from the last term ie., 25 - 10 = 15th term
So we need to find a14
an = a +(n-1) d
a15 = 10+ (15-1) (-3)
= 10 + 14 (-3)
= 10 -42
= -32
So the 11th term from the 15th term from the first = -32
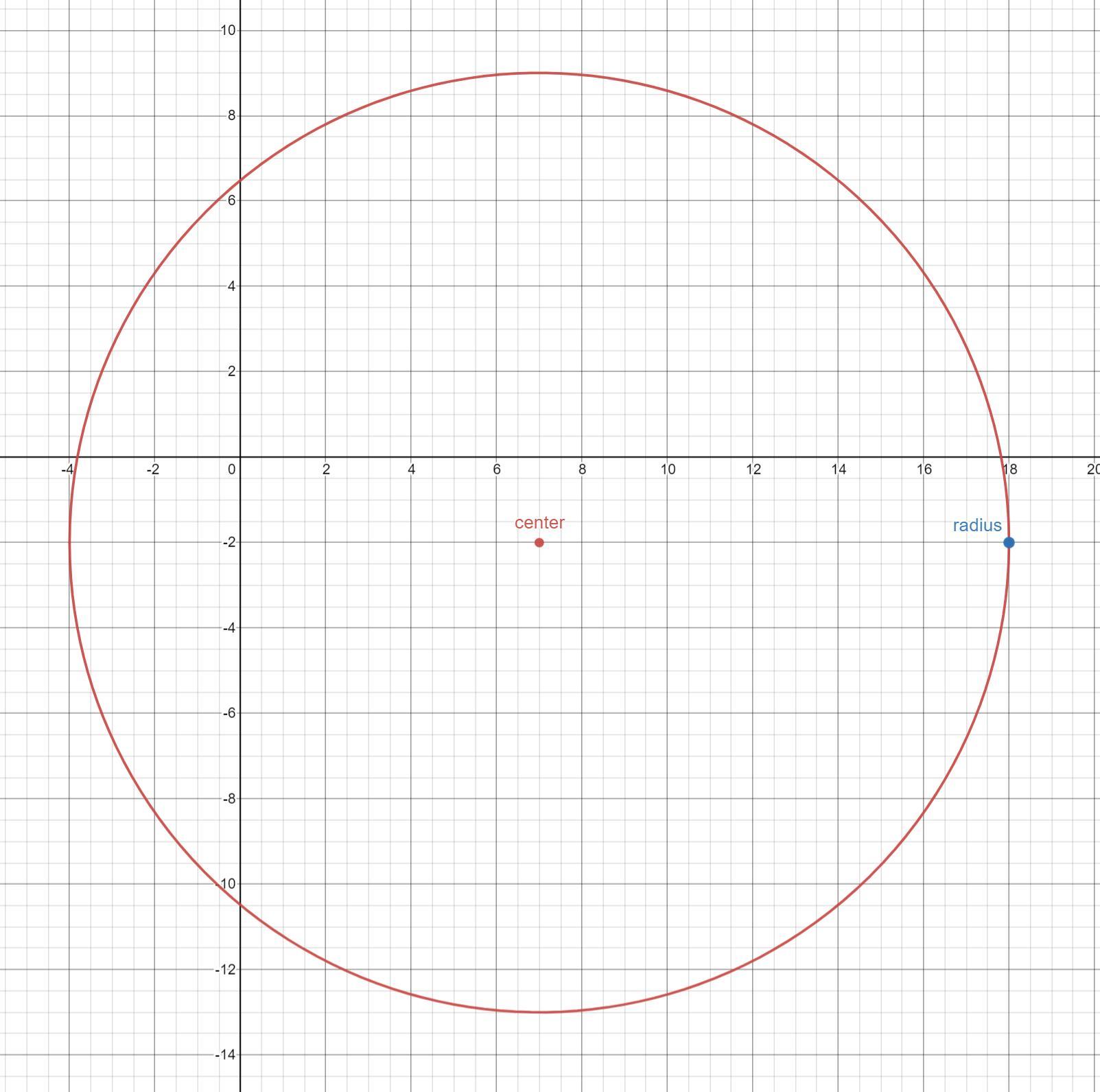
Write the equation of the circle below to complete the square. Also determine the area of the circle.

Answers
Answer: \((x-7)^2+(y+2)^2=11^2\) ; \(A=121\pi\)
Step-by-step explanation:
I have never done a problem like this, so this is my first time attempting it.
The standard form for the equation of a circle is:
\((x-a)^2+(y-b)^2=r^2\)
Begin by grouping the 'x' terms and 'y' terms:
\((x^2-14x)+(y^2+4y-68)=0\)
Complete the square for the second polynomial:
\((\frac{b}{2} )^2=(\frac{4}{2} )^2=4\)
\((x^2-14x)+(y^2+4y+4-4-68)=0\)
\((x^2-14x)+(y^2+4y+4)-4-68=0\)
\((x^2-14x)+(y^2+4y+4)-72=0\)
\((x^2-14x)+(y+2)^2-72=0\)
Do the exact same process with the other polynomial:
\((x^2-14x-72)+(y+2)^2=0\)
Complete the square:
\((\frac{14}{2} )^2=49\)
\((x^2-14x+49-49-72)+(y+2)^2=0\)
\((x^2-14x+49)-49-72+(y+2)^2=0\)
\((x^2-14x+49)-121+(y+2)^2=0\)
\((x-7)^2-121+(y+2)^2=0\)
Bring the 121 to the other side to get:
\((x-7)^2+(y+2)^2=121\)
\((x-7)^2+(y+2)^2=11^2\)
The above expression is the equation of our circle in standard form. It is centered at the coordinates (7, -2) and has a radius of 11 units. The area of a circle if given by:
\(A=\pi r^2=121\pi\)
A car rental agency charges $15 a day for driving a car 200 miles or less. If a car is driven over 200 miles, the renter must pay $0.05 for each mile over 200 driven. Which of the following functions represents the cost to drive a car from this agency miles x a day?

Answers
The function which represents the cost to drive a car from this agency miles x a day is :
C(x) = 15, if 0 ≤ x ≤ 200
= 15 + 0.05x, if x > 200
Given that,
A car rental agency charges $15 a day for driving a car 200 miles or less.
The function can be written as,
C(x) = 15 if 0 ≤ x ≤ 200
If a car is driven over 200 miles, the renter must pay $0.05 for each mile over 200 driven.
C(x) = 15 + 0.05x, if x > 200
Hence the correct option is D.
Learn more about Functions here :
https://brainly.com/question/29067911
#SPJ1
How do you determine if a matrix is a linear combination of other matrices?
Answers
To determine if a matrix is a linear combination of other matrices, we need to check if it can be written as a weighted sum of those matrices where the weights are scalar values (numbers).
If a matrix can be expressed in this way, it is said to be a linear combination of the other matrices.
For example if we have matrices: A, B & C and we can write matrix D as follows:
D = 2A + 3B + CThen D is a linear combination of matrices A, B and C. This concept is important in linear algebra as it allows us to express one matrix in terms of others. Making it easier to manipulate & analyze the matrices. A matrix is a linear combination of other matrices if it can be expressed as a weighted sum of those matrices where the weights are scalar values.
Learn more about linear combination brainly.com/question/8881662
#SPJ4
Anthony decided to buy two DVDs that were not included in the deal. His price before tax was $19.99. What was the cost of the two DVDs after the 8.25% tax was added?
Answers
Given:
Cost of 2 DVDs before tax = $19.99
Tax rate = 8.25%
To find:
The cost of 2 DVDs after the tax was added.
Solution:
We have,
Cost of 2 DVDs before tax = $19.99
Tax rate = 8.25%
So,
Tax = 8.25% of Cost of 2 DVDs before tax
\(Tax=\dfrac{8.25}{100}\times 19.99\)
\(Tax=1.649175\)
\(Tax\approx 1.65\)
Now,
Cost of 2 DVDs after tax = Cost of 2 DVDs before tax + Tax
= $19.99 + $1.65
= $21.64
Therefore, the cost of 2 DVDs after the tax is $21.64.
GIVING BRAINLIST
Which set of ordered pair represents a function?
A. { (1,2), (2,3), (3,4), (4,5) }
B.{ (-5, 2), (-6,5), (-7, 9), (-8, 10), (-5, 11) }
C.{ (1,3), (2,4), (5,6), (2,8) }
D.{ (4,9), (5,8), (8,6), (4,7) }
Answers
Answer:
a
Step-by-step explanation:
So the only right answer is A!
Analyze the proportion below and complete the instructions that follow. Use a model to find the missing value in the proportion. A. 4 B. 5 C. 10 D. 22 Please select the best answer from the choices provided A B C D
Answers
Step-by-step explanation:
The area of a rectangle is 1,872 ft2. The ratio of the length to the width is 9:13. Find the perimeter of the rectangle.
176 ft
You want to make a scale drawing of your bedroom to help arrange your furniture. You decide on a scale of 3 in. = 2 ft. Your bedroom is a 12 ft by 14 ft rectangle. What should the dimensions of your drawing be?
18 in. by 21 in.
If 5/y + 7/x=24 and 12/y + 2/x=24, find the ratio of x to y.
5/7
Simplify the ratio 8ft/12in. Use the conversion 12 in. = 1 ft.
8/1
Analyze the proportion below and complete the instructions that follow.
2x+5/3 = x-5/4
-7
If a+b/2a-b = 5/4 and b/a+9 = 5/9, find the value of b.
30
Analyze the ratio below and complete the instructions that follow.
$30:$6
Simplify the ratio.
5:1
If 14/3 = x/y then 14/x =
3/y
Analyze the diagram below and complete the instructions that follow.
In the diagram, AB:BC is 3:4 and AC = 42. Find BC.
24
Analyze the diagram below and complete the instructions that follow.
If AB:BC is 3:11, solve for x.
9
If a, b, c, and d are four different numbers and the proportion a/b = c/d is true, which of the following is false?
b/a = c/d
Analyze the diagram below and complete the instructions that follow.
Find the ratio of the width to the length of the rectangle, then simplify the ratio. Use the conversion 100 cm = 1 m.
3/4
Simplify the ratio 3 gal./24 qt. Use the conversion 4 qt = 1 gal.
1/2
The area of a rectangle is 4,320 ft2. The ratio of the length to the width is 6:5. Find the length of the rectangle.
72 ft
Analyze the diagram below and complete the instructions that follow.
Given that CB/CA = DE/DF, find BA.
10.5
Analyze the proportion below and complete the instructions that follow.
2/3 = 8/x
3, 8
Analyze the diagram below and complete the instructions that follow.
Are the polygons shown here similar? Justify your answer. The images are not drawn to scale.
Yes, PQR ~TSV with a scale factor of 1:√3
All __________ are similar.
squares
Analyze the diagram below and complete the instructions that follow.
Determine which 2 triangles are similar to each other. The images are not drawn to scale.
GHI ~ JKL
Analyze the diagram below and complete the instructions that follow.
Pentagon PQRST ~ pentagon XYZVW. Find the value of b. The images are not drawn to scale.
3
Analyze the diagram below and complete the instructions that follow.
If ABC ~ XYZ, find XY. The images are not drawn to scale.
24
ABC is a right triangle. The legs of ABC are 9 ft and 12 ft. The shortest side of XYZ is 13.5 ft, and ABC ~ XYZ How long is the hypotenuse of XYZ?
22.5 ft
5/7 multiply by 8/9 then simplest form
Answers
Answer:
0.6 or 40/1
Step-by-step explanation:
Oki 5/7 x 8/9 = 40/63 = 0.63 Do you mean like this way or the other ?
5/7 x 9 = 45/63 and 8/9 x 7 = 56/63 x 45\63 = 2520\63 = 40/1
Hope that this will help you.
1. demand can be said to control supply and demand.
(Consumer, Producer)
Answers
Answer:
The law of demand says that at higher prices, buyers will demand less of an economic good. The law of supply says that at higher prices, sellers will supply more of an economic good. These two laws interact to determine the actual market prices and volume of goods that are traded on a market.
Supply is generally considered to slope upward: as the price rises, suppliers are willing to produce more. Demand is generally considered to slope downward: at higher prices, consumers buy less. ... The relationship between the supply and demand for a good (or service) and changes in price is called elasticity.
The cost of renting a bike at Centre Island in Toronto is represented by the equation C = 2n + 10, where C is the cost of renting a bike and n is the number of hours of bike rental. [A4]
a) How long did you rent the bike for if the cost is $30? ✓✓
b) Rearrange C = 2n + 10 to isolate n. ✓✓
Answers
Answer:
A) 10
B) (C+10)/2 = n
Step-by-step explanation:
A) We know that the total cost is represented by the variable C. So, basically the problem is saying that the variable C is equal to 30. If we plug that into the equation, we get 30 = 2n + 10. Solving this, we get n = 10.
B) Isolating a variable means to only have the specified variable on one side and have all other integers and variable on the other side. So, the final answer would be (C+10)/2 = n.
C- cost
n- number of hrs
C=30$
n=?
2n+10=30
2n=30-10
2n=20
n=20/2
n=10 hrs
You rented the bike for 10 hrs.
Someone please helppp

Answers
Answer:
k = 5/6
Step-by-step explanation:
First, we can make this have the form of a quadratic function, or ax²+bx+c=0. To do this, we can first subtract 10x from both sides to get
(2k+1)x²-8x=-6
Next, we can add 6 to both sides, resulting in
(2k+1)x²-8x+6 = 0
For a quadratic function of form ax²+bx+c=0, we can see that a=2k+1, b=-8, and c=6. We can then apply the quadratic equation, or
x= (-b ± √(b²-4ac))/(2a) to get our roots to be
x= (8 ± √(64-4(6)(2k+1)))/(2*(2k+1))
= (8 ± √(64-(48k+24)))/(4k+2)
= (8 ± √(40-48k))/(4k+2)
For the roots to be equal, we must have the two roots equal to each other. We can write this as
(8 + √(40-48k))/(4k+2) = (8 - √(40-48k))/(4k+2)
multiply both sides by (4k+2) to remove the denominator
8+√(40-48k) = 8 - √(40-48k)
subtract 8 from both sides to isolate the square roots
√(40-48k) = - √(40-48k)
The only number that is equal to its negative self (and is real) is 0. Therefore, √(40-48k) = - √(40-48k) = 0, so we have
√(40-48k) = 0
square both sides to remove the square root
40-48k = 0
add 48k to both sides to isolate the k and its coefficient
40 = 48k
divide both sides by 48 to isolate k
k = 40/48 = 5/6
if 600 cubic centimeters of water flow into the container in one minute, about how many minutes will it take to fill the container?
Answers
a. The volume of the spherical container is approximately 37,785.41 cubic centimeters.
b. It will take approximately 62.98 minutes to fill the container with a flow rate of 600 cubic centimeters per minute.
a. To find the volume of the spherical container, we can use the formula:
V = (4/3)πr^3
where V is the volume and r is the radius of the sphere. However, we need to first find the radius of the sphere using the given surface area.
The surface area of a sphere is given by the formula:
A = 4πr^2
Given that the surface area is approximately 5,538.96 square centimeters, we can equate the formula for surface area to the given value and solve for r:
4πr^2 = 5,538.96
r^2 = 5,538.96 / (4π)
r^2 ≈ 441.57
Taking the square root of both sides, we get:
r ≈ √441.57 ≈ 21.02
Now that we have the radius, we can substitute it into the formula for volume to find the volume of the container:
V = (4/3)π(21.02)^3 ≈ 37,785.41 cubic centimeters
Therefore, the volume of the container is approximately 37,785.41 cubic centimeters.
b. If 600 cubic centimeters of water flow into the container in one minute, we can calculate the time it takes to fill the container by dividing the volume of the container by the flow rate:
Time = Volume / Flow rate
Time ≈ 37,785.41 / 600 ≈ 62.98 minutes
Therefore, it will take approximately 62.98 minutes to fill the container.
The correct question should be :
A spherical container has a surface area of about 5,538.96 square centimeters.
a. What is the volume of the container?
b. If 600 cubic centimeters of water flow into the container in one minute, about how many minutes will it take to fill the container?
To learn more about volume visit : https://brainly.com/question/463363
#SPJ11
Use the image above to solve .What is the slope? How much would 12 pieces of art cost? Use y=mx+b to solve.

Answers
Answer:510
Step-by-step explanation: