Expand and simplify:
3(2p - 5) + 2(p + 3)
Answers
Answer:
8p - 9
Step-by-step explanation:
→ Expand brackets
6p - 15 + 2p + 6
→ Collect like terms
8p - 9
Related Questions
1.
(04.02 LC)
Which of the following is a common characteristic of a binomial distribution? (4 points)
There are more than two possible outcomes.
The probability of success is the same in all trials.
There are infinitely many observations.
You should perform x trials until you observe a success.
Each trial is dependent on the previous trial.
Answer: The probability of success is the same in all trials.
Answers
The probability of success, p, is the same for each trial. Each outcome is either a success (P) or a failure (Q). The correct option is A.
What is the independent probability?Independence is a fundamental notion in probability theory, as in statistics and the theory of stochastic processes.
You should perform x trials until you observe a success.
We have given that,
There are exactly two possible outcomes success and failure.
We have to determine the following is NOT a common characteristic of a binomial distribution.
By process of elimination:-
A binomial experiment is a statistical experiment that must meet certain requirements
All trials are independent.
There are a fixed number of n trials.
The probability of success, p, is the same for each trial.
To learn more about the probability visit:
brainly.com/question/24756209
#SPJ1
n Exercises 7–12, find ||u || for the given exercise, and give a unit vector in the direction of u.
Answers
To find the magnitude (|u|) and unit vector in the direction of a vector u, you can use the following formula:
|u| = √ux2 + uy2 + uz2
Where ux, uy, and uz are the components of the vector.
Then, the unit vector in the direction of u is given by:
uhat = (ux/|u|, uy/|u|, uz/|u|).
Therefore, for exercises 7-12, find the magnitude (|u|) of the given vector, then divide each component of the vector by the magnitude to obtain the unit vector.
For example, if u = (3, 4, -5), then |u|= √32 + 42 + (-5)2 = √74 = 8.66.
The unit vector in the direction of u would then be (3/8.66, 4/8.66, -5/8.66).
To know more about unit vector refer here:
https://brainly.com/question/30279109
#SPJ11
Find five rational numbers between -1/2 and 4/7
Answers
The rational numbers from the given numbers are -5/7, -6/7, 1/7, 2/7,3/7.
According to the statement
we have to find that the rational numbers.
So, For this purpose, we know that the
Rational number, a number that can be represented as the quotient p/q of two integers such that q ≠ 0.
From the given information:
The given numbers are between -1/2 and 4/7
Then to find the numbers then
Rational numbers = (-1/2 +4/7) /2
Rational numbers = (-1 +2/7)
Rational numbers = -5/7.
And then the number becomes -6/7, 2/7,3/7.
Now, The rational numbers from the given numbers are -5/7, -6/7, 1/7, 2/7,3/7.
So, The rational numbers from the given numbers are -5/7, -6/7, 1/7, 2/7,3/7.
Learn more about rational numbers here
https://brainly.com/question/12088221
#SPJ9
What are the coordinates of B

Answers
Answer:
The coordinates of B is (-5, 4).
the distance from a point to the waterfall is 1000 yards. the angle from the ground to the top of the waterfall is 17. what is the height of the waterfall?
Answers
The height of the waterfall is approximately 309 yards.
To find the height of the waterfall, we can use trigonometry. The given information states that the distance from the point to the waterfall is 1000 yards, and the angle from the ground to the top of the waterfall is 17 degrees.
We can use the tangent function, which relates the height (opposite side) to the distance (adjacent side) and the angle:
tan(angle) = height / distance
Substituting the given values:
tan(17 degrees) = height / 1000 yards
To isolate the height, we can multiply both sides of the equation by 1000:
1000 * tan(17 degrees) = height
Using a calculator, we can calculate the value of the tangent of 17 degrees:
tan(17 degrees) ≈ 0.309
Substituting this value:
1000 * 0.309 = height
Therefore, the height of the waterfall is approximately 309 yards.
To learn more about trigonometry visit:
brainly.com/question/12068045
#SPJ11
Solve the matrix equation for the unknown matrix X. (If not possible, enter IMPOSSIBLE in any cell of the matrix.) A = [7 3 4 3] B = [7 2 7 2] C = [3 3 4 0 0 8] D = [10 30 30 30 30 0] 5(X - C) = D x = []
Answers
After solving the equation 5(X - C) = D , for the given matrices , we get , the unknown matrix X is = \(\left[\begin{array}{ccc}5&9\\10&6\\6&8\end{array}\right]\) .
In the question ,
it is given that , the matrices are
A = \(\left[\begin{array}{ccc}7&3\\4&3\end{array}\right]\) B = \(\left[\begin{array}{ccc}7&2\\7&2\end{array}\right]\) C = \(\left[\begin{array}{ccc}3&3\\4&0\\0&8\end{array}\right]\) and D = \(\left[\begin{array}{ccc}10&30\\30&30\\30&0\end{array}\right]\) .
and the equation is given as 5(X - C) = D ,
and we have to find the value of unknown matrix X .
let the matrix X be = \(\left[\begin{array}{ccc}a&b\\c&d\\e&f\end{array}\right]\)
Substituting , the given matrices A , B , C and D in the equation 5(X - C) = D ,
we get ,
5(X - C) = D ,
5X - 5C = D ,
5\(\left[\begin{array}{ccc}a&b\\c&d\\e&f\end{array}\right]\) - 5\(\left[\begin{array}{ccc}3&3\\4&0\\0&8\end{array}\right]\) = \(\left[\begin{array}{ccc}10&30\\30&30\\30&0\end{array}\right]\)
5\(\left[\begin{array}{ccc}a&b\\c&d\\e&f\end{array}\right]\) = \(\left[\begin{array}{ccc}10&30\\30&30\\30&0\end{array}\right]\) - \(\left[\begin{array}{ccc}15&15\\20&0\\0&40\end{array}\right]\)
Simplifying further ,
we get ,
5\(\left[\begin{array}{ccc}a&b\\c&d\\e&f\end{array}\right]\) = \(\left[\begin{array}{ccc}25&45\\50&30\\30&40\end{array}\right]\)
Dividing both sides by 5 ,
we get ,
X = \(\left[\begin{array}{ccc}a&b\\c&d\\e&f\end{array}\right]\) = \(\left[\begin{array}{ccc}5&9\\10&6\\6&8\end{array}\right]\)
Therefore , the matrix X is = \(\left[\begin{array}{ccc}5&9\\10&6\\6&8\end{array}\right]\) .
The given question is incomplete , the complete question is
Solve the matrix equation for the unknown matrix X.
A = \(\left[\begin{array}{ccc}7&3\\4&3\end{array}\right]\) B = \(\left[\begin{array}{ccc}7&2\\7&2\end{array}\right]\) C = \(\left[\begin{array}{ccc}3&3\\4&0\\0&8\end{array}\right]\) and D = \(\left[\begin{array}{ccc}10&30\\30&30\\30&0\end{array}\right]\) . the equation is
5(X - C) = D .
Learn more about Matrix here
https://brainly.com/question/7172481
#SPJ4
heeeeeeeeeeeeeeeeeeeeeeeeeeeeeeeeeeeeeeeeeeeeeeeeelp thx in advance :)

Answers
Answer:
After multiplying, we get two Pythagorean triples (sorry for not writing it all out, it's a really long process). With those, we can simplify down to 289/100
What is the answer?!!

Answers
Answer:
12.
Step-by-step explanation:
How tall is this table?

Answers
Answer:
Table: 105 cm
Step-by-step explanation:
"m" = mouse
"c" = cat
"t" = table
equations:
t + c - m = 120
t - c + m = 90
___________
2t = 210
t = 210/2 = 105
Hope this helps
Your school roof is made of four trapezoids. Two of them have the bases of 14 m and 6 m respectively with the 8 m height. The remaining two have the bases of 12 m and 10 m with 6m height. How much roof material is needed to cover the roof?
Answers
Answer:
292 m² of roof material is needed to cover the roof.
Step-by-step explanation:
In order to find how much roof material is needed to cover the roof, we must find the total amount of the area of the roof.
Because the roof is made of 4 trapezoids, we must use the formula:
Area= [(A+B)/2] × H
A and B represents the two parallel lines while H represents the height.
In order to solve the equation, you must use the Order of Operations, PEMDAS.
P= Parenthesis
E= Exponents and roots
M= Multiplication
D= Division
A= Addition
S= Subtraction
(If there is a parenthesis inside a parenthesis. Start by simplifying the parenthesis in the inside).
So:
A.The first set of two trapezoids
Area = [(14 + 6)/2] × 8
= [(20)/2] × 8
= 10 × 8
= 80 m²
One trapezoid is equal to 80 m². In order to find the total amount of both trapezoids, multiply the amount by 2.
So: 80 × 2= 160 m²
The total amount of both trapezoids is 160 m².
B.The second set of two trapezoids
Area= [(12 + 10)/2] × 6
= [(22)/2] × 6
= 11 × 6
= 66 m²
One trapezoid is equal to 66 m². In order to find the total amount of both trapezoids, multiply the amount by 2.
So: 66 × 2= 132 m²
The total amount of both trapezoids is 132 m².
Last step is to add both amounts in order to know the total amount needed of roof material that covers the roof.
So: 160 + 132= 292 m²
So the amount of roof material needed to cover the roof is 292 m².
I hope this helps! I'm sorry if its wrong or too complicated.
What is the domain of the relation?
ty
4
2
-4<=x<=4
-4
-4<=X<=6
4
-4
3 of 11
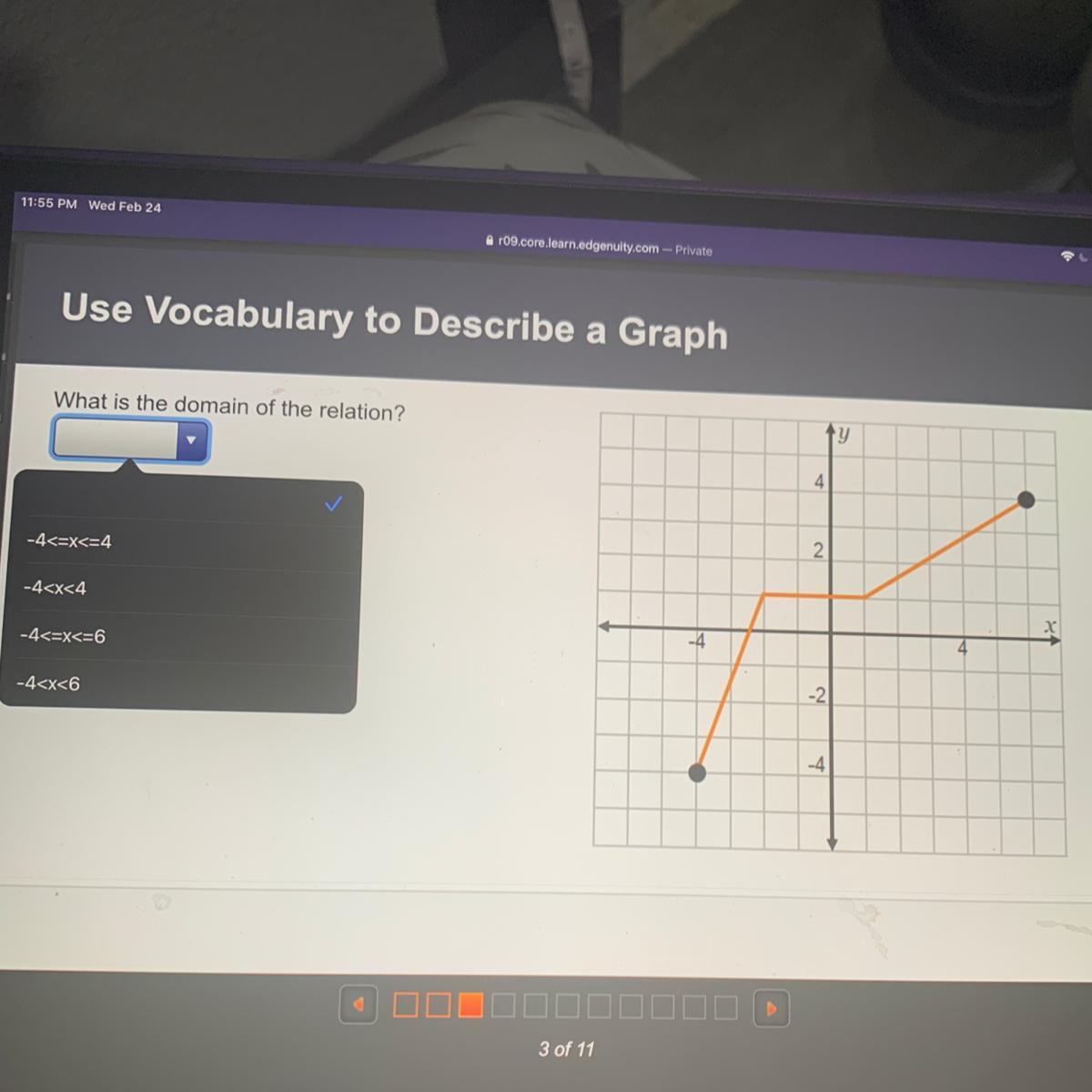
Answers
Answer:
-4 ≤ x ≤ 6
Step-by-step explanation:
When we talk of the domain, we are referring to the possible x-values
from the diagram, we can see that we have the x-values at -4 and 6
The dotted line means that -4 and 6 are in the domain
Thus, the two points represent the end and starting point of the domain
Writing this in interval notation, we have the representation as;
-4 ≤ x ≤ 6
PLS HELP WILL MARK YOU BRAINLIEST! NO FAKE ANSWERS PLEASEEE
GRAPHING
SHOW SOLUTION ON HOW TO SOLVE X AND Y INTERCEPTS OF NUMBER 1 AND 2 AND GRAPH

Answers
Answer:
This may not be correct because it is generated by system. (High chance for it to be very accurate machine these days don't make mistake).
The one that contains horizontal line is question 2.
Please tell me if there is anything not going along.




Answer:
6x-3y+12=0
X-INTERCEPTS
Plug y=0 into the equation and solve the resulting equation 6x+12=0 for x.
The x-intercept: (−2,0)
Y-INTERCEPTS
Plug x=0 into the equation and solve the resulting equation 12−3y=0 for y.
The y-intercept: (0,4)
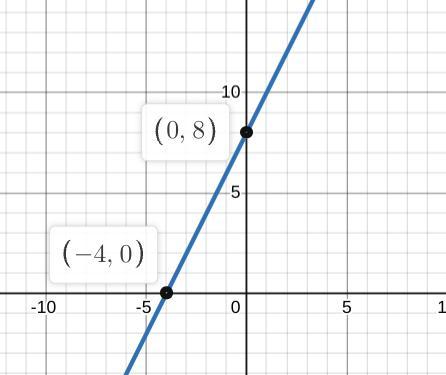
2x-y+8=0
X-INTERCEPTS
Plug y=0 into the equation and solve the resulting equation 2x+8=0 for x.
The x-intercept: (−4,0)
Y-INTERCEPTS
Plug x=0 into the equation and solve the resulting equation 8−y=0 for y.
The y-intercept: (0,8)
X+y=2
X-INTERCEPTS
Plug y=0 into the equation and solve the resulting equation x=2 for x.
The x-intercept:(2,0)
Y-INTERCEPTS
Plug x=0 into the equation and solve the resulting equation y=2 for y.
The y-intercept: (0,2)
2x-2y=4
X-INTERCEPTS
Plug y=0 into the equation and solve the resulting equation 2x=4 for x.
The x-intercept: (2,0)
Y-INTERCEPTS
Plug x=0 into the equation and solve the resulting equation −2y=4 for y.
The y-intercept: (0,−2)



What is the domain of the function

Answers
We get domain from X-axis:
0 ≤ x < ∞5/6 divided by 1/3
o 6/18
o 10/6
o 10/18
o 5/18
Answers
Answer:
5/6 divided by 1/3 = 15/6
Step-by-step explanation:
(5/6)/(1/3) = (5/6)(3/1) = 15/6
Now, (5/6)x(1/3) = 5/18
Find the slope of the tangent line to the given polar curve at the point specified by the value of \( \theta \). \[ r=\cos (\theta / 3), \quad \theta=\pi \]
Answers
The derivative of \(r\) with respect to \(\theta\) can be found using the chain rule. Let's proceed with the differentiation:
\frac{dr}{d\theta} = \frac{d}{d\theta}\left(\cos\left(\frac{\theta}{3}\right)\right)
To differentiate \(\cos\left(\frac{\theta}{3}\right)\), we treat \(\frac{\theta}{3}\) as the inner function and differentiate it using the chain rule. The derivative of \(\cos(u)\) with respect to \(u\) is \(-\sin(u)\), and the derivative of \(\frac{\theta}{3}\) with respect to \(\theta\) is \(\frac{1}{3}\). Applying the chain rule, we have:
\frac{dr}{d\theta} = -\sin\left(\frac{\theta}{3}\right) \cdot \frac{1}{3}
Now, let's evaluate this derivative at \(\theta = \pi\):
\frac{dr}{d\theta} \bigg|_{\theta=\pi} = -\sin\left(\frac{\pi}{3}\right) \cdot \frac{1}{3}
The value of \(\sin\left(\frac{\pi}{3}\right)\) is \(\frac{\sqrt{3}}{2}\), so substituting this value, we have:
\frac{dr}{d\theta} \bigg|_{\theta=\pi} = -\frac{\sqrt{3}}{2} \cdot \frac{1}{3} = -\frac{\sqrt{3}}{6}
Therefore, the slope of the tangent line to the polar curve \(r = \cos(\theta / 3)\) at the point specified by \(\theta = \pi\) is \(-\frac{\sqrt{3}}{6}.
Learn more about Tangent Line here :
https://brainly.com/question/31179315
#SPJ11
At a wedding there were 40 people from gromms side and 56 people from bride's family at the wedding
find the ratio of the groom's family to the bride's family at the wedding
Answers
Answer:
5 : 7
Step-by-step explanation:
groom's side: 40
bride's side: 56
ratio groom to bride = 40/56 = 20/28 = 10/14 = 5/7
Answer: 5 : 7
Explanation- 50+56 Is 106. So 106- 10=96.
There is your answer 96.
Given that events A and B are independent with P(A) = 0.68 and
P(A and B) = 0.544, determine the value of P(B), rounding to the nearest
thousandth, if necessary.
Answers
If events A and B are independent with P(A) = 0.68 and P(A and B) = 0.544 then the value of P(B) is 0.8
We know that if events A and B are independent, then:
P(A and B) = P(A) × P(B)
We are given P(A) = 0.68 and P(A and B) = 0.544.
Substituting these values into the equation above, we get:
0.544 = 0.68 × P(B)
Solving for P(B), we get:
P(B) = 0.544 / 0.68 = 0.8
P(B) = 0.800
Hence, if events A and B are independent with P(A) = 0.68 and
P(A and B) = 0.544 then the value of P(B) is 0.8
To learn more on probability click:
https://brainly.com/question/11234923
#SPJ1
Create a table to show how the number of days is related to the number of hours. Show at least 5 days.
Answers
Answer:
Days Hours
1 24
2 48
3 72
4 96
5 120
6 144
Step-by-step explanation:
hope this helped:) just add lines and it will be a table
Jackie went to the movie theater where he bought drinks and popcorn bags for his group of friends. Each drink was $4 and a bag of popcorn was $6. He spent a total of $108. He bought a total of 22 items combined. Write a system of equations that could be used to determine the number of drinks and popcorn bags that Jackie bought at the movie theatre. Define the variables used.
Answers
Answer:
x-intercept is 8, meaning if you get 8 candy bars, you can get 0 popcorn bags.
y-intercept is 6, meaning if you get 6 popcorn bags, you can get 0 candy bars. When Jackie had 0 candy bars, she had 6 (y-intercept) bags of popcorn and when Jackie had 0 bags of popcorn, she had 8 (x-intercept) bars of candy.
Step-by-step explanation:
The y-axis represents the amount of popcorn bags she has and the x-axis has the amount of candy bars. When the linear line intercepts with either axis, at least one axis is always at zero.
4 times 67 please only serious awnsersss
Answers
Answer:
268 hope i helped
Step-by-step explanation:
Answer:
268!
Step-by-step explanation:
You can easily g0ogle this so you don't need to waste points!
Work out the area of the garden. HELP ASAP i’ll make u brainliest ♡︎

Answers
Answer: 120m2
Step-by-step explanation:8x11=88
19-11=8- length of triangle base. 1/2 base x height= 4x8=32
32+88=120m2
if two polygons are similar the corresponding angles must be
Answers
If two polygons are similar the corresponding angles between the two polygons must be congruent (have equal measures).
If two polygons are similar, it means that they have the same shape but possibly different sizes. In this case, the corresponding angles between the two polygons must be congruent (have equal measures).
More formally, if two polygons are similar, then their corresponding angles are equal. This property holds true for all corresponding angles of similar polygons, regardless of the number of sides or the size of the polygons.
It is a fundamental property of similarity in geometry.
Therefore, if you have two similar polygons, you can conclude that the corresponding angles of those polygons will be congruent.
To know more about polygons
https://brainly.com/question/12622435
#SPJ11
to create
MNO was dilated, then_____,YHQ.
O rotated
O reflected
O translated
O dilated
Answers
Answer:
O rotated
Step-by-step explanation:
I dont know what to do please help

Answers
Answer:
V = π(8^2)(31) = 6,232.9 m^3
which row of pascal’s triangle would you use to expand (2x 10y)15? row 10 row 12 row 15 row 25 how many terms are in this expansion? 3 terms
Answers
15th row of the Pascal's Triangle is used to expand \((2x+10y)^{15}\). There will be 16 terms in the expansion.
Pascal's Triangle is used to find the co-efficient for binomial expansions.
The nth row of the Pascal's Triangle gives the coefficients in the expansion of binomial expressions of degree n. For example, in the expansion of \((x+y)^2\) , the binomial coefficients are 1,2,1 which is given in the 2nd row of Pascal's Triangle. Also the expansion has three terms, therefore. The Pascal triangle starts with 0th row consisting of only 1.
Here the given expression is \((2x+10y)^{15}\) of degree 15. So its binomial coefficient is given in the 15th row of Pascal's triangle.
The number of terms in the binomial expansion of degree n will be n+1 because the coefficients in nth row will n+1 in number.
Hence there will be 16 terms in the expansion of \((2x+10y)^{15}\).
Learn more about Pascal's Triangle at https://brainly.com/question/11995132
#SPJ4
Answer:
The person above helped, and below I've attached a ss with all of the parts of this question answered - Hope it helps!
Step-by-step explanation:
Brainliest would be very much appreciated :) - hope you have a good day!

2. The diagram shows a circle inside a rectangle. Work out the shaded region,
Give your answer to 3 significant figures.
8 cm
17 cm
32 cm

Answers
Answer:
342.938 cm²
Step-by-step explanation:
Area of shaded region = area of rectangle - area of circle
= (length*width) - (π*radius²)
= (32*17) - (π8²)
= 544 - 201.06192983
= 342.93807017 ≈ 342.938 cm²
What value of x is in the solution set of 3(x - 4) ≥ 5x + 2 ?
A. -10
B. .-5
C. 5
D. 10
PLS HELP!!
Answers
Answer:
x ≥ - 7
Step-by-step explanation:
3(x - 4) ≥ 5x + 2
3x - 12 ≥ 5x + 2
3x - 12 + 12 ≥ 5x + 2 + 12
3x - 5x ≥ 5x - 5x + 14
- 2x ≥ 14
- 2x ÷ - 2 ≥ 14 ÷ - 2
x ≥ - 7
If x 2 is added to x, the sum is 42. Which of the following could be the value of x?
Answers
Answer:
the x equals 14
Step-by-step explanation:
hope it helps
Enter the solutions from least to greatest. (x +1)(3x +4)=0 urgent i need help
Answers
Answer:
x = - \(\frac{4}{3}\), x = - 1
Step-by-step explanation:
Given
(x + 1)(3x + 4) = 0
Equate each factor to zero and solve for x
x + 1 = 0 ⇒ x = - 1
3x + 4 = 0 ⇒ 3x = - 4 ⇒ x = - \(\frac{4}{3}\)
Solutions from least to greatest are
x = - \(\frac{4}{3}\) , x = - 1
Two liters of water are poured into an empty vase shaped like a rectangular prism. The base area is 100 square centimeters. What is the height of the water? (1 L = 1000 cm³)
PLEASE HELP QUICK 50 POINTS!!!!!