Emily practices the piano 826 minutes in 2 weeks. Assuming she practices the same amount every week, how many minutes would she practice in 3 weeks?
Answers
The number of minutes Emily would practice the piano in three weeks will be 1,239 minutes, if she practices 826 minutes in 2 weeks assuming she practices the same amount every week by solving the function f(x)=2x=826
To determine the total number of minutes Emily would practice in three weeks, you can use the principle of ratio and proportions since Emily practices the same amount every week.
Here's how:
Let x be the number of minutes Emily practices per week.
Then, the number of minutes she practices in 2 weeks will be equal to 2x
Therefore, using the information given in the problem,
2x = 826
We can then solve for x: (solving function f(x))
2x = 826
Divide both sides by 2:
2x/2 = 826/2
x = 413
Now that we know Emily practices for 413 minutes every week, we can find the total number of minutes she would practice in three weeks by multiplying 413 by 3:
413 × 3 = 1239
Therefore, Emily would practice for 1,239 minutes in three weeks.
To know more about function refer here:
https://brainly.com/question/32599830
#SPJ11
Related Questions
Consider the following lottery: P=(1,p 1
;2,p 2
;3,p 3
) (a) Jack is an expected utility maximizer and his utility function is u(1)=1,u(2)=2,u(3)=3. In the probability (Marchak-Machina) triangle with p 1
on the horizontal and p 3
on the vertical axis, sketch a indifference curve for Jack. What is the slope of this curve? (b) Alice is an expected utility maximizer and her utility function is u(1)=1,u(2)=4,u(3)=6. Does Alice prefer receiving 2 for sure or a 50:50 gamble between 1 and 3 ? (c) In the same probability triangle sketch an indifference curve for Alice. What is the slope of this curve? (d) Bob is also an expected utility maximizer and his utility function is u(1)=9,u(2)=12,u(3)=18. Does Bob prefer receiving 2 for sure or a 50:50 gamble between 1 and 3 ? (e) In the same probability triangle sketch an indifference curve for Bob. What is the slope of this curve? (f) Infer a general principle from your findings in (a)-(e) above. Instead of the numbers for consequences and utilities, use general symbols x 1
for the monetary reward amounts, and u(x 1
),u(x 2
),u(x 3
) for their utilities. Find the equations for the curves of constant expected value and of constant expected utility. Find a condition involving the x=(x 1
,x 2
,x 3
) and the u under which the latter curves are steeper. Express this condition in a way that tells you something about this person's attitude toward risk.
Answers
(a) For Jack, with the utility function u(1) = 1, u(2) = 2, and u(3) = 3, the indifference curve represents combinations of probabilities (p1, p3) that yield the same utility level for Jack. Since Jack's utility increases with the outcome value, the indifference curve will be upward sloping.
To sketch the indifference curve for Jack, we connect the points (p1, p3) that yield the same utility level. The specific shape of the indifference curve depends on the utility function and the values of p1 and p3. However, since the utility values increase linearly, the indifference curve will be a straight line. The slope of this indifference curve can be calculated as the change in p3 divided by the change in p1. Since the utility function is linear, the slope will be constant. The slope of the indifference curve is given by (change in p3)/(change in p1) = (u(3) - u(1))/(u(2) - u(1)) = (3 - 1)/(2 - 1) = 2.
(b) For Alice, with the utility function u(1) = 1, u(2) = 4, and u(3) = 6, we can compare the expected utilities to determine her preference.
The expected utility of receiving 2 for sure is u(2) = 4.
The expected utility of a 50:50 gamble between 1 and 3 is (1/2)u(1) + (1/2)u(3) = (1/2)(1) + (1/2)(6) = 3.5.
Since the expected utility of receiving 2 for sure (4) is greater than the expected utility of the 50:50 gamble (3.5), Alice prefers receiving 2 for sure.
(c) To sketch the indifference curve for Alice, we connect the points (p1, p3) that yield the same utility level according to her utility function u(1) = 1, u(2) = 4, and u(3) = 6. Similar to Jack, the indifference curve will be upward sloping since Alice's utility increases with the outcome value.
The slope of this indifference curve can be calculated as (u(3) - u(1))/(u(2) - u(1)) = (6 - 1)/(4 - 1) = 5/3.
(d) For Bob, with the utility function u(1) = 9, u(2) = 12, and u(3) = 18, we can compare the expected utilities.
The expected utility of receiving 2 for sure is u(2) = 12.
The expected utility of a 50:50 gamble between 1 and 3 is (1/2)u(1) + (1/2)u(3) = (1/2)(9) + (1/2)(18) = 13.5.
Since the expected utility of the 50:50 gamble (13.5) is greater than the expected utility of receiving 2 for sure (12), Bob prefers the 50:50 gamble.
(e) To sketch the indifference curve for Bob, we connect the points (p1, p3) that yield the same utility level according to his utility function u(1) = 9, u(2) = 12, and u(3) = 18. Similar to Jack and Alice, the indifference curve will be upward sloping.
The slope of this indifference curve can be calculated as (u(3) - u
(1))/(u(2) - u(1)) = (18 - 9)/(12 - 9) = 3.
(f) The findings in parts (a) to (e) demonstrate that individuals' attitudes toward risk differ based on their utility functions. The slope of the indifference curve represents the marginal rate of substitution between the probabilities of different outcomes. Steeper indifference curves indicate a higher marginal rate of substitution and imply a higher aversion to risk.
In general, for a person with a utility function u(x1), u(x2), u(x3) and outcome values x=(x1, x2, x3), the equation for the curve of constant expected value is:
x1p1 + x2p2 + x3p3 = E
where E is the expected value.
The equation for the curve of constant expected utility is:
\(u(x1)p1 + u(x2)p2 + u(x3)p3 = U\)
where U is the constant expected utility level.
The condition for the indifference curve to be steeper, indicating higher risk aversion, is:
u''(x) > 0
This condition implies that the second derivative of the utility function with respect to the outcome values is positive, indicating diminishing marginal utility and higher risk aversion.
Please note that the equations and conditions provided are based on general principles and can be applied to utility functions and outcomes in various decision-making scenarios.
Learn more about utility function
https://brainly.com/question/30652436
#SPJ11
\(Find \: H.C.F \: of \: \\ 900 \: , 270 \\ using \: e.d.l \: \\ ( \: Euclid's \: division \: lemma \: )\)
H.C.F of 900 & 270
Answers
Answer:
90Step-by-step explanation:
Factorize both numbers:
900 = 2*2*3*3*5*5270 = 2*3*3*3*5Common factors are:
2*3*3*5So HCF is:
HCF(900, 270) = 2*3*3*5 = 90\(\Large \red \mid \: \underline {\rm {{{\color{blue}{Explanation...}}}}} \: \red \mid\)
We know that ,As per Euclid Division Algorithm.
\( \longrightarrow \: \Large\underbrace {\rm {{{\color{red}{ \: a \: = \: bq \: + \: r \: }}}}} \)
a denotes dividendb denotes divisorq denotes quotientr denotes remainder◆━━━━━▣✦▣━━━━━◆Using Euclid Division Algorithm\(\large\bf{\purple{ \hookrightarrow \: }} \tt \: \: 900 \: = \: 270 \: \times \: 3 \: + \: 90\)
Here ,\(\large\bf{\orange{ \implies \: }} \: \:r \: \neq \: 0\)
Again Applying Euclid Division Algorithm
\(\large\bf{\purple{ \hookrightarrow \: }} \tt \: 270 \: = \: 90 \: \times \: 3 \: + \: 0\)
Here ,\(\large\bf{\orange{ \implies \: }} \: \:r \: = \: 0\)
As the reminder is 0 , 90 will be the greatest common divisor for the two given numbers.
So,\({\boxed{ \Large{ \blue{ \bf{ \underline{ HCF \: = \: 90}}}}}}\)
◆━━━━━▣✦▣━━━━━◆![[tex]Find \: H.C.F \: of \: \\ 900 \: , 270 \\ using \: e.d.l \: \\ ( \: Euclid's \: division \: lemma](https://i5t5.c14.e2-1.dev/h-images-qa/answers/attachments/Nu97J4pKyBnfU63KEjveqFBGeFyjCq1q.jpeg)
Which graph has a rate of change of zero?

Answers
Answer:
The horizontal line
Step-by-step explanation:
Answer:
The horizontal line has a rate of change of 0, since it has a slope of 0Step-by-step explanation:
On the other hand, the vertical line has an undefined slope.
Slope is rise/run or change n y over change in x.
If the y axis coordinate never changes, then rise = 0. The only number that changes is x. x changes 1 for every 0 y changes. 0/1 = 0.
In a vertical line, however, the x coordinate is the one that doesn't change. We reverse the number from before, but we have a problem 1/0 is an undefined number since you cannot divide by 0.
Answer is second graph shown
Find the image of (2,7) after a reflection over the x-axis
Answers
Answer:
(2, -7)
Step-by-step explanation:
The reflecting over the x axis changes the y coordinate to its opposite.
*Hope this helped!! Have a nice day! : )*
What is the slope of a perpendicular line to 3.2 + 4y = 9?
Answers
please help me I need

Answers
Answer
1
\((a + b)^{2} = {a}^{2} + 2ab + {b}^{2} \)
Therefore
Answer:
1
Step-by-step explanation:
SEE IMAGE FOR Solution ...

Geometry:
Classify the relationship between each pair of angles as alternate interior, alternate exterior, corresponding, or consecutive interior angles.
1. <6 and <3
2. <4 and <7
3. <5 and <4
Pls help me i have no idea what i'm doing:(
Answers
Answer:
Step-by-step explanation:
.<6 and <3
SOLUTION:
Interior angles that are non adjacent and lie on
opposite. In the figure, angles <5 and <4 are consecutive interior
angles. sides of the transversal are alternate interior
angles.
<4 and <7
SOLUTION:
In the figure, angles 1 and 3 are corresponding
angles. Use the Corresponding Angles Postulate:
If two parallel lines are cut by a transversal, then
each pair of corresponding angles is congruent
Combine the following expressions.

Answers
Answer:
11x²√2x
Step-by-step explanation:
Step 1: Simplify the square roots
\(\sqrt{18x^{5}} = 3x^{2}\sqrt{2x}\)
\(2\sqrt{32x^5} = 8x^{2} \sqrt{2x}\)
√18 = √9(√2)
√32 = √16(√2)
\(\sqrt{x^5} =\sqrt{x^4} \sqrt{x} = x^{2} \sqrt{x}\)
Step 2: Add them together
11x²√2x
Find the values of a and b that make the following piecewise defined function both continuous and differentiable everywhere. f(x) = 3x + 4, X<-3
2x2 + ax + b. X>-3
Answers
The values of a and b that make the piecewise defined function f(x) = 3x + 4, for x < -3, and f(x) = 2x^2 + ax + b, for x > -3, both continuous and differentiable everywhere are a = 6 and b = 9.
To ensure that the piecewise defined function is continuous at the point where x = -3, we need the left-hand limit and right-hand limit to be equal. The left-hand limit is given by the expression 3x + 4 as x approaches -3, which evaluates to 3(-3) + 4 = -5.
On the right-hand side of the function, when x > -3, we have the expression 2x^2 + ax + b. To find the value of a, we need the derivative of this expression to be continuous at x = -3. Taking the derivative, we get 4x + a. Evaluating it at x = -3, we have 4(-3) + a = -12 + a. To make this expression continuous, a must be equal to 6.
Next, we find the value of b by considering the right-hand limit of the piecewise function as x approaches -3. Substituting x = -3 into the expression 2x^2 + ax + b, we get 2(-3)^2 + 6(-3) + b = 18 - 18 + b = b. To make the function continuous, b must equal 9.
Therefore, the values of a and b that make the piecewise defined function both continuous and differentiable everywhere are a = 6 and b = 9.
Learn more about piecewise
brainly.com/question/28225662
#SPJ11
Please answer ASAP AND PLEASE DONT BE A SCAM What is the value of x?
97.4 mm
98 mm
101.2 mm
104 mm

Answers
The value of x in the given triangle ΔVKT is, 166 mm
What is Pythagoras theorem?It is the most important theorem of mathematics, which tells us the relationship between sides of the right angle triangle, which are known as Base(A), Height(B), Hypotenuse(H).
Pythagoras theorem,
H² = A² + B²
Given that.
A triangle ΔVKT,
It contains two more right angle triangles,
ΔYKT & ΔVYT
By using Pythagoras theorem we can calculate the value of YT and then Value of VY,
TK² = TY² + YK²
129.2² = TY² + 68²
TY = 109.85
Now by applying Pythagoras theorem in another triangle YVT
The value of VY = 93.9 ≈ 94 mm
Now the length of VK = x = 94 + 68
= 168 mm
To know more about Pythagoras theorem check:
https://brainly.com/question/343682
#SPJ1
Paul will pay for his new car in 36 monthly payments. If his car loan is for $20,538 then how much will Paul pay each month?
Answers
Answer:
570.50
Step-by-step explanation:
I think it's the correct answer maybe it is maybe it's not
Paul has acquired a car loan of amount $20,538 and is paying off the loan amount in 36 months.
This means that Paul will be paying $570.50 every month till the completion of 36 months.
The given parameters for the calculation of the monthly installment:
Loan amount= $20,538 Total months to repay the loan= 36 monthsCalculation of the monthly amount of Paul's loan:
\(\begin{aligned}\text{Monthly installment of loan}= \frac{\text{Total Amount of Loan Acquires}}{ \text{Total Time taken to Repay off the Loan}} \end{aligned}.\)
\(\begin{aligned}\text{Monthly Installment of Loan}= \frac{\$20,538}{36}\end{aligned}\)
\(\begin{aligned}\text{Monthly Installment of Loan}=\$570.50\end{aligned}\)
Therefore, the monthly installment of paul will be $57.50.
To know more about the calculation of installment, refer to the link below:
https://brainly.com/question/84351
SOMEONE please give me motivation for school--
DNENEF JEB i have Fs like WHyyywe NEED schOOL
Answers
Answer:
My friend, you have to look further than how stressful school presently is. I advice you to find something you love while you're young and devote your time to it. As a high school senior, I am super into computers and I practice game design as a hobby. you need to get something that drives you. also you gotta practice study especially if you wanna make it into college. Stay strong man
Answer:
I can't add anything beacuse of copyright
PLEASE HELP ME I BEG I WILL GIVE BRAINLIEST
Researchers fed mice a specific amount of Aldrin, a poisonous pesticide, and studied their nervous systems to find out why Aldrin causes convulsions. The absolute refractory period, time required for nerves to recover after a stimulus, was measured and varies Normally. The measurements, in milliseconds, for five mice were 2.1, 2.3, 2.3, 2.4, and 2.5.
Part A: Find the mean refractory period and the standard error of the mean. (2 points)
Part B: Calculate a 95% confidence interval for the mean absolute refractory period for all mice when subjected to the same treatment. (4 points)
Part C: Suppose the mean absolute refractory period for unpoisoned mice is known to be 2.1 milliseconds. Aldrin poisoning should slow nerve recovery and therefore increase this period. Do the data give good evidence to support this theory? What can you conclude from a hypothesis test? Justify your response with statistical reasoning. (4 points)
Answers
Answer:
Mean = (2.2 + 2.4 + 2.5 + 2.5 + 2.6 + 2.7)/6 = 2.48
Standard deviation = √(summation(x - mean)²/n
n = 6
Summation(x - mean)² = (2.2 - 2.48)^2 + (2.4 - 2.48)^2 + (2.5 - 2.48)^2 + (2.5 - 2.48)^2 + (2.6 - 2.48)^2 + (2.7 - 2.48)^2 = 0.1484
Standard deviation = √(0.1484/6
s = 0.16
Standard error = s/√n = 0.16/√6 = 0.065
Part B
Confidence interval is written as sample mean ± margin of error
Margin of error = z × s/√n
Since sample size is small and population standard deviation is unknown, z for 98% confidence level would be the t score from the student t distribution table. Degree of freedom = n - 1 = 6 - 1 = 5
Therefore, z = 3.365
Margin of error = 3.365 × 0.16/√6 = 0.22
Confidence interval is 2.48 ± 0.22
Part C
We would set up the hypothesis test. This is a test of a single population mean since we are dealing with mean
For the null hypothesis,
H0: µ = 2.3
For the alternative hypothesis,
H1: µ > 2.3
This is a right tailed test
Since the number of samples is small and no population standard deviation is given, the distribution is a student's t.
Since n = 6
Degrees of freedom, df = n - 1 = 6 - 1 = 5
t = (x - µ)/(s/√n)
Where
x = sample mean = 2.48
µ = population mean = 2.3
s = samples standard deviation = 0.16
t = (2.48 - 2.3)/(0.16/√6) = 2.76
We would determine the p value using the t test calculator. It becomes
p = 0.02
Assuming significance level, alpha = 0.05.
Since alpha, 0.05 > than the p value, 0.02, then we would reject the null hypothesis. Therefore, At a 5% level of significance, the sample data showed significant evidence that the mean absolute refractory period for all mice when subjected to the same treatment increased.
Step-by-step explanation:
. The balance sheet of Trinagar Company for the subsequent two years is provided to you: Liabilities Year I (Rs.) 50,000 70,000 80,000 50,000 250,000 Share capital. Debentures... Creditors..... Retained earning.. Year I (Rs.) i. ii. iii. iv. 100,000 50,000 40,000 60,000 250,000 Additional information for year II: Sales Rs. 180,000. Cost of goods sold Rs. 100,000. Cash operating expenses Rs. 50,000. Dividend paid Rs. 5,000. V. Interest paid Rs. 5,000. Required: Cash flow statement. Year II (Rs.) 250,000 100,000 80,000 430,000 Assets Fixed assets at cost ... Stock Debtors.. Cash balance. CROA Year II (Rs.) 230,000 100,000 40,000 60,000 430,000
Answers
To prepare the cash flow statement, we can use the indirect method, which involves adjusting the net income figure for non-cash items and changes in working capital to arrive at the net cash flow from operating activities. Then, we can account for cash flows from investing and financing activities to arrive at the net change in cash and cash equivalents.
Cash flow statement applying indirect methodHere's the cash flow statement for Year II:
Cash flow from operating activities:
Net income: Rs. 30,000 (180,000 - 100,000 - 50,000 - 5,000 - 5,000)
Adjustments for non-cash items:
Depreciation: Rs. 30,000 (230,000 - 200,000)
Changes in working capital:
Increase in stock: Rs. 10,000 (100,000 - 90,000)
Increase in debtors: Rs. 20,000 (60,000 - 40,000)
Increase in creditors: Rs. 10,000 (80,000 - 70,000)
Net cash flow from operating activities: Rs. 100,000
Cash flow from investing activities:
Purchase of fixed assets: Rs. 30,000 (230,000 - 200,000)
Net cash flow from investing activities: Rs. -30,000
Cash flow from financing activities:
Repayment of debentures: Rs. 20,000 (50,000 - 30,000)
Payment of dividends: Rs. 5,000
Net cash flow from financing activities: Rs. -25,000
Net change in cash and cash equivalents: Rs. 45,000 (100,000 - 30,000 - 25,000)
Cash and cash equivalents at the beginning of the year: Rs. 60,000
Cash and cash equivalents at the end of the year: Rs. 105,000
Learn more on cash flow statement here https://brainly.com/question/24179665
#SPJ1
The dotplot displays the total number of miles that the 28 residents of one street in a certain community traveled to work in one five-day workweek. Which of the following is closest to the percentile rank of a resident from this street who traveled 85 miles to work that week?
60
70
75
80
85
Answers
The required answer is the closest percentile rank of the resident from this street who traveled 85 miles to work that week is 75%.
Explanation:-
The dot plot displays the total number of miles that the 28 residents of one street in a certain community traveled to work in one five-day workweek. The percentile rank of a resident from this street who traveled 85 miles to work that week is 75% (approximately).How to find percentile rank? Percentile rank is used to show the percentage of scores that are lower than the given score. For example, if a score has a percentile rank of 80, it means that 80% of the scores are lower than that score. The formula to find the percentile rank of a given score is:
Percentile rank = (number of scores below given score / total number of scores) x 100%
Here, the given score is 85 miles traveled to work in a week, and the total number of scores is 28. to find the number of scores that are below 85 miles from the dot plot .
From the given dot plot, there are 21 scores below 85 miles. So, the percentile rank of the resident who traveled 85 miles to work is:
Percentile rank = (number of scores below given score / total number of scores) x 100%Percentile rank = (21 / 28) x 100%Percentile rank = 75% (approximately)
Therefore, the closest percentile rank of the resident from this street who traveled 85 miles to work that week is 75%.
To know about percentile rank . To click the link.
https://brainly.com/question/30782647.
#SPJ11
What is 115 lbs in kg?
Answers
Answer:
115 lbs = 52.16 kilograms
Step-by-step explanation:
115 pounds is a little over 52kg
PLEASE HELP!!! I NEED TO TURN THI IN SOON !!!
Question and instructions in the image below!!!
Will mark brainliest if correct!!!

Answers
Answer:
Im pretty sure its the 3rd one
Step-by-step explanation:
I did this a while ago
If f(x) = 3x -121, find the sum of values when f(x) = 4.
Write your answers for x as simplified fractions.
I
and x =
The sum of the values when f(x) = 4 is
Answers
The required value of the function x is 41.6
What is a function ?This relationship is typically represented as y = f(x), or "f of x," where y and x are coupled such that for each value of x, there is a specific value of y. This means that f(x) can only have one value for a given x. In set theory jargon, a function connects an element x to an element f(x) in another set.
Given : f (x ) = 3x - 121
to find ; value of f ( x ) when f (x) = 4
Since two values of f(x ) are given
we can simply equate these two values
and the answer which we will get is the final answer
thus we get
3x - 121 = 4
3x = 121 + 4
3x = 125
x = 125/ 3
x = 41.6
To know more about functions and values you may visit the link which is mentioned below:
https://brainly.com/question/2514827
#SPJ1
Is it AB C D I will will mark as brainly

Answers
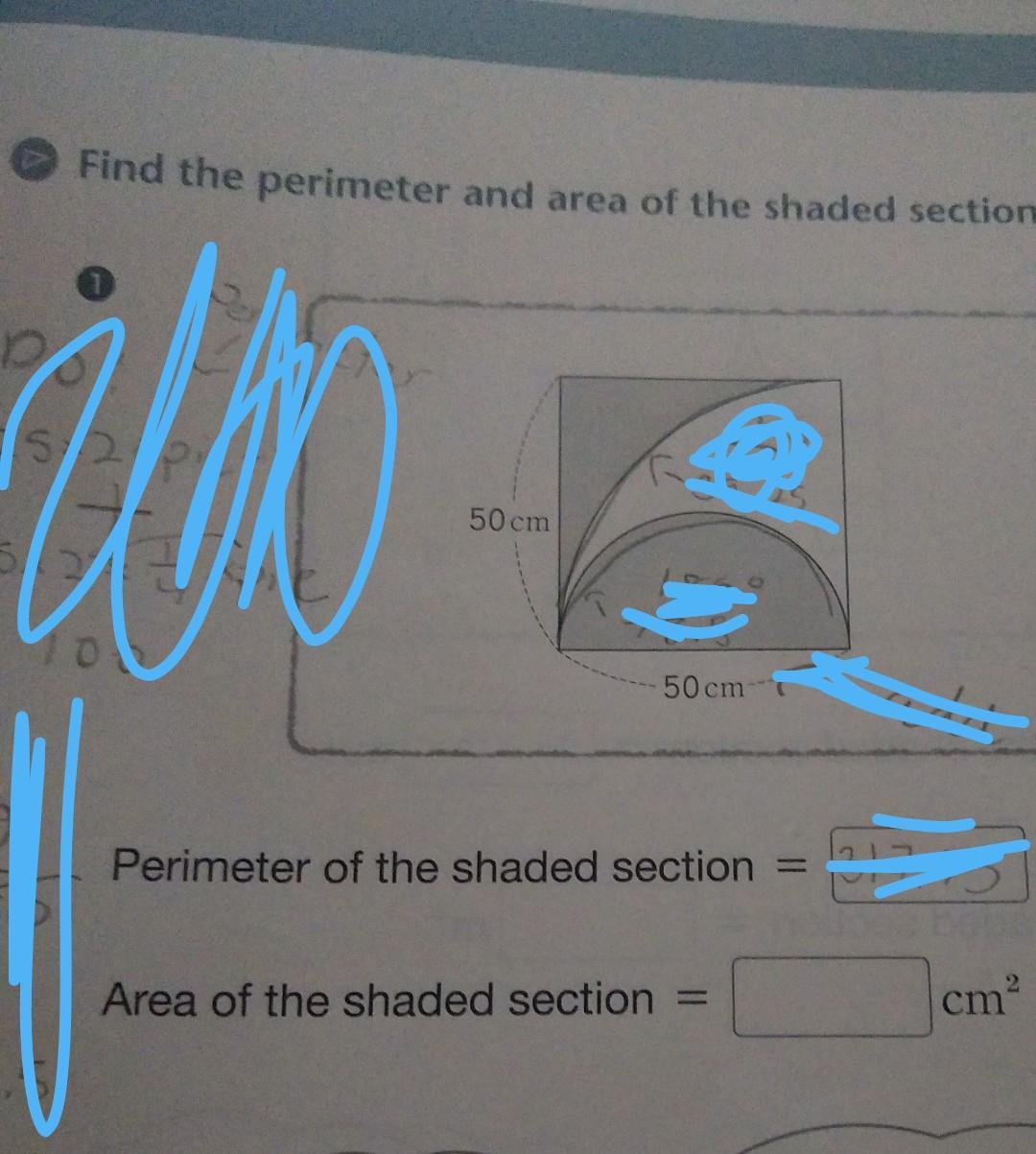
rly need help with this perimeter problem to find perimeter of shaded section! pls give full explanation. ignore notes
(will mark brainiest ☺️)
Answers
Find the volume of the prism.
A. 578.8 ft
B. 552.9 ft
C. 598.1 ft
D. 591.5 ft

Answers
Answer:
591.5 \(ft^{3}\)
Step-by-step explanation:
Volume = length x width x height
Length = 13 ft. Because it is the same size has the height
Width = 7 ft.
Height = 13 ft.
13ft x 13ft x 7ft = 1,183 ft
BUT, that can't be the answer because we're solving a prism
So divide the volume.
1,183 divided by 2 equals 591.5
ABCD and CFGH are parallelograms. Determine whether each statement is true or false.
True or False – ∠D≅∠G
True or False – AD¯¯¯¯¯¯¯¯≅BC¯¯¯¯¯¯¯¯
True or False – ∠A≅∠G
True or False – ∠B≅∠F
Wally modeled a window with FGHJ. For what values of x and y is FGHJ a parallelogram?
x=11, y=21
x=12, y=25
x=11, y=25
x=12, y=21
Answers
The correct values of x and y for FGHJ to be a parallelogram are x=11 and y=25.
Regarding the parallelograms:
True or False – ∠D≅∠G: True. In parallelograms, opposite angles are congruent, so ∠D and ∠G are congruent.
True or False – AD¯¯¯¯¯¯¯¯≅BC¯¯¯¯¯¯¯¯: False. In parallelograms, opposite sides are congruent, so AD¯¯¯¯¯¯¯¯ is not necessarily congruent to BC¯¯¯¯¯¯¯¯.
True or False – ∠A≅∠G: False. ∠A and ∠G are not necessarily congruent in parallelograms.
True or False – ∠B≅∠F: False. ∠B and ∠F are not necessarily congruent in parallelograms.
Regarding the window FGHJ:
To determine the values of x and y for FGHJ to be a parallelogram, we need opposite sides to be parallel and congruent.
Looking at the given options:
x=11, y=21: Not a parallelogram, as opposite sides are not parallel and congruent.
x=12, y=25: Not a parallelogram, as opposite sides are not parallel and congruent.
x=11, y=25: A possible parallelogram, as opposite sides FG and HJ are parallel and congruent.
x=12, y=21: Not a parallelogram, as opposite sides are not parallel and congruent.
Therefore, the correct values of x and y for FGHJ to be a parallelogram are x=11 and y=25.
For more questions on values
https://brainly.com/question/843074
#SPJ8
In which of the following situations can you approximate the sampling distribution with the given values of p and n by using a normal distribution? Op = 24 and n = 30 Op = .90 and n = 32 O More than one of the above Op = .08 and n = 50 O p = .70 and n=9
Answers
The situation where we can approximate the sampling distribution with a normal distribution is Op = .90 and n = 32.
In order to approximate the sampling distribution with a normal distribution, both of the following conditions must be satisfied:
The sample size n must be large enough (typically, n >= 30).
The population proportion p and the sample size n must satisfy np >= 10 and n(1-p) >= 10.
Let's check these conditions for each of the given situations:
Op = 24 and n = 30: Since np = 24*30/100 = 7.2 < 10 and n(1-p) = 22.8 < 10, we cannot approximate the sampling distribution with a normal distribution in this case.
Op = .90 and n = 32: Since np = 28.8 >= 10 and n(1-p) = 3.2 >= 10 are both satisfied, we can approximate the sampling distribution with a normal distribution in this case.
Op = .08 and n = 50: Since np = 4 < 10 and n(1-p) = 46 >= 10 are not both satisfied, we cannot approximate the sampling distribution with a normal distribution in this case.
Op = .70 and n=9: Since np = 6.3 >= 10 and n(1-p) = 2.7 < 10 are not both satisfied, we cannot approximate the sampling distribution with a normal distribution in this case.
Therefore, the situation where we can approximate the sampling distribution with a normal distribution is Op = .90 and n = 32.
To learn more about sampling visit:
https://brainly.com/question/13287171
#SPJ11
find an equation of the line tangent to the curve at the point corresponding to the given value of t. x=t^2-23, y=t^3 + t; t=5
Answers
The equation of the line tangent to the curve at the point corresponding to the given value of t.
x = t²-23 and y = t³ + t, at t = 5 is
38x - 5y + 574 = 0
Given, a curve with the points represented by
x = t²-23 and y = t³ + t, at t = 5
we have to find an equation of the line tangent to the curve at the given point on the curve.
so, the given point is (x , y) = (5² - 23 , 5³ + 5)
(x , y) = (2 , 130)
Now, the slope of the curve at that point be,
dy/dx = (3t² + 1)/(2t)
dy/dx = 76/10
Now, on using the slope-intercept form, we get
(y - 130)/(x - 2) = 38/5
5(y - 130) = 38(x - 2)
5y - 650 = 38x - 76
38x - 5y + 574 = 0
Hence, the equation of the line tangent to the curve at the point corresponding to the given value of t.
x = t²-23 and y = t³ + t, at t = 5 is
38x - 5y + 574 = 0
Learn more about Straight Lines here https://brainly.com/question/29264109
#SPJ4
a square of area is 36 cm squared is cut to make two triangles, A and B. The ratio of area A to area B is 2:1. Work out the dimensions of rectangles A and B.
Answers
Dimensions of:
Rectangle A are 6 cm by 4 cm.
Rectangles B are 6 cm by 2 cm.
Step-by-step explanation:
It is given that:
The area of square is 36 cm^2.
Hence, the side of the square is given as:
Area=(side)²
36=(side)²
side=6
Hence, the side of square is 6 cm.
Now, as the area of two rectangles A and B are in the ratio:
2:1
This means that, the area of rectangle A is 24 cm².
and area of rectangle B is 12 cm²
( Since, 36/(2+1)=36/3=12
Area of rectangle A=2×12=24 cm²
Area of rectangle B=1×12=12 cm². )
Hence, the dimensions of A are:
6 cm by 4 cm.
and the dimensions of rectangle B are:
6 cm by 2 cm.
a line segment is a part of a line that is bounded by two distinct end points and contains every point on the line between its endpoints. True/False?
Answers
The statement "a line segment is a part of a line that is bounded by two distinct end points and contains every point on the line between its endpoints." is True because a line segment is defined as a part of a line that is bounded by two distinct end points and contains all the points on the line between those endpoints.
A line segment is a part of a line that has two endpoints and connects them. It is the shortest distance between two points and has a definite length, but no width or height. A line segment can be part of a straight line or a curved line.
A line segment is a section of a line that is defined by two distinct end points and includes every point on the line between them. It is the basic building block of geometry and can be used to measure distances, angles, and shapes.
To learn more about line segment link is here
brainly.com/question/30072605
#SPJ4
PLEASE HELP 33 POINTS AND I WILL MARK BRAINLIEST!!!!!!!! THANKS!!!!!!!!!!!
Sarah has two similar regular pyramids with pentagon-shaped bases. The smaller has a scale factor of 2:3 when compared to the larger. Only the smaller pyramid is shown.
She calculates the area of the base of the pyramid (through long, hard work) to be 220.22 square units. The height of the pyramid is 9 units. Now she needs to calculate the volume of the pyramid.
(a) Calculate the volume of the pyramid for Sarah.
(b) "Oh, no!" Sarah exclaims. "Now I have to go through all this hard work again to find the volume of the larger pyramid!" Does she? Explain.
(c) Calculate the volume of the larger pyramid for Sarah.
Answers
(a) Volume of any Pyramid = \(\frac{1}{3} * (Base-Area)*height = \frac{1}{3} *220.22*9 = 660.66\) cubic units
(b) Sarah doesn't have to go through all the hard work as the smaller pyramid has a ratio of 2:3 to the bigger pyramid, so we get the equation, to help us solve it.
\(660.66 = \frac{2}{3} *(Area-of-larger-pyramid)\)
Hope that helps!
Step-by-step explanation:
(a) To calculate the volume of a pyramid, you can use the formula: V = (1/3) * B * h, where V is the volume, B is the area of the base, and h is the height of the pyramid.
In this case, Sarah has the area of the base (B) as 220.22 square units and the height (h) as 9 units. Let's substitute these values into the formula:
V = (1/3) * 220.22 * 9
V ≈ 660.66 square units
Therefore, the volume of the smaller pyramid is approximately 660.66 cubic units.
(b) No, Sarah doesn't need to go through the hard work again to find the volume of the larger pyramid. Since the two pyramids are similar, the ratio of their volumes will be equal to the cube of the ratio of their corresponding sides.
The scale factor of the smaller pyramid compared to the larger pyramid is 2:3. Since the scale factor is determined by the corresponding sides, it applies to both the base length and the height of the pyramid. Therefore, the volume of the smaller pyramid compared to the larger pyramid is (2/3)^3 = 8/27.
In other words, the volume of the smaller pyramid is 8/27 times the volume of the larger pyramid. So if Sarah already knows the volume of the smaller pyramid, she can simply multiply it by 27/8 to find the volume of the larger pyramid.
(c) To calculate the volume of the larger pyramid, we can use the ratio mentioned above:
Volume of the larger pyramid = (Volume of the smaller pyramid) * (27/8)
Volume of the larger pyramid ≈ 660.66 * (27/8)
Volume of the larger pyramid ≈ 2239.99 square units
Therefore, the volume of the larger pyramid for Sarah is approximately 2239.99 cubic units.
Please mark as y
the following stem-and-leaf plot shows scores on a statistics final exam. find the number of outliers. 2 00 3 468 4 357 5 01677 6 235 7 6899 8 233569999 9 01268 10 0
Answers
In the given stem-and-leaf plot, we can conclude that there are at least 5 outliers
The number of outliers in the given stem-and-leaf plot can be determined by identifying values that are significantly higher or lower than the majority of the data.
To find the number of outliers in the stem-and-leaf plot, we need to analyze the data distribution and identify values that deviate significantly from the rest of the scores.
Looking at the stem-and-leaf plot, we observe that the majority of the scores are concentrated between 20 and 90. The numbers 2, 3, 4, 5, 6, 7, 8, 9, and 10 represent the tens digit of the scores, while the leaves represent the ones digit.
Upon examining the plot, we notice that there are a few values that stand out from the rest. These values are 00, 01677, 6899, 233569999, and 01268. Outliers are typically defined as values that fall outside the "typical" range of the data, and these values appear to deviate significantly from the majority of the scores.
To determine the exact number of outliers, we need to apply specific criteria. One common method is to use the 1.5 × IQR (interquartile range) rule. The IQR is calculated as the difference between the third quartile (Q3) and the first quartile (Q1) of the data. Any values below Q1 - 1.5 × IQR or above Q3 + 1.5 × IQR are considered outliers.
In this case, we don't have the exact raw data to calculate the quartiles and IQR. However, based on visual inspection of the stem-and-leaf plot, we can still identify the values mentioned earlier as outliers due to their significant deviation from the majority of the scores.
Therefore, in the given stem-and-leaf plot, we can conclude that there are at least 5 outliers
Learn more about outliers here:
brainly.com/question/31174001
#SPJ11
What is a complex root of a polynomial?
Answers
The roots of a polynomial are the values of x for which the polynomial evaluates to 0.
A complex root is a root for which the real and imaginary parts are not both 0. If a polynomial has a complex root, that means that there is at least one value of x for which the polynomial evaluates to 0.
This can happen in two ways: either the polynomial has a real root and an imaginary root, or it has two complex roots. In either case, the complex roots must be found in order to determine the polynomial's factorization.
For more questions like Complex root click the link below:
https://brainly.com/question/17998260
#SPJ4
531
x 47
Long multiplication :) please help