Answers
Related Questions
Okay i need help! A novelist can write 1 7/8 pages in ¾ hour. At that rate, How much can she write in 1 hour?
Answers
Answer:
2 3/8
Step-by-step explanation:
a particle moves in a straight line with the given velocity ()=6cos() (in m/s).v(t)=6cos(t) (in m/s). find the displacement and distance traveled over the time interval [0,5].[0,5π].
Answers
The displacement and distance traveled depend on the given time interval.
To find the displacement, we need to integrate the velocity function from 0 to 5 or 0 to 5π, depending on the given time interval.
If the time interval is [0,5], we have:
Displacement = ∫[0,5] v(t) dt
Displacement = ∫[0,5] 6cos(t) dt
Displacement = [6sin(t)] from 0 to 5
Displacement = 6sin(5) - 6sin(0)
Displacement ≈ 2.785 meters
If the time interval is [0,5π], we have:
Displacement = ∫[0,5π] v(t) dt
Displacement = ∫[0,5π] 6cos(t) dt
Displacement = [6sin(t)] from 0 to 5π
Displacement = 0 - 6sin(0)
Displacement = 0 meters
To find the distance traveled, we need to integrate the absolute value of the velocity function over the given time interval.
If the time interval is [0,5], we have:
Distance = ∫[0,5] |v(t)| dt
Distance = ∫[0,5] |6cos(t)| dt
Distance = ∫[0,π/2] 6cos(t) dt + ∫[π/2,5] -6cos(t) dt
Distance = [6sin(t)] from 0 to π/2 + [-6sin(t)] from π/2 to 5
Distance = 6 - 6sin(5) ≈ 2.215 meters
If the time interval is [0,5π], we have:
Distance = ∫[0,5π] |v(t)| dt
Distance = ∫[0,5π] |6cos(t)| dt
Distance = ∫[0,π] 6cos(t) dt + ∫[π,2π] -6cos(t) dt + ∫[2π,3π] 6cos(t) dt + ∫[3π,4π] -6cos(t) dt + ∫[4π,5π] 6cos(t) dt
Distance = [6sin(t)] from 0 to π + [-6sin(t)] from π to 2π + [6sin(t)] from 2π to 3π + [-6sin(t)] from 3π to 4π + [6sin(t)] from 4π to 5π
Distance = 0 meters
Know more about displacement here;
https://brainly.com/question/30087445
#SPJ11
The equation y=21.2x represents Austin's earnings in dollars and cents, y,
for working x hours. Find the rate of change.
Answers
Given:
The equation is
\(y=21.2x\)
Where, y is earnings for working in dollars and cents and x is the number of working hours.
To find:
The rate of change.
Solution:
The slope intercept form of a linear function is
\(y=mx+b\) ...(i)
Where, m is the slope and b is the y-intercept.
We have,
\(y=21.2x\) ...(ii)
On comparing (i) and (ii), we get
\(m=21.2\)
Therefore, the rate of change is 21.2 dollar and cents per hour.
PLEASE HELP ME WILL GAVE BRAINLIEST The measures of the angle of a triangle are shown in the figure below solve for x
65°
76°
(6x-9)°

Answers
Answer:
x=8
Step-by-step explanation:
180-76-65=39
6x-9=39
6x=48
x=8
Answer:
you do 65+76+6 times 8 -9 and then what ever you get is the answers.
Step-by-step explanation:
x=8
production of a daily vitamin tablet, results in tablets with varying amounts of vitamin c. it is claimed that the average amount of vitamin c per tablet is at least 200 milligrams. the consumer watchdog bureau tests a random sample of 70 tablets. the mean content of vitamin c for this sample is 194.3 milligrams, while the standard deviation is 21 milligrams. what is the approximate p-value for the appropriate test?
Answers
To answer this question, we can use a one-sample t-test to determine whether the mean amount of vitamin c per tablet is significantly different from the claimed average of 200 milligrams. The null hypothesis is that the mean amount of vitamin c per tablet is equal to 200 milligrams, while the alternative hypothesis is that it is less than 200 milligrams.
We can calculate the test statistic using the formula:
t = (sample mean - population mean) / (sample standard deviation / sqrt(sample size))
In this case, the sample mean is 194.3 milligrams, the population mean is 200 milligrams, the sample standard deviation is 21 milligrams, and the sample size is 70 tablets. Plugging in these values, we get:
t = (194.3 - 200) / (21 / sqrt(70)) = -2.47
Using a t-table with 69 degrees of freedom (70 minus 1), we can find the p-value associated with this test statistic. The p-value is the probability of obtaining a test statistic as extreme or more extreme than the one we observed, assuming the null hypothesis is true. Looking up -2.47 in the t-table, we find a two-tailed p-value of approximately 0.016. However, since our alternative hypothesis is one-tailed (we are only interested in whether the mean amount of vitamin c is less than 200 milligrams), we need to divide this p-value by 2 to get the appropriate one-tailed p-value.
To know more about one-sample t-test visit :-
https://brainly.com/question/14263025
#SPJ11
If f(x) = 2x2 - 5 and g(x) = x2 - 4x - 8, find (f - g)(x).
O A. (f- g)(x) = x2 - 4x - 3
O B. (f- g)(x) = x2 + 4x + 3
O C. (f- g)(x) = 3x2 - 4x - 13
O D. (f - g)(x) = -x2 - 13
Answers
The value of (f - g)(x) is x² + 4x + 3 if f(x) = 2x² - 5 and g(x) = x² - 4x - 8 option (B) is correct.
What is a function?
It is defined as a special type of relationship, and they have a predefined domain and range according to the function every value in the domain is related to exactly one value in the range.
We have:
f(x) = 2x² - 5
g(x) = x² - 4x - 8
(f - g)(x) = f(x) - g(x)
= (2x² - 5) - (x² - 4x - 8)
= 2x² - 5 - x² + 4x + 8
= x² + 4x + 3
(f - g)(x) = x² + 4x + 3
Thus, the value of (f - g)(x) is x² + 4x + 3 if f(x) = 2x² - 5 and g(x) = x² - 4x - 8 option (B) is correct.
Learn more about the function here:
brainly.com/question/5245372
#SPJ1
In high school X, approximately 9 percent of the students saw a certain movie on opening night. From a random sample of 200 students from high school Y, 22 saw the movie on opening night. Consider a hypothesis test to investigate whether the proportion of all students in high school Y who saw the movie on opening night is greater than that of high school X. Which of the following is the standard deviation used to calculate the test statistic for the one-sample z-test?
a. √(11(89)/200)
b. √(09(91)/200)
c. √(22(78)/200)
d. √(22(78)/200)
Answers
The standard deviation used to calculate the test statistic for the one-sample z-test is option c, i.e. √(22(78)/200).
Let’s take p as the proportion of students from high school Y who watched the movie on opening night.
Then the sample proportion, ˆp = 22/200 = 0.11
We need to find out whether the proportion of all students in high school Y who saw the movie on opening night is greater than that of high school X. The sample proportion for high school X is 0.09.
We can use a one-sample z-test with the following hypotheses.
H₀: p ≤ 0.09
Hₐ: p > 0.09
The null hypothesis represents the assumption that the proportion of students in high school Y who watched the movie on opening night is less than or equal to that of high school X.
The alternative hypothesis represents the assumption that the proportion of students in high school Y who watched the movie on opening night is greater than that of high school X.We calculate the test statistic, z, as follows:
z = (ˆp - p₀) / σ
Where p₀ = 0.09 and σ is the standard deviation of the sample proportion.
We know that
σ = √[(p₀(1 - p₀)) / n]
Where n = 200.
σ = √[(0.09 x 0.91) / 200]
σ = 0.0274
Therefore,
z = (0.11 - 0.09) / 0.0274 = 0.7299
The p-value for this test is P(Z > 0.7299) = 0.2333
At the 5% level of significance, we fail to reject the null hypothesis since the p-value is greater than 0.05. Thus, we do not have sufficient evidence to conclude that the proportion of all students in high school Y who saw the movie on opening night is greater than that of high school X.
Hence option c is correct.
To know more about the "standard deviation": https://brainly.com/question/24298037
#SPJ11
Q.5. Use empirical rule (68-95-99.7% rule) to calculate the following:
[20]
SAT (combined) scores of 200 college-bound seniors in high school has the normal distribution with mean 1050 and standard deviation 150.
a) What is the 84th percentile?
Note: nth percentile is the value for which n% are below that value. So 84th percentile indicates the value for which 84% of seniors will get less than that score.
b) Find the value x such that the 16% of all seniors have SAT score below x.
c) What are the 2.5th and 97.5th percentiles of this distribution?
d) How many seniors scored between 600 and 1350?
e) How many seniors scored less than 1200?
Answers
84th percentile = 1260, 16th percentile = 900, 2.5th percentile = 850, 97.5th percentile = 1300, 112 seniors between 600 and 1350, 180 seniors scored less than 1200.
To calculate the 84th percentile, we need to use the empirical rule. The mean is 1050 and the standard deviation is 150. So, 68% of the seniors will lie within one standard deviation from the mean, 95% within two standard deviations, and 99.7% within three standard deviations. Therefore, 84% of the seniors will lie within 1.12 standard deviations from the mean. This means the 84th percentile is 1050 + 1.12 * 150 = 1260. Similarly, for the 16th percentile, we will find the value 1.04 standard deviations below the mean i.e. 900. For the 2.5th percentile, we will find the value 0.84 standard deviations below the mean i.e. 850. For the 97.5th percentile, we will find the value 1.64 standard deviations above the mean i.e. 1300. The number of seniors scoring between 600 and 1350 is 112, and the number of seniors scoring less than 1200 is 180.
Learn more about percentile here
https://brainly.com/question/28383051
#SPJ4
Kelsey knit a total of 6 centimeters of scarf over 2 nights. After 4 nights of knitting, how many centimeters of scarf will Kelsey have knit in total? Assume the relationship is directly proportional.
Answers
Answer:
\(12\) cm
Step-by-step explanation:
If Kelsey knit a total of \(6\) cm of scarf over \(2\) nights, then we know that she can knit \(\frac{6}{2}=3\) cm each night. Therefore, after \(4\) nights of knitting, Kelsey would have knit a total of \(3*4=12\) cm of scarf in total. Hope this helps!
28. for the following case, would the mean or the median probably be higher, or would they be about equal? explain.
Answers
To determine whether the mean or the median would be higher, or if they would be about equal, we need more specific information about the case or dataset in question.
The mean and median are statistical measures used to describe different aspects of a dataset.
Mean: The mean is the average value of a dataset and is calculated by summing all the values and dividing by the total number of values. The mean is sensitive to extreme values or outliers since it takes into account every value in the dataset.
Median: The median is the middle value in a sorted dataset. If the dataset has an odd number of values, the median is the middle value itself. If the dataset has an even number of values, the median is the average of the two middle values. The median is less affected by extreme values or outliers since it only depends on the order of values.
Without specific information about the dataset, it is difficult to determine whether the mean or the median would be higher or if they would be about equal. Different datasets can exhibit different characteristics, such as skewed distributions or symmetric distributions, which can influence the relationship between the mean and the median.
In general terms, if the dataset is symmetrical and does not contain extreme values, the mean and the median are likely to be about equal. However, if the dataset is skewed or contains extreme values, the mean may be influenced more by these outliers, potentially making it higher or lower than the median.
To provide a more accurate assessment, please provide additional details about the case or dataset under consideration.
Learn more about median here:
https://brainly.com/question/300591
#SPJ11
A baseball team plays in a stadium that holds 50000 spectators. When the ticket price is $10, the average attendance is 27000. When the price is lowered to $6, the average attendance rose to 39000. Find a demand function, D(q), where q is the quantity or number of spectators and D(q) is linear.
Answers
Answer:
the answer is below
Step-by-step explanation:
Demand seems to be based on price.
Therefore we must consider two things:
that "x" is equal to the price and that "y" is equal to the average attendance.
Thus:
the two points would be:
(x1, y1) = (10,27000)
(x2, y2) = (6.39000)
The slope of a straight line is given by:
m = (y2-y1) / (x2-x1)
we replace:
m = (39000 - 27000) / (6 - 10) = 12000 / -4 = -3000
The equation of a straight line can be expressed like this
y = m * x + b.
where
m is the slope and b is the y-intercept.
we replace
y = -3000 * x + b.
To solve for b, replace x and y with the value of one of the points on the line.
We choose (6.39000). and we replace:
39000 = -3000 * 6 + b
39000 = -18000 + b
39000 + 18000 = b
b = 57000.
if we replace we have:
the equation becomes y = -3000 * x + 57000
since it is the demand and * x is the price.
t = d (x), therefore the equation becomes
d (x) = -3000 * x + 57000.
d (x) = 57000 - 3000 * x.
when x = 0, the price is 0 and the demand will be 57000, which will be more than the stadium can contain because the stadium can only contain 50,000.
So:
when x = 6, the price is 6 and the demand is 57000 - 18000 = 39000.
when x = 10, the price is 10 and the demand is 57000 - 30000 = 27000.
Which two fractions name point R on the number line?

Answers
Answer:
The answer is D.) 6/8 and 3/4
Step-by-step explanation:
If you count how many lines there are you get 8 lines so we know the denominator has to be eight and since the point is on the 6th line the first one is 6/8 and since you can simplify it you get 3/4 which gives you D.)
Hope I helped
A brainliest is always appreciated
A group of coins is shown below. What is the probability and it's compliment of randomly selecting a nickel?
Answers
Answer:
Please post the full question.
Step-by-step explanation:
I am trying to help, but the question is missing some key info.
Please dont delete, as when he posts the full thing, I will reply to this answer with the answer and explanation.
What’s the answer to this?
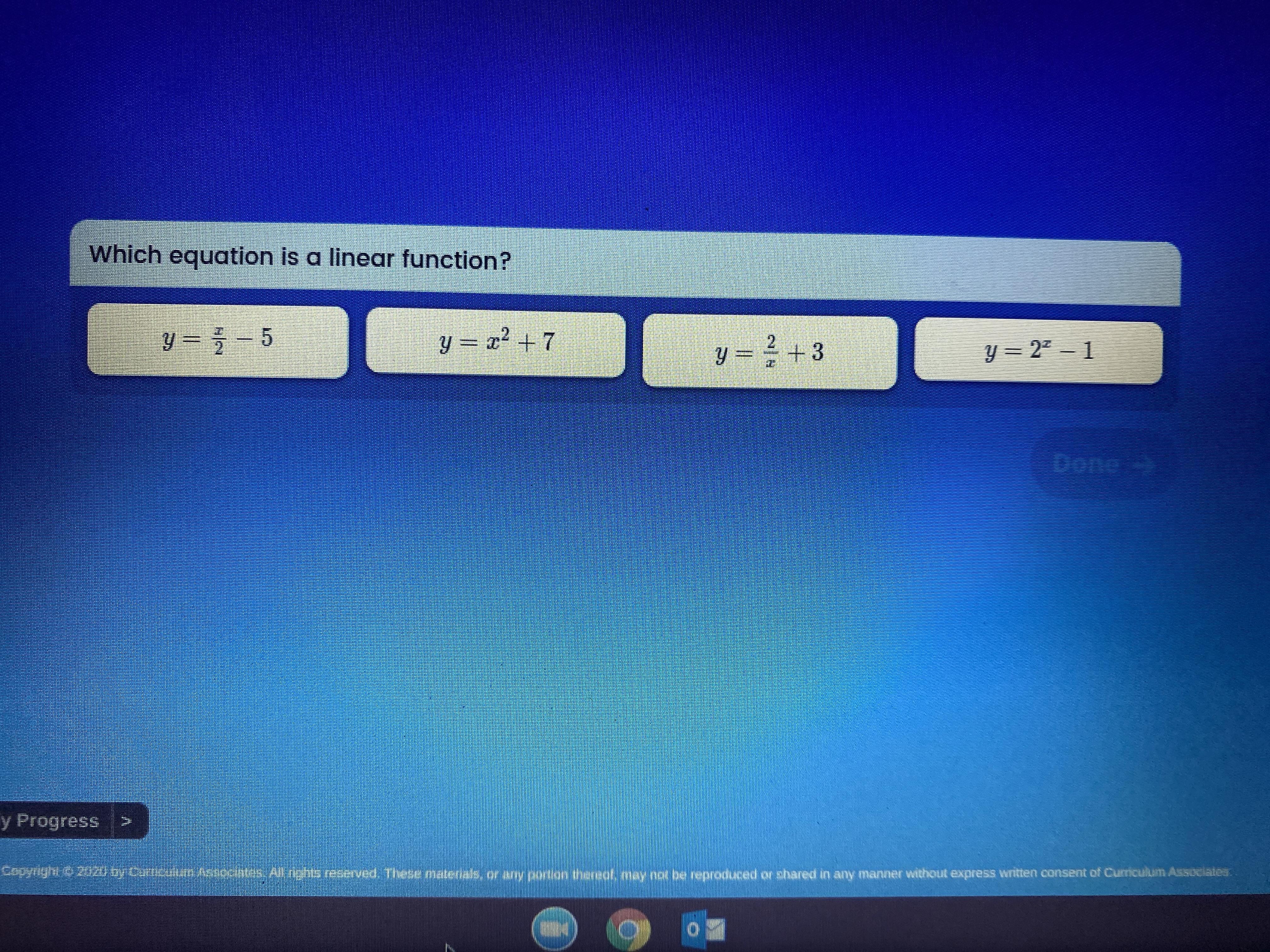
Answers
Answer:
A
Step-by-step explanation:
Linear functions are those whose graph is a straight line. A linear function has the following form. y = f(x) = a + bx. A linear function has one independent variable and one dependent variable. The independent variable is x and the dependent variable is y.
if a vanilla scoop cost 10$, and the other flavors cost 15$ a scoop, what is the probability a double-dip cone will cost 30$?
Answers
The subject of the formula below is y.
a=4x/t - p
Rearrange the f make x the subject.
Answers
Answer:
x = t(a+p)/4
Step-by-step explanation
Given the expression
a=4x/t - p
We are to make x the subject of the formula
a=4x/t - p
Add p to both sides
a+p = 4x/t - p+p
a+p = 4x/t
Cross multiply
t(a+p) = 4x
Rearrange
4x = t(a+p)
Divide both sides by 4
4x/4 = t(a+p)/4
x = t(a+p)/4
Identify each angle as an inscribed angle or a central angle.

Answers
Answer:
∠AFE is an inscribed angle because the vertex lies on the edge of the circle
∠DOB is a central angle because the vertex lies at the center of the circle
∠HJG is an inscribed angle because the vertex lies on the edge of the circle
Challenger Elementary School has 800 students. Every Wednesday, 12% of the students stay after school for Chess Club. How many students attend on Wednesday’s?
Answers
Answer:
96 students attend chess club after school on Wednesday's.
Step-by-step explanation:
To solve this problem, simply multiply 800 x 0.12.
800 x 0.12 = 96
I hope this was helpful to you! If it was, please consider rating, pressing thanks, and marking my answer Brainliest. It would help a lot. Have a great day!
Answer:
\(\huge\boxed{\text{96 students}}\)
Step-by-step explanation:
In order to find how many students stay back for Chess Club, we want to find 12% of 800.
To do this, we can set up a percentage proportion.
\(\frac{12}{100} = \frac{x}{800}\)
We can now solve for x by cross multiplying.
\(800 \cdot 12 = 9600\\9600 \div 100 = 96\)
So, 96 students attend Chess Club on Wednesdays.
Hope this helped!
y=mx+b (-6,-3) and (0,9)
Answers
Answer:
y = 2x + 9
Step-by-step explanation:
The equation of a line in slope- intercept form is
y = mx + b ( m is the slope and b the y- intercept )
Calculate m using the slope formula
m = \(\frac{y_{2}-y_{1} }{x_{2}-x_{1} }\)
with (x₁, y₁ ) = (- 6, - 3) and (x₂, y₂ ) = (0, 9)
m = \(\frac{9+3}{0+6}\) = \(\frac{12}{6}\) = 2
The line crosses the y- axis at (0, 9) ⇒ b = 9
y = 2x + 9 ← equation of line
a number y is 15 larger than a positive number x. if their sum is not more than 85, what are the possible values of y?
Answers
Answer:
y = x + 15 –(1)
x + y = 85
Putting (1)'s value,
x + x + 15 = 85
2x + 15 = 85
2x = 85 - 15 = 70
x = 70/2 = 35
\(\)
The value of y is 50.
Two simultaneous equations can be formed from the given question:
y - x = 15 equation 1
y + x = 85 equation 2
In order to determine the value of x, subtract equation 1 from 2
2x = 70
Dvidie both sides of the equation by 2
x = 70 / 2
x = 35
Substitute for x in equation 1
y - 35 = 15
y = 35 + 15
y = 50
To learn more about simultaneous equations, please check: https://brainly.com/question/25875552
Nelson makes 6 quarts of lemonade. The ratio of pints to quarts is 2 colon 1.

Answers
Answer:
12:6, or 12 pints of lemonade
PLEASE HELP QUESTION IN PIC.

Answers
Answer:
Perimeter= 40
Area= 120.7
Step-by-step explanation:
find the volume of the given solid. bounded by the coordinate planes and the plane 6x + 4y + z = 24
Answers
Therefore, the volume of the solid bounded by the coordinate planes and the plane 6x + 4y + z = 24 is 96 cubic units.
To find the volume of the solid bounded by the coordinate planes (xy-plane, xz-plane, and yz-plane) and the plane 6x + 4y + z = 24, we need to determine the region in space enclosed by these boundaries.
First, let's consider the plane equation 6x + 4y + z = 24. To find the x-intercept, we set y = 0 and z = 0:
6x + 4(0) + 0 = 24
6x = 24
x = 4
So, the plane intersects the x-axis at (4, 0, 0).
Similarly, to find the y-intercept, we set x = 0 and z = 0:
6(0) + 4y + 0 = 24
4y = 24
y = 6
So, the plane intersects the y-axis at (0, 6, 0).
To find the z-intercept, we set x = 0 and y = 0:
6(0) + 4(0) + z = 24
z = 24
So, the plane intersects the z-axis at (0, 0, 24).
We can visualize that the solid bounded by the coordinate planes and the plane 6x + 4y + z = 24 is a tetrahedron with vertices at (4, 0, 0), (0, 6, 0), (0, 0, 24), and the origin (0, 0, 0).
To find the volume of this tetrahedron, we can use the formula:
Volume = (1/3) * base area * height
The base of the tetrahedron is a right triangle with sides of length 4 and 6. The area of this triangle is (1/2) * base * height = (1/2) * 4 * 6 = 12.
The height of the tetrahedron is the z-coordinate of the vertex (0, 0, 24), which is 24.
Plugging these values into the volume formula:
Volume = (1/3) * 12 * 24
= 96 cubic units
To know more about volume of the solid,
https://brainly.com/question/30079268
#SPJ11
ASAP! What is the surface area of the solid?
14 square inches
20 square inches
24 square inches
32 square inches

Answers
Answer:
I think is is 14 square inches.
Step-by-step explanation:
2 plus 2 plus 5 plus 5 = 14
I don't know if this is right.
not times because 2 time 2 times 5 times 5 equals 100. there is no 100 . divide is not working and subtract is not working so add. I hoke this is correct and helps.
PLEASE HELP ASAP:
1) What is the measure of ⦣4?
2) How many degrees are in a triangle?
3) What is the measure of ⦣8?
4) What is the measure of ⦣5?
5) If the measure of ⦣9 = 9x + 27, what is the value of x?
6) What is the measure of ⦣1?
7) Supplementary angles will add up to...
8) What is the measure of ⦣3?
9) What is the measure of ⦣6?
10) What is the measure of ⦣2?
11) If you add together the degrees from ⦣1 and ⦣2, they will equal..

Answers
Answer:
1. <4=115 degrees
2. 180 degrees
4. 5 degrees
6. 110 degrees
7. 180 degrees
8.65 degrees
Step-by-step explanation:
HELP!!! answer quickly pls

Answers
The first one B
Step by step explanation:
B is (2,4) then do 2+1 which is 3 and then 4 -3 which is 1 and your left with (3,1) which is point B
Probit coefficients are typically estimatedâ using:
A.
the method of maximum likelihood.
B.
the OLS method.
C.
by transforming the estimates from the linear probability model.
D.
nonlinear least squaresâ (NLLS).
Answers
Probit coefficients are typically estimated using:
A. the method of maximum likelihood.
The method of maximum likelihood is used to estimate the probit coefficients. This method aims to find the coefficients that maximize the likelihood of observing the given sample data. It involves an iterative process to identify the most likely parameter values for the model, making it suitable for nonlinear models like the probit model. Maximum likelihood estimation is a widely used method in econometric analysis due to its desirable properties, such as consistency and asymptotic efficiency.
In summary, probit coefficients are estimated using the method of maximum likelihood, which provides the most accurate and efficient estimates for this type of model.
Learn more about maximum likelihood here: brainly.com/question/30625970
#SPJ11
The price of an item yesterday was $120. Today, the price rose to $156. Find the percentage increase.
Answers
Answer:30%
Step-by-step explanation:
GAIN = 156- 120=$ 36
% GAIN = \(\frac{gain}{old cost price } X 100%\\\)
= \(\frac{36}{120} X 100%\)
=30%
two linear functions are combined with addition, and then the same two linear functions are combined by multiplication.which functions could be the result of the combinations? select two options.16x – 1217x – 1272x2 – 96x72x2
Answers
The two options that could result from combining two linear functions with addition are "16x – 12" and "17x – 12". The r to your question is:
To combine two linear functions with addition, you simply add the coefficients of the same variables. For example, if you have the functions 3x + 4 and 2x - 5, when you combine them with addition, you add the coefficients of x and the constant terms. So, 3x + 4 + 2x - 5 becomes (3 + 2)x + (4 - 5) = 5x - 1.
To combine two linear functions with multiplication, you multiply the coefficients of the same variables. For example, if you have the functions 3x + 4 and 2x - 5, when you combine them with multiplication, you multiply the coefficients of x and the constant terms. So, (3x + 4)(2x - 5) becomes
(3 * 2)x^2 + (3 * -5)x + (4 * 2x) + (4 * -5)
= 6x^2 - 15x + 8x - 20
= 6x^2 - 7x - 20.
To know more about functions visit:
https://brainly.com/question/31062578
#SPJ11
HELP BTW I NEED THE BOTTOM PART DONE NOTHING RLSE !

Answers
Answer:
0=0 one solution minus three equal to zero no solution
