describe the difference in the shape and nature of the t and z distributions. in what ways are they the same? do they change in shape? if so, what causes the change? if not, why not?
Answers
The difference between the t and z distributions is primarily in their shape and nature.
The t-distribution is more spread out and has heavier tails than the z-distribution, which is more symmetrical and closer to a normal distribution. This is due to the fact that the t-distribution is based on smaller sample sizes and therefore has more variability, while the z-distribution is based on larger sample sizes and has less variability.
In terms of the way,s they are the same, both the t and z distributions are used to make inferences about population parameters based on sample data. They both also have a mean of 0 and are symmetrical about the mean.
The change in the shape of the t-distribution occurs as the sample size increases. As the sample size increases, the t-distribution becomes more like the z-distribution, with less spread and thinner tails. This is because larger sample sizes result in less variability and therefore a distribution that is closer to the normal distribution. The cause of the change is the increase in sample size.
The z-distribution, on the other hand, does not change in shape as the sample size increases because it is already based on large sample sizes and therefore has less variability. So, the answer to the question "do they change in shape? if so, what causes the change? if not, why not?" is that the t-distribution does change in shape as the sample size increases, while the z-distribution does not, for the reasons explained above.
To know more about t and z distributions refer here:
https://brainly.com/question/30032500#
#SPJ11
Related Questions
Help me find the figure , area formula and the area

Answers
So the area is 432
Answer:
We are given a length of 27 cm and a width of 16 cm and we can also see that our figure is a rectangle. This means that we are able to use the area formula for the rectangle which is \(A=l*w\).
Therefore, we can just multiply 27 cm by 16 cm and we get \(A = 432\ cm^2\)
Hope this helps! Let me know if you have any questions
Lines / and mare parallel. Line / can be represented by the equation 3x + 4y = 8. If line m passes through the po
m?
y-5= -
(x+2)
y +5 = -
44-2)
y-5= f «+2)
y+5 = ģ«-2)
Answers
Answer:B
Step-by-step explanation:
Answer:
B y-5=- 3/4(x-2)
Step-by-step explanation:
3 Simplify 3e - e + 4e
Answers
which programming language was used in the first generation conputer
I:machine language
2:assembly language
3:high level language
3:natural language
Answers
Answer:
machine language
Step-by-step explanation:
The computers in this generation used machine code as the programming language.
The machine language also referred to as the native language of the computer system is the first generation programming language. In the machine language, a programmer only deals with a binary number. They are translation free and can be directly executed by the computers.
which of the following is the best description of a population that has a stable age distribution? responses a large population that is growing at a constant rate a large population that is growing at a constant rate a large population with a negative growth rate a large population with a negative growth rate a population that is in the early stages of logistic population growth a population that is in the early stages of logistic population growth a growing population in which the proportions of individuals in the different age classes remain constant a growing population in which the proportions of individuals in the different age classes remain constant a small population that has not yet achieved exponential growth
Answers
the best description of a population that has a stable age distribution is (e) a growing population in which the proportions of individuals in the different age classes remain constant.
What does it mean to have a stable age distribution?Such a population will have a steady age distribution since the proportion of people in each age group will remain constant. Independent of the initial age distribution is this steady age distribution and is solely dependent on maintaining stable fertility and death rates.
It has been demonstrated that a closed population's annual rate of increase tends to become constant when it is exposed to age-specific fertility and mortality rates for a considerable amount of time.
A population that has reached this point is referred to as stable, and this consistent rate of growth is known as the intrinsic rate of natural increase.
Such a population will have a steady age distribution since the proportion of people in each age group will remain constant.
to learn more about stable age distribution visit:
brainly.com/question/29762197
#SPJ1
which pairs of angles in the figure below are verticle angles
Answers
Answer: Can you add a picture
Step-by-step explanation:
does a parallelogram have 2 pairs of parallel sides
Answers
Answer:
A parallelogram is a quadrilateral with 2 pairs of parallel sides
Can you guys helppppppppppppp

Answers
Step-by-step explanation:
to find this angle you can use the sin ratio seeing that the opposite has been given which is 35 and the hypotenuse which is 48.so the solution will be
sin?=opposite/hypotenuse
sin?=35/48
sin?=0.729
?=sin inverse of 0.729
?=46.8 rounded off to 47°
I hope this helps
Hi
in application of sinus formula : ( must learn your trigonimetric formulas by heart !)
value of ? is sin^(-1) = (35/48) = 46,8. so 47 when rounded to the nearest degree
What is the following property called X Y z X Y XZ?
Answers
The following property: X (Y + Z ) = XY + XZ is known as the Distributive Property.
The distributive property is also known as the distributive law of multiplication over addition and subtraction. The name itself signifies that the operation includes dividing or distributing something. The distributive law is applicable to addition and subtraction.
X (Y + Z ) = XY + XZ (left distributive law) and (X + Y) Z = XZ + YZ (right distributive law). This property also allows like terms to be combined so AX + BX = (A + B)X.
This distributive law is also applicable to subtraction and is expressed as, A (B - C) = AB - AC. This means operand A is distributed between the other two operands.
To know more about distributive property visit: brainly.com/question/5637942
#SPJ4
On a coordinate plane, a parabola opens up. It goes through (negative 7, 5), has a vertex at (negative 4, negative 4), and goes through (negative 1, 5).
The graph of the function f(x) = x2 + 8x + 12 is shown. Which statements describe the graph? Check all that apply.
The vertex is the maximum value.
The axis of symmetry is x = –4.
The domain is all real numbers.
The range is all real numbers.
The function is increasing over (–∞, –4).
The x-intercepts are at (–6, 0) and (–2, 0).
Answers
Answer:
B: The axis of symmetry is x + -4.
C: The domain is all real numbers.
F: The x-intercepts are at (-6, 0) and (-2, 0).
Step-by-step explanation:
worked on edge 2022
The answer is the First, Third, and Last
A train arrived at the station. If you count cars starting from the first one, the dining car is th 7th in the row. If you count cars starting from the last one, then the dining car is the 8th in a row. How many cars are there in this train?
Answers
Answer:
15
Step-by-step explanation:
from the first then it is 0+7=7
and from the last it is 0+8=8
then the number of cars available in the station is 8+7=15
The label on a \frac{1}{4}
4
1
-pound bag of seeds states that it will cover an area of 7575 square feet. How many square feet do the seeds cover per pound?
Answers
Answer:
\(183.63\) square feet area is covered by seeds per pound
Step-by-step explanation:
Given
\(41\frac{1}{4}\) pound bag of seed cover an area of \(7575\) square feet
\(\frac{165}{4}\) pound bag of seed cover an area of \(7575\) square feet
Or
\(7575\) square feet area is covered by \(\frac{165}{4}\) pound bag of seed
Area in square feet covered by one pound bag of seed
\(= \frac{7575}{\frac{165}{4} } \\= \frac{7575 * 4}{165} \\= 183.63\) square feet
the mean annual tuition and fees for a sample of 14 private colleges in california was $33500 with a standard deviation of $7350. a dotplot shows that it is reasonable to assume that the population is approximately normal. can you conclude that the mean tuition and fees for private institutions in california is less than $35000? use the o
Answers
We can conclude that the mean tuition and fees for private institutions in California are less than $35000 with 95% confidence.
Yes, we can conclude that the mean tuition and fees for private institutions in California are less than $35000. We can use a one-sample t-test to determine whether the mean annual tuition and fees for the sample of 14 private colleges in California is significantly less than $35000.
First, we need to calculate the t-statistic:
t = (sample mean - hypothesized mean) / (standard error of the mean)
The hypothesized mean is $35000, the sample mean is $33500, and the standard error of the mean is calculated as follows:
standard error of the mean = standard deviation / sqrt(sample size)
standard error of the mean = $7350 / sqrt(14)
standard error of the mean = $1965.15
Plugging in these values, we get:
t = ($33500 - $35000) / $1965.15
t = -1.555
Using a t-table with 13 degrees of freedom (14-1), we find that the critical value for a one-tailed test with alpha = 0.05 is -1.771. Since our calculated t-value (-1.555) is greater than the critical value (-1.771), we fail to reject the null hypothesis that the mean tuition and fees for private institutions in California are $35000. Therefore, we can conclude that the mean tuition and fees for private institutions in California are less than $35000 with 95% confidence.
Learn more about mean here: brainly.com/question/31101410
#SPJ11
What is the opposite of the number shown by the dot on this number line?
A) |.37|
B) .37
C) -.37

Answers
Answer:
C.
Step-by-step explanation:
The opposite of a positive number is the same number, but negative. In this case, 0.37 -> -0.37.
The replacement value of a home is 132,000. The home is insured for 80% of its value. What is the amount of insurance coverage?
Answers
Answer:
105,600
Step-by-step explanation:
80% of 132,000 is 105,600, therefore this is how much the insurance will cover
just the red triangle use the Phytogram theorem please :(
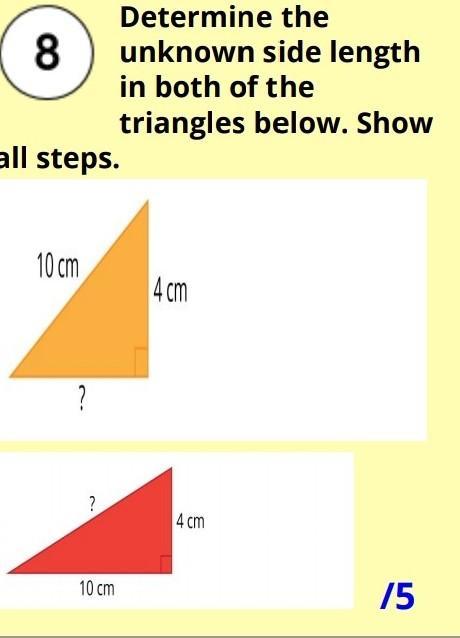
Answers
Explanation: The formula for the triangle is A^2 + B^2 = C^2. So plugging that into this equation is 10^2 + 4^ = C^2, making it 116 = C^2, square root both sides and it equals 10.770033.
Determine whether the given set S is a subspace of the vector space V.
A. V=P5, and S is the subset of P5 consisting of those polynomials satisfying p(1)>p(0).
B. V=ℝ3, and S is the set of vectors (x1,x2,x3) in V satisfying x1−6x2+x3=5.
C. V=ℝn, and S is the set of solutions to the homogeneous linear system Ax=0 where A is a fixed m×n matrix.
D. V=C2(I), and S is the subset of V consisting of those functions satisfying the differential equation y″−4y′+3y=0.
E. V is the vector space of all real-valued functions defined on the interval [a,b], and S is the subset of V consisting of those functions satisfying f(a)=5.
F. V=Pn, and S is the subset of Pn consisting of those polynomials satisfying p(0)=0.
G. V=Mn(ℝ), and S is the subset of all symmetric matrices
Answers
(a) Given that V=P5, and S is the subset of P5 consisting of those polynomials satisfying p(1)>p(0).We know that p(1) > p(0) is a linear function as it satisfies the following criteria:If p and q are two polynomials satisfying p(1) > p(0) and q(1) > q(0), then for any real number c, cp+q(1) > cp+q(0).
Therefore, S is a subspace of P5.(b) Let V=ℝ3, and S is the set of vectors (x1,x2,x3) in V satisfying x1−6x2+x3=5.The zero vector, (0,0,0), is not in S since 0−6(0)+0=0 ≠ 5. Therefore, S is not a subspace of V.(c) Let V=ℝn, and S is the set of solutions to the homogeneous linear system Ax=0 where A is a fixed m×n matrix.Ax=0 is a homogeneous linear system. Therefore, the trivial solution x=0 is in S. Therefore, S is a subspace of V.(d) Let V=C2(I), and S is the subset of V consisting of those functions satisfying the differential equation y″−4y′+3y=0.The zero function, y=0, is in S. If f and g are in S, then f+g and cf are also in S for any scalar c. Therefore, S is a subspace of V.(e) Let V be the vector space of all real-valued functions defined on the interval [a,b], and S is the subset of V consisting of those functions satisfying f(a)=5.The zero function, f(x)=0, is in S. If f and g are in S, then f+g and cf are also in S for any scalar c. Therefore, S is a subspace of V.(f) Given that V=Pn, and S is the subset of Pn consisting of those polynomials satisfying p(0)=0.The zero polynomial, p(x)=0, is in S. If p and q are in S, then p+q and cp are also in S for any scalar c. Therefore, S is a subspace of V.(g) Let V=Mn(ℝ), and S is the subset of all symmetric matricesThe zero matrix, 0, is in S. If A and B are symmetric, then A+B and cA are also symmetric. Therefore, S is a subspace of V.
To know more about subspace visit:
https://brainly.com/question/15580495
#SPJ11
What is quotient formula?
Answers
A quotient is a number created when two numbers are divided. The formula for this is Quotient = Dividend ÷ Divisor.
The division is the process of dividing one number by any other number to produce a different number. Consequently, the dividend is the number that is divided in this instance. A number's divisor is the one that divides the given number. The quotient is the number that we get as a result.
Then, the quotient formula is given by Quotient = Dividend ÷ Divisor. For example, 50÷5=10. Here, 50 is the dividend, 5 is the divisor, 10 is the quotient, and 0 is the remainder.
To know more about quotient:
https://brainly.com/question/29786626
#SPJ4
Events D and E are independent, with P(D)- 0.6 and P(D and E) - 0.18. Which of the following is true? A. P(E)- 0.12 B. P(E) = 0.4 C. P(D or E)-0.28 D. P(D or E) 0.72 E. P(D or E)-0.9
Answers
The correct statement is: A. P(E) = 0.3. The probability of event E, denoted as P(E), is equal to 0.3.
To determine the correct answer, let's analyze the given information.
We know that events D and E are independent, which means that the occurrence of one event does not affect the probability of the other event happening.
Given:
P(D) = 0.6
P(D and E) = 0.18
Since events D and E are independent, the probability of both events occurring (P(D and E)) can be calculated as the product of their individual probabilities:
P(D and E) = P(D) * P(E)
Substituting the given values:
0.18 = 0.6 * P(E)
To find the value of P(E), we can rearrange the equation:
P(E) = 0.18 / 0.6
P(E) = 0.3
Therefore, the correct answer is A. P(E) = 0.3.
Learn more about probability here: https://brainly.com/question/32117953
#SPJ11
Follow the given steps to subtract mixed numbers with different denominators: Step 1– Convert the mixed numbers into improper fractions. Step 2– Find the common multiple of both the denominators. Step 3– Convert the fractions as common denominators.
Answers
The steps for subtracting mixed numbers are the transformation of improper fractions, subtraction of denominator, then subtracting numerator, making it the smallest term, and reverse.
Finding the difference between two mixed numbers is required when subtracting mixed numbers. The steps for subtracting mixed numbers are as follows:
Step 1: Transform the mixed values into improper fractions in step 1. To achieve this, divide the total by the denominator, then add the numerator. The new numerator is the outcome, and it is positioned above the denominator.
Step 2: Identify a common denominator in step two. You must identify a common denominator to subtract fractions with various numerators.
Step 3: Subtract the fractions in step three. Subtract the numerators from the fractions while maintaining the same denominator.
Step 4: Make the outcome simpler. Simplify the outcome if you can by breaking the fraction down into its smallest terms. 13/4 in this instance may be expressed as 3 1/4.
Step 5: Reverse the incorrect fraction to a mixed number in step 5. Divide the numerator by the denominator to return the improper fraction to a mixed number. The full number serves as the numerator, and the remainder serves as the quotient.
Learn more about mixed numbers at
https://brainly.com/question/24137171
#SPJ4
The question is -
How to Subtract mixed numbers?
The price of an oven was increased by 20% to £156.
What was the price before the increase?
Answers
Answer:
Step-by-step explanation:
Before the increase it was 100%
So 20+100=120
156/120*100=130$
Don’t do it like 20/100 *156 and then minus it from 156 because you will get a wrong answer
(This was answered by another person on a another question.)
Answer:
130
Step-by-step explanation:
x * 1.2 = 156
x = 156/1.2
x = 130
can some one help plz
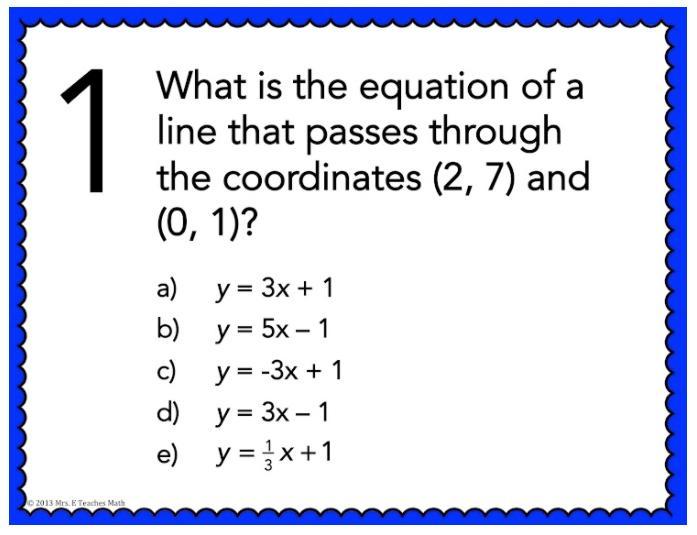


Answers
Is considering starting a new factory. if the required rate of return for this factory is 14.25 percent. based solely on the internal rate of return rule, should nadia accept the investment?
Answers
The internal rate of return (IRR) is a financial metric used to evaluate the profitability of an investment project. It is the discount rate that makes the net present value (NPV) of the project equal to zero. In other words, it is the rate at which the present value of the cash inflows equals the present value of the cash outflows.
To determine whether Nadia should accept the investment in the new factory, we need to compare the IRR of the project with the required rate of return, which is 14.25 percent in this case.
If the IRR is greater than or equal to the required rate of return, then Nadia should accept the investment. This means that the project is expected to generate a return that is at least as high as the required rate of return.
If the IRR is less than the required rate of return, then Nadia should reject the investment. This suggests that the project is not expected to generate a return that is high enough to meet the required rate of return.
So, to determine whether Nadia should accept the investment, we need to calculate the IRR of the project and compare it with the required rate of return. If the IRR is greater than or equal to 14.25 percent, then Nadia should accept the investment. If the IRR is less than 14.25 percent, then Nadia should reject the investment.
To know more about internal rate of return refer here:
https://brainly.com/question/31870995#
#SPJ11
Felicia invests a sum of money into a savings account which gets 4.25% per year compound interest.
After 25 years Felicia has £10380 in the account.
How much did Felicia invest in the account at the beginning?
Answers
\(~~~~~~ \textit{Compound Interest Earned Amount} \\\\ A=P\left(1+\frac{r}{n}\right)^{nt} \quad \begin{cases} A=\textit{accumulated amount}\dotfill & \pounds 10380\\ P=\textit{original amount deposited}\\ r=rate\to 4.25\%\to \frac{4.25}{100}\dotfill &0.0425\\ n= \begin{array}{llll} \textit{times it compounds per year}\\ \textit{per year, thus once} \end{array}\dotfill &1\\ t=years\dotfill &25 \end{cases}\)
\(10380 = P\left(1+\frac{0.0425}{1}\right)^{1\cdot 25} \implies 10380=P(1.0425)^{25} \\\\\\ \cfrac{10380}{(1.0425)^{25}}=P\implies 3666.87\approx P\)
A fifteen-year bond, which was purchased at a premium, has semiannual coupons. The amount for amortization of the premium in the second coupon is $982.42 and the amount for amortization in the fourth coupon is $1052.02. Find the amount of the premium. Round your answer to the nearest cent. Answer in units of dollars. Your answer 0.0% must be within
Answers
The amount of the premium is $1844.19.
Let's assume that the face value of the bond is $1000 and the premium is x dollars.
It is known that the bond has a semi-annual coupon and it is 15-year bond, meaning that it has 30 coupons.
Then the premium per coupon is `(x/30)/2 = x/60`.
The first coupon has the premium amortization of `x/60`.
The second coupon has the premium amortization of $982.42.
The third coupon has the premium amortization of `x/60`.
The fourth coupon has the premium amortization of $1052.02.And so on.
The sum of the premium amortizations is equal to the premium x: `(x/60) + 982.42 + (x/60) + 1052.02 + ... = x`.
This can be rewritten as: `(2/60)x + (982.42 + 1052.02 + ...) = x`
Notice that the sum of the premium amortizations from the 4th coupon is missing.
The sum of these values can be written as `x - (x/60) - 982.42 - (x/60) - 1052.02 = (28/60)x - 2034.44`.
Therefore, the equation can be written as: `(2/60)x + 2034.44 = x`.Solving for x, we get: `x = $1844.19`.
Therefore, the amount of the premium is $1844.19.
Know more about face value here,
https://brainly.com/question/32486794
#SPJ11
a recipe for sparking grape juice calls for 1 1/2 quarts of sparking water and 3/4 quarts of grape juice. how much sparking water would you need to mix with 9 quarts of grape juice?
Answers
Answer:
you would need 18 quarts of sparkling water to mix with 9 quarts of grape juice
Step-by-step explanation:
1 1/2 is double of 3/4 so you just need to double 9 quarts of grape juice
Answer:
18 quarts of sparkling water
Step-by-step explanation:
9÷0.75=12 to figure how many times the recipe is multiplied.
12x1.5=18 to figure out how much sparkling water
3/4 = 0.75
1 1/2 = 1.5
What is the quotient: (3x2 + 8x – 3) ÷ (x + 3) ?. Answer.
A) 3x2 – 11
B) 3x – 11
C) 3x2 – 11 –
D) 3x – 11 –
Answers
Situation:
Find the substance's half-life, in days.
• Round your answer to the nearest tenth.
A 25 gram sample of a substance that's
used for drug research has a k-value of
0.1229.
Enter the correct answer.
N = Noe
-kt
DONE
No = initial mass (at time t = 0)
?
N = mass at time t
k = a positive constant that depends on
the substance itself and on the units
used to measure time
t = time, in days
Answers
Answer:
5.6 days
Step-by-step explanation:
We are given;
Initial Mass; N_o = 25 g
Mass at time(t); N_t = 25/2 = 12.5 (I divide by 2 because we are dealing with half life)
k = 0.1229
Formula is given as;
N_t = N_o•e^(-kt)
Plugging in the relevant values;
12.5 = 25 × e^(-0.1229t)
e^(-0.1229t) = 12.5/25
e^(-0.1229t) = 0.5
(-0.1229t) = In 0.5
-0.1229t = -0.6931
t = -0.6931/-0.1229
t = 5.6 days
The ratio of the ages of Clara and John is 3 to 4,
respectively.
In 20 years, the ratio of the ages of Clara and John will be 7
to 8.
Find how many years that Clara is younger than John.
Answers
Clara is currently 5 years younger than John.
Let's assume the current age of Clara as "3x" and the current age of John as "4x," where "x" represents the common factor of their ages.
According to the given information, the ratio of their ages in 20 years will be 7 to 8. This means that if we add 20 years to their current ages, the new ratio will be 7 to 8. So, we can set up the following equation:
(3x + 20) / (4x + 20) = 7/8
To solve this equation, we can cross-multiply:
8(3x + 20) = 7(4x + 20)
24x + 160 = 28x + 140
160 - 140 = 28x - 24x
20 = 4x
x = 5
Now we can find their current ages:
Clara's current age = 3x = 3 × 5 = 15 years
John's current age = 4x = 4 × 5 = 20 years
To find how many years Clara is younger than John, we subtract Clara's age from John's age:
John's age - Clara's age = 20 - 15 = 5 years
Therefore, Clara is currently 5 years younger than John.
Learn more about common factor here:
https://brainly.com/question/30961988
#SPJ11
As the degrees of freedom increase, the t distribution approaches the _____ distribution. a. exponential b. p c. normal d. uniform
Answers
As the degrees of freedom (DF) increase, the t distribution approaches the normal distribution. Therefore, the correct answer option is: C. normal distribution.
What is the degrees of freedom (DF)?The degrees of freedom (DF) in a sample are typically used to correct a possible bias that exists between the alternative and null hypotheses because they are the maximum number of logically independent numerical values (data points) in the final calculation of a statistical problem.
What is a normal distribution?A normal distribution is sometimes referred to as the Gaussian distribution and it can be defined as a probability distribution that is continuous and symmetrical on both sides of the mean, which shows that all data near the mean have a higher frequency than data that are far from the mean.
In this context, we can reasonably infer and logically deduce that the t-distribution generally approaches the normal distribution as the degrees of freedom (DF) increase.
Read more on degrees of freedom here: https://brainly.com/question/22849398
#SPJ4