Answers
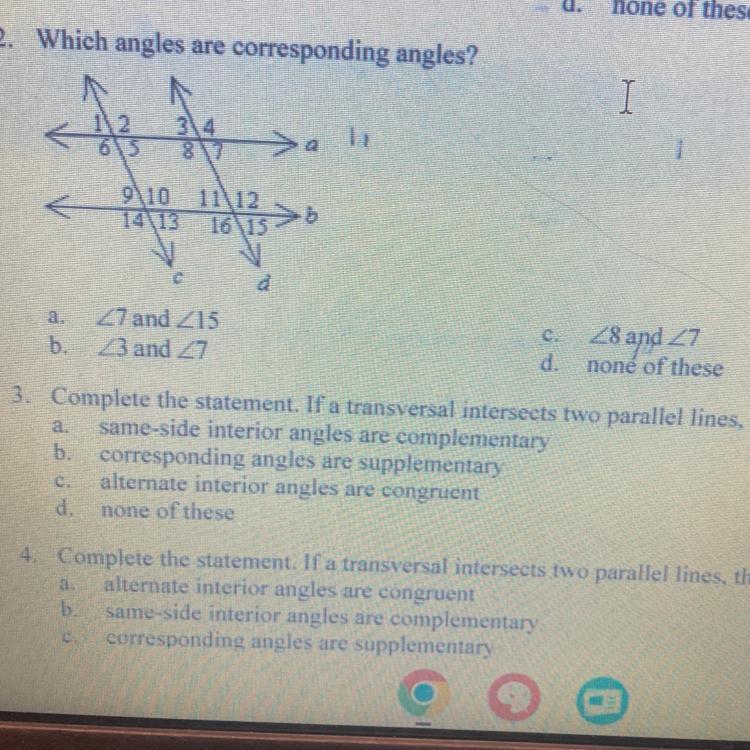
Answer: A
Step-by-step explanation:
I'm assuming you mean question 1.
a is correct
b is not correct
c is not correct
d is not correct
Related Questions
Which relation is displayed in the table?
A: {(-2, -3), (1, -1), (2, -2), (3, 3)}
B: {(-3, -2), (-1, 1), (2, -2), (3, 3)}
C: {(-2, -3), (-1, 1), (-2, 2), (3, 3)}
D: {(-2, -3), (-1, 1), (-2, -2), (3, 3)}

Answers
The relation displayed in the table is
B: {(-3, -2), (-1, 1), (2, -2), (3, 3)}How to find the relation in the tableThe relation in the table is compared by identifying how a coordinate point are expressed as ordered pair and how they are expressed as a table
For instance, say (b, c) is represented in a table as
x y
a b
Using this instance and writing out the values in the table we have
(3, 3), (-1, 1), (2, -2), and (-3, 2)
This is similar to option B making option B the appropriate option
Learn more about ordered pair at
https://brainly.com/question/11139505
#SPJ1
A map Uses a scale of — centimeter = 75 kilometers.
50 kilometers
Answers
Answer:
Step-by-step explanation:
75
Neal estimated √50 by determining that the two perfect squares nearest 50 are 49 and 64. select the two consecutive whole numbers that the √50 is between to complete the sentence. √50 is between and .
Answers
Two consecutive whole numbers representing the estimated square root of 50 are 7 and 8.
Number need to estimate square root = 50
To estimate √50,
Neal found that the two perfect squares nearest 50 are 49 and 64.
Since 50 is closer to 49 than to 64,
Square root of 49 is equal to 7
Square root of 64 is equal to 8.
We know that √50 is closer to the square root of 49 which is 7.
Estimated two consecutive whole numbers are 7 and 8.
Therefore, estimated √50 is between the two consecutive whole numbers 7 and 8.
Learn more about estimation here
brainly.com/question/11679843
#SPJ4
The above question is incomplete, the complete question is:
Neal estimated √50 by determining that the two perfect squares nearest 50 are 49 and 64. Select the two consecutive whole numbers that the √50 is between to complete the sentence.
!! PLEASE ANSWER QUICKLY TIMED REVIEW WILL LIST BRANLIEST!!!

Answers
Answer:
First box is 4 2nd box is 6
Step-by-step explanation:
The next box that is empty will be 4 and the last empty box will be 6
Step-by-step explanation:
2*1=2
2*2= 8
2*3=6
What is a power of 3 equation called?
Answers
A power of 3 equation is called as cubic equation.
The term equation in math is known as a mathematical statement with an 'equal to' symbol between two expressions that have equal values.
Here we need to find the term that has been used for power of 3 equation.
Here we have to know that the power of 3 equation is known as cubic equation.
in other terms the polynomial equation which has a degree of three is called a cubic polynomial equation or trinomial polynomial equation.
As we all know that the power of the variable is the maximum up to 3, therefore, we get three values for a variable, say x.
The general form of the power of 3 equation is written as
=> ax³ + bx² + cx + d = 0
To know more about equation here.
https://brainly.com/question/10413253
#SPJ4
in a haplodiploid system, calculate the relatedness of a son to a maternal aunt.
Answers
In a haplodiploid system, the relatedness of a son to a maternal aunt is 75%.
In a haplodiploid system, males develop from unfertilized eggs and are haploid, while females develop from fertilized eggs and are diploid. This means that sons inherit all of their genetic material from their mother, including her alleles from both her haploid sets of chromosomes. Maternal aunts, on the other hand, share one set of haploid chromosomes with their nephew (the son), as they are the sister of his mother. Therefore, the relatedness between a son and his maternal aunt in a haplodiploid system is 0.75 or 75%.
In a haplodiploid system, the relatedness of a son to a maternal aunt can be calculated using the following steps:
1. Determine the relatedness of the son to his mother: In haplodiploid systems, sons are haploid and inherit their single set of chromosomes from their mother. This means that they are 100% related to their mother, as they share all her genes.
2. Determine the relatedness of the maternal aunt to the son's mother: The maternal aunt is a sister of the son's mother. In haplodiploid systems, sisters share 75% of their genes, as they get half of their genes from their mother and the other half from their father (who, as a haploid male, gives all his genes to his daughters).
3. Calculate the relatedness of the son to his maternal aunt: To determine the relatedness of the son to his maternal aunt, multiply the son's relatedness to his mother (100%) by the maternal aunt's relatedness to the son's mother (75%).
Relatedness of son to maternal aunt = (1.0) * (0.75) = 0.75 or 75%
So, in a haplodiploid system, the relatedness of a son to a maternal aunt is 75%.
Learn more about haplodiploid system here: brainly.com/question/31081669
#SPJ11
 help pleaseeeeee !!!! i’ll give brainliest !

Answers
10) opposite
For any positive integer n, let An denote the surface area of the unit ball in Rn, and let Vn denote the volume of the unit ball in Rn. Let i be the positive integer such that Ai>Ak for all k k not equal to i. Similarly let j be the positive integer such that Vj>Vk for all k not equal to j. Find j−i.
Answers
To find the value of j - i, we need to determine the relationship between the surface areas (An) and volumes (Vn) of the unit ball in Rn for different positive integers n.
For the unit ball in Rn, the formula for surface area (An) and volume (Vn) are given by:
An = (2 * π^(n/2)) / Γ(n/2)
Vn = (π^(n/2)) / Γ((n/2) + 1)
where Γ denotes the gamma function.
To find the value of j - i, we need to identify the positive integers i and j such that Ai > Ak for all k not equal to i, and Vj > Vk for all k not equal to j.
First, let's analyze the relationship between An and Vn. By comparing the formulas, we can see that:
An / Vn = [(2 * π^(n/2)) / Γ(n/2)] / [(π^(n/2)) / Γ((n/2) + 1)]
= 2 / [n * (n-1)]
From this equation, we can deduce that An / Vn > 1 if and only if 2 > n * (n-1).
To find the positive integer i, we need to identify the highest positive integer n for which 2 > n * (n-1) holds true. We can observe that this condition is satisfied for n = 2. Therefore, i = 2.
Now, let's find the positive integer j. We need to identify the lowest positive integer n for which 2 > n * (n-1) does not hold true. We can observe that this condition is no longer satisfied for n = 3. Therefore, j = 3.
Finally, we can calculate j - i as follows:
j - i = 3 - 2 = 1
Therefore, j - i equals 1.
To know more about integer visit-
brainly.com/question/32066988
#SPJ11
find the distance between the two points rounding to the nearest 10th! PLS HELP ASAP!!!!

Answers
Answer:
8.5
Step-by-step explanation:
d = √[(-7+1)²+(-1-5)²]
= √[(-6)²+(-6)²]
=√(36+36)
=√72
= 8.5
Answer:
8.5
Step-by-step explanation:
we can plug those coordinates into the distance formula
d = √(x2 - x1)2 + (y2 - y1)2
d= √((-7)-(-1)^2+((-1)-(5)^2
d = √36+36
d=√72 = 8.48 or 8.5
researchers recruited over 1{,}0001,0001, comma, 000 patients with chronic back pain to participate in a study about the benefits of acupuncture. each subject was randomly assigned to one of these treatment plans: traditional chinese acupuncture, placebo acupuncture, and conventional pain treatment (medication and physical therapy). what is the primary purpose of randomly assigning subjects to the different treatment plans?
Answers
Accupunture is best these days for medication and health therapy.
What is accupunture.?Acupuncture is a traditional medical practice in which a doctor inserts needles into certain body parts of a patient. The earliest documents date back at least 2,500 years. [1] "Acupuncture points" are locations on the body where tiny metal needles are inserted. It is a type of complementary medicine[2], and it is fundamental to Traditional Chinese medicine[3] (TCM). [4] It makes use of the yin and yang principle.It is debatable and has yielded conflicting findings to research acupuncture using the concepts of "evidence-based medicine" (science research). [9] Although the majority of research indicates that acupuncture's effects are primarily attributable to placebo, certain studies indicate that acupuncture can reduce pain. [10] There is evidence that any advantages of acupuncture are transient. [11] When compared to conventional medical therapies, there is inadequate evidence to justify the use of acupuncture. [12] In the long run, acupuncture is not more effective than conventional medicine. [13]Learn more about accupunture click here:
https://brainly.com/question/452258
#SPJ4
given r (x)=-2(x+12)2 +7, what is the value of r (-5)
Answers
r(-5)=-28 + 7
r(-5)= -21
Factor of 3x-3a+xy-ay
Answers
Answer:
(3 - y) & (x - a) are the factors
Step-by-step explanation:
3x-3a+xy-ay
=> 3x - xy - 3a - ay
=> x(3 - y) - a(3 - y)
=> (3 - y)(x - a)
(3 + y) & (x - a) are the factors of the expression 3x-3a+xy-ay.
Here, we have,
given that,
the expression is : 3x-3a+xy-ay.
now, we have,
To factor the expression 3x - 3a + xy - ay, we can group the terms as follows:
3x-3a+xy-ay
=> (3x - 3a) + (xy - ay)
Now, let's factor out the common factors from each group:
=> 3(x - a) + y(x - a)
Notice that both terms now have a common factor of (x - a).
We can factor out this common factor:
=> (x - a)(3 + y)
Therefore, the factored form of the expression 3x - 3a + xy - ay is (x - a)(3 + y).
Hence, (3 + y) & (x - a) are the factors of the expression 3x-3a+xy-ay.
To learn more on Expression click:
brainly.com/question/14083225
#SPJ2
Which expressions simplify to 0? Check all that apply.
8g – 8g
(–2g) – 2g
5g + (–5g)
One-half g + one-half g
Negative two-thirds g + two-thirds g
Answers
The expressions that simplify to zero are:
8g – 8g
5g + (–5g)
Negative two-thirds g + two-thirds g
Let us consider each of the expressions to determine the ones that simplify to zero (0)
For 8g - 8g
This is a direct subtraction of terms of the same value. Therefore, 8g - 8g = 0
For (–2g) – 2g
This can be further simplified as -2g - 2g = -4g
Therefore, (–2g) – 2g does not simplify to zero
For One-half g + one-half g
This can be written mathematically as:
\(\frac{1}{2}g + \frac{1}{2}g = g\)
Therefore, One-half g + one-half g does not simplify to zero
Negative two-thirds g + two-thirds g
\(-\frac{2}{3}g+ \frac{2}{3}g = 0\)
Therefore, Negative two-thirds g + two-thirds g simplifies to zero
Learn more here: https://brainly.com/question/25702685
Answer:
A , C , E :)
Step-by-step explanation:
help please!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!

Answers
Answer:
24 cm²
Step-by-step explanation:
The area of a rhombus is half the product of its diagonals.
\(\boxed{\sf Area\;of\;a\;rhombus=\dfrac{diagonal\;1 \cdot diagonal\;2}{2}}\)
Therefore, to find the area of the rhombus, we need to find the lengths of the diagonals AC and BD.
The diagonals of a rhombus are perpendicular bisectors of each other.
The point of intersection of the diagonals of rhombus ABCD is point O.
We can use the given information to find the lengths of BO and OC, then double these to find diagonals AC and BD.
The sides of a rhombus are equal in length. Therefore, if the perimeter of rhombus ABCD is 20 cm, each side length is 5 cm.
Therefore, the hypotenuse of right triangle BOC is BC = 5 cm.
If the ratio of AC : BD = 4 : 3, then the ratio of OC : BO = 2 : 1.5.
Let OC = 2x and BO = 1.5x.
Use Pythagoras Theorem to find the value of x.
\(\begin{aligned}BO^2+OC^2&=BC^2\\(1.5x)^2+(2x)^2&=5^2\\1.5^2x^2+2^2x^2&=25\\2.25x^2+4x^2&=25\\6.25x^2&=25\\x^2&=4\\\sqrt{x^2}&=\sqrt{4}\\x&=2\end{aligned}\)
Therefore, to find the lengths of OC and BO, substitute x = 2:
\(OC = 2x = 2(2) = 4\; \sf cm\)
\(BO = 1.5x = 1.5(2) = 3\; \sf cm\)
As the diagonals of a rhombus bisect each other:
\(AC = 2\cdot OC=2 \cdot 4 = 8\; \sf cm\)
\(BD = 2\cdot BO=2 \cdot 3 = 6\; \sf cm\)
Finally, substitute the lengths of the diagonals into the formula for the area of a rhombus:
\(\begin{aligned}\textsf{Area of rhombus $ABCD$}&=\sf \dfrac{diagonal\;1 \cdot diagonal\;2}{2}}\\\\&= \dfrac{AC \cdot BD}{2}\\\\&=\dfrac{8 \cdot 6}{2}\\\\&=\dfrac{48}{2}\\\\&=24\; \sf cm^2 \end{aligned}\)
Therefore, the area of rhombus ABCD is 24 cm².
Pls help it due today

Answers
Answer:
B
Step-by-step explanation:
Answer: Choice C. y = (-1/2)x+7
=========================================================
Explanation:
The slope of the graphed line is -1/2 because we drop down 1 unit on the y axis each time we move to the right 2 units along the x axis. We could apply the slope formula for any two points on the diagonal line to also get a slope of -1/2.
The slope formula is m = (y2-y1)/(x2-x1).
Any two parallel lines have the same slope, but different y intercepts. So the parallel line will also have slope -1/2. Right away we can see the answer must be choice C. This is the only equation with slope -1/2 of the choices given.
Recall that slope intercept form y = mx+b has m as the slope. Not to be confused with the label m of the graphed line.
Note how plugging x = 6 into the equation for choice C leads to...
y = (-1/2)*x + 7
y = (-1/2)*6 + 7
y = -3 + 7
y = 4
Showing that (x,y) = (6,4) is on the equation y = (-1/2)x + 7. This confirms the answer.
---------------------------
Let's say we didn't have multiple choice answers to pick from, and instead it was fill in the blank. To find the y intercept b, we can do the following
y = mx+b
y = (-1/2)x + b ... plug in given slope m = -1/2
4 = (-1/2)*6 + b .... plug in the given point (x,y) = (6,4)
4 = -3 + b
4+3 = -3+b+3 ... adding 3 to both sides
7 = b
b = 7
Knowing that m = -1/2 and b = 7, we can update y = mx+b into y = (-1/2)x+7
Suppose babies born in a large hospital have a mean weight of 3685 grams, and a variance of 330,625. If 113 babies are sampled at random from the hospital, what is the probability that the mean weight of the sample babies would be less than 3631 grams
Answers
The probability that the mean weight of the sample babies would be less than 3631 grams is 0.1591 or 15.91%.
The mean weight of the babies (μ) = 3685 grams.
The variance (σ²) = 330625.
Therefore, the standard deviation (σ) = √330625 = 575.
The sample size (n) = 113.
The sample mean = μ - 3685 grams.
The sample standard deviation (s) = σ/√n = 575/√113 = 54.09145.
We are asked to find the probability that the mean weight of the sample babies is less than 3631 grams, that is,
P(X < 3631) = P(Z < {(3631 - 3685)/54.09145})
Using the formula: Z = (x - μ)/s,
or, P(Z < -0.9983) = 0.1591 or 15.91%.
From the table of area under the z-score.
P(X < 3631) can also be calculated using calculator function:
Normalcdf(-100000000,3631,3685,54.0195), which gives the value 0.1591 or 15.91%.
Thus, the probability that the mean weight of the sample babies would be less than 3631 grams is 0.1591 or 15.91%.
Learn more about the probability of sampling distributions at
https://brainly.com/question/15291567
#SPJ4
The picture shows 3 steel rods. The rods are congruent, solid cylinders. The area of the top of the one rod is 7.07 square centimeters. The area of the body of one rod is 39.58 square centimeters. Find the total surface area of the 3 rods.
a) 53.72
b) 46.65
c) 139.95
d) 161.16

Answers
Answer: D, 161.16 square centimeters.
Step-by-step explanation:
The surface area of a cylinder (shape of each steel rod) is given by this formula:
\(A = 2\pi rh + 2\pi r^2\), where the first 2*pi*r*h measures the area of the curved surface while the second 2*pi*r^2 measures the area of the two circles on the top and bottom.
We are given the area of the top of one rod, which is 7.07 square centimeters.
We are also given the area of the body of one rod, which is 39.58 square centimeters.
The top of one rod is the shape of a circle, and we know there are two circles on both sides of a cylinder, so the surface area of both circular sides are 7.07.
The total surface area of a cylinder is the body plus both circular ends, so the surface area for this cylinder is:
39.58 + 7.07 + 7.07 = 53.72
There are three cylinders, so multiply this value by three to get the answer, 161.16 square centimeters.
a polynomial function p(x) with rational coefficients has the given roots (10-square root of 6 and 4i). Find two additional roots of p(x)=0
Answers
Answer:
6px
Step-by-step explanation:
hope this help
A water balloon is thrown upward from a height of 5 feet with an initial velocity of 35 feet per second. The quadratic function \large h\left(t\right)=-16t^2+35t+5 represents the height of the balloon, h, in feet t seconds after it is thrown. When does the water balloon reach the height of 20 feet? round your answer to the nearest thousandth.
Answers
Since, The equation of the height with the time is provided and the balloon is thrown upwards against gravity from 5 feet, The answer is 0.255 sec.
What do you mean by equation?A mathematical equation is a formula that uses the equals sign to represent the equality of two expressions. The definition of an equation in algebra is a mathematical statement that demonstrates the equality of two mathematical expressions. For instance, the equation 3x + 5 = 14 consists of the two equations 3x + 5 and 14, which are separated by the 'equal' sign.
What is initial and final velocity?When gravity first exerts force on an object, its initial velocity defines how quickly the object moves. The final velocity, on the other hand, is a vector number that gauges a moving body's speed and direction after it has reached its maximum acceleration.
initial height = 5
final height =20
height to travel =20-5 =15
\(15 = 16t^{2}+35t+5\\16t^{2}+35t-10 =0\)
solving we get, t = 0.255 or -2.44
since, -2.44 is not possible,
t = 0.255 seconds.
To learn more about motion visit:
https://brainly.com/question/27102563
#SPJ4
Find the exact length of the curve.y = 3 + 4x^3/2, 0 ≤ x ≤ 1
Answers
The exact length of the curve. y = 3 + 4x^3/2, 0 ≤ x ≤ 1 is L = ∫√(9+108x-144x^(5/2)) / √(9-16x^3) dx, from 0 to 1.
To find the length of the curve, we need to use the arc length formula:
L = ∫√(1+(dy/dx)^2) dx, where y = 3 + 4x^(3/2) and 0 ≤ x ≤ 1.
First, we need to find dy/dx:
dy/dx = (12x^(1/2))/2√(3 + 4x^(3/2))
dy/dx = 6x^(1/2)/√(3 + 4x^(3/2))
Now, we can substitute this into the arc length formula:
L = ∫√(1+(6x^(1/2)/√(3 + 4x^(3/2)))^2) dx, from 0 to 1.
Simplifying the inside of the square root, we get:
L = ∫√(1+(36x)/(3 + 4x^(3/2))) dx, from 0 to 1.
We can simplify this further by multiplying the numerator and denominator of the fraction by (3 - 4x^(3/2)):
L = ∫√(1+36x(3-4x^(3/2))/(9-16x^3)) dx, from 0 to 1.
Expanding the numerator, we get:
L = ∫√((9+108x-144x^(5/2))/(9-16x^3)) dx, from 0 to 1.
Simplifying the expression under the square root, we get:
L = ∫√(9+108x-144x^(5/2)) / √(9-16x^3) dx, from 0 to 1.
We can evaluate this integral using numerical methods, such as Simpson's rule or the trapezoidal rule, to get an approximation of the length of the curve. The exact length of the curve cannot be expressed in a finite number of terms, but it can be approximated to any desired degree of accuracy using numerical methods.
To learn more about trapezoidal rule click here
brainly.com/question/31426104
#SPJ11
Write the equation g(x) in vertex form of a
quadratic function for the transformations given
the function f(x) = x2.
Let g(x) be the function whose graph is a
translation 4 units left and 1 unit up of the
graph of f(x).
Answers
The equation g(x) in vertex form of a quadratic function for the transformations whose graph is a translation 4 units left and 1 unit up of the graph of f(x) is (x-4)² + 1
Given a quadratic function for the transformations given the function f(x) = x²
If the function g(x) of the graph is translated 4 units to the left, the equation becomes (x-4)² (note that we subtracted 4 from the x value
Translating the graph 1 unit up will give the final function g(x) as (x-4)² + 1 (We added 1 since it is an upward translation.)Hence the equation g(x) in vertex form of a quadratic function for the transformations whose graph is a translation 4 units left and 1 unit up of the graph of f(x) is (x-4)² + 1
Learn more here: https://brainly.com/question/15381183
How do you solve a 3 system of equations with 3 variables?
Answers
One way to solve a system of 3 equations with 3 variables is to use Gaussian elimination or Gauss-Jordan elimination method.
Gaussian elimination method:
Write the augmented matrix of the system of equations.Use row operations to transform the matrix into an upper triangular matrix.Use back substitution to find the values of the variables.The steps for Gaussian elimination are as follows:
Write the system of equations in matrix form, where the coefficients of the variables are in the matrix and the constants are in the column vector on the right side.Use row operations to transform the matrix into an upper triangular matrix. These operations include adding or subtracting multiples of one row to another, and multiplying or dividing a row by a non-zero scalar.Use back substitution to find the values of the variables. Starting with the last equation, substitute the known values of the variables into the other equations to find the value of the next variable. Repeat until all variables are known.Gauss-Jordan elimination method is similar to Gaussian elimination, however in the final step, it converts the matrix into a Reduced Row Echelon Form (RREF) which leads to more easily solving the system of equations.
Learn more about Gauss-Jordan elimination method here:
https://brainly.com/question/12090959
#SPJ4
To make 5 blueberry pies,Mindy needs about 2 pounds of blueberries. How many pounds of blueberries does Mindy need to make 20 blueberry pies?
Answers
Answer:
so 5 pies is 2 pounds so 20 pies is 8 lbs of blueberries
Step-by-step explanation:
5 pies is 2 lbs
10 is 4 lbs
15 is 6 lbs
20 is 8 lbs of blueberries
if 5+2t-3=6 what is the value of t
Answers
Answer:
t = 2
Step-by-step explanation:
5+2t-3=6
2t + 2 = 6
2t = 6 - 2
2t = 4
t = 4/2
t = 2
Answer:
T = 2
Step-by-step explanation:
5 + 2T - 3 = 6
2 + 2T = 6
2T = 4
T = 2
Could someone help me and explain?

Answers
Answer:
length 3 inwidth 2 inStep-by-step explanation:
Since none of the answer choices match the drawing of the gardener, we assume the question is referring to the drawing of the partner.
The gardener's drawing is 1/4 of actual size. So, in terms of the gardener's drawing, actual size is ...
gardener's drawing = (1/4)actual size
actual size = 4(gardener's drawing)
__
The partner's drawing is 1/20 of actual size, so is ...
partner's drawing = actual size/20 = (4(gardener's drawing))/20
partner's drawing = (4/20)(gardener's drawing)
partner's drawing = (gardener's drawing)/5
__
Then the {length, width} of the partner's drawing are ...
partner's drawing {length, width} = {15 in, 10 in}/5 = {3 in, 2 in}
The partner's drawing has a length of 3 inches and a width of 2 inches.

Here is a triangle. Find cos(A), sin(A), and tan(A). Explain your reasoning.

Answers
Answer:
\(\cos(A)=\dfrac{3\sqrt{10}}{10}\)
\(\sin(A)=\dfrac{\sqrt{10}}{10}\)
\(\tan(A)=\dfrac13\)
Step-by-step explanation:
Calculate the hypotenuse of ΔABC using Pythagoras' Theorem: \(a^2+b^2=c^2\) (where a and b are the legs and c is the hypotenuse of a right triangle)
Given:
a = 2b = 6\(\implies 2^2+6^2=c^2\\\\\implies 4 + 36 = c^2\\\\\implies c^2=40\\\\\implies c=\sqrt{40} \\\\\implies c=2\sqrt{10}\)
Trig ratios:
\(\sin(\theta)=\dfrac{O}{H} \ \ \ \ \cos(\theta)=\dfrac{A}{H} \ \ \ \ \tan(\theta)=\dfrac{O}{A}\)
where \(\theta\) is the angle, O is the side opposite the angle, A is the side adjacent the angle, H is the hypotenuse in a right triangle.
\(\cos(A)=\dfrac{6}{2\sqrt{10} }=\dfrac{3\sqrt{10}}{10}\)
\(\sin(A)=\dfrac{2}{2\sqrt{10} }=\dfrac{\sqrt{10}}{10}\)
\(\tan(A)=\dfrac{2}{6}=\dfrac13\)
From a hot-air balloon, Violet measures a 27^{\circ} ∘ angle of depression to a landmark that’s 790 feet away, measuring horizontally. What’s the balloon’s vertical distance above the ground? Round your answer to the nearest tenth of a foot if necessary.
Answers
The balloon’s vertical distance above the ground is 402.53 ft
How to solve for the vertical distanceTwo legs of a right triangle are formed by the horizontal and the vertical. The tangent relation is applicable because the provided angle is the angle next to the horizontal leg:
TOA = Tangent Opposite Adjacent
Tangent = opposite / adjacent
cross multiply
opposite = tan * adjacent
The tangent is 27 degrees
The adjacent is 790 feet away
= tan 27 degrees * 790
= 402.53
This balloon can be said to be 402.53 ft above the ground.
Read more on distance here: https://brainly.com/question/24834665
#SPJ1
Answer:
402.5
Step-by-step explanation:
I got the question right
Question Andre bought 8 bagels and ate 2/3 of them. Sandra bought 20 bagels and ate 1/4 of them. Who ate more bagels?
Answers
Answer:
Andre
Step-by-step explanation:
Andre ate 2/3 of 8 bagels, so to get the number of bagels-
8 *2/3
8/1*2/3 = 16/3 = 5 1/3
Meanwhile Sandra ate 1/4 of 20, so,
20*1/4 = 20/4 = 5
A bag contains tiles. 3 tiles of red, 6 tiles of green, 3 tiles are blue. A tile will be randomly selected from the bag. What is the probability In decimal form from the tile will be green?
Answers
Answer:
The probability is 0.5 tiles will be green
Step-by-step explanation:
Hope this helps
if each of seven persons in a group shakes hands with each of the other six persons, then a total of forty-two handshakes occurs.
A. True
B. False
Answers
Answer:
B. False
Step-by-step explanation:
You want to know if it is true that when each of seven persons in a group shakes hands with each of the other six persons, a total of forty-two handshakes occurs.
HandshakesThe count of 42 handshakes includes every one of 7 shaking hands with each of the remaining 6, without regard to whether they met before.
The count will include A shaking hands with B, and B shaking hands with A. That handshake will be counted twice, so the number 42 overstates the actual number of handshakes by a factor of 2.
It is false that there will be 42 handshakes. (There will be only 21.)