Answers
Step-by-step explanation:
\( \cos( \beta ) = \frac{5}{13} = 0.385\)
Cos (B) in the right angled triangle is 5/13 .
Given,
Right angled triangle .
Now,
In right angled triangle
Perpendicular = 12
Base = 5
Hypotenuse = 13
So,
According to trigonometric functions,
CosФ = base / hypotenuse
sinФ = perpendicular / hypotenuse
tanФ = perpendicular / base
So,
Substitute the value in the function of cos,
Cos(B) = base / hypotenuse
Cos(B) = 5 / 13
Thus the value of function cos (B) is 5/13 .
Know more about trigonometric functions,
https://brainly.com/question/25618616
#SPJ2
Related Questions
Write and solve a system of equations to answer the question. Check your solution.
At a food court, Pedro and his friends buy 3 pretzels and 2 drinks for a total cost of $5.97. They return
to the same food court and buy 12 more pretzels and 6 more drinks for a total cost of $20.88.
What is the cost of 1 pretzel, and what is the cost of 1 drink?
Answers
Answer: Let the cost of one pretzel be $p and the cost of one drink be $d. We can create a system of two equations based on the given information:
3p + 2d = 5.97 (equation 1)
12p + 6d = 20.88 (equation 2)
To solve this system, we can use the elimination method. First, we can multiply equation 1 by 3 to eliminate p:
9p + 6d = 17.91 (equation 3)
Next, we can subtract equation 3 from equation 2 to eliminate p:
3p = 2.97
p = 0.99
Now we can substitute p = 0.99 into equation 1 or equation 2 to solve for d. Let's use equation 1:
3(0.99) + 2d = 5.97
2d = 2.97
d = 1.485
Therefore, the cost of one pretzel is $0.99 and the cost of one drink is $1.485. To check our solution, we can substitute these values into equation 2:
12(0.99) + 6(1.485) = 20.88
This is true, so our solution is correct.
Step-by-step explanation: :)
in in the bending rheometer = 0.4mm, 0.5mm, 0.65mm, 0.82mm,
0.98mm, and 1.3mm for t = 15s, 30s, 45s, 60s, 75s, and 90s, what
are the values of S(t) and m. Does this asphalt meet PG grading
requirement
Answers
It is given that PG grading requirement is met if the values of S(t) are between -3.2 and +3.2. As all the calculated values of S(t) lie within this range, the asphalt meets PG grading requirement.
Given data: Bending rheometer: 0.4mm, 0.5mm, 0.65mm, 0.82mm, 0.98mm, and 1.3mm for t = 15s, 30s, 45s, 60s, 75s, and 90s.
We are supposed to calculate the values of S(t) and m to check if the asphalt meets PG grading requirement.
Calculation of m:
Mean wheel track rut depth = (0.4+0.5+0.65+0.82+0.98+1.3)/6
= 0.7933mm
Calculation of S(t)
S(t) = (x - m)/0.3
Where, x = 0.4mm, 0.5mm, 0.65mm, 0.82mm, 0.98mm, and 1.3mm
Given, m = 0.7933mm
Substituting these values into the formula above:
S(15s) = (0.4 - 0.7933)/0.3
= -1.311S(30s)
= (0.5 - 0.7933)/0.3
= -0.9777S(45s)
= (0.65 - 0.7933)/0.3
= -0.4777S(60s)
= (0.82 - 0.7933)/0.3
= 0.128S(75s)
= (0.98 - 0.7933)/0.3
= 0.62S(90s)
= (1.3 - 0.7933)/0.3
= 1.521
It is given that PG grading requirement is met if the values of S(t) are between -3.2 and +3.2. As all the calculated values of S(t) lie within this range, the asphalt meets PG grading requirement.
To know more about range visit
https://brainly.com/question/29463327
#SPJ11
find the critical value(s) and rejection region(s) for the type of z-test with level of significance . include a graph with your answer. right-tailed test, a=0.03.
Answers
Answer:
c
Step-by-step explanation:
The red line represents the critical value, and the shaded region on the right-hand side of the red line represents the rejection region. If the calculated test statistic is greater than the critical value of z, which is 1.88 in this case, we will reject the null hypothesis.
The critical value(s) and rejection region(s) for the type of z-test with a level of significance a = 0.03 and a right-tailed test are as follows :Step 1: Determine the critical value of zThe critical value is calculated by using the normal distribution table and the level of significance. A right-tailed test will have a critical value of zα. For a level of significance of 0.03, we will look for the z-value that corresponds to 0.03 in the normal distribution table.Critical value for a = 0.03 is z = 1.88 (approx).Step 2: Determine the Rejection Region The rejection region for a right-tailed test is defined as any z-value that is greater than the critical value. That is, if the test statistic is greater than 1.88, we reject the null hypothesis at the 0.03 level of significance, and if it is less than or equal to 1.88, we fail to reject the null hypothesis.Therefore, the rejection region for a right-tailed test with a level of significance of 0.03 is as follows:Rejection Region: Z > 1.88 OR Z ≤ -1.88Graph: The graph for the given values will be as follows:The red line represents the critical value, and the shaded region on the right-hand side of the red line represents the rejection region. If the calculated test statistic is greater than the critical value of z, which is 1.88 in this case, we will reject the null hypothesis.
To know more about critical value Visit:
https://brainly.com/question/32607910
#SPJ11
Jeremy has volleyball practice every third day in soccer practice every 5th day today he had both practices and how many days will he have both practices on the same day again
Answers
Answer:
He will have it on the 15th day
George earns $455 per week. George receives a 20% raise. How can George calculate his new weekly pay rate? Select all calculations that will result in George's new weekly pay rate. divide $455 by 0.20 divide $455 by 1.20 multiply $455 by 0.20 multiply $455 by 1.20 solve for x: x/455 = 120/100 solve for x: 455/x = 20/100
Answers
Answer:
To calculate George's new weekly pay rate after a 20% raise, we can use the following formula:
New weekly pay rate = Old weekly pay rate + (Old weekly pay rate x Percent raise)
Or, mathematically:
New weekly pay rate = 455 + (455 x 0.20)
Simplifying the calculation:
New weekly pay rate = 455 + 91
New weekly pay rate = 546
Therefore, George's new weekly pay rate is $546.
Out of the given options, the calculations that will result in George's new weekly pay rate are:
multiply $455 by 1.20
solve for x: x/455 = 120/100 (this is equivalent to multiplying $455 by 1.20)
Please please help meeeee

Answers
We get the Polynomials quotient and remainder as 2x+7 and 5 respectively.
What are Polynomials ?An expression that consists of variables, constants, and exponents that is combined using mathematical operations like addition, subtraction, multiplication, and division is referred to as a polynomial.
A polynomial is an equation made up of coefficients and indeterminates that uses only the addition, subtraction, multiplication, and powers of positive-integer variables.
Polynomial long division is an extended variant of the well-known mathematical operation known as long division. It is an algorithm for dividing a polynomial by another polynomial of the same or lower degree. Due to the fact that it breaks down a more difficult division issue into simpler ones, it may be completed quickly by hand.
The polynomials are
2X^2 - X +16
and
x - 3
Dividing by 2X^2 - X +16 BY x-3 = 2x + 7
We get the quotiant and remainder as 2x+7 and 5 respectively.
To learn more about Polynomials refer to :
brainly.com/question/29123928
#SPJ1
Simplify.
7 x – 4 + x
—————-
2x – 1
6x - 5
8x - 4
———
2x – 1
4x - 4

Answers
really stuck on this question idrk why.. can anyone help me?
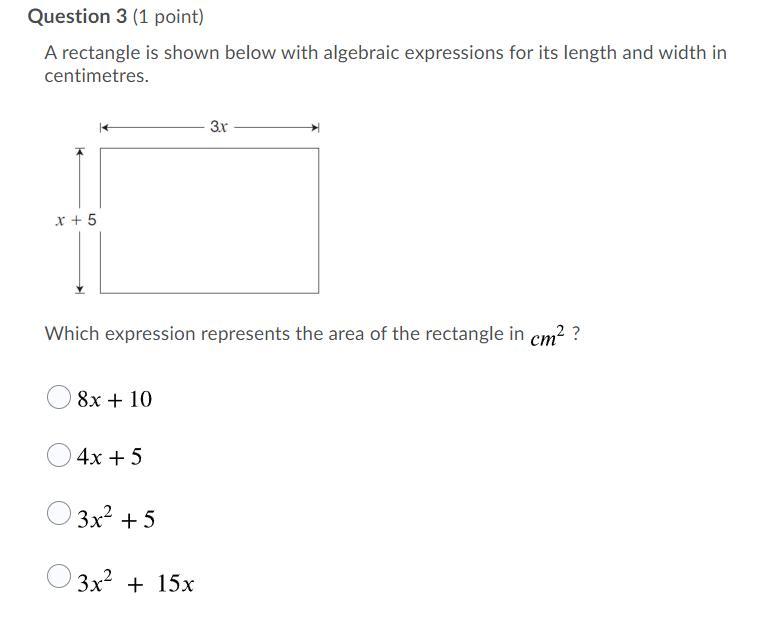
Answers
Answer:
i think its C) 3x^2 + 5
Step-by-step explanation:
because it matches it matched the rectangle, I'm so sorry if this is wrong I tried my best good luck!!
Answer:
3x^2 + 15x
Step-by-step explanation:
area = length x width
a = 3x(x + 5)
a = 3x^2 + 15x
Kate places greeting cards from two different companies on a display rack that can hold up to 90 cards. She
has agreed to display at least 40 of company a's cards on the rack and at least 25 of company b's cards.
kate makes a profit of $0. 30 on each card she sells from company a and $0. 32 on each card she sells from
company b.
Answers
To get the maximum profit, Kate should display as many cards from company B as possible, since she makes a higher profit from those cards.
Let x be the number of cards from company A and y be the number of cards from company B.
The constraints are:
x + y ≤ 90 (the display rack can hold up to 90 cards) x ≥ 40 (at least 40 of company A's cards must be displayed) y ≥ 25 (at least 25 of company B's cards must be displayed)The objective function is:
P = 0.30x + 0.32y (the profit from selling the cards)
To maximize the profit, we need to maximize the value of y. Since the display rack can hold up to 90 cards, we can set y = 90 - x.
Substituting this into the objective function:
P = 0.30x + 0.32(90 - x)
P = 0.30x + 28.8 - 0.32x
P = -0.02x + 28.8
To maximize P, we need to minimize x. Since x must be at least 40, we can set x = 40.
Substituting this back into the objective function:
P = -0.02(40) + 28.8
P = 28
So the maximum profit Kate can make is $28, by displaying 40 cards from company A and 50 cards from company B.
Learn more about profit margin at https://brainly.com/question/28180283
#SPJ11
According to lecture, ___________ refers to an unequal distribution of rewards between men and women, reflecting their different positions in a social hierarchy.
Answers
According to the lecture, the term that refers to an unequal distribution of rewards between men and women, reflecting their different positions in a social hierarchy, is gender inequality.
Gender inequality is a concept that highlights the disparities and imbalances in the treatment, opportunities, and rewards experienced by individuals based on their gender. It acknowledges that women and men occupy different positions in social, economic, and political structures, leading to unequal distribution of resources, power, and privileges. In the context of the lecture, gender inequality specifically refers to the differential allocation of rewards, such as income, career opportunities, education, and social status, between men and women.
This unequal distribution of rewards can manifest in various forms, including gender pay gaps, limited access to leadership positions, underrepresentation of women in decision-making roles, and societal interest and stereotypes that perpetuate gender-based discrimination.
Learn more about interest here:
https://brainly.com/question/30955042
#SPJ11
factorize:-
x³–3x²–9x–5
no links or spam answers please!
Answers
Answer:
X³ – 3x² – 9x – 5
= x³ + x² – 4x² – 4x – 5x – 5
= x²( x + 1) – 4x ( x + 1) – 5(x + 1)
= (x + 1)(x² – 4x – 5)
= (x + 1)(x² -5x + x – 5)
= (x + 1)(x – 5) (x + 1)
hence, (x +1), (x -5) and (x +1) are the factors of given polynomial .
Was this answer helpful?
please with answer and explanation!!

Answers
Subtract the height of the dive from
The total distance he dove:
15 - 9 = 6
The pool is 6 meters deep
Solve each equation. 4 y-6=2 y+8
Answers
The solution of the linear equation in one variable 4y - 6 = 2y + 8 is at y = 7.
According to the given question.
We have a linear equation in one variable.
4y - 6 = 2y + 8
As we know that, the linear equations in one variable is an equation which is expressed in the form of ax+b = 0, where a and b are two integers, and x is a variable and has only one solution.
Thereofre, the solution of the linear equation in one variable 4y - 6 = 2y + 8 is given by
4y - 6 = 2y + 8
⇒ 4y - 2y - 6 = 8 ( subtracting 2y from both the sides)
⇒ 2y -6 -8 = 0 (subtracting 8 from both the sides)
⇒ 2y - 14 = 0
⇒ 2y = 14
⇒ y = 14/2
⇒ y = 7
Hence, the solution of the linear equation in one variable 4y - 6 = 2y + 8 is at y = 7.
Find out more information about equation here:
https://brainly.com/question/28583552
#SPJ4
how do I Translate the following verbal statement into an algebraic equation and then solve: Krystal bought a nightstand on sale for $170, which was one-fourth of the original price. What was the original price of the nightstand
Answers
Answer:the original price is $680
Step-by-step explanation:
1/4(x)=170
4×1/4(x)=4×170
x=$680
The original price of the nightstand is $680.
What is an equation?In mathematics, an equation is a formula that expresses the equality of two expressions, by connecting them with the equals sign =.
Given that, Krystal bought a nightstand on sale for $170, which was one-fourth of the original price.
Let the original price of nightstand be x.
Now, 1/4x =170
x=170×4
x=$680
Therefore, the original price of the nightstand is $680.
To learn more about an equation visit:
https://brainly.com/question/14686792.
#SPJ5
calculate the molecular weight of a gas with a density of 1.524 g/l at stp.
Answers
To calculate the molecular weight of a gas with a density of 1.524 g/l at STP, we can use the ideal gas law: PV = nRT. At STP, the pressure (P) is 1 atm, the volume (V) is 22.4 L/mol, and the temperature (T) is 273 K. The molecular weight of the gas with a density of 1.524 g/L at STP is approximately 32.0 g/mol.
Rearranging the equation, we get n = PV/RT.
Next, we can calculate the number of moles (n) of the gas using the given density of 1.524 g/l. We know that 1 mole of any gas at STP occupies 22.4 L, so the density can be converted to mass by multiplying by the molar mass (M) and dividing by the volume: density = (M*n)/V. Rearranging the equation, we get M = (density * V) / n.
Substituting the given values, we get n = (1 atm * 22.4 L/mol) / (0.0821 L*atm/mol*K * 273 K) = 1 mol. Then, M = (1.524 g/L * 22.4 L/mol) / 1 mol = 34.10 g/mol. Therefore, the molecular weight of the gas is 34.10 g/mol.
To calculate the molecular weight of a gas with a density of 1.524 g/L at STP, you can follow these steps:
1. Recall the ideal gas equation: PV = nRT
2. At STP (Standard Temperature and Pressure), the temperature (T) is 273.15 K and the pressure (P) is 1 atm (101.325 kPa).
3. Convert the density (given as 1.524 g/L) to mass per volume (m/V) by dividing it by the molar volume at STP (22.4 L/mol). This will give you the number of moles (n) per volume (V):
n/V = (1.524 g/L) / (22.4 L/mol)
4. Calculate the molar mass (M) of the gas using the rearranged ideal gas equation, where R is the gas constant (8.314 J/mol K):
M = (n/V) * (RT/P)
5. Substitute the values and solve for M:
M = (1.524 g/L / 22.4 L/mol) * ((8.314 J/mol K * 273.15 K) / 101325 Pa)
6. Calculate the molecular weight of the gas:
M ≈ 32.0 g/mol
Therefore, the molecular weight of the gas with a density of 1.524 g/L at STP is approximately 32.0 g/mol.
Learn more about molecular weight at: brainly.com/question/27988184
#SPJ11
work out the curved surface area of this cylinder to 1d.p.
Answers
The curved surface area of a cylinder with radius of 7 cm and height of 15 cm is 259.7 cm²
What is an equation?An equation is an expression that shows how two numbers and variables are related using mathematical operations such as addition, subtraction, exponent, division and multiplication.
The curved surface area of a cylinder is:
Curved surface area = 2π * radius * height
From the image:
Radius = 7 cm, height = 15 cm, therefore:
Curved surface area = 2π * 7 cm * 15 cm = 259.7 cm²
The curved surface area is 259.7 cm²
Find out more on equation at: https://brainly.com/question/2972832
#SPJ1

A Farm stand sells two types of grapes the cost of green grapes can be represented by the equasion y=1.5x, where y is the total cost for x pounds. the graph represent the cost of black grapes. what statement must be true?
answers
a) three pounds of green grapes cost 6.00
b) two pounds of black grapes cost 3.00
c)black grapes cost less per pound than green grapes
d)black grapes cost more per pound the green grapes
Answers
Answer:
I dont know because their is no graph angel O stop looking up the answer
Step-by-step explanation:
ITs not A
find the area of ABCD with vertices A(4.-3) B(6.-3) C(9,-7) D(7,-7)
Answers
Total area of quadrilateral ABCD = (-4) + (-6) = -10 square units.
To find the area of quadrilateral ABCD, we can divide it into two triangles, calculate the area of each triangle, and then add them together.
Triangle ABC:
Using the coordinates of points A(4, -3), B(6, -3), and C(9, -7), we can calculate the base and height of triangle ABC. The base is the distance between points A and B, which is 6 - 4 = 2 units. The height is the vertical distance from point C to the line containing points A and B, which is the difference in y-coordinates between points C and the y-coordinate of points A or B. So, the height is -7 - (-3) = -4 units.
Area of triangle ABC = (1/2) * base * height = (1/2) * 2 * (-4) = -4 square units.
Triangle ACD:
Using the coordinates of points A(4, -3), C(9, -7), and D(7, -7), we can calculate the base and height of triangle ACD. The base is the distance between points A and D, which is 7 - 4 = 3 units. The height is the vertical distance from point C to the line containing points A and D, which is the difference in y-coordinates between points C and the y-coordinate of points A or D. So, the height is -7 - (-3) = -4 units.
Area of triangle ACD = (1/2) * base * height = (1/2) * 3 * (-4) = -6 square units.
Adding the areas of the two triangles:
Total area of quadrilateral ABCD = (-4) + (-6) = -10 square units.
Since the area is negative, it suggests that the points are not arranged in the correct order or the order of the vertices does not form a convex quadrilateral. Double-checking the order of the vertices may be necessary to ensure the correct area calculation.
For more such questions on quadrilateral
https://brainly.com/question/27991573
#SPJ8
If a and b are non-zero and non-parallel vectors such that λa+ub is parallel to ha+tb, where λ ,u, h, b €R and h is not 0, b is not 0, show that λ/h = u/t
Answers
Answer:
Step-by-step explanation:
Since λa + ub is parallel to ha + tb, we can write:
λa + ub = k(ha + tb)
where k is some constant. We can simplify this equation by expanding both sides:
λa + ub = kha + ktb
Rearranging the terms, we get:
λa - kha = ktb - ub
Factoring out a, we get:
a(λ - kh) = b(kt - u)
Since a and b are non-parallel, they are linearly independent, which means that a and b are not multiples of each other. This also means that the only way for the left side of the equation to be equal to 0 is if λ - kh = 0, or λ = kh. Similarly, the only way for the right side of the equation to be equal to 0 is if kt - u = 0, or u/t = k/h.
Therefore, we have shown that λ/h = u/t.
Hobart bus tickets have numbers from 000000 to 999999. The number on the first ticket for each
bus is random, with subsequent ticket numbers going up by one each time. In the rare event of
going past 999999, the next number is 000000. A lucky ticket is one where the sum of the digits is
21.
What is the average sum of the digits on a ticket?
Answers
The average sum of the digits on a ticket will be 15.78
How to calculate the averageThe first digit can be any number from 0 to 9, so its average value is (0+1+2+3+4+5+6+7+8+9)/10 = 4.5.
For the second digit, we can use the fact that the first digit is equally likely to be any of the numbers from 0 to 9. If the first digit is 0, the second digit can be any number from 0 to 9. So the average value of the second digit is (0+1+2+3+4+5+6+7+8+9)/10 + (0+1+2+3+4)/9 = 4.05.
Similarly, we can calculate the average value of the third digit as (0+1+2+3+4+5+6+7+8+9)/10 + (0+1+2+3+4+5)/9 + (0+1+2+3)/8 = 3.125.
Continuing in this way, we find that the average value of each digit position is:
First digit: 4.5
Second digit: 4.05
Third digit: 3.125
Fourth digit: 2.25
Fifth digit: 1.35
Sixth digit: 0.5
So the average sum of the digits on a ticket is 4.5 + 4.05 + 3.125 + 2.25 + 1.35 + 0.5 = 15.78
Learn more about average on;
https://brainly.com/question/20118982
#SPJ1
Hobart bus tickets have numbers from 0 to 9. The number on the first ticket for each
bus is random, with subsequent ticket numbers going up by one each time. In the rare event of
going past 9, the next number is 0. A lucky ticket is one where the sum of the digits is
21.
What is the average sum of the digits on a ticket?
diring the cold winters a lake near the srtic circle at the beggining of spring the icestarts to melt
Answers
Answer:
During winter in the Arctic, a lake gets frozen solid with ice. But as spring comes, the ice starts to melt. This happens because the temperature gets warmer and there's more sunlight. It's a natural process that happens every year and shows the change of seasons.
Step-by-step explanation:
A fair coin is tossed 5 times. Calculate the probability that (a) five heads are obtained (b) four heads are obtained (c) one head is obtained A fair die is thrown eight times. Calculate the probability that (a) a 6 occurs six times (b) a 6 never happens (c) an odd number of 6s is thrown.
Answers
To calculate the probabilities, we need to use the concept of binomial probability.
For a fair coin being tossed 5 times:
(a) Probability of getting five heads:
The probability of getting a head in a single toss is 1/2.
Since each toss is independent, we multiply the probabilities together.
P(Head) = 1/2
P(Tails) = 1/2
P(Five Heads) = P(Head) * P(Head) * P(Head) * P(Head) * P(Head) = \((1/2)^5\) = 1/32 ≈ 0.03125
So, the probability of obtaining five heads is approximately 0.03125 or 3.125%.
(b) Probability of getting four heads:
There are five possible positions for the four heads.
P(Four Heads) = (5C4) * P(Head) * P(Head) * P(Head) * P(Head) * P(Tails) = 5 * \((1/2)^4\) * (1/2) = 5/32 ≈ 0.15625
So, the probability of obtaining four heads is approximately 0.15625 or 15.625%.
(c) Probability of getting one head:
There are five possible positions for the one head.
P(One Head) = (5C1) * P(Head) * P(Tails) * P(Tails) * P(Tails) * P(Tails) = 5 * (1/2) * \((1/2)^4\) = 5/32 ≈ 0.15625
So, the probability of obtaining one head is approximately 0.15625 or 15.625%.
For a fair die being thrown eight times:
(a) Probability of a 6 occurring six times:
The probability of rolling a 6 on a fair die is 1/6.
Since each roll is independent, we multiply the probabilities together.
P(6) = 1/6
P(Not 6) = 1 - P(6) = 5/6
P(Six 6s) = P(6) * P(6) * P(6) * P(6) * P(6) * P(6) * P(Not 6) * P(Not 6) = \((1/6)^6 * (5/6)^2\) ≈ 0.000021433
So, the probability of rolling a 6 six times is approximately 0.000021433 or 0.0021433%.
(b) Probability of a 6 never happening:
P(No 6) = P(Not 6) * P(Not 6) * P(Not 6) * P(Not 6) * P(Not 6) * P(Not 6) * P(Not 6) * P(Not 6) = \((5/6)^8\) ≈ 0.23256
So, the probability of not rolling a 6 at all is approximately 0.23256 or 23.256%.
(c) Probability of an odd number of 6s:
To have an odd number of 6s, we can either have 1, 3, 5, or 7 6s.
P(Odd 6s) = P(One 6) + P(Three 6s) + P(Five 6s) + P(Seven 6s)
\(P(One 6) = (8C1) * P(6) * P(Not 6)^7 = 8 * (1/6) * (5/6)^7P(Three 6s) = (8C3) * P(6)^3 * P(Not 6)^5 = 56 * (1/6)^3 * (5/6)^5P(Five 6s) = (8C5) * P(6)^5 * P(Not 6)^3 = 56 * (1/6)^5 * (5/6)^3P(Seven 6s) = (8C7) * P(6)^7 * P(Not 6) = 8 * (1/6)^7 * (5/6)\)
P(Odd 6s) = P(One 6) + P(Three 6s) + P(Five 6s) + P(Seven 6s)
Calculate each term and sum them up to find the final probability.
After performing the calculations, we find that P(Odd 6s) is approximately 0.28806 or 28.806%.
Learn more about binomial probability here:
https://brainly.com/question/12474772
#SPJ11
how do u do thissss??
reflect this shape in the line y=x :)

Answers
Answer:
the x-coordinate and y-coordinate change places.
Step-by-step explanation: so you reflect a point across the line y = x, the x-coordinate and y-coordinate change places. If you reflect over the line y = -x, the x-coordinate and y-coordinate change places and are negated (the signs are changed). the line y = x is the point (y, x). the line y = -x is the point (-y, -x).
A soccer ball kicked with an initial velocity of 39 ft/sec and an angle of 44° with the ground. Find the parametric equations that model the motion. What was its maximum height?
step by step please asap
Answers
The soccer ball reaches a maximum height of approximately 34.8 feet.
How to solveThe parametric equations for the motion of the soccer ball are:
x(t) = 39*cos(44°)t ≈ 26.9t
y(t) = \(39\sin(44)*t - 16t^2\) ≈ \(22.7t - 16t^2\)
where t is the time elapsed since the ball was kicked.
To find the maximum height of the ball, we need to find the vertex of the parabolic trajectory given by y(t).
The maximum height occurs at the vertex, which is at the time t = -b/2a, where a = -16 and b = 39*sin(44°).
So, t = -b/2a ≈ 1.1 seconds.
Substituting this value of t into the equation for y(t), we get the maximum height:
y(max) = \(39*\sin(44)1.1 - 16(1.1)^2 = 34.8 feet.\)
Therefore, the soccer ball reaches a maximum height of approximately 34.8 feet.
Read more about height here:
https://brainly.com/question/1739912
#SPJ1
Use your intuition to decide whether the following two events are likely to be independent or associated.Event A: Drawing a club from a deck of cards.Event B: Drawing a card with a black symbol from a deck of cards.
Answers
Based on my intuition, I believe that the two events, drawing a club and drawing a card with a black symbol, are likely to be associated. This can be answered by the concept of Probability.
This is because clubs are always black symbols, and therefore the probability of drawing a club and the probability of drawing a black symbol are not independent of each other. In other words, if we know that a card is a club, then we also know that it is a black symbol.
Therefore, these two events are associated.
To learn more about Probability here:
brainly.com/question/30034780#
#SPJ11
What’s the answer for 350÷10*3
Answers
original price: $60 Markup: 15% Retail Price:??
Answers
Answer:
51
Step-by-step explanation:
Vladimir says that the equation of the line that passes through points (negative 5, negative 3) and (10, 9) is y = four-fifths x + 1. Robyn says that the line passes through the points (negative 10, negative 7) and (negative 15, negative 11). Who is correct?
only Vladimir
only Robyn
both Vladimir and Robyn
neither Vladimir nor Robyn
Answers
Answer:
C. both Vladimir and Robyn
Step-by-step explanation:
Just took the test. Hope this helps
Both Vladimir and Robyn are correct. Then the correct option is C.
What is the equation of a line passing through two points?Suppose the given points are (x₁, y₁) and (x₂, y₂), then the equation of the straight line joining both two points is given by
\(\rm (y - y_1) = \left [ \dfrac{y_2 - y_1}{x_2 - x_1} \right ] (x -x_1)\)
Vladimir says that the equation of the line that passes through points (-5, -3) and (10, 9) is y = (4/5) x + 1.
Robyn says that the line passes through the points (-10, -7) and (-15, -11).
Then the equation of the line will be
y + 7 = [(-11 + 7) / (-15 + 10)] (x + 10)
y + 7 = (4/5)x + 8
y = (4/5)x + 1
Both Vladimir and Robyn are correct.
Then the correct option is C.
Learn more about straight-line equations here:
https://brainly.com/question/380976
#SPJ2
find the eigenvalues and eigenbasis rotation about the e3-axis through an angle of 90◦, counterclockwise as viewed from the positive e3-axis in r3
Answers
The eigenvalues are λ1 = i and λ2 = -i, and the eigenvectors correspond to any vector along the e3-axis.
To find the eigenvalues and eigenvectors for a rotation about the e3-axis through an angle of 90 degrees counterclockwise, we can use the concept of rotation matrices.
A rotation of 90 degrees counterclockwise about the e3-axis can be represented by the following rotation matrix:
\(R= \left[\begin{array}{ccc}cos\theta&-sin\theta&0\\sin\theta&cos\theta&0\\0&0&1\end{array}\right]\)
In this case, θ = 90 degrees.
To find the eigenvalues, we need to solve the characteristic equation:
|A - λ| = 0
where A is the rotation matrix and λ is the eigenvalue.
Substituting the values of the rotation matrix, we have:
\(R= \left[\begin{array}{ccc}cos\theta&-sin\theta&0\\sin\theta&cos\theta&0\\0&0&1\end{array}\right] - \lambda \left[\begin{array}{ccc}1&0&0\\0&1&0\\0&0&1\end{array}\right] = 0\)
Simplifying the determinant, we get:
(cos(θ) - λ)(cos(θ) - λ) - (-sin(θ)sin(θ)) = 0
Expanding and simplifying further:
cos²(θ) - 2λcos(θ) + λ² + sin²(θ) = 0
Since cos²(θ) + sin²(θ) = 1, the equation simplifies to:
1 - 2λcos(θ) + λ² = 0
Plugging in θ = 90 degrees:
1 - 2λ(0) + λ² = 0
1 + λ² = 0
Solving for λ, we find two complex eigenvalues:
λ1 = i
λ2 = -i
The eigenvectors can be obtained by solving the equation:
(A - λI)v = 0
For each eigenvalue, substitute it back into the rotation matrix equation and solve for v.
For λ1 = i:
\(R= \left[\begin{array}{ccc}cos\theta&-sin\theta&0\\sin\theta&cos\theta&0\\0&0&1\end{array}\right] - i\left[\begin{array}{ccc}1&0&0\\0&1&0\\0&0&1\end{array}\right] v1 = 0\)
Simplifying, we have:
\(\left[\begin{array}{ccc}-i&0&0\\0&-i&0\\0&0&1\end{array}\right] v1= 0\)
From this, we can see that any vector v1 along the e3-axis will be an eigenvector corresponding to λ1 = i.
For λ2 = -i, the same result applies.
Therefore, the eigenvalues are λ1 = i and λ2 = -i, and the eigenvectors correspond to any vector along the e3-axis.
Visit to know more about Eigenvalue:-
brainly.com/question/13050052
#SPJ11
Which of the following sets has a median of 26?
7, 12, 17, 22, 31, 33, 35
0, 6, 15, 26, 26, 26
3, 8, 14, 16, 21, 31, 33, 33, 34, 34
14, 14, 18, 22, 25, 26, 26, 26, 27
Answers
Answer:
it is going to be 19
Step-by-step explanation:
(17+21)/2=19
Answer:
c. the 3rd one
Step-by-step explanation:
