axline computers manufactures personal computers at two plants, one in texas and the other in hawaii. the texas plant has 45 employees; the hawaii plant has 15. a random sample of 10 employees is to be asked to fill out a benefits questionnaire. (round your answers to four decimal places.) (a) what is the probability that none of the employees in the sample work at the plant in hawaii?
Answers
The probability that none of the employees in the sample work at the plant in hawai is 0.0112
Simply put, probability is the likelihood that something will occur. When we don't know how an event will turn out, we can discuss the likelihood or likelihood of several outcomes. Statistics is the study of events that follow a probability distribution.
PH= 20/60 = 1/3
P(T) = 2/3
It is hypergeometric distribution
P(X=k)={\frac {{\binom {K}{k}}{\binom {N-K}{n-k}}}{\binom {N}{n}}},
where
N = 60
K = 20 for Hawai; 40 for Texas
n = 10
P(X=0) = 0.0112
Hence The probability that none of the employees in the sample work at the plant in hawai is 0.0112
To know more about probability of getting none visit :
https://brainly.com/question/12043778
#SPJ4
Related Questions
What are the solutions to the following equation (c+5)^2=36
Answers
The quadratic equation (c+5)²=36 will give c = 1,-11.
What exactly is a quadratic equation?A quadratic equation is a type of equation in algebra that involves a variable (usually denoted as "x") raised to the power of 2, or squared. The standard form of a quadratic equation is:
ax² + bx + c = 0
where a, b, and c are constants, with a≠0. The goal is to solve for the variable x, which may have one or two possible solutions depending on the coefficients of the equation.
Now,
To solve the equation (c + 5)² = 36:
Taking the square root of both sides of the equation
√(c + 5)² = ±√36
Simplify the left-hand side using the rule that √a² = |a|:
|c + 5| = ±6
Solve for c in each case by subtracting 5 from both sides of the equation:
c + 5 = 6 or c + 5 = -6
Solve for c in each equation:
c = 1 or c = -11
Therefore, the solutions to the equation (c + 5)² = 36 are c = 1 and c = -11.
To know more about quadratic equations visit the link
https://brainly.com/question/30098550
#SPJ1
50 POINTSSSSSS
If C(n) = 20 - \(\frac{5}{8}\) m, find C(0) and C(16). What is the zero of C?
Answers
Consider the linear system {3x_1 - X_2 = 4 X_1 – 2x_2 = 3 2x_1 + 3x_2 = 2} (a) Find the least squares solutions of the above system. (b) Compute the least squares error vector and least squares error.
Answers
(A) The above linear system's least squares solutions are x₁ = 1/7 and x₂ = 22/21.
(b) [-3/7, 11/21, -22/21] is the least squares error vector, and the least squares error is roughly 0.8571.
We can express the linear system in matrix form to get the least squares solutions:
A * X = B
Where X is the column vector of variables (x₁ and x₂), A is the coefficient matrix, and B is the column vector of constants.
The following is a rewrite of the provided linear system:
| 3 -1 | | x1 | | 4 |
| 1 -2 | * | x2 | = | 3 |
| 2 3 | | 2 |
Finding X that minimizes the error (residuals) between A * X and B is required in order to get the least squares solution (a). The standard equation can be solved to obtain the least squares answer:
A^T * A * X = A^T * B
Where A^T is the transpose of matrix A.
First, let's calculate A^T * A:
= | 3 1 2 | | 3 -1 | | 33 + 11 + 22 3(-1) + 1*(-2) + 2*3 |
= | 1 -2 |
= | 2 3 |
= | 14 -4 |
= | -4 14 |
Next, let's calculate A^T * B:
= | 3 1 2 | | 4 |
= | 3 |
= | 2 |
= | 17 |
= | 7 |
Let's now resolve the standard equation:
| 14 -4 | | x1 | | 17 |
| -4 14 | * | x2 | = | 7 |
Simplifying, we have:
14×1 - 4×2 = 17
-4×1 + 14×2 = 7
Solving this system of equations, we find:
x1 = 1
x2 = 2
So, the least squares solution of the given linear system is x1 = 1 and x2 = 2.
Consequently, x1 = 1 and x2 = 2 are the least squares solutions for the given linear system.
(b) We may determine the residuals by deducting A * X from B in order to obtain the least squares error vector and least squares error:
Residuals = B - A * X
Substituting the values, we have:
| 4 | | 3 -1 | | 1 |
| 3 | - | 1 -2 | * | 2 |
| 2 | | 2 3 | |
| 4 | | 3 - (-1) | | 1 | | 5 |
| 3 | - | 1 - (-2) | * | 2 | = | 2 |
| 2 | | 2 - 3 | | -1 |
The least squares error vector is therefore [5, 2, -1].
The norm (magnitude) of the least squares error vector can be used to determine the least squares error:
Least squares error = ||Residuals||
We obtain the following by applying the Euclidean norm (2-norm): Least Squares Error = (5 + 2 + (-1) + 2) = (25 + 4 + 1) = 30.
As a result, the least squares error is roughly 30.
To learn more about linear system link is here:
brainly.com/question/29175254
#SPJ4
Which type of function is represented by the equation 6y=2(x−5)
Answers
The type of the function that is represented by 6y = 2(x - 5) is a linear function
What are linear functions?Linear functions are equations that have constant average rates of change. Note that the constant average rates of change can also be regarded as the slope or the gradient
How to determine the type of the function?The equation of the function is given as
6y = 2(x - 5)
Divide both sides of the equation by 6
So, we have
y = 1/3(x - 5)
Open the bracket
y = 1/3x - 5/3
The form of the above equation is
y = mx + c
The equation represented by y = mx + c is a linear function
Hence, the type of the function that is represented by 6y = 2(x - 5) is a linear function
Read more about linear function at
https://brainly.com/question/15602982
#SPJ1
What is the hypotenuse

Answers
square root of 2704 is 52
hypotenuse is 52
The second angle of a triangle is 20 more than the first angle. The third angle is two times the first. Find the three angles.
Answers
Answer:
\(\large\boxed{\textsf{First Angle = 40}^{\circ}}\)
\(\large\boxed{\textsf{Second Angle = 60}^{\circ}}\)
\(\large\boxed{\textsf{Third Angle = 80}^{\circ}}\)
Step-by-step explanation:
\(\textsf{We are asked to find the measurement of 3 unknown angles. We are given that}\)
\(\textsf{a Triangle is the shape, meaning that there are 3 angles total.}\)
\(\large\underline{\textsf{What is a Triangle?}}\)
\(\textsf{A Triangle is a 3-sided shape with 3 angles. Sometimes, these can be congruent.}\)
\(\textsf{Because a Triangle has 3 angles, the sum of the angles' measurements is equal}\)
\(\textsf{to 180}^{\circ}.\)
\(\large\underline{\textsf{Forming an Equation;}}\)
\(\textsf{We know that the first angle is not stated to relate to any other angles, hence}\)
\(\textsf{let's call this angle \boxed{\tt x.}}\)
\(\textsf{The Second Angle is 20 more than the first angle, or x. This is represented as}\)
\(\boxed{\tt x + 20.}\)
\(\textsf{The Third Angle is twice the measurement of the first angle. This is represented}\)
\(\textsf{as;} \ \boxed{\tt 2x.}\)
\(\textsf{Remember that these angles add up to 180}^{\circ}, \ \textsf{hence their combined sum is}\)
\(\textsf{identified.}\)
\(\underline{\textsf{Our Equation;}}\)
\(\boxed{\tt x^{\circ} + x^{\circ} + 20^{\circ} + 2x^{\circ} = 180^{\circ}}\)
\(\large\underline{\textsf{Solving;}}\)
\(\textsf{Let's begin by solving for x. Afterwards we can find the measures of all the angles}\)
\(\textsf{by Substitution.}\)
\(\underline{\textsf{Solving for x;}}\)
\(\tt x^{\circ} + x^{\circ} + 20^{\circ} + 2x^{\circ} = 180^{\circ}\)
\(\textsf{We first should consider that there are like terms in this equation. All the x's can}\)
\(\textsf{combine together since they're alike.}\)
\(\tt \boxed{\tt x^{\circ} + x^{\circ}} + 20^{\circ} + \boxed{\tt 2x^{\circ}} = 180^{\circ}\)
\(\underline{\textsf{This results as;}}\)
\(\tt 4x^{\circ} + 20^{\circ}= 180^{\circ}\)
\(\textsf{Our next step should be isolating x, this involves removing 20 from the left side.}\)
\(\textsf{This involves using the Properties of Equalities which state that whenever a}\)
\(\textsf{constant is used to manipulate an equation, the expressions still show equality.}\)
\(\textsf{For our problem, using the Subtraction Property of Equality, when we subtract}\)
\(\textsf{20 from both sides of the equation, then the equation remains equal.}\)
\(\underline{\textsf{Subtract 20 from both sides of the equation;}}\)
\(\tt 4x^{\circ} + 20^{\circ} - 20^{\circ} = 180^{\circ} - 20^{\circ}\)
\(\tt 4x^{\circ} = 160^{\circ}\)
\(\textsf{Using the Division Property of Equality, we are able to divide each side by 4 to}\)
\(\textsf{remove the coefficient of 4 from x.}\)
\(\tt \frac{4x^{\circ}}{4} = \frac{160^{\circ}}{4}\)
\(\large\boxed{\tt x = 40^{\circ}}\)
\(\large\underline{\textsf{Finding the Unknown Angles;}}\)
\(\textsf{We know that the measure of x is 40, which represents the measure of the first}\)
\(\textsf{angle.}\)
\(\large\boxed{\textsf{First Angle = 40}^{\circ}}\)
\(\textsf{We know that the second angle is 20 more than the first angle. Knowing that}\)
\(\textsf{the first angle is 40, the sum is 60.}\)
\(\large\boxed{\textsf{Second Angle = 60}^{\circ}}\)
\(\textsf{We know that the third angle is twice the measure of the first angle. This means}\)
\(\textsf{40 is multiplied by 2, which gives us a product of 80.}\)
\(\large\boxed{\textsf{Third Angle = 80}^{\circ}}\)
help on proof
Prove that 1+3+5+...+ (2n+1) = (n+1)² Verify that for all n 1
Answers
Using mathematical induction, we have prove that 1+3+5+...+ (2n+1) = (n+1)²
The first step is to verify the base case, which is n=1.
Then, assume that the equation is true for some arbitrary value k, and use that assumption to show that it is true for k+1.
Step 1: Base Case
When n=1,1+3=42=2²
Therefore, the equation holds for n=1
Step 2: Induction Hypothesis
Assume that the equation holds for some value k, so:1+3+5+...+(2k+1) = (k+1)²
Step 3: Inductive Step
To show that the equation holds for k+1, we need to add the next term, which is (2k+3):1+3+5+...+(2k+1)+(2k+3) = (k+1)² + (2k+3)
Factoring out (k+2) from the right-hand side gives:(k+2)²+(2k+3) = (k+1)² + (2k+3)
Simplifying the left-hand side gives:k²+4k+4+2k+3 = k²+2k+1+2k+3
The right-hand side simplifies to (k+1)², so:k²+4k+7 = (k+1)²Therefore, the equation holds for k+1 as well.
Step 4: Conclusion
Using the principle of mathematical induction, we have shown that the equation holds for all n greater than or equal to 1.
Learn more about mathematical induction. from the given link
https://brainly.com/question/29503103
#SPJ11
In the morning a group of hikers hiked 3 ½ miles, took a break, and then hiked another 2 ¾ miles. The group then stopped for lunch. In the afternoon, the group the hiked 4 ¼ miles, and took another break. How many more miles do they need if the whole trail is 14 miles long?
Answers
Answer:
3 2/4
Step-by-step explanation:
1/2 is equivalent to 2/4 so you add 3 2/4 + 2 3/4 and that = 6 1/4 and its simple math from there on, 6 1/4 + 4 1/4 = 10 2/4 finally 14 - 10 2/4 = 3 2/4
5.5 miles are left to cover.
What is Unitary Method?The unitary technique involves first determining the value of a single unit, followed by the value of the necessary number of units.
For example, Let's say Ram spends 36 Rs. for a dozen (12) bananas.
12 bananas will set you back 36 Rs. 1 banana costs 36 x 12 = 3 Rupees.
As a result, one banana costs three rupees. Let's say we need to calculate the price of 15 bananas.
This may be done as follows: 15 bananas cost 3 rupees each; 15 units cost 45 rupees.
Given:
In the morning a group of hikers hiked 3 ½ miles
and then hiked another 2 ¾ miles. then group the hiked 4 ¼ miles.
Total miles to cover = 14 miles
So, number of miles to be driven
= 14 - ( 3 ½ + 2 ¾ + 4 ¼)
= 14 - ( 7/2 + 11/4 + 17/4)
= 14- (14/4 + 11/4 + 17/4)
= 64 - 42 / 4
= 22/4= 11/2
= 5.5 miles
Hence, 5.5 miles are left to cover.
Learn more about Unitary Method here:
https://brainly.com/question/22056199
#SPJ5
A television that costs $500 is marked up 250%. What is the new price if the television?
Answers
Answer:1,750
Step-by-step explanation:
sorry if im wrong I tried
Can someone help me ASAP !
Which fraction and decimal forms match the long division problem?
15) 7.000
6 0
1 00
90
100
90
10
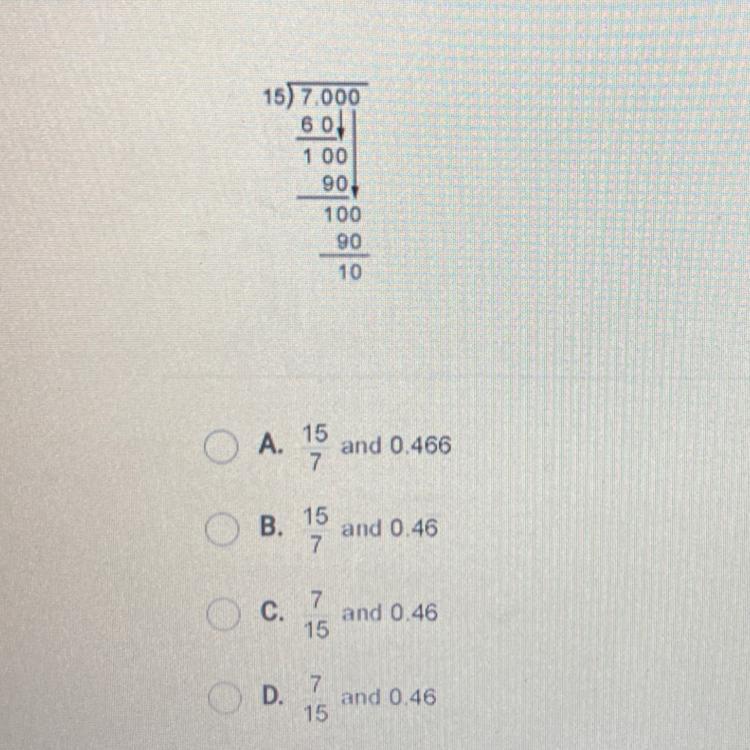
Answers
Answer:
It has to be either A or B. Im sorry Im not completely sure which one it is. I believe it is B. im sorry of im incorrect.
Answer: c
Step-by-step explanation:
Dan earns £400 over the course of a five-day week. How much is that per day?
Answers
Answer:
£80
Step-by-step explanation:
If there are 5 days in a work week, and he earns £400 for the whole week, you would divide £400 by 5. You get 80, so Dan earns £80 each day.
Answer:
£80
Step-by-step explanation:
divide £400 by 5
400/5 = 80
Select the correct location on the graph. (in screenshot)
The given equation is graphed.
Which point represents a negative solution for x?
PLEASE HELP :(

Answers
The solutions are given by the intersections of the graphs, we conclude that the negative solution for x is x = -2.
Which point represents a negative solution for x?
Here we have a system of equations graphed, each graph (red and blue ones) represents each side of the given equation:
The red one represents the right side, while the blue one represents the left side.
Then, the solutions are all of these points where the two graphs intersect (meaning that both equations have the same value in that point).
Then, the negative solution for x is given by the intersection in the left side of the vertical axis, which happens at x = -2, so we conclude that the negative solution for x is x = -2
If you want to learn more about systems of equations:
https://brainly.com/question/13729904
#SPJ1
Need help quick please! Find the surface area. Hint you will need to use Pythagorean theorem

Answers
Answer:
80
Step-by-step explanation:
what is "The temperature outside at 6 a.m. is 4 degrees celsius. The temperature rising by 1.5 degrees celsius every hour." in a mathematical equation!! please help
Answers
Answer:
7 a.m is 5.5
Step-by-step explanation:
(6=4)+1.5=(_+1.5)
make a T chart
thats how it should work for you
On a scale drawing of a basketball court, 1 inch equals 8 feet. What is the actual area of the basketball court if the scale drawing is 11.75 inches by6.25 inches? Enter your answer in the box.ft2
Answers
Answer:
The actual area of the basketball court is;
\(A=4700\text{ }ft^2\)Explanation:
Given that the scale of the drawing is;
\(1\text{ inch }\rightarrow8ft\)And the dimension of the basketball court on the scale drawing is;
\(11.75\text{ inches by 6.25 inches}\)The actual dimension will be;
\(\begin{gathered} 11.75\text{ }\times8ft\text{ by 6.25 }\times8ft \\ 94\text{ ft by 50 ft} \end{gathered}\)Therefore, the actual area of the basketball court will be;
\(\begin{gathered} A=l\times w \\ A=94\times50\text{ }ft^2 \\ A=4700\text{ }ft^2 \end{gathered}\)The actual area of the basketball court is;
\(A=4700\text{ }ft^2\)Solve for x: 1 over 3 (2x − 8) = 4. (1 point)
Group of answer choices
2
6
10
10 over 3
Answers
Answer:
x=6
Step-by-step explanation:
\(2x - 8 = 4 \\ 2x = 4 + 8 \\ 2x = 12 \\ x = \frac{12}{2} \\ x = 6\)
A unit of pressure called "feet of liquid substance- Y " (or ft−Y ) is equivalent to the pressure that will exist one ft below the surface of Y 's surface. If the conversion factor for this unit is 1 atm=41.5ft−Y,… - ... the density of the liquid substance Y is
Answers
The density of the liquid substance Y can be determined by using the conversion factor 1 atm = 41.5 ft⁻Y and the density of the liquid substance Y is approximately 19.68 ft⁻Y.
Conversion factor: 1 atm = 41.5 ft⁻Y
The "feet of liquid substance - Y" unit is defined as the pressure equivalent to the pressure that exists one foot below the surface of substance Y. In other words, if we go one foot below the surface of substance Y, the pressure will be equivalent to 1 ft⁻Y.
Since pressure is directly related to the density of a liquid, we can equate the pressure in units of atm to the pressure in units of ft⁻Y.
Therefore, we can say:
1 atm = 41.5 ft⁻Y
From this equation, we can conclude that the conversion factor for pressure between atm and ft⁻Y is 41.5.
we can calculate the conversion factor from "feet of liquid substance - Y" (ft⁻Y) to atm.
To convert from ft⁻Y to atm, we can use the inverse of the given conversion factor:
Conversion factor: 1 atm = 41.5 ft⁻Y
Taking the reciprocal of both sides:
1 / 1 atm = 1 / 41.5 ft⁻Y
Simplifying the equation:
1 atm⁻¹ = 0.024096 ft⁻Y⁻¹
Now, to find the density of the liquid substance Y in units of ft⁻Y, we can multiply the given density in g/cm³ by the conversion factor:
Density in ft⁻Y = 816.55 g/cm³ * 0.024096 ft⁻Y⁻¹
Calculating the density in ft⁻Y:
Density in ft⁻Y ≈ 19.68 ft⁻Y
learn more about conversion factor here:
https://brainly.com/question/30166433
#SPJ11
Select all expressions that equivalent

Answers
........................
the expected cell frequency is based on the researcher's opinion.
True or false
Answers
False. The expected cell frequency in statistical analysis, specifically in the context of contingency tables and chi-square tests, is not based on the researcher's opinion. Instead, it is determined through mathematical calculations and statistical assumptions.
In contingency tables, the expected cell frequency refers to the expected number of observations that would fall into a particular cell if the null hypothesis of independence is true (i.e., if there is no relationship between the variables being studied). The expected cell frequency is calculated based on the marginal totals (row totals and column totals) and the overall sample size.
The expected cell frequency is computed using statistical formulas and is not influenced by the researcher's opinion or subjective judgment. It is a crucial component in determining whether the observed frequencies in the cells significantly deviate from what would be expected under the null hypothesis.
By comparing the observed cell frequencies with the expected cell frequencies, statistical tests like the chi-square test can assess the association or independence between categorical variables in a data set.
Thus, the statement "the expected cell frequency is based on the researcher's opinion" is false. The expected cell frequency is derived through statistical calculations and is not subject to the researcher's subjective input.
To learn more about cell frequency
https://brainly.com/question/31994396
#SPJ11
Choose the equation that represents the line passing through the point (2, - 5) with a slope of −3.
Answers
We must determine the y-intercept, b using the slope and point provided.
\(-5 = -3(2) + b\\\\-5 = -6 + b\\\\1 = b\)
Now that we know the y-intercept, b, we can create the equation of this line: y = -3x + 1.
Show that lim (0) = 1, where (1) is the principal value of the nth root of i. 100
Answers
\(lim_{(x --> 0)} f(x) = 1\). It is proved that (1) is the principal value of the nth root of i.
Given the function \(f(x) = (1^{1/n})/x\).
We are to show that \(lim_{(x --> 0)} f(x) = 1\), where 1 is the principal value of the nth root of i.
Formula used: The principal value of the `n`th root of i is \(cos ((\pi)/(2n)) + i sin ((\pi)/(2n))\).
Since f(x) = \((1^{1/n})/x\), we can simplify f(x) as follows: f(x) = \(1/x^{(1/n)}\).
As x approaches 0, f(x) becomes f(0) = \(1^{(1/n)}/0\).
Here, we assume that `n` is even, so that n = 2m.
Substituting n with 2m, we have \(f(0) = (cos((\pi)/(2n)) + i sin((\pi)/(2n)))^{(1/2m)}\).
This is the principal value of the nth root of i, which is equal to `1`.
To learn more about root click here https://brainly.com/question/28707254
#SPJ11
NEED ANSWER ASAP PLEASE. Which line is AC NOT a transversal to?
Α) ΜΑ
B) NB
C) MN
D) PC

Answers
Answer:
Option C is correct.Step-by-step explanation:
A transversal is a line that is intersecting at 2 points. Let's verify all options to see which one is the odd one out.
__________________________________________________Option A
This line is a transversal to AC because it is intersecting in between AC. Hence, Option A is incorrect for this question.
__________________________________________________Option B
This line is also a transversal to AC because it is intersecting in between AC. Hence, Option B is incorrect for this question.
__________________________________________________Option C
This line is not a transversal because it is not intersecting AC. Hence, this will be the correct answer for this question.
__________________________________________________Option D
This line is a transversal because it is intersecting in between AC. Hence, Option D is incorrect for this question.
__________________________________________________After verifying all options, Option C is the most reasonable option. Hence, Option C is correct.
Hoped this helped.
\(BrainiacUser1357\)
The National Center for Education Statistics reported that 47% of college students work to pay for tuition and living expenses. Assume that a sample of 450 college students was used in the study.
Answers
Using the z-distribution, it is found that the 95% confidence interval for the proportion of college students who work to pay for tuition and living expenses is: (0.4239, 0.5161).
If we had increased the confidence level, the margin of error also would have increased.
What is a confidence interval of proportions?A confidence interval of proportions is given by:
\(\pi \pm z\sqrt{\frac{\pi(1-\pi)}{n}}\)
In which:
\(\pi\) is the sample proportion.z is the critical value.n is the sample size.In this problem, we have a 95% confidence level, hence\(\alpha = 0.95\), z is the value of Z that has a p-value of \(\frac{1+0.95}{2} = 0.975\), so the critical value is z = 1.96. Increasing the confidence level, z also increases, hence the margin of error also would have increased.
The sample size and the estimate are given as follows:
\(n = 450, \pi = 0.47\).
The lower and the upper bound of the interval are given, respectively, by:
\(\pi - z\sqrt{\frac{\pi(1-\pi)}{n}} = 0.47 - 1.96\sqrt{\frac{0.47(0.53)}{450}} = 0.4239\)
\(\pi + z\sqrt{\frac{\pi(1-\pi)}{n}} = 0.47 + 1.96\sqrt{\frac{0.47(0.53)}{450}} = 0.5161\)
The 95% confidence interval for the proportion of college students who work to pay for tuition and living expenses is: (0.4239, 0.5161).
More can be learned about the z-distribution at https://brainly.com/question/25890103
#SPJ1
What two numbers multiply to get -36 and add to get 5
Answers
To estimate the height of a flagpole, Marci, who is 5 feet tall, stands so that her lines of sight to the top and bottom of the pole form a 90° angle. What is the height of the pole to the nearest foot? 9 ft
20 ft
25 ft
50 ft
Answers
The height of the pole is 20 feet (to the nearest foot).
Hence, option (B) is the correct answer.
What is the height of the pole to the nearest foot?Given that Marci, who is 5 feet tall, stands so that her lines of sight to the top and bottom of the pole form a 90° angle.
.Let the height of the pole be x feet
Now, using similar triangles, we can say that:
`5/x = x/h`
Where h is the distance between Marci and the pole.
Hence, we can write:
`h^2 = x^2 + 5^2`or`h^2 = x^2 + 25`
Now, using the given data, we know that `h = x + 5`
Thus, substituting h in the second equation, we get:
`(x+5)^2 = x^2 + 25`
Expanding the terms, we get:`
x^2 + 10x + 25 = x^2 + 25`
Simplifying the terms, we get:
`10x = 0x = 0`
Thus, the height of the pole is:
`x = h - 5`
But we know that h = x + 5
Hence, `x = h - 5 = (x+5) - 5 = x`
Therefore, the height of the pole is:x = 20 feet
Learn more about height of flag pole at
https://brainly.com/question/15368409
#SPJ11
I’m a bit stuck please help me out

Answers
On solving the question we can say that Therefore, the solutions to the inequality given inequality are: x < 4 or x > 6.
What is inequality?An inequality in mathematics is a relationship between two expressions or values that are not equal. Imbalance therefore leads to inequality. An inequality establishes a connection between two values that are not equal in mathematics. Equality is different from inequality. The inequality sign () is most commonly used when two values are not equal. Various inequalities are used to contrast values, no matter how small or large. Many simple inequalities can be solved by changing both sides until only variables remain. But many things contribute to inequality.
two inequalities
4x - 6 < 10
4x < 16
x < 4
2x - 4 > 8
2x > 12
x > 6
Therefore, the solutions to the given inequality are:
x < 4 or x > 6.
To know more about inequality visit:
https://brainly.com/question/29914203
#SPJ1
The power reducing formula for cos(θ) is cos 2
(θ)= 2
1+cos(2θ)
(a) Verify this identity when x= 6
7π
. (b) Plot f=cos 2
(x)− 2
1+cos(2x)
on the indicated domain. Since this is a trigonometric identity, f(x) should be 0 for all x. If you do not get y=0, explain why.
Answers
The given identity is not true for all values of \(`x`\).
To verify the given identity when \(`x = 6π/7`\), substitute the value of \(`x`\) in the given identity.
So,
\(`cos2(x) = cos2(6π/7)`\\ `cos(2x) = cos(2 × 6π/7) \\\\ cos(12π/7)`\\Now, \\`cos(12π/7) = cos(7π − 5π/7) \\ − cos(5π/7)`\)
Using the power reducing formula,
\(`cos2(θ) = 2(1 + cos(2θ)\\ = 1 + cos(2θ)`\\So, \\`cos2(6π/7) = 1 + cos(2 × 6π/7)\\ = 1 + cos(12π/7) \\= 1 − cos(5π/7)`.\)
Hence, the given identity is verified when \(`x = 6π/7`\).
(b) Now, we need to plot the graph of \(`f(x) = cos2(x) − 2/(1 + cos(2x))`\) on the indicated domain. The given identity states that \(`f(x)`\) should be 0 for all values of \(`x`\).
We can substitute a few values of \(`x` $ in `f(x)`\) and check if we get \(`0`\) or not. If we get \(`0`\), then we can conclude that the identity holds true for all values of \(`x`\).
However, it may be possible that we don't get \(`0`\) for some value of \(`x`\) because the function \(`f(x)`\) is undefined for some values of \(`x`\) (because of the denominator
\(`1 + cos(2x)`).\)
Therefore, we need to check the domain of the given function first. The denominator \(`1 + cos(2x)`\) should not be equal to \(`0`\).
Therefore, \(`cos(2x) ≠ −1`or `2x ≠ π`or `x ≠ π/2`\)
So, the domain of \(`f(x)` is `R − {π/2}`\).
Now, we can check a few values of \(`x`\) to see if \(`f(x)`\) is \(`0`\) or not. If it is not \(`0`\), then we need to explain why it is not \(`0`\).
Let's check \(`x = 0`.\\`f(0) = cos2(0) − 2/(1 + cos(2 × 0))\\ = 1 − 2/(1 + 1) \\= 1/2 ≠ 0`\)
Let's check \(`x = π/4`.\\`f(π/4) = cos2(π/4) − 2/(1 + cos(2 × π/4))\\ = (1/2)2 − 2/(1 + 0) \\= 1/2 − 2 \\= −3/2 ≠ 0`\)
We can also see that the graph of \(`f(x)`\) is not symmetric about the y-axis. Therefore, the identity does not hold true for all values of \(`x`\).
Hence, the given identity is not true for all values of \(`x`\).
To know more about identity visit:
https://brainly.com/question/11539896
#SPJ11
What is 5.88 divided by -4
Answers
Answer:
-1.47
Step-by-step explanation:
5.88/-4= -1.47
What transformation was not done to the linear parent function, f(x) = x, to get the function g(x) = - 5(x - 3) - 8 ? A. Reflection over the x-axis B. Shift down 8 units C. Horizontal stretch by a factor of 5 D. Shift right 3 units
Answers
Answer:
A. Reflection over the x-axis.
Step-by-step explanation:
Now, we present how to transform \(f(x) = x\) into \(g(x) = -5\cdot (x-3)-8\) below:
1) \(f(x) = x\) Given.
2) \(f(x-3) = x - 3\) Horizontal shift to the right.
3) \(-5\cdot [f(x-3)] = -5\cdot (x-3)\) Vertical scale factor.
4) \(-5\cdot f(x-3)-8 = -5\cdot (x-3)-8\) Vertical shift downwards.
5) \(g(x) = -5\cdot (x-3)-8\) Definition/Result
In consequence, the only transformation which was not done to the linear parent function was a reflection over the x-axis. The correct answer is A.
What is the quotient when the polynomial 4x2 - 2x - 12 is divided by 2x - 4?