A triangle has angles that measure 52.4 and 16.4. Which equation can be used to find the value of x, the third measure of the triangle?
Answers
If a triangle has angles that measure 52.4 and 16.4, then the equation which can be used to find the value of x, the third measure of the triangle is x = 180 - (52.4 + 16.4)= 111.2°.
To find the value of x, follow these steps:
The sum of all angles of a triangle is equal to 180°. Therefore, we can find the third angle of the triangle by subtracting the sum of the two angles from 180°.To find the value of x, we need to subtract the sum of the angles 52.4° and 16.4° from 180°. ⇒x = 180 - (52.4 + 16.4) ⇒x = 180 - 68.8 ⇒x = 111.2°.Thus, the equation which can be used to find the value of x, the third measure of the triangle is: x = 180 - (52.4 + 16.4)= 111.2°.
Learn more about triangle:
brainly.com/question/2217700
#SPJ11
Related Questions
Is the function g(x)= -2x^2 -2x+7 linear or nonlinear?
HELPP plsss
Answers
Answer:
Is non-linear
Step-by-step explanation:
It is a quadratic function
A sterilization procedure yields a decimal reduction time of
0.65 minutes. Calculate the minimum sterilization time required to
yield 99.9% confidence of successfully sterilizing 50 L of medium
containing 10^6 contaminating organisms using this procedure.
Answers
The minimum sterilization time required to achieve a 99.9% confidence level in successfully sterilizing 50 L of medium containing 10^6 contaminating organisms is approximately 1.95 minutes.
To calculate the minimum sterilization time required to yield 99.9% confidence of successfully sterilizing 50 L of medium containing 10^6 contaminating organisms, we need to use the concept of decimal reduction time (D-value) and the number of organisms.
The D-value represents the time required to reduce the population of microorganisms by one log or 90%. In this case, the given D-value is 0.65 minutes.
To achieve a 99.9% confidence level, we need to reduce the population of microorganisms by three logs or 99.9%, which corresponds to a 10^-3 reduction.
To calculate the minimum sterilization time, we can use the following formula:
Minimum Sterilization Time = D-value × log10(N0/Nf)
Where:
D-value is the decimal reduction time (0.65 minutes).
N0 is the initial number of organisms (10^6).
Nf is the final number of organisms (10^6 × 10^-3).
Let's calculate it step by step:
Nf = N0 × 10^-3
= 10^6 × 10^-3
= 10^3
Minimum Sterilization Time = D-value × log10(N0/Nf)
= 0.65 minutes × log10(10^6/10^3)
= 0.65 minutes × log10(10^3)
= 0.65 minutes × 3
= 1.95 minutes
Therefore, the minimum sterilization time required to yield 99.9% confidence of successfully sterilizing 50 L of medium containing 10^6 contaminating organisms using this procedure is approximately 1.95 minutes
To learn more about confidence level visit : https://brainly.com/question/15712887
#SPJ11
Solve for the volume of the pyramid. Show your work and explain the steps you used to solve.
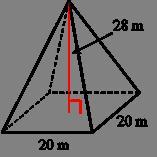
Answers
The volume of the pyramid is 3733.33 m³.
Having a square base and four lateral sides, a square pyramid is a type of pyramid in geometry. Having three or more triangular faces that intersect above its base, a pyramid is a particular kind of polyhedron (the apex).
The building is in the shape of a square pyramid.
The base of the building is 20 meters long.
The height of the building is 28 meters.
The volume of the right square pyramid is given as:
V = ( 1/3)a²h
Where a is the base and h is the height.
Now we have,
a = 20 m and h = 28 m
So,
V = ( 1/3 ) × 20 × 20 × 28
V = ( 1/3 ) × ( 20 )² × 28
V = 11200/3
V = 3733.33 m³
Learn more about volume here:
brainly.com/question/12146629
#SPJ9
Point (25,125) means that the monthly home heating bill was $
for an average monthly temperature of
°F.
Answers
The point (25,125) means that the monthly home heating bill was $5 for an average monthly temperature of 1°F.
How to interpret the meaning of the point?From the question, we have the following parameters that can be used in our computation:
Point (25, 125)
This point can be expressed as
(x, y) = (25, 125)
The average monthly temperature bill is then calculated using the following equation
Average monthly temperature bill = y/x
Substitute the known values in the above equation, so, we have the following representation
Average monthly temperature bill = 125/25
Evaluate
Average monthly temperature bill = 5
Hence, the average monthly temperature = 5
Read more about slope at
https://brainly.com/question/3493733
#SPJ1
what happens to an inequality sign when the inequality is multiplied or divided by a negative number
Answers
When an inequality is multiplied or divided by a negative number, the inequality sign will flip, meaning it will change its direction. For example, if you have a > b and you multiply or divide both sides by a negative number, the inequality will become a < b. This is because the relationship between the values reverses when multiplied or divided by a negative number.
Explanation:
When an inequality is multiplied or divided by a negative number, the direction of the inequality sign is flipped. This is because multiplication or division by a negative number, results in a reversal of the order of the numbers on the number line.
To see why this happens, consider the following example:
Suppose we have the inequality x < 5. If we multiply both sides of this inequality by -1, we get -x > -5. Notice that we have flipped the inequality sign from "<" to ">". This is because multiplying by -1 changes the sign of x to its opposite, and also changes the sign of 5 to its opposite, resulting in a reversal of the order of the numbers on the number line.
Similarly, if we divide both sides of the inequality x > 3 by -2, we get (-1/2)x < (-3/2). Here, we have again flipped the inequality sign from ">" to "<". This is because dividing by a negative number also changes the order of the numbers on the number line.
In general, if we have an inequality of the form a < b or a > b, where a and b are real numbers, and we multiply or divide both sides by a negative number, we obtain:
If we multiply by a negative number, the inequality sign is flipped. For example, if a < b and c < 0, then ac > bc.
If we divide by a negative number, the inequality sign is also flipped. For example, if a > b and c < 0, then a/c < b/c.
Therefore, it is important to be mindful of the signs of the numbers involved when performing operations on inequalities. If we multiply or divide by a negative number, we must flip the direction of the inequality sign accordingly.
Know more about the inequality click here:
https://brainly.com/question/30231017
#SPJ11
Write the equation of the circle with a radius of 10 and a center at (3,-1)

Answers
Answer:
\(\textsf{b)} \quad (x-3)^2+(y+1)^2=100\)
Step-by-step explanation:
\(\boxed{\begin{minipage}{5 cm}\underline{Equation of a circle}\\\\$(x-h)^2+(y-k)^2=r^2$\\\\where:\\ \phantom{ww}$\bullet$ $(h, k)$ is the center. \\ \phantom{ww}$\bullet$ $r$ is the radius.\\\end{minipage}}\)
Given:
center = (3, -1)radius = 10Substitute the given center and radius into the formula to create the equation of a circle with the given parameters:
\(\implies (x-3)^2+(y-(-1))^2=10^2\)
\(\implies (x-3)^2+(y+1)^2=100\)
What is the perimeter of a rectangle that
is 5 inches by 3 inches?
Answers
Answer:16
Step-by-step explanation:
Which pair of points represents a line segment with a slope of and a length of 15 units?

Answers
By using slope, it can be calculated that
(-8, -5) and (1, 7) represents a line with slope of \(\frac{4}{3}\) and length 15 units
What is slope of a line?
Slope of a line is the tangent of the angle that the line makes with the positive direction of x axis.
If \(\theta\) is the angle that the line makes with the positive direction of x axis, then slope (m) is given by
m = \(tan\theta\)
For the first option
(-11, 7) and (1, -2)
Slope = \(\frac{-2-7}{1 - (-11)}\)
= \(-\frac{9}{18}\)
= \(-\frac{1}{2}\)
First option is wrong
For the second option
(-2, -6) and (4, 2)
Slope = \(\frac{2 - (-6)}{4 - (-2)}\)
= \(\frac{8}{6}\)
= \(\frac{4}{3}\)
Length =
\(\sqrt{(4 - (-2))^2 + (2-(-6))^2}\\\sqrt{36+64}\\\sqrt{100}\\\)
10 units
Second option is wrong
For the third option
(-3, -2) and (9, 7)
Slope = \(\frac{7 - (-2)}{9 - (-3)}\)
= \(\frac{9}{12}\)
= \(\frac{3}{4}\)
Third option is wrong
For the fourth option
(-8, -5) and (1, 7)
Slope = \(\frac{7 - (-5)}{1 - (-8)}\)
= \(\frac{8}{6}\)
= \(\frac{4}{3}\)
Length =
\(\sqrt{(1 - (-8))^2 + (7-(-5))^2}\\\sqrt{81+144}\\\sqrt{225}\\\)
15 units
Fourth option is correct
To learn more about slope, refer to the link-
https://brainly.com/question/3493733
#SPJ1
y = 2x + 3
when x = -1, y =
Answers
Answer: y = 1
Step-by-step explanation:
y = 2(-1) +3
y = -2 + 3
y = 1
Which equation represents a line that passes through (2, –left-parenthesis 2, negative StartFraction one-half EndFraction right-parenthesis.) and has a slope of 3?
y – 2 = 3(x + y minus 3 equals 2 left-parenthesis x plus StartFraction one-half EndFraction right-parenthesis.)
y – 3 = 2(x + y minus 3 equals 2 left-parenthesis x plus StartFraction one-half EndFraction right-parenthesis.)
y + y plus StartFraction one-half EndFraction equals 3 left-parenthesis x minus 2 right-parenthesis. = 3(x – 2)
y + y plus StartFraction one-half EndFraction equals 2 left-parenthesis x minus 3 right-parenthesis. = 2(x – 3)\
Answers
The distance between two points is a line. The equation of the line is y = 3x - 8
Equation of a line
The equation of a line in slope - intercept form is given as;
y = mx + b
where
m is the slope
b is the intercept
Given the following
slope 'm' = 3
Determine the y-intercept
-2= 3(2) + b
-2 = 6 + b
b = -8
Determine the equation
y = 3x + (-8)
y = 3x - 8
Hence the equation of the line is y = 3x - 8
Learn more on equation of a line here; https://brainly.com/question/11602229
#SPJ1
At the instant shown, the shaft and plate rotates with an angular velocity of 19 rad/s and angular acceleration of 9 rad/s2 0.6 m 0.2 m 0.4m 0.3 m 0.3 m 0.4 m Part A eterminethe wocl a n D sea c Enter the ", y, and Z components of the velocity separated by commas vec m/s Submit Request Answer Part B Determine the acceleration of point D located on the corner of the plate at this instant. Enter the , y, and Z components of the velocity separated by commas vec m/s Submit Request Answer
Answers
The acceleration components of point D are, ax = 3.6 m/s², ay = 6.4 m/s², az = -1.14 m/s²
At the instant shown, the shaft and plate rotates with an angular velocity of 19 rad/s and angular acceleration of 9 rad/s2. The given diagram is shown below. Angular velocity (w) = 19 rad/sAngular acceleration (α) = 9 rad/s²Plate dimension AB = 0.6 m, BC = 0.2 m, CD = 0.4 m, DA = 0.3 m, AE = 0.3 m and EF = 0.4 m.
Determine the wocl and Dsea of Enter the ", y, and Z components of the velocity separated by commas vec m/sThe velocity components for point D can be calculated using the following formula.Vd = R x wWhere R is the position vector of D relative to the origin.
According to the given diagram, the position vector of point D relative to the origin is, R = 0.2i + 0.4j + 0.3kThe velocity of point D can be calculated as follows.Vd = R x w = 0.2i + 0.4j + 0.3k x 19The cross product of R and w can be calculated as follows.i j k 0.2 0.4 0.3 0 19 0 = [(0.4 × 0) - (0.3 × 19)] i - [(0.2 × 0) - (0.3 × 0)] j + [(0.2 × 19) - (0.4 × 0)] kVd = -5.7i + 6.8kThus, the velocity components of point D are, Vx = -5.7 m/s, Vy = 0 m/s, Vz = 6.8 m/s
Determine the acceleration of point D located on the corner of the plate at this instant. Enter the, y, and Z components of the velocity separated by commas vec m/sThe acceleration components of point D can be calculated using the following formula.aD = R x α + w x (w x R)Where R is the position vector of D relative to the origin.
According to the given diagram, the position vector of point D relative to the origin is, R = 0.2i + 0.4j + 0.3kThe acceleration of point D can be calculated as follows.aD = R x α + w x (w x R) = (0.2i + 0.4j + 0.3k) x 9 + 19 x (19 x (0.2i + 0.4j + 0.3k)) = (0.4k - 0.3j) x 9 + 19 x (0.4 x (0.4j - 0.3k))aD = 3.6i + 6.4j - 1.14k.
Learn more about Acceleration
brainly.com/question/12550364
#SPJ11
What is the slope of the line that contains the points (1,4) and (4,8)
Answers
Answer:
i think 4/3 is the answer

In a binomial situation, n=18 and π=0.60. Determine the expected
value
Answers
The expected value in a binomial situation with n = 18 and π = 0.60 is E(X) = np = 18 * 0.60 = 10.8.
In a binomial situation, the expected value, denoted as E(X), represents the average or mean outcome of a random variable X. It is calculated by multiplying the number of trials, denoted as n, by the probability of success for each trial, denoted as π.
In this case, we are given n = 18 and π = 0.60. To find the expected value, we multiply the number of trials, 18, by the probability of success, 0.60.
n = 18 (number of trials)
π = 0.60 (probability of success for each trial)
To find the expected value:
E(X) = np
Substitute the given values:
E(X) = 18 * 0.60
Calculate the expected value:
E(X) = 10.8
learn more about binomial here:
https://brainly.com/question/31049218
#SPJ4
If \($4^x = 3$\), what is \($4^{2x-2}?$\)
Answers
\(4^x=3 \\\\[-0.35em] ~\dotfill\\\\ 4^{2x-2}\implies 4^{2x}\cdot 4^{-2}\implies (4^x)^2\cdot \cfrac{1}{4^2}\implies \cfrac{(4^x)^2}{16}\implies \cfrac{(3)^2}{16}\implies \cfrac{9}{16}\)
Use the expression 5(6 + 4x) to answer the following:
Part A: Describe the two factors in this expression.
Part B: How many terms are in each factor of this expression?
Part C: What is the coefficient of the variable term?
Answers
Answer:
A: 6 and 4x
B: SEE BELOW
C: 20 and X
Step-by-step explanation:
A: becuase of the 5 on the outside of the parentheses you would multiply both by 5 making the factors 6 and 4x
B: I just answered it in A
C 5*4x = 20x
20 is the coefficient and X is the variable
If x-1= k and k=3, what is the value of x
3
Answers
x-1=3
move 1 to the other side, making it positive
x=3+1
x=4
Answer:
x-1/3 = k where k=3
We must be happy enough to find the value of only one valuable here, that is, x.
therefore, x-1/3=3
To find what is x-1, all we need to do is,
Take the denominator of the fraction to the answer (3)and multiply them. (that's how you'll find the values in the numerator)
So, you'll get,
x-1=3*3
x-1=9
To find the value of x,
The other number (-1) should be taken to the answer (9)
(Remember that the sign changes when the number crosses the equals sign)
therefore,
x= 9 + 1 (-1 will turn to +1 on crossing the equal sign)
Therefore , by addition, normally,
you'll get x=10
Hope this helps:)
1
. a) Write in standard form:
i) 2 470 000
Answers
two million four hundred seventy thousand
Exercise 3.4.3: Proving algebraic statements with direct proofs. Prove each of the following statements using a direct proof. (a) For any positive real numbers, x and y, x + y >= √xy.
Answers
The statement "For any positive real numbers x and y, x + y >= √xy" can be proven using a direct proof by squaring both sides of the inequality and manipulating the expressions to show their equivalence.
To prove the statement using a direct proof, we start by assuming that x and y are positive real numbers. Our goal is to show that x + y >= √xy.
First, we square both sides of the inequality:
(x + y)^2 >= (√xy)^2
Expanding the left side of the inequality:
x^2 + 2xy + y^2 >= xy
Next, we simplify the inequality by subtracting xy from both sides:
x^2 + xy + y^2 >= 0
This inequality holds true for any real numbers x and y because the sum of squares is always non-negative.
Since we assumed x and y to be positive real numbers, the inequality x^2 + xy + y^2 >= 0 is always true. Therefore, we have shown that x + y >= √xy using a direct proof.
Learn more about real numbers here: brainly.com/question/15352175
#SPJ11
a nurse is converting a toddler's weight from lb to kg. if the toddler weighs 20 lb 8 oz, what is the toddler's weight in kg? (round the answer to the nearest tenth. use a leading zero if it applies. do not use a trailing zero.)
Answers
Answer:
9.3
Step-by-step explanation:
Answer: 9.3
Step-by-step
1lb = 16 oz
20 x 16 = 320
320 oz + 8 oz = 328
1 oz = 0.283495
328 + 0.283495 = 9.298636
Round 9.298636 = 9.3
A square garden has a 20 ft fence surrounding it. How much will it cost to mulch the garden if one bag covers 2.5 square feet and costs $3.99 per bag?
Answers
Answer:
$39.90
Step-by-step explanation:
Ok square, all sides equal.
20ft fence would be perimeter.
Covers is area.
20/4=5
5 is the length of each side.
A=5*5
A=25
one bag=2.5
25=2.5x
x=10
it takes 10 bags of mulch.
10*3.99
$39.90 to cover it all
Answer:
39.90
Step-by-step explanation:
name a type of
• plane. not a model one word hyphenated but two words total
Answers
A jet-liner is a type of plane not a model one word hyphenated but two words total.
A jet-liner is a type of plane that is specifically designed for passenger transportation on long-haul flights. It combines the efficiency and speed of a jet engine with a spacious cabin to accommodate a large number of passengers.
Jet-liners are commonly used by commercial airlines to transport people across continents and around the world. These planes are characterized by their high cruising speeds, advanced avionics systems, and extended range capabilities.
They are equipped with multiple jet engines, typically located under the wings, which provide the necessary thrust to propel the aircraft forward. Jet-liners also feature a pressurized cabin, allowing passengers to travel comfortably at high altitudes.
The design of jet-liners prioritizes passenger comfort, with amenities such as reclining seats, in-flight entertainment systems, and lavatories. They often have multiple seating classes, including economy, business, and first class, catering to a wide range of passengers' needs.
Overall, jet-liners play a crucial role in modern air travel, enabling efficient and comfortable transportation for millions of people worldwide.
For more such questions on jet-liner
https://brainly.com/question/32730843
#SPJ8
En una playa de estacionamiento hay 40 vehículos entre autos y motos. Si en total se cuentan 120 llantas, halla el número de autos que hay
Answers
Answer:
20 carros
Step-by-step explanation:
Dado que un automóvil tiene cuatro neumáticos, multipliqué la C por 4
M es para motocicletas. ya que las motos tienen 2 neumáticos. Multipliqué M por 2
de hecho, la respuesta está en la imagen de arriba

I need help my brain is too small to answer this

Answers
Answer:
5/6 or 0.83
Step-by-step explanation:
5 ÷ 6 = 0.83 or 5/6
If x and y vary directly and y is 70 when x is 14, find y when x is 3
Answers
14=70K
K=14/70
K=5
X=KY
3=(5)Y
3=5y
Y=3/5 or 1.7
evaluate the integral by reversing the order of integration. 4 0 12 5ex2 dx dy 3y
Answers
To reverse the order of integration, we need to rewrite the limits of integration in terms of the other variable.
The value of the given integral by reversing the order of integration is (5/12)(\(e^{24}\) - 1).
The given integral is ∫∫ \(5e^{(2x)}\) dx dy, where the limits of x are from 0 to 4 and the limits of y are from 0 to 3y.
To integrate with respect to y first, we need to express the limits of y in terms of x.
From the limits of y given, we have 0 ≤ y ≤ 3y, which simplifies to 0 ≤ y.
Now we need to find the upper limit of y. To do this, we set the expression for the upper limit equal to the constant 12, which is the upper limit of x.
So we have 3y = 12, which gives y = 4.
Thus, the limits of integration become ∫∫ \(5e^{(2x)}\) dy dx, where the limits of y are from 0 to 4 and the limits of x are from 0 to 3y.
Now we can integrate with respect to y:
∫∫ \(5e^{(2x)}\) dy dx = ∫ 0^4 ∫ 0^(3y) \(\int\limits^4_0 \int\limits^{(3y)}_0 5e^{(2x)} dx dy\)
= \(\int\limits^4_0 [5/2 e^{(2x)}]_0^{(3y)} dy\)
= \(\int\limits^4_0 [5/2 (e^{(6y)} - 1)] dy\)
= \([5/12 (e^{(6y)} - 1)]_0^4\)
= \((5/12)(e^{24} - 1)\)
Note that the order of integration can be reversed if the integrand is continuous on a rectangular region that contains the original region of integration.
To learn more about integration, refer:-
https://brainly.com/question/18125359
3SPJ11
can someone please tell me what 383.0 divided by 90 is?????????
Answers
Sure!!!
The answer is 4.25555!
Hope this helps! Have a great day! :D :)
Answer:
The answer is 4.2555555556
luke measured a swimming pool and made a scale drawing. the scale he used was 7 centimeters : 3 meters. the pool is 105 centimeters long in the drawing. how long is the actual pool?
Answers
The actual length of the swimming pool be 15m if the scale used by Luke is 7cm : 3m.
Let the actual length of the pool be x m.
According to the given question.
Luke measured a swimming pool and made a scale drawing. The scale he used was 7 centimeters : 3 meters.
⇒ The scale is 7cm : 3m.
Also, the length of the pool in the drawing is 105cm.
Therefore, the actual length of the swimming pool is given by
7cm : 3m = 105cm : x (by proportion fromula)
⇒ 7x = 105
⇒ x = 15m
Hence, the actual length of the swimming pool be 15m if the scale used by Luke is 7cm : 3m.
Find out more information about scale here:
https://brainly.com/question/17403281
#SPJ4
What is the length of PQ¯¯¯¯¯?
Enter your answer as a decimal in the box. Round only your final answer to the nearest tenth.
km
A horizontally-aligned triangle P Q R. Side P R is labeled as 6 kilometers. Side R Q is labeled as 9 kilometers. Angle R is labeled as 34 degrees.
Answers
The length of PQ is given as follows:
PQ = 5.24 km.
What is the law of cosines?The Law of Cosines is a trigonometric formula that relates the lengths of the sides of a triangle to the cosine of one of its angles. It is also known as the Cosine Rule.
The Law of Cosines states that for any triangle with sides a, b, and c and angle C opposite to side c, the following equation holds true:
c^2 = a^2 + b^2 - 2ab cos(C)
For the angle of 34º, we have that:
PQ is the opposite segment.6 km and 9 km are the adjacent segments.Hence the length of PQ is obtained as follows:
(PQ)² = 6² + 9² - 2 x 6 x 9 x cosine of 34 degrees
PQ = sqrt(6² + 9² - 2 x 6 x 9 x cosine of 34 degrees)
PQ = 5.24 km.
More can be learned about the law of cosines at https://brainly.com/question/4372174
#SPJ1

Find the median and mean of the following data set:
14, 16, 48, 22, 50, 39
Answers
Median = 30.5
Mean = 31.5
========================================================
Explanation:
The first task is to sort the items from smallest to largest.
{14, 16, 48, 22, 50, 39} sorts to {14, 16, 22, 39, 48, 50}
The median is the middle-most value. Since we have an even number of items here, the middle-most position is a tie between slots 3 and 4
The values in those slots are 22 and 39 respectively. The midpoint of them is (22+39)/2 = 30.5 which is the median
-----------------
To get the mean, we add up the values
14+16+22+39+48+50 = 189
Then we divide by 6 since there are 6 numbers here
189/6 = 31.5 is the mean
Blake buys a couch for $1000 and sells it for $1200. Calculate his profit as a percentage of the cost price.
Answers
Answer:
He earned 200 dollars
Step-by-step explanation:
He got a 20% profit.