-17 + 20 + -3 = ?
also please try to explain it if u can :3
Answers
Answer:
000000000000000
Step-by-step explanation:
no explanation
Related Questions
Please help, I'm really confused. What is the solution to the equation cube root x+4 plus cube root 2x+8 equals 0?
x = -12
x = -4
x = 4
x = 12
It's not x=4, I already got it wrong.
Answers
2. A large appliance company estimates that the variance of the life of its appliances is 3.
You work for a consumer advocacy group and are asked to test the claim at a = 0.05.
You find that the sample of the lives of 27 appliances has a variance of 2.8.
Answers
Answer:
30
Step-by-step explanation:
Answer:
if their are choices you should list them. The givens are pretty specific though.
W = E * I is the answer
E (voltage) = W/I is a more refined answer. <<< answe
Step-by-step explanation:
Jasmine is baking and selling cupcakes for $15 per dozen. She would like to make more than $120 in cupcake sales. Which number line below represents the solutions to this situation?
Answers
Let x be the number of dozens of cupcake made by Jasmine.
Her total sales= Sales Per Dozen X Number of Dozens of cupcake sold
= $15 X x = $15x
Since she would like to make more than $120 in cupcake sales.
It means her total sales must be greater than $120.
15x>120
x>120/15
x>8
The number line attached represents the solutions to the situation.
All integers to the right of 8 (excluding 8) satisfies the situation.
Hope this helps!:)
Ecuación de la hipérbola con centro en (0;0), focos en abrir paréntesis 0 coma espacio menos raíz cuadrada de 28 cerrar paréntesis espacio y espacio abrir paréntesis 0 coma espacio raíz cuadrada de 28 cerrar paréntesis espacio ,eje conjugado = 2 raíz cuadrada de 3
Answers
Answer:
\(\frac{y^{2}}{25}-\frac{x^{2}}{3}=1\)
Step-by-step explanation:
Para resolver este problema debemos tomar en cuenta los datos que nos dan y la ecuación de una hipérbola. Comencemos con los datos:
centro: (0,0)
focos: \((0,-\sqrt{28}),(0,\sqrt{28})\)
eje conjugado = \(2\sqrt{3}\)
por los focos podemos ver que la hipérbola se dirige hacia el eje y, por lo que debemos tomar la siguiente forma de la ecuación de la parábola:
\(\frac{y^{2}}{a^{2}}+\frac{x^{2}}{b^{2}}=1\)
de los focos podemos obtener que:
\(c=\sqrt{28}\)
y del eje conjugado podemos saber que al dividir la longitud del eje conjugado dentro de 2 obtenemos b, así que:
\(b=\sqrt{3}\)
podemos utilizar la siguiente fórmula para obtener a:
\(c^{2}-a^{2}=b^{2}\)
si despejamos a en la ecuación obtenemos lo siguiente:
\(a=\sqrt{c^{2}-b^{2}}\)
ahora podemos sustituir los valores:
\(a=\sqrt{(\sqrt{28})^{2}-(\sqrt{3})^{2}}\)
\(a=\sqrt{28-3}\)
\(a=\sqrt{25}\)
a=5
así que media vez conozcamos a, podemos sustituir los datos en la ecuación de la hipérbola así que obtenemos lo siguiente:
\(\frac{y^{2}}{a^{2}}+\frac{x^{2}}{b^{2}}=1\)
\(\frac{y^{2}}{(5)^{2}}+\frac{x^{2}}{(\sqrt{3})^{2}}=1\)
\(\frac{y^{2}}{25}+\frac{x^{2}}{3}=1\)
si graficamos la hipérbola, queda como en el documento adjunto.

In an hour, Ashley can produce 10 milkshakes or 30 ice cream sundaes, while Sara can produce 16 milkshakes or 80 ice cream sundaes. Which statement is false about Sara and Ashley?
O Ashley's opportunity cost of producing 1 milkshake is 3 sundaes.
O Sara has an absolute advantage in both goods.
O Sara has a comparative advantage in producing milkshakes.
O Sara's opportunity cost of producing 1 milkshake is 5 sundaes.
Charlie and Don are farmers who produce beef and corn. In a year, Charlie can produce 10 tons of beef or 40 bushels of corn, whereas Don can produce 10 tons of beef or 20 bushels of corn. To maximize their total output of beef and corn ___
O Charlie and Don each spend half of their time producing beef and the other half producing corn
O Charlie produces beef and corn while Don produces nothing
O Don produces beef and Charlie produces corn
O Charlie produces beef and Don produces corn
Answers
The false statement about Sara and Ashley can be derived by examining the opportunity cost of producing a milkshake. Opportunity cost is the loss of other alternatives when one alternative is chosen.In an hour, Ashley can produce 10 milkshakes or 30 ice cream sundaes,
while Sara can produce 16 milkshakes or 80 ice cream sundaes. Since Sara can produce milkshakes more efficiently, she has a comparative advantage in producing milkshakes. So, the false statement is the first statement which is Ashley's opportunity cost of producing 1 milkshake is 3 sundaes.To maximize their total output of beef and corn, Charlie and Don should specialize in the product for which they have a comparative advantage.
They should allocate their resources to maximize the production of beef and corn by specializing in the product for which they have a comparative advantage. Therefore, Charlie produces beef and Don produces corn.The correct option is:O Sara's opportunity cost of producing 1 milkshake is 5 sundaes.O Charlie produces beef and Don produces corn.
To know more about statement visit:
https://brainly.com/question/33442046
#SPJ11
BERE 6 1011 THE DIAGRAM SHOWS THREE SQUARES WHOSE SIDE LENGTHS MEET TO FORM A RIGHT TRIANGLE. USE THE DIAGRAM TO TYPE THE SOLUTION TO EACH QUESTION BELOW. If the area of square 1 A is 225 ft2 and the area of square B is 400 ft2, what is the area of If the area of square 2 A is 81 ft2 and the area of square C is 1,681 ft2, what is the area of C square C? square B? А If the perimeter of A 3 is 36 ft and the perimeter of B is 48 ft, what is the perimeter of If the area of C is 400 ft2 and the perimeter of B is 64 ft, what is the side length of square A? B square C? Messwering the Medla IC. 2019 C 1
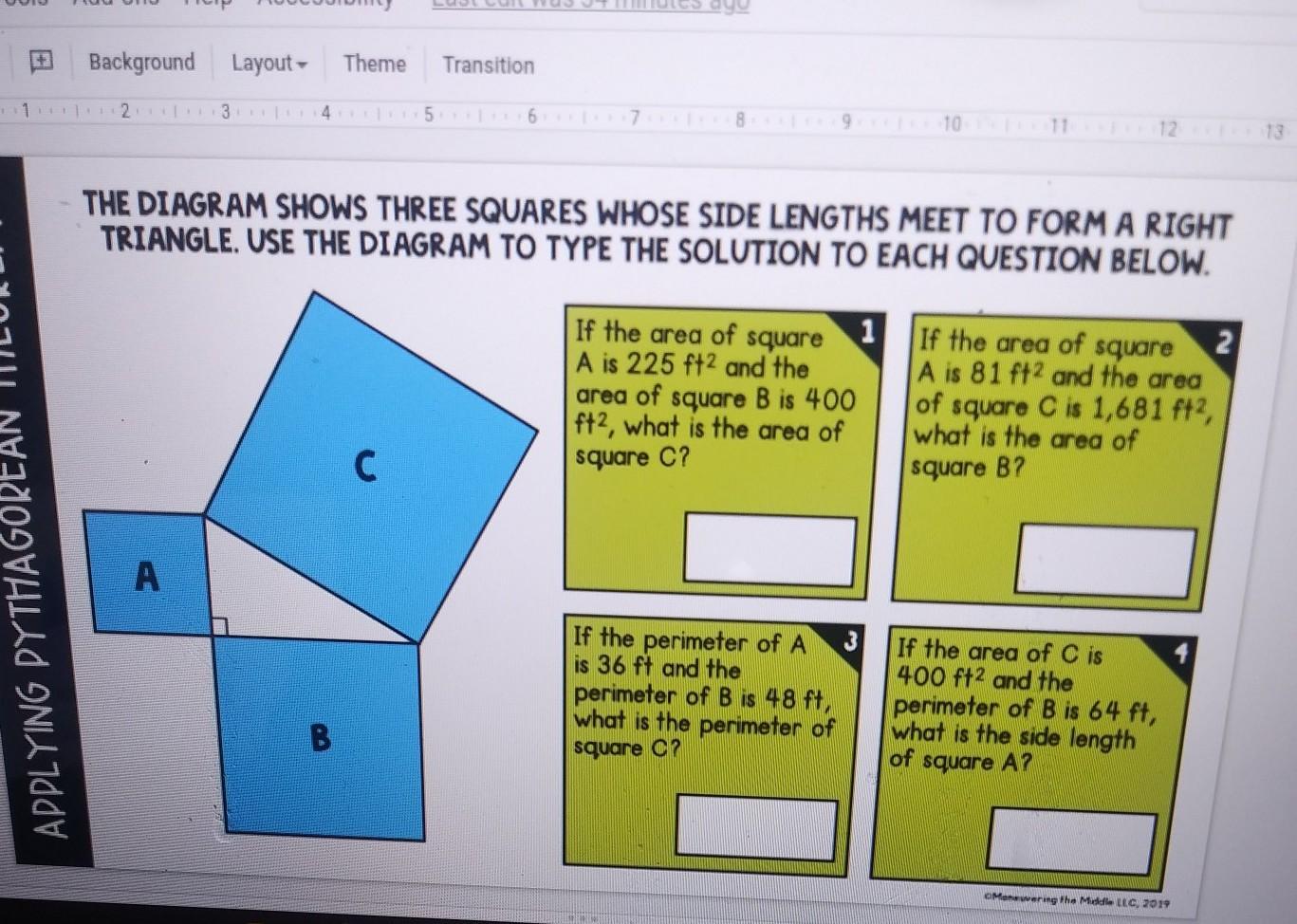
Answers
The area of a square is L X L
A = l x l
Where l is the side of the square
\(\begin{gathered} \text{Square A = 225ft}^2 \\ \text{Square B = 400ft}^2 \\ \text{Square A and square B form a right angle triangle to one side of the square C} \\ \text{ Since, Area of a square = l}^2 \\ \text{For Square A } \\ 225=l^2 \\ \text{Take the squareroot of both sides} \\ l\text{ = }\sqrt[]{225} \\ l\text{ = 15ft} \\ \text{For square B} \\ 400=l^2 \\ \text{Take the square root of both sides} \\ l\text{ = }\sqrt[]{400} \\ l\text{ = 20 ft} \\ To\text{ find one side of square C, we n}eed\text{ to apply Pythagora's theorem} \\ \text{Hypotenus}^2=opposite^2+adjacent^2 \\ \text{Opposite = 15, and adjacent = 20} \\ \text{Hypotenus}^2=15^2+20^2 \\ \text{Hypotenus}^2\text{ = 225 + 400} \\ \text{Hypotenus}^2\text{ = 625} \\ \text{Take the square root of both sides} \\ \text{hypotenus = }\sqrt[]{625} \\ \text{Hypotenus = 25ft} \\ \text{Hence, the area of Square C} \\ \text{Square C = 25 x 25} \\ \text{Square C = 625ft}^2 \end{gathered}\)Question 2
Area of Square C = Area of Square A + Area of square B
\(\begin{gathered} \text{Area of square C = 1681 ft}^2 \\ \text{Area of square A = 81 ft}^2 \\ \text{Area of Square C = Area of square B + Area of Square A} \\ \text{Area of Square B = Area of square C - Area of Square A} \\ \text{Area of square B = 1681 - 81} \\ \text{Area of square B = 1600 ft}^2 \end{gathered}\)can someone help me with 3 and 4 please?

Answers
For #3
A quadratic expression is in standard from when written as ax^2 + bx + c = 0. Where, a, b, and c are constants.
The only option that is written in the form above is option C. Where a=-1, b=-5, and c=7.
For #4
First how is 3x^2 in standard form?
Recall that the standard form of a quadratic is ax^2 + bx + c = 0. So lets alter 3x^2 a bit to show that it is in standard form.
3x^2 ==> 3x^2+0x+0
As you can see 3x^2 is in standard form, but the constants b and c are zero.
Second, how is 3x^2 in factored form?
Factored form is written as (a+x)(b+x). So let's alter 3x^2.
3x^2 ==> 3(0+x)(0+x)
3(0+x)(0+x) = 3(x)(x) which is 3x^2
Hence it can be said that 3x^2 is in both standard and factored form.
Graph the point (-2,3) and 3 more points using a slope of -1/2
Answers
Answer:
Step-by-step explanation:
(-2,3) k=-1/2
Find the equation of the line:
\(y=kx+b\)
\(\displaystyle\\3=-\frac{1}{2}*(-2)+b\\\\3=1+b\\ 3-1=1+b-1\\2=b\\Thus,\\y=-\frac{1}{2} x+2\)

The required 3 more coordinates are (0, 2), (1, 3/2), and (2, 1).
Given that,
To determine points using a slope of -1/2 and the point (-2,3).
Coordinate, is represented as the points on the x-axis and y-axis of the graph.
Here,
using the slope-intercept form of the equation,
y = mx + c
we have a point (-2, 3) and m = -1/2
Put point and slope in the equation,
3 = -1/2 * -2 + c
c = 3 - 1
c = 2
Now equation would be
y = -1/2x + 2
Now,
For x = 0
y = 2
Coordinate will be (0,2)
For x = 1
y = -1/2 + 2
y = 3/2
Coordinate will be (1, 3/2)
For x = 2
y = -1/2 * 2 + 2
y = 1
Coordinate will be (2, 1)
Thus, the required 3 more coordinates are (0, 2), (1, 3/2), and (2, 1).
Learn more about coordinate here:
brainly.com/question/13498438
#SPJ2
which graph represents a function?

Answers
Answer: The 2nd graph
Step-by-step explanation:
Using the vertical line test, all other 3 graphs have two points that meet on the same lines. This immediately rules all other 3 graphs out as a graph cannot have two points with the same x-axis.
Abe starts working out at a gym and begins to lose weight. His weight can be calculated using the equation y = 250 − 3x where y is his current weight and x is the number of weeks he has been working out. Which statement best describes Abe's weight loss?
Answers
Answer:
The number of weeks Abe has been working out is the independent variable in the equation.
Step-by-step explanation:
Since Abe's weight is given by the equation
y = 250 − 3x
Every increase or decrease in the value of x in the equation will invariably lead to a change in the value of y.
If for instance Abe works out 5 weeks, her weight would be:
y= 250- 3*5
y=250-15
y=235
If she works out 3 weeks, her weight would be
y=250-3*3
y=250-9
y=241
Hence y weight is dependent variable while x weeks worked out is the independent variable in the equation
The sum of three consecutive terms of an arithmetic sequence is 27, and the sum of their square is 293. What is the absolute difference between the greatest and the least of these three numbers in the arthritic sequence?The answer is 10 I don’t know how to get there
Answers
The sum of three consecutive terms of an arithmetic sequence is 27, and the sum of their square is 293. What is the absolute difference between the greatest and the least of these three numbers in the arthritic sequence?
Let
x -----> first consecutive term
so
x+d ----> second consecutive term
x+2d ----> third consecutive term
where
d -----> common factor
we have that
x+(x+d)+(x+2d)=27
3x+3d=27------> simplify -----> x+d=9 -----> equation 1
the sum of their square is 293
so
x^2+(x+d)^2+(x+2d)^2=293
x^2+(x^2+2xd+d^2)+(x^2+4xd+d^2)=293 ---------> equation 2
Solve the system by graphing
see the attached figure
the solution is the point (4,5)
so
x=4
d=5
therefore
the first term is 4
the second term is 4+5=9
the third term is 4+2(5)=14
the difference is 14-4=10

Please help simplify and write your answer in the form a + bi

Answers
Answer:
\( 2 - 4i\)
Step-by-step explanation:
\( \frac{10}{1 + 2i} \\ \\ = \frac{10}{1 + 2i} \times \frac{1 - 2i}{1 - 2i} \\ \\ = \frac{10(1 - 2i)}{ {1}^{2} - {(2i)}^{2} } \\ \\ = \frac{10 - 20i}{ {1} - {4i}^{2} } \\ \\ = \frac{10 - 20i}{ {1} - {4( - 1)} } \\ ( \because \: {i}^{2} = - 1) \\ \\ = \frac{10 - 20i}{1 + 4} \\ \\ = \frac{5(2 - 4i)}{5} \\ \\ = 2 - 4i\)
SOMEONE PLEASE HELP ME QUESTION 5 2 PARTS IN THE PICTURE!!!!!

Answers
Answer:
x ≥ 6; see below for a graph
Step-by-step explanation:
First inequality
7x -6 > 8
7x > 14 . . . . . . add 6
x > 2 . . . . . . . .divide by 7
Second inequality
3x+4 ≥ 22
3x ≥ 18 . . . . . . subtract 4
x ≥ 6 . . . . . . . . divide by 3
The values of x that will satisfy both inequalities are x ≥ 6. (All of those values are > 2.)
The solution to the inequalities is x ≥ 6.
__
Your graph will have a solid dot at x=6, indicating 6 is part of the solution set. It will be shaded to the right, with an arrow at the right end.

Suppose you have a job that pays $13.50 per hour and you work anywhere from 10 to 40 hours per week. a. Write an equation, with a restriction on the variable I, that gives the amount of money, y, you will earn for working 2 hours in one week. y = _____ , Preview with ____ <= x <= ____ b. Use the function rule you have written in part a. to find the y values for the given z values: x = 10, y = ___ x = 20, y= ___
x = 30, y = ____. x = 40, y = ____ c. Construct a line graph from the information found in b. 520+ -480+ 440+ 400- 360 320- 280- 240 200 160+ 120+ 80- 40+ 10 20 30 40 Clear All Draw: Line Dot Open Dot d. State the domain and range of this function. Domain: ____ <= x <= ______
Range: <= y <= _____
e. What is the minimum amount you can earn in a week with this job? You'll earn at least $ ______.
What is the maximum amount? You can earn up to $ ____.
Answers
The maximum amount you can earn is $540
a. y = 13.50x , 10 <= x <= 40
b. x = 10, y = 135; x = 20, y= 270; x = 30, y = 405; x = 40, y = 540
c. Domain: 10 <= x <= 40; Range: 0 <= y <= 540
d. The minimum amount you can earn in a week with this job is $135. The maximum amount you can earn is $540.
To learn about Domain
brainly.com/question/29452843
#SPJ11
what is standard error of mean
Answers
The standard error of mean is a technique for calculating the sampling distribution's standard deviation.
A technique for assessing the standard deviation of a sampling distribution is standard error of the mean. The acronym SEM stands for standard error of the mean, which is another name for it. For instance, in a sample space, the sample mean is often the projected population mean value. But, if we choose another sample from the same population, the result can be different.
As a result, a population of the sampled means with unique variance and means will emerge. The standard deviation of such a sample mean, which includes all feasible samples taken from the same specified population, could be referred to as the standard error of mean. SEM is an approximation of standard deviation that was determined from the sample.
The standard error of the mean (SEM) demonstrates how the mean changes when the same quantity is evaluated in various tests. Consequently, the SEM will be higher if the outcome of random fluctuations is crucial. Yet if repeated attempts fail to reveal any change in the data points, the standard error of the mean will be equal to zero.
Learn more about Standard error of mean:
https://brainly.com/question/22554353
#SPJ4
true or false: if a data set is approximately normally distributed, its normal probability plot would be s-shaped.
Answers
Can some one pls helppp

Answers
Answer:
3111
Step-by-step explanation:
rewrite (√3111)^2 as 3111
Measures of the two triangles DEF and PQR
are shown in the figure. Check if the
triangles are congruent. If congruent, which
of the following statements is true?Justify.
∆DEF ≅ ∆PQR ;
∆ DEF ≅ ∆ QPR ;
∆DEF ≅ ∆RPQ
Answers
Triangles ABC and triangle PQR are congruent triangles based on angle-side-angle congruency theorem.
What are congruent triangles?Two triangles are said to be congruent if they have the same shape and their corresponding sides are congruent to each other. Also, their corresponding angles are congruent to each other.
Triangles ABC and triangle PQR are congruent triangles based on angle-side-angle congruency theorem.
Find out more on congruent triangles at: https://brainly.com/question/2938476
#SPJ1

Anna makes a pot of soup How many times will Anna need to fill the measuring cup to measure 7/8 cups of turnips?
Answers
3 1/2 times Anna needs to fill the measuring cup.
How many times does Anna need to fill the cup?Given,
She is using a 1/4 cup measuring cup to measure 7/8 cup of turnips
Solution:
Since she is using a 1/4 cup measuring cup, divide 7/8 cups by 1/4.
7/8 /1/4 = 7/8 * 4/1
= 7/2
= 3 1/2 times.
Therefore,
3 1/2 times Anna needs to fill the measuring cup.
To learn more about measuring cups, refer
https://brainly.com/question/20966276
#SPJ9
given the sets {5, 7, 9}, {1, 11, 4}, {2, 3, 6, 8, 10}, what are the resulting disjoint sets after the operation union(7, 4}?
Answers
The resulting disjoint sets after the operation union({7, 4}) would be: {5, 7, 9, 1, 11, 4, 2, 3, 6, 8, 10}.
The union of two sets A and B is denoted by A ∪ B and is defined as the set of all elements that are either in A or in B or in both. Therefore, the resulting disjoint sets after the operation union({7, 4}) would be:
{5, 7, 9, 1, 11, 4, 2, 3, 6, 8, 10}.
To calculate the union, we can use the following formula:
A ∪ B = {x : x ∈ A or x ∈ B}
Therefore, the union of {7, 4} can be calculated as:
{7, 4} ∪ {7, 4} = {x : x ∈ {7, 4} or x ∈ {7, 4}}
= {7, 4}
Therefore, the resulting disjoint sets after the operation union({7, 4}) would be: {5, 7, 9, 1, 11, 4, 2, 3, 6, 8, 10}.
Learn more about disjoint sets here:
https://brainly.com/question/29278472
#SPJ4
Students rented buses and vans to go to the basketball game. There were 8
vans and 12 buses for 360 students. Write an equation that models this situation
where x is the number of students that fits on each van and y is the number of
students that fits on each bus.
Answers
Answer:
22
Step-by-step explanation:

the area of a trapezoidal cornfield iowa is 18000 sq m. the 100-meter side io is parallel to the 150-meter side wa. this field is divided into four sections by diagonal roads iw and oa. find the areas of the triangular sections.
Answers
The areas of the four triangular sections in the trapezoidal cornfield in Iowa are: Section 1: 7200 sq m, Section 2: 10800 sq m, Section 3: 10800 sq m, Section 4: Approximately 14988.86 sq m
To find the areas of the triangular sections, we need to first find the lengths of the diagonals IW and OA.
Given that IO is parallel to WA, we can use the properties of trapezoids to find the length of the diagonal IW.
Since the area of the trapezoidal field is 18000 sq m, we can use the formula for the area of a trapezoid:
Area = (a + b) * h / 2
where a and b are the lengths of the parallel sides, and h is the height (the distance between the parallel sides).
In this case, a = 100 m, b = 150 m, and the area = 18000 sq m. We can plug these values into the formula and solve for the height:
18000 = (100 + 150) * h / 2
36000 = 250 * h
h = 36000 / 250
h = 144 m
Now that we have the height, we can use the properties of triangles to find the length of the diagonal IW.
In a right triangle with one leg being the height (h) and the hypotenuse being the diagonal (IW), we can use the Pythagorean theorem:
IW² = h² + b²
IW² = 144² + 100²
IW² = 20736 + 10000
IW² = 30736
IW ≈ 175.21 m
Similarly, we can find the length of the diagonal OA using the same steps:
OA² = h² + a²
OA² = 144² + 150²
OA² = 20736 + 22500
OA²= 43236
OA ≈ 207.93 m
Now, we can find the areas of the triangular sections.
Section 1: Triangle IOW
The base is IO, which is 100 m, and the height is h, which is 144 m. Using the formula for the area of a triangle:
Area1 = (base * height) / 2
Area1 = (100 * 144) / 2
Area1 = 7200 sq m
Section 2: Triangle IWA
The base is WA, which is 150 m, and the height is h, which is 144 m. Using the formula for the area of a triangle:
Area2 = (base * height) / 2
Area2 = (150 * 144) / 2
Area2 = 10800 sq m
Section 3: Triangle OWA
The base is WA, which is 150 m, and the height is h, which is 144 m. Using the formula for the area of a triangle:
Area3 = (base * height) / 2
Area3 = (150 * 144) / 2
Area3 = 10800 sq m
Section 4: Triangle OAI
The base is OA, which is approximately 207.93 m, and the height is h, which is 144 m. Using the formula for the area of a triangle:
Area4 = (base * height) / 2
Area4 = (207.93 * 144) / 2
Area4 ≈ 14988.86 sq m
The areas of the triangular sections are as follows:
- Section 1: 7200 sq m
- Section 2: 10800 sq m
- Section 3: 10800 sq m
- Section 4: Approximately 14988.86 sq m
To find the areas of the triangular sections in the trapezoidal cornfield in Iowa, we first need to find the lengths of the diagonals IW and OA. By using the formula for the area of a trapezoid, we can determine the height of the trapezoid, which is 144 m. Using the Pythagorean theorem, we can find the lengths of the diagonals IW and OA. IW is approximately 175.21 m, while OA is approximately 207.93 m. Next, we can calculate the areas of the triangular sections. Section 1 has a base of 100 m and a height of 144 m, resulting in an area of 7200 sq m. Section 2 and Section 3 both have a base of 150 m and a height of 144 m, resulting in areas of 10800 sq m each. Finally, Section 4 has a base of approximately 207.93 m and a height of 144 m, resulting in an area of approximately 14988.86 sq m.
In conclusion, the areas of the four triangular sections in the trapezoidal cornfield in Iowa are:
- Section 1: 7200 sq m
- Section 2: 10800 sq m
- Section 3: 10800 sq m
- Section 4: Approximately 14988.86 sq m
To Know More about resulting visit:
brainly.com/question/27751517
#SPJ11
FILL IN THE BLANK a(n) ____ consists of a rectangle divided into three sections.
Answers
Answer:
Step-by-step explanation:4
Assume tuition at Penn State cost $6,142 (per semester) in 2007 and $7,562 in 2012. If the price index was 207.34 in 2007 and 226 in 2012, then we could say:
Answers
Based on the given information, we can conclude that tuition at Penn State has increased more rapidly than inflation.
The price index measures the average change in prices of a basket of goods and services over time. In this case, the price index increased from 207.34 in 2007 to 226 in 2012, indicating an overall inflation rate of approximately 9% over that period. On the other hand, tuition at Penn State increased from $6,142 to $7,562, representing an increase of approximately 23%.
When comparing the rates of increase, we can see that tuition has outpaced inflation. This implies that the cost of education at Penn State has been rising more rapidly than the general increase in prices for goods and services. It suggests that factors specific to the education industry, such as rising costs of resources, facilities, and personnel, have contributed to the higher tuition rates.
Learn more about inflation here:
https://brainly.com/question/31257026
#SPJ11
The complete question is:
Assume tuition at Penn State cost $6,142 (per semester) in 2007 and $7,562 in 2012. If the price index was 207.34 in 2007 and 226 in 2012, then we could say:
has increased more slowly than inflation.suffers from menu costs due to inflation,has increased more rapidly than inflation.has increased at about the same rate as inflation.is an inferior good.f(x)=log5x what Is the range of the function
Answers
The range of the function f(x) = log5x is (-∞, +∞).The function f(x) = log5x represents the logarithm base 5 of x. To determine the range of this function, we need to consider the possible values that the logarithm can take.
The range of the logarithm function y = log5x consists of all real numbers. The logarithm function is defined for positive real numbers, and as x approaches 0 from the positive side, the logarithm approaches negative infinity. As x increases, the logarithm function approaches positive infinity.
The range of the function is the set of all possible output values. In this case, the range consists of all real numbers that can be obtained by evaluating the logarithm
log5(�)log 5 (x) for �>0 x>0.
Since the base of the logarithm is 5, the function log5x will take on all real values from negative infinity to positive infinity. Therefore, the range of the function f(x) = log5x is (-∞, +∞).
In other words, the function can output any real number, ranging from negative infinity to positive infinity. It does not have any restrictions on the possible values of its output.
For more such questions on Logarithm:
https://brainly.com/question/31117877
#SPJ8
Answer: All real numbers
Step-by-step explanation:
Edge
Select all the correct answers.
Which two surfaces need NOT be sanitized between the two tasks?
a cutting board used to first slice bananas and then dice them
a grater used to first grate carrots and then cheese
a prep table used to first cut meat and then make sandwiches
a cup used first to measure sugar and then flour
a knife used to first filet fish and then slice ham
Answers
The two surfaces that need NOT be sanitized between the two tasks are:
A cup used first to measure sugar and then flour.
A knife used to first filet fish and then slice ham.
In both cases, there is no risk of cross-contamination between allergens or harmful bacteria.
The two surfaces that need NOT be sanitized between the two tasks are:
A cup used first to measure sugar and then flour.
A knife used to first filet fish and then slice ham.
In both cases, there is no risk of cross-contamination between allergens or harmful bacteria. The cup is being used for dry ingredients (sugar and flour), which pose a minimal risk of contamination. Similarly, the knife is being used on two different types of proteins (fish and ham), but as long as it is properly cleaned after use, there is no immediate risk of cross-contamination.
For such more questions on Sanitizing Surfaces Not Required
https://brainly.com/question/28974722
#SPJ11
What is the average rate of change of f(x) = −x2 + 3x + 6 over the interval −3 ≤ x ≤ 3? A. −2 B. −1 C. 3 D. 6
Answers
A function is a relation between a set of inputs and a set of possible outputs, where each input is uniquely associated with a single output.the average rate of change of f(x) over the interval \([-3, 3]\) is:
\((6 - (-12)) / 6 = 18/6 = 3\) Thus, option C is correct.
What is the average rate of change of f(x)?The average rate of change of a function over an interval is given by the difference in the function values at the endpoints of the interval, divided by the length of the interval.
Therefore, to find the average rate of change of \(f(x) = − + 3x + 6\) over the interval \(−3 ≤ x ≤ 3\), we need to evaluate the function at the endpoints of the interval and then divide by the length of the interval.
\(f(-3) = + 3(-3) + 6 = -9 - 9 + 6 = -12\)
\(f(3) = + 3(3) + 6 = -9 + 9 + 6 = 6\)
The length of the interval is 3 - (-3) = 6.
Therefore, the average rate of change of f(x) over the interval \([-3, 3]\) is:
\((6 - (-12)) / 6 = 18/6 = 3\)
Learn more about function here:
brainly.com/question/12431044
#SPJ1
In triangle QRS, the measure of angle RSQ is 29.1*, and the measure of angle
QRS is 76*. What is the measure of angle SQR?*
Answers
Answer:
74.9 degrees
Step-by-step explanation:
All angles in a triangle must add up to 180 degrees. Add 29.1 and 76 to get 105.1, and then you can subtract that from 180 to get 74.9.
is x/3 = 5/6, then x =
Answers
X=(5/6)/⅓
=5/2
find the slope of the line 3x-5y =0
Answers
Answer:
slope= ⅗
Step-by-step explanation:
Rewrite the equation into the slope-intercept form, y=mx +c, where m is the gradient and c is the y-intercept.
3x- 5y= 0
+5y on both sides of the equation:
5y= 3x
Divide both sides by 5:
y= ⅗x
From the coefficient of x, the slope of the line is ⅗.